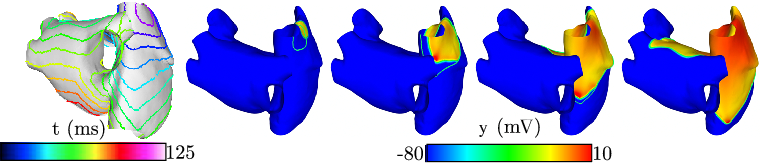
Section: New Results

Model-Data Interaction

A Luenberger observer for reaction-diffusion models with front position data

Participants : Dominique Chapelle, Annabelle Collin, Philipe Moireau [correspondant] .

We propose a Luenberger observer for reaction-diffusion models with propagating front features, and for data associated with the location of the front over time. Such models are considered in various application fields, such as electrophysiology, wild-land fire propagation and tumor growth modeling. Drawing our inspiration from image processing methods, we start by proposing an observer for the eikonal-curvature equation that can be derived from the reaction-diffusion model by an asymptotic expansion. We then carry over this observer to the underlying reaction-diffusion equation by an "inverse asymptotic analysis", and we show that the associated correction in the dynamics has a stabilizing effect for the linearized estimation error. We also discuss the extension to joint state-parameter estimation by using the earlier-proposed ROUKF strategy. We then illustrate and assess our proposed observer method with test problems pertaining to electrophysiology modeling, including with a realistic model of cardiac atria. Our numerical trials show that state estimation is directly very effective with the proposed Luenberger observer, while specific strategies are needed to accurately perform parameter estimation – as is usual with Kalman filtering used in a nonlinear setting – and we demonstrate two such successful strategies.

Identification of weakly coupled multiphysics problems. Application to the inverse problem of electrocardiography

Participants : Cesare Corrado [Reo team] , Jean-Frédéric Gerbeau [Reo team] , Philippe Moireau [correspondant] .

This work addresses the inverse problem of electrocardiography from a new perspective, by combining electrical and mechanical measurements. Our strategy relies on the definition of a model of the electromechanical contraction which is registered on ECG data but also on measured mechanical displacements of the heart tissue typically extracted from medical images. In this respect, we establish in this work the convergence of a sequential estimator which combines for such coupled problems various state of the art sequential data assimilation methods in a unified consistent and efficient framework. Indeed, we aggregate a Luenberger observer for the mechanical state and a Reduced-Order Unscented Kalman Filter applied on the parameters to be identified and a POD projection of the electrical state. Then using synthetic data we show the benefits of our approach for the estimation of the electrical state of the ventricles along the heart beat compared with more classical strategies which only consider an electrophysiological model with ECG measurements. Our numerical results actually show that the mechanical measurements improve the identifiability of the electrical problem allowing to reconstruct the electrical state of the coupled system more precisely. Therefore, this work is intended to be a first proof of concept, with theoretical justifications and numerical investigations, of the advantage of using available multi-modal observations for the estimation and identification of an electromechanical model of the heart.

Data assimilation for hyperbolic conservation laws. A Luenberger observer approach based on a kinetic description

Participants : Anne-Céline Boulanger [Ange team] , Benoît Perthame [Mamba team] , Philippe Moireau [correspondant] , Jacques Sainte-Marie [Ange team] .

Developing robust data assimilation methods for hyperbolic conservation laws is a challenging subject. Those PDEs indeed show no dissipation effects and the input of additional information in the model equations may introduce errors that propagate and create shocks. We propose a new approach based on the kinetic description of the conservation law. A kinetic equation is a first order partial differential equation in which the advection velocity is a free variable. In certain cases, it is possible to prove that the nonlinear conservation law is equivalent to a linear kinetic equation. Hence, data assimilation is carried out at the kinetic level, using a Luenberger observer also known as the nudging strategy in data assimilation. Assimilation then amounts to the handling of a BGK type equation. The advantage of this framework is that we deal with a single “linear” equation instead of a nonlinear system and it is easy to recover the macroscopic variables. The study is divided into several steps and essentially based on functional analysis techniques. First we prove the convergence of the model towards the data in case of complete observations in space and time. Second, we analyze the case of partial and noisy observations. To conclude, we validate our method with numerical results on Burgers equation and emphasize the advantages of this method with the more complex Saint-Venant system.

Optimal observer for parabolic problems

Participants : Karine Mauffrey, Philippe Moireau [correspondant] .

We aim at proposing optimal observers strategies for reconstructing the solution of general systems of PDEs using available observations, including both wave-type equations and heat-like equations. The main objective of this work is to present a self-contained analysis. For a general parabolic system, we have established the exponential stability of the operator occurring in the equation satisfied by the error between the target and the optimal observer. The proof relies on two major hypotheses: an observability inequality satisfied by the observation operator and a controllability property for the modeling error operator by which model noises enter the dynamics (controllability property which is related to the invertibility of the solution of the associated infinite dimensional Riccati equation). Then we have tackled the discretisation questions and demonstrated that the discrete-time Kalman filter is an adequate discretization of the continuous-time Kalman filter. Finally we have also studied the strong formulation of the Kalman observer using a kernel representation of the Riccati operator.

Elastography by magnetic resonance imaging

Participants : Guillaume Bal [Columbia Unviersity] , Cedric Bellis [LMA Marseille] , Sébastien Imperiale [correspondant] , Francois Monard [University of Washington- Seattle] .

We have studied the potential application of elastography by Magnetic Resonance Imaging (MRI) within the framework of linear elasticity. We assume given internal full-field MRI measurements of the deformations of a non-homogeneous isotropic solid, and the aim is the quantitative reconstruction of the associated physical parameters. Upon using polluted measurements, a variational formulation is constructed, its inversion enabling the recovery of the parameters. The analysis of this inversion procedure provides existence and uniqueness results while the reconstruction stability with respect to the measurements is investigated. As the inversion procedure requires differentiating the measurements twice, a numerical differentiation scheme has been proposed and analyzed. It is based on a regularization that allows an optimally stable reconstruction of the sought parameters.