Keywords
Computer Science and Digital Science
- A5.9.2. Estimation, modeling
- A6.4.1. Deterministic control
- A6.4.4. Stability and Stabilization
- A6.4.5. Control of distributed parameter systems
- A9.5. Robotics
Other Research Topics and Application Domains
- B1.1.8. Mathematical biology
- B2.1. Well being
- B5.6. Robotic systems
- B7.2.1. Smart vehicles
1 Team members, visitors, external collaborators
Research Scientists
- Denis Efimov [Team leader, Inria, Researcher, HDR]
- Leonid Fridman [Inria, Chair, until Jan 2022, HDR]
- Andrey Polyakov [Inria, Researcher, HDR]
- Rosane Ushirobira [Inria, Researcher, HDR]
Faculty Member
- Jean-Pierre Richard [Centrale Lille, Professor]
Post-Doctoral Fellow
- Gabriele Perozzi [Inria, from Jul 2020]
PhD Students
- Youness Braidiz [Centrale Lille, until Oct 2020]
- Nelson De Figueiredo Barroso [Inria]
- Deesh Dileep [Centrale Lille, until Feb 2020]
- Alex Dos Reis De Souza [Inria]
- Anatolii Khalin [Inria]
- Wenjie Mei [China Scholarship Council]
- Artem Nekhoroshikh [Centrale Lille and ITMO University, Russia]
- Haik Jan Silm [Centrale Lille and KULeuven , Belgium, until Jun 2020]
- Jijju Thomas [Centrale Lille and TU Eindhoven, The Netherlands]
- Quentin Voortman [Centrale Lille and TU Eindhoven, The Netherlands]
- Siyuan Wang [China Scholarship Council]
- Yu Zhou [China Scholarship Council, from Nov 2020]
Technical Staff
- Fiodar Hancharou [Inria, Engineer]
Administrative Assistants
- Nathalie Bonte [Inria, until Mar 2020]
- Amelie Supervielle [Inria, from Mar 2020]
Visiting Scientists
- Rakesh Kumar [Indo-French Centre for the Promotion of Advanced Research, India, from Mar 2020 until May 2020]
- Junfeng Zhang [Hangzhou Dianzi University, China, until Aug 2020]
External Collaborator
- Gerald Dherbomez [CNRS, Engineer]
2 Overall objectives
Valse team studies the estimation and control problems arising in the analysis and the design of distributed, uncertain and interconnected dynamical systems:
- Using the concepts of finite-time/fixed-time/hyperexponential convergence and stability, the main idea is to separate and hierarchize in time the control and estimation processes, which are distributed in space. This greatly simplifies their analysis and the design for large-scale solutions.
- The main areas of investigation and application are Internet of things and cyber-physical systems.
- The team aims to draw up algorithms for decentralized finite-time control and estimation. The methodology to be developed includes extensions of the theory of homogeneous systems and of finite-time/fixed-time/hyperexponential convergence and stability notions. A particular attention is given to applications in real-world scenarios.
- It is a joint proposal with the CNRS CRIStAL UMR 9189 (Centrale Lille and Université de Lille).
3 Research program
Valse team works in the domains of control science: dynamical systems, stability analysis, estimation, and automatic control. Our developments are focused on the theoretical and applied aspects related to control and estimation of large-scale multi-sensor and multi-actuator systems based on the use of the theories of finite-time/fixed-time/hyperexponential convergence and homogeneous systems. The Lyapunov function method and other methods of analysis of dynamical systems form a basis for the studies in Valse team.
The key idea of the research program for the team is that a fast (non-asymptotic) convergence of the regulation and estimation errors increases the reliability of intelligent distributed actuators and sensors in complex scenarios, such as interconnected cyber-physical systems (CPSs).
The expertise of Valse's members in theoretical developments of control and estimation theory (finite-time control and estimation algorithms in centralized context 118, 104, 115, 114, 111, homogeneity framework for differential equations 119, 106, 105, 107, 109, 120, 116, time-delay systems 108, 110, 123, distributed systems 117 and algebraic-based methods for estimation 121, 122) is an essential ingredient to achieve our objective.
The generic chart of different goals and tasks included in the scientific work program of Valse, and interrelations between them are presented in Fig. 1. We have selected three main objectives to pursue with the related tasks to fulfill:
- The first objective consists in design of control and estimation solutions for CPS and IoT, which is the principal aim of Valse, it will contain the main outcomes of our research.
- The second objective is more theoretical, which is needed to make the basement for our design and analysis parts in the previous goal.
- The third objective deals with applications, which will drive the team and motivate the theoretical studies and selected design performances.
All these objectives are interconnected: from a particular problem in an IoT application, it is planned to design a control or estimation algorithm, which leads to the development of theoretical tools; and vice versa, a new theoretical advance can provide a possibility for the development of novel tools, which can be used in applications.
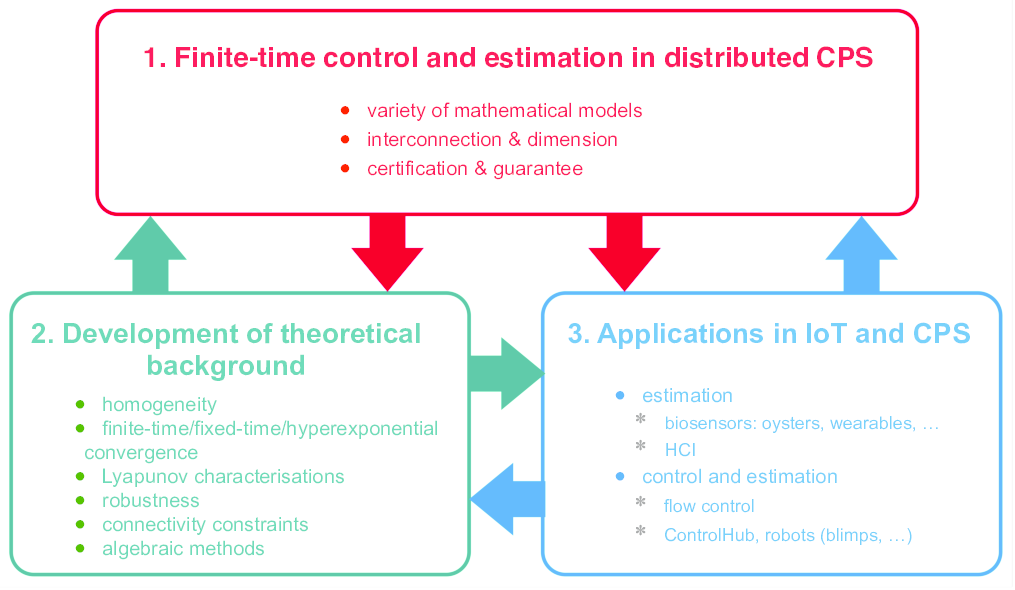
Structure of the objectives and tasks treated in Valse
To explain our motivation: why to use finite-time? Applying any method for control/estimation has a price in terms of its advantages and disadvantages. There is no universal framework that is the best always and everywhere. Finite-time may appear as a luxurious property for a physical system, requiring the use of nonlinear tools. Of course, if an asymptotic convergence and a linear model are enough for solving a given problem, then there is no reason to develop something else. However, most of the present problems in CPS and IoT are nonlinear (i.e. they have various local behaviors that cannot be collected in only one linear model). Design and analysis of various local linearized models and solutions are luxurious, too. The theory of homogeneity can go beyond linearity offering many new features while not appearing as severe as other nonlinear tools and having almost all hints of the linear framework. Suppose that, thanks to the homogeneity theory, finite-time/fixed-time can be obtained with limited difficulty while adding the bonuses of stronger robustness and faster convergence compared to the linear case? We are convinced that the price of going beyond linear control and estimation can be strongly dropped down by maturing the theory of homogeneity and finite/fixed-time convergence. We are also convinced that it will be compensated in terms of robustness and speed, which can be demanded in the new areas of application as IoT, for example.
4 Application domains
An objective of the team is the application of the developed control and estimation algorithms for different scenarios in IoT or CPSs. The participation in various potential applications allows Valse team to better understand the features of CPSs and their required performances, and to formulate properly the control and estimation problems that have to be solved. Here is a list of ongoing and potential applications addressed in the team:
- smart bivalve-based biosensor for water quality monitoring (ANR project
WaQMoS, the developed
sensor is shown in Fig. 2): in living beings,
the presence of persistent external perturbations may be difficult
to measure, and important model uncertainties render the application
of conventional techniques complicated; another issue for estimation
is the consensus-seeking between animals for a contamination detection 102;

Figure 2: The valvometer used in ANR project WaQMoS
- control and estimation for flying vehicles, e.g. quadrotors
or blimps given in Fig. 3 (PhD Centrale Lille): nonlinearity of the model and its
uncertainty coupled with important aerodynamic perturbations have
to be compensated by fast (finite- or fixed-time) and robust control
and estimation algorithms;

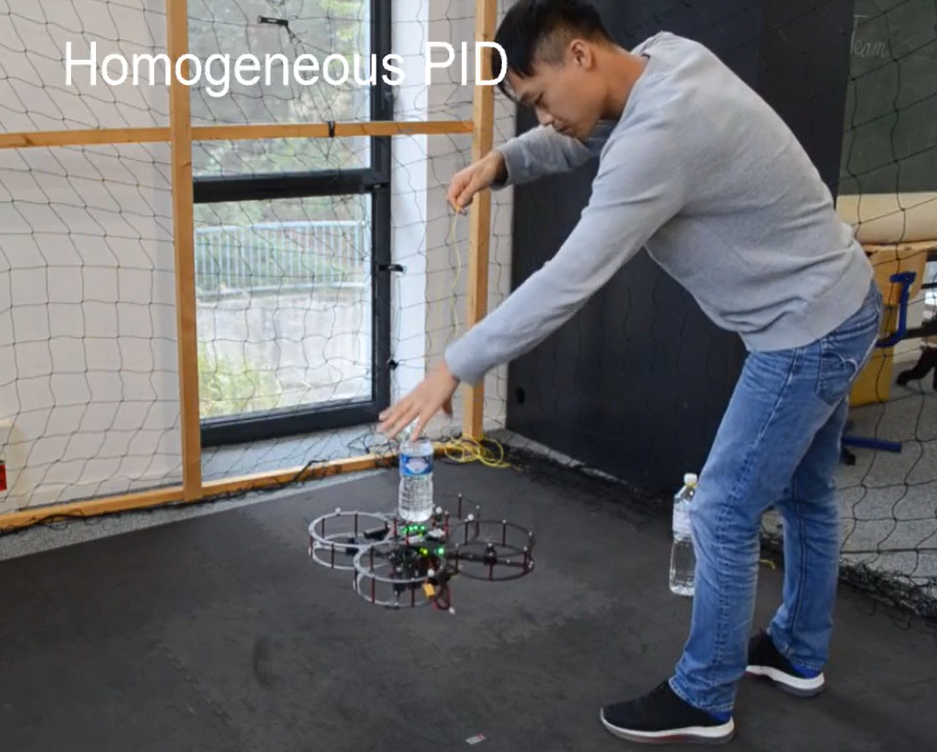
Figure 3: Blimp and quadrotor robots
- human behavior modeling and identification with the posterior design of algorithms for human-computer interaction (ANR project TurboTouch): robust finite-time differentiators demonstrate good estimation capabilities needed for prediction in this application 122, 103;
- human physiological characteristics estimation (like emotion detection, galvanic skin response filtering, fatigue evaluation in collaborations with Neotrope and Ellcie Healthy): intelligent robust filtering and finite-time distributed estimation are key features in these scenarios;
- path planning for autonomous vehicles taking into account the behavior of humans (PhD CIFRE with SEQUEL/SCOOL team and Renault): application of interval and finite-time adaptive estimation and prediction techniques allows for treating the uncertainty of the environment by reducing the computational complexity of reinforcement learning 1131;
- flow control (in the framework of ContrATech subprogram of CPER ELSAT,
see also 112): the case of control and
estimation of a distributed-parameter system with very fast and uncertain
dynamics, where finite-time solutions developed by Valse are necessary (an example of results is given in Fig. 4);
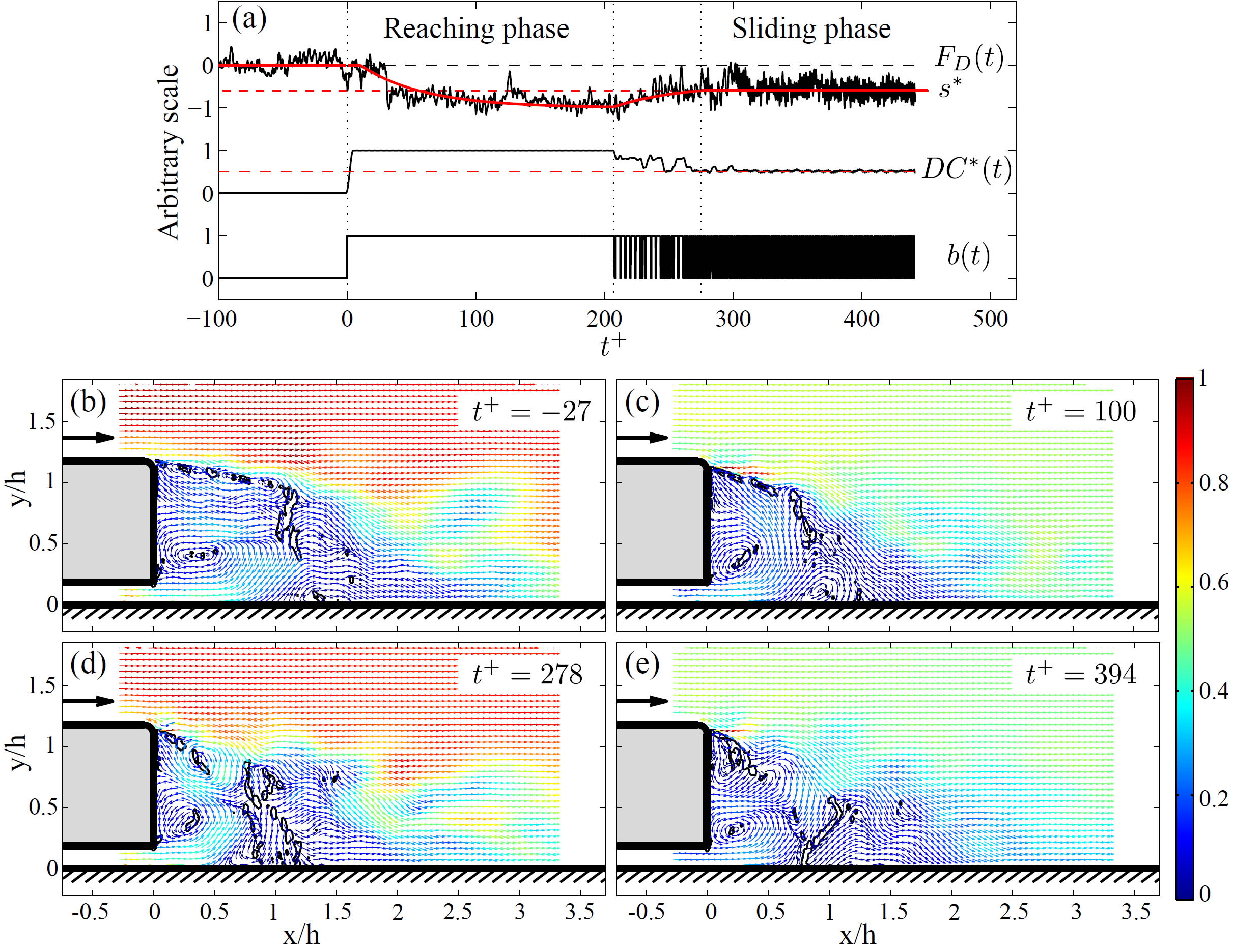
Figure 4: Particle Image Velocimetry on flow control for an Ahmed body (LAMIH wind tunnel)
- control of synthetic microbial communities (in the framework of IPL COSY, the experimental platform is shown in Fig. 5): here again, the problem is an important uncertainty of the model,
which can be handled by robust sliding mode control algorithms, or
by applying adaptive finite-time estimation and identification tools;
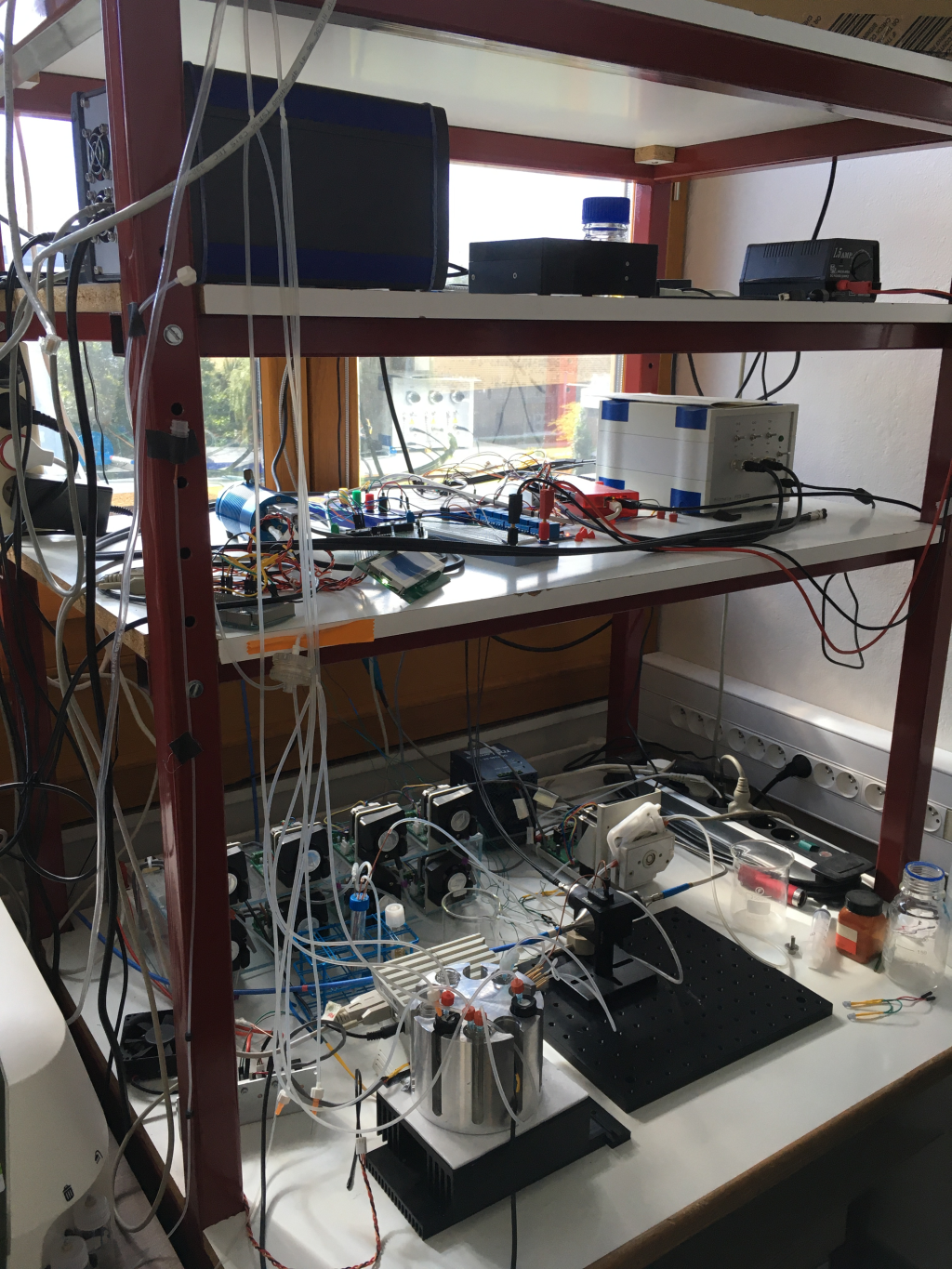
Figure 5: Chemostat platform at Inria, Grenoble
It is worth highlighting a widespread distribution of various scientific domains in the list of applications for the team given above. Such an interdisciplinarity for Valse is unsurprising, since the control theory is a science of systems whose interest today is, by nature, to interface with other disciplines and their fields of application. This is also well aligned with the domain of CPSs, which by its origin requires multidisciplinary competencies.
5 Highlights of the year
- This year Valse published 6 papers in Automatica and 6 in IEEE Transaction on Automatic Control (the top journals in the domain of control theory).
- A report96 on interval prediction of COVID-19 outbreak development with taking into account the societal feedback was written in April 2020, and it was downloaded (all versions in total) more than 2000 times.
- End of the UCoCoS project: The European Commission has approved the final report and the financial statements of UCoCoS, H2020 ITN with TU Eindhoven and KU Leuven. The team still has 2 PhD students supported by the remaining funds, they will defend their theses in 2021 (PhD duration in The Netherlands must be 4 years at least).
- ControlHub, which is the platform for on-line testing the control and estimation algorithms, was opened for beta testing this year.
6 New software and platforms
6.1 New platforms
ControlHub is the platform for the rapid prototyping of control algorithms of cyber-physical systems. It allows the End-User to have remote access to real experimental setups and to validate mathematical control and estimation algorithms.
7 New results
7.1 Interval prediction for COVID-19 outbreak
Participants: Rosane Ushirobira, Denis Efimov.
In 96, 27, a new version of the well-known epidemic mathematical SEIR model is used to analyze the pandemic course of COVID-19 in eight different countries. One of the proposed model’s improvements is to reflect the societal feedback on the disease and confinement features. The SEIR model parameters are allowed to be time-varying, and the ranges of their values are identified by using publicly available data for France, Italy, Spain, Germany, Brazil, Russia, New York State (US), and China. The identified model is then applied to predict the SARS-CoV-2 virus propagation under various conditions of confinement. For this purpose, an interval predictor is designed, allowing variations and uncertainties in the model parameters to be taken into account. The code and the utilized data are available on Github.
7.2 Distributed systems
Participants: Rosane Ushirobira, Denis Efimov, Jean-Pierre Richard.
In 4, the distributed estimation problem is solved for continuous-time observer nodes that obtain real-time measurements, but communicate with their neighbors over a communication network. To this end, the digital communication between the observer nodes is modeled by the time-delay approach, where variable sampling intervals, transmission delays and packet dropouts are taken into account. A Linear Matrix Inequality (LMI) for the design of the observer gains is derived using Halanay’s inequality, the feasibility of which guarantees exponential stability with a selected convergence rate up to a maximum total delay. A comparison of the maximal delay on a numerical example shows the advantage of a distributed observer over a centralized one.
In 18, a methodology is proposed to design sampled-data fixed-order decentralized controllers for Multiple Input Multiple Output (MIMO) linear time-invariant time-delay systems. Imperfections in the communications links between continuous-time plants and controllers arising due to transmission time-delay, aperiodic sampling, and asynchronous sensors and actuators are considered. A frequency domain-based direct optimization approach towards controller design is proposed. The methodology has been implemented in a publicly available software, which supports system models in terms of delay differential-algebraic equations. The effectiveness of the methodology is illustrated using a numerical example. The paper 45 deals with exponential stability analysis of decentralized, sampled-data, linear time-invariant systems with asynchronous sensors and actuators. We consider the case where each controller in the decentralized setting has its own sampling and actuation frequency, which translates to asynchrony between sensors and actuators. Additionally, asynchrony may be induced by delays between the sampling instants and actuation update instants as relevant in a networked context. By characterizing the properties of the operators using small-gain type integral quadratic constraints, we provide criteria for exponential stability of the asynchronous, decentralized state-space models. This group of results is obtained in collaboration with SHOC team of CRIStAL lab of CNRS.
A design of a distributed observer is proposed in 41 for continuous-time systems with nonlinear observer nodes such that the estimation errors converge in a finite time to zero. By taking advantage of individual observability decompositions, the designs for the locally observable and the unobservable substate are made independent from each other. For the observable substate of each node, standard centralized finite-time observer techniques are applied. To estimate distributively the unobservable substate, the observer nodes employ consensus coupling in a linear term and an additional term embedded in a fractional power. The approach is derived using homogeneity arguments, and it leads to a simple design with an LMI that is guaranteed to be feasible under general conditions.
7.3 Consistent discretisation of homogeneous systems
Participants: Andrey Polyakov, Denis Efimov.
In 38, a discretisation scheme for asymptotically stable homogeneous systems is proposed. This scheme exploits the information provided by a homogeneous Lyapunov function of the system. The main features of the scheme are: 1) the discretisation method is explicit and; 2) the discrete-time system preserves the asymptotic stability, the convergence rate, and the Lyapunov function of the original continuous-time system.
The paper 14 deals with the analysis of the time-discretization of the super-twisting algorithm, with an implicit Euler method. It is shown that the discretized system is well-posed. The existence of a Lyapunov function with convex level sets is proved for the continuous-time closed-loop system. Then the global asymptotic Lyapunov stability of the unperturbed discrete-time closed-loop system is proved. The convergence to the origin in a finite number of steps is proved also for the unperturbed case. Numerical simulations demonstrate the superiority of the implicit method with respect to an explicit discretization with significant chattering reduction.
A survey on this subject can be found in 13.
Two latter works are done in collaboration with TRIPOP team of Inria.
7.4 Chemostat control and estimation
Participants: Denis Efimov, Andrey Polyakov.
In 21, the problem of state estimation of a bioreactor containing a single substrate and several competing species is studied. This scenario is well-known as the competition model, in which multiple species compete for a single limiting nutrient. Considering the total biomass to be the only available measurement, the challenge is to estimate the concentration of the whole state vector. To achieve this goal, the estimation scheme is built by the coupling of two estimation techniques: an asymptotic observer, which depends solely on the operating conditions of the bioreactor, and a finite-time parameter estimation technique, which drops the usual requirement of the persistence of excitation. The presented methodology achieves the estimation of each competing species.
Continue in the same line, the problem of robust stabilization of the concentrations of two different species of living organisms, which compete for a single limiting substrate in a bioreactor, is considered in 20. This stabilization is performed using discontinuous feedback control laws that ensure the coexistence of all species. The control laws are designed considering bounded parametric uncertainties on the kinetic rates.
7.5 Analysis and design of homogeneous and finite-time stable systems
Participants: Andrey Polyakov, Denis Efimov.
The work 11 investigates robustness of finite-time stability property for a homogeneous nonlinear dynamical system with sufficiently small affine inputs. In addition, robust stability conditions are presented for the systems admitting homogeneous approximations at the origin or at infinity. The effects of additional stable unmodeled dynamics in the input channel on robust stability are investigated. The utility of the obtained results is illustrated via robustness analysis of homogeneous observer with time-varying gains.
In 54, a robust nonlinear control is designed for stabilizing linear MIMO systems. The presented control law homogenizes a linear system (without its transformation to a canonical form) with a specified degree and stabilizes it in a finite time (or with a fixed-time attraction to any compact set containing the origin) if the degree of homogeneity is negative (positive). The tuning procedure is formalized in LMI form. Performance of the approach is illustrated by simulations and experiments.
The paper 31 gathers the analysis of non-asymptotic convergence rates (finite-time and fixed-time) with the property of Input-to-State Stability (ISS). Theoretical tools to determine this joint property are presented for the case where an explicit ISS Lyapunov function is known, and when it remains in implicit form (e.g. as a solution of an algebraic equation). For the case of finite-time ISS, necessary and sufficient conditions are given whereas for the fixed-time case only a sufficient condition is obtained.
7.6 Adaptive finite/fixed-time estimation
Participants: Denis Efimov.
The paper 28 deals with the problem of adaptive estimation, i.e. the simultaneous estimation of the state and time-varying parameters, in the presence of measurement noise and state disturbances, for a class of uncertain nonlinear systems. An adaptive observer is proposed based on a nonlinear time-varying parameter identification algorithm and a sliding-mode observer. The nonlinear time-varying parameter identification algorithm provides a fixed-time rate of convergence to a neighborhood of the origin, while the sliding-mode observer ensures ultimate boundedness for the state estimation error attenuating the effects of the external disturbances. LMIs are provided for the synthesis of the adaptive observer, while the convergence proofs are given based on the Lyapunov and ISS theories.
In papers 5, 48, the problem of estimation in the linear regression model is studied under the hypothesis that the regressor may be excited on a limited initial interval of time only. The estimation solution is searched on a finite interval of time based on the framework of finite-time or fixed-time converging dynamical systems. The robustness issue is analyzed, and a short-time input-to-state stability property is introduced for finite/fixed-time converging time-varying systems with a sufficient condition, which is formulated with the use of a Lyapunov function. Several estimation algorithms are proposed and compared with existing solutions.
7.7 Model for pointing tasks with computer mouse
Participants: Rosane Ushirobira, Denis Efimov.
In 7, we studied one of the most fundamental human‐computer interaction issues: the pointing task. It can be described simply as reaching a target with a cursor starting from an initial position (e.g. executing a movement using a computer mouse to select an icon). A switched dynamic model is proposed to handle cursor movements in indirect pointing tasks. The model contains a ballistic movement phase (governed by a nonlinear model in Lurie form) and a corrective movement phase (described by a linear visual‐feedback system). The stability of the model is first established, and the derived model is then validated with experimental data acquired in a pointing task with a mouse. This work is done in collaboration with LOKI team of Inria.
7.8 Control of PDEs
Participants: Andrey Polyakov, Jean-Pierre Richard.
In 40, we propose a sliding mode controller for a MIMO model of flow separation in boundary layers. The model consists in a bilinear system with constant delays in both the state and the input. The main motivation to consider such a class of systems is that, as we have previously shown, it is suitable for input-output modelling and control design of some turbulent flow control systems. Stability and robustness properties of the control scheme are studied by means of Volterra equations theory, which provides easily verifiable stability conditions. The theoretical basics for this problem are given in 39, where a state-dependent switching controller for MIMO bilinear systems with constant delays in both the state and the input is proposed. The control input is assumed to be restricted to take only a finite number of values. The stability analysis of the closed-loop is based on a Lyapunov-Krasovskii functional, and the design is reduced to solve a system of LMIs. The controller can be designed by considering (state) delay-dependent or delay-independent conditions.
The existence of a locally Lipschitz continuous homogeneous Lyapunov function is proven for a class of asymptotically stable homogeneous infinite dimensional systems with unbounded nonlinear operators in 2.
A sliding mode control methodology to linear evolution equations with uncertain but bounded inputs and noise in observations is proposed in 52. We first describe the reachability set of the state equation in the form of an infinite-dimensional ellipsoid, and then steer the minimax center of this ellipsoid toward a finite-dimensional sliding surface in finite time by using the standard sliding mode output-feedback controller in equivalent form. We demonstrate that the designed controller is the best (in the minimax sense) in the class of all measurable functionals of the output.
7.9 Robot control and estimation
Participants: Denis Efimov, Andrey Polyakov.
In 51, a robust controller design for an indoor blimp robot is proposed for a surveillance application. The commonly used 6 degrees of freedom dynamic model is simplified under reasonable assumptions and decoupled into two independent parts. The blimp simplified horizontal plane movement model is complemented with disturbance terms to ensure the modeling accuracy, then it is transformed to a simpler form for the ease of controller design. Next, the disturbance terms are evaluated by the designed real-time estimator, and the perturbation estimates are compensated in the conceived motion controller for cancellation of the influence of disturbances. The performance and robustness of the disturbance compensation-based controller are verified by both simulations and experiments on the developed blimp robot.
The objective of 34 is to develop an algorithm for the estimation of time-varying wind parameters by taking into account a detailed quadrotor model. The design objectives include the time convergence optimization, robustness to measurement noises, and a guaranteed convergence of the estimates to the true values under mild applicability conditions. It is supposed that the estimation algorithm can use an inertial measurement unit (accelerometers, gyroscopes) sensors augmented with an earth reference tracking system and rotor rotational velocity sensors. To this end, three time-varying parameter estimation algorithms are introduced, compared and finally merged to estimate the varying wind velocity in on-board quadrotor systems.
In the work 50 a procedure for an “upgrade” of a linear PID controller to a non-linear homogeneous one is developed and verified by experiments with quadrotor. The controller design is based on a generalized homogeneity (dilation symmetry) of the system. Its parameters are obtained from the gains of linear controller. The issues of digital implementation of the proposed controller are discussed.
The works in this direction are done in collaboration with DEFROST team of Inria.
7.10 Control of passive multistable systems
Participants: Denis Efimov, Rosane Ushirobira.
In 9, the stability robustness with respect to external perturbations is investigated for the class of passive and strictly passive systems, which have several invariant compact and globally attracting subsets in the unforced scenario. It is assumed that the storage and supply rate functions are sign-definite with respect to these sets. The results are obtained within the framework of ISS and integral ISS for multistable systems. Two applications (related to the model of multispecies populations) of the proposed theory are used to illustrate its efficiency.
7.11 Analysis and design for time-delay systems
Participants: Denis Efimov.
The conditions of existence of a Lyapunov-Krasovskii functional (LKF) for nonlinear ISS neutral type systems are proposed in 25. The system under consideration depends nonlinearly on the delayed state and the delayed state derivative, and satisfies the conditions for the existence and uniqueness of the solutions. The LKF and the system properties are defined in a Sobolev space of absolutely continuous functions with bounded derivatives.
The paper 22 is devoted to stability analysis of homogeneous time-delay systems applying the Lyapunov-Krasovskii theory, and a generic structure of the functional is given that suits for any homogeneous system of non-zero degree (and can also be used for any dynamics admitting a homogeneous approximation). The obtained stability conditions are utilized to evaluate the domain of attraction for the delayed twisting control algorithm.
Considering a retarded nonlinear system, 1 proposes several modifications of the Lyapunov-Razumikhin approach guaranteeing the existence of an upper estimate on convergence rate of the system solutions. The cases of exponential, finite-time and fixed-time (with respect to a ball) convergences are studied.
7.12 Homogeneity for discrete-time systems
Participants: Denis Efimov, Andrey Polyakov.
In 3, we study robustness properties of discrete-time homogeneous systems. We also analyse stability and robustness properties of a more general class of nonlinear discrete-time systems that can be approximated by homogeneous ones. We apply the results to the investigation of stability properties of discretized continuous-time systems.
7.13 Adaptive stabilization by delay
Participants: Denis Efimov.
An output robust adaptive control is designed in 46 for a class of Lipschitz nonlinear systems, under assumption that the measurements are available with a constant bias and the state equations are linearly parameterized by unknown parameters and external disturbances. A dynamic state reconstruction (synthesis of an observer) is avoided by using delayed values of the output in the feedback and adaptation laws. The analysis of robust stability for the resulted time-delay system is performed by using the Lyapunov-Krasovskii approach. The control and adaptation gains can be selected as a solution of the proposed LMIs. This research is motivated by a nonlinear pendulum control problem, and the efficacy of the developed control is demonstrated on this application through experiments.
8 Partnerships and cooperations
8.1 International initiatives
8.1.1 Inria associate team not involved in an IIL
8.1.2 Inria international partners
8.2 European initiatives
The team is involved in 1 EU project UCoCoS (Understanding and Controlling of Complex Systems), coordinator W. Michiels (KUL, Belgium).
8.3 National initiatives
8.3.1 ANR
- Digitslid (Digital set-valued and homogeneous sliding mode control and differentiators: the implicit approach), coordinator B. Brogliato (Inria, Grenoble)
- Finite4SoS (Finite Time Control and Estimation for System of Systems), coordinator W. Perruquetti (École Centrale de Lille)
- WaQMoS (Coastal waters quality surveillance using bivalve mollusk-based sensors), coordinator D. Efimov (Inria, Lille)
8.3.2 Inria project labs
The team participates in IPL COSY (real-time COntrol of SYnthetic microbial communities), coordinator E. Cinquemani (Inria, Grenoble).
8.4 Regional initiatives
- ControlHub CPER Data, coordinator A. Polyakov
- “ContrATech” subprogram of CPER ELSAT, coordinator J.-M. Foucaut (LMFL)
9 Dissemination
9.1 Promoting scientific activities
9.1.1 Scientific events: selection
Member of the conference program committees
- J.-P. Richard, EUCA-IEEE ECC 2020, Saint Petersburg, Russia (18th European Control Conference, 1st virtual ECC) June 12-15, 2020
Reviewer
The members of the team participate in reviewing for all major international conferences and journals in the domain of control theory: IEEE Transactions on Automatic Control, Automatica, European Journal of Control, International Journal of Control, International Journal on Robust and Nonlinear Control, Asian Journal of Control, SIAM Journal on Control and Optimization, etc.
9.1.2 Journal
Member of the editorial boards
- R. Ushirobira, Guest editor, European Journal of Control
- D. Efimov, Guest editor, International Journal of Control
- D. Efimov, Guest editor, European Journal of Control
- D. Efimov, Guest editor, International Journal on Robust and Nonlinear Control
- D. Efimov, Associate editor, IFAC Journal on Nonlinear Analysis: Hybrid Systems
- D. Efimov, Associate editor, Asian Journal of Control
- D. Efimov, Associate editor, IEEE Transactions on Automatic Control
- D. Efimov, Associate editor, Automatica
9.1.3 Research administration
- J.-P. Richard, Director of the professional training “Researcher” for last year students at Centrale Lille
- R. Ushirobira, a member of the executive board of CIMPA
- D. Efimov, co-chair of International PhD Award in Systems & Control
9.2 Teaching - Supervision - Juries
9.2.1 Teaching
- Master: J.-P. Richard, Dynamical systems, 10h, M2, Université de Lille
- Licence: R. Ushirobira, Basic courses in Linear algebra and Calculus, 71h, L3, Polytech Lille
- Master: D. Efimov, Dynamical systems, 17h, M2, Université de Lille
- Licence: D. Efimov, Estimation for engineers, 24h, L3, Centrale Lille
9.2.2 Supervision
Defenses:
- PhD: Haik Silm, 07/2020
- PhD: Edouard Leurent, 10/2020
- PhD: Nelson de Figueiredo Barroso, 12/2020
- PhD: Youness Braidiz, 12/2020
- PhD: Siyaun Wang, 12/2020
9.2.3 Juries
- D. Efimov, CNAM, Paris
- A. Polyakov, INSA Lyon
- J.-P. Richard, Ecole Centrale de Nantes
- R. Ushirobira, Université de Lille
9.3 Popularization
- R. Ushirobira, D. Efimov, Inria fête de la science Si les huîtres pouvaient parler…
9.3.1 Internal or external Inria responsibilities
- R. Ushirobira, president of the Recruitment research committee of Inria Lille (PhD, postdoc, secondments, visitors)
- R. Ushirobira, officer in charge of scientific popularization of Inria Lille
10 Scientific production
10.1 Major publications
- 1 article On estimation of rates of convergence in Lyapunov-Razumikhin approach Automatica 116 2020
- 2 article Homogeneous Lyapunov Functions for Homogeneous Infinite Dimensional Systems with Unbounded Nonlinear Operators Systems and Control Letters 2020
- 3 article Discrete-Time Homogeneity: Robustness and Approximation Automatica 2020
- 4 article Distributed observers with time-varying delays IEEE Transactions on Automatic Control December 2020
- 5 articleFixed-time estimation of parameters for non-persistent excitationEuropean Journal of Control55September 2020, 24-32
- 6 article On necessary and sufficient conditions for output finite-time stability Automatica 2020
10.2 Publications of the year
International journals
International peer-reviewed conferences
Conferences without proceedings
Scientific books
Scientific book chapters
Edition (books, proceedings, special issue of a journal)
Doctoral dissertations and habilitation theses
Reports & preprints
10.3 Other
Patents
10.4 Cited publications
- 102 articleVelocity estimation of valve movement in oysters for water quality surveillanceIFAC-PapersOnLine482015, 333--338
- 103 conference Frequency Domain Forecasting Approach for Latency Reduction in Direct Human-Computer Interaction Proc. 56th IEEE Conference on Decision and Control (CDC) Melbourne 2017
- 104 articleRobust finite-time output feedback stabilization of the double integratorInternational Journal of Control8832015, 451--460
- 105 articleOn Homogeneity and Its Application in Sliding ModeInt. J. Franklin Institute35142014, 1866--1901
- 106 articleVerification of ISS, iISS and IOSS properties applying weighted homogeneitySystems & Control Letters622013, 1159--1167
- 107 articleOn conditions of oscillations and multi-homogeneityMathematics of Control, Signals, and Systems2812015, 1--37URL: http://dx.doi.org/10.1007/s00498-015-0157-y
- 108 articleDevelopment of Homogeneity Concept For Time-Delay SystemsSIAM Journal on Optimization and Control5232014, 1403--1808
- 109 articleRealization and Discretization of Asymptotically Stable Homogeneous SystemsIEEE Trans. Automatic Control62112017, 5962--5969
- 110 articleWeighted Homogeneity for Time-Delay Systems: Finite-Time and Independent of Delay StabilityIEEE Trans. Automatic Control6112016, 210--215
- 111 articleBoundary time-varying feedbacks for fixed-time stabilization of constant-parameter reaction-diffusion systemsAutomatica1032019, 398--407URL: https://doi.org/10.1016/j.automatica.2019.02.013
- 112 articleSISO model-based control of separated flows: Sliding mode and optimal control approachesInternational Journal of Robust and Nonlinear Control27182017, 5008-5027
- 113 conference Interval Prediction for Continuous-Time Systems with Parametric Uncertainties Proc. 58th IEEE Conference on Decision and Control (CDC) Nice 2019
- 114 articleFinite-time and Fixed-time Observer Design: Implicit Lyapunov function approachAutomatica8712018, 52-60
- 115 article On Homogeneous Finite-Time Control for Linear Evolution Equation in Hilbert Space IEEE Transactions on Automatic Control 2018
- 116 articleConsistent Discretization of Finite-time and Fixed-time Stable SystemsSIAM Journal on Optimization and Control5712019, 78--103
- 117 articleOn Homogeneous Distributed Parameter SystemsIEEE Trans. Automatic Control61112016, 3657--3662
- 118 articleFinite-time and fixed-time stabilization: Implicit Lyapunov function approachAutomatica512015, 332-340
- 119 articleNonlinear feedback design for fixed-time stabilization of linear control systemsIEEE Transactions on Automatic Control57(8)2012, 2106-2110
- 120 articleTime-Varying Parameter Identification Algorithms: Finite and Fixed-Time ConvergenceIEEE Transactions on Automatic Control6272017, 3671--3678URL: https://dx.doi.org/10.1109/TAC.2017.2673413
- 121 conference Estimating the infection rate of a SIR epidemic model via differential elimination Proceedings of ECC Naples 2019
- 122 conferenceA forecasting algorithm for latency compensation in indirect human-computer interactionsProceedings of ECCAlborg2016, 1081--1086
- 123 articleA note on delay robustness for homogeneous systems with negative degreeAutomatica7952017, 178--184

