Keywords
Computer Science and Digital Science
- A6. Modeling, simulation and control
- A6.2. Scientific computing, Numerical Analysis & Optimization
- A6.2.1. Numerical analysis of PDE and ODE
- A6.2.7. High performance computing
Other Research Topics and Application Domains
- B4. Energy
- B4.3.4. Solar Energy
- B5.3. Nanotechnology
- B5.5. Materials
- B8. Smart Cities and Territories
- B8.2. Connected city
1 Team members, visitors, external collaborators
Research Scientists
- Stephane Lanteri [Team leader, Inria, Senior Researcher, HDR]
- Théophile Chaumont-Frelet [Inria, Researcher]
- Mahmoud Elsawy [Inria, Starting Faculty Position]
- Frédéric Valentin [Inria, International Chair, Advanced Research Position]
Faculty Members
- Yves D Angelo [Univ Côte d’Azur, Professor, HDR]
- Stéphane Descombes [Univ Côte d'Azur, Professor, HDR]
- Claire Scheid [Univ Côte d'Azur, Associate Professor]
Post-Doctoral Fellows
- Josselin Defrance [Inria, from Feb 2021]
- Nicolas Lebbe [Inria]
- Patrick Vega Roman [Inria, until May 2021]
PhD Students
- Jeremy Grebot [École supérieure d'électricité, CIFRE]
- Zakaria Kassali [Inria]
- Thibault Laufroy [Univ Côte d'Azur]
- Massimiliano Montone [Inria]
Technical Staff
- Alexis Gobé [Inria, Engineer]
- Guillaume Leroy [Inria, Engineer]
Interns and Apprentices
- Enzo Isnard [Inria, from Jun 2021]
- Cedric Legrand [Inria, from May 2021 until Oct 2021]
- Ying Zhao [Inria, until Mar 2021]
Administrative Assistant
- Montserrat Argente [Inria]
Visiting Scientist
- Christina Kyrou [CNRS CREHA and UCA LJAD, from Oct 2021]
External Collaborator
- Frédéric Valentin [Laboratorio Nacional de Computacao Cientifica, until Sep 2021]
2 Overall objectives
ATLANTIS is a joint project-team between Inria, CNRS and Université Côte d’Azur thanks to its association with the J.A. Dieudonné Mathematics Laboratory (UMR 7351). It is a follow-up of the NACHOS project-team from which it inherits a well identified expertise on theoretical and methodological aspects of the solution of PDE (Partial Differential Equation) systems modeling electromagnetic and elastodynamic wave propagation. Relying on this expertise, our research activities in ATLANTIS aim at studying and impacting more deeply some scientific and technological challenges raised by physical problems involving waves in interaction with nanostructured matter. A crucial component in the implementation of this scientific endeavor lies in a close networking with physicists who bring the experimental counterpart of the proposed research.
Nanostructuring of materials has paved the way for manipulating and enhancing wave-matter interactions, thereby opening the door for the full control of these interactions at the nanoscale. In particular, the interaction of light waves (or more general optical waves) with matter is a subject of rapidly increasing scientific importance and technological relevance. Indeed, the corresponding science, referred to as nanophotonics46, aims at using nanoscale light-matter interactions to achieve an unprecedented level of control on light. Nanophotonics encompasses a wide variety of topics, including metamaterials, plasmonics, high resolution imaging, quantum nanophotonics and functional photonic materials. Previously viewed as a largely academic field, nanophotonics is now entering the mainstream, and will play a major role in the development of exciting new products, ranging from high efficiency solar cells, to personalized health monitoring devices able to detect the chemical composition of molecules at ultralow concentrations. Plasmonics 53 is a field closely related to nanophotonics. Metallic nanostructures whose optical scattering is dominated by the response of the conduction electrons are considered as plasmonic media. If the metallic structure presents an interface with a positive permittivity dielectric, collective oscillations of surface electrons create waves (called surface plasmons) that are guided along the interface, with the unique characteristic of subwavelength-scale confinement. Nanofabricated systems that exploit these plasmon waves offer fascinating opportunities for crafting and controlling the propagation of light in matter. In particular, it can be used to channel light efficiently into nanometer-scale volumes. As light is squeezed down into nanoscale volumes, field enhancement effects occur resulting in new optical phenomena that can be exploited to challenge existing technological limits and deliver superior photonic devices. The resulting enhanced sensitivity of light to external parameters (for example, an applied electric field or the dielectric constant of an adsorbed molecular layer) shows also great promise for applications in sensing and switching.
In ATLANTIS, our short-term and medium-term activities mainly focus on research fields related to nanophotonics. Driven by a number of nanophotonics-related physical drivers, our overall objectives are on one hand to design and develop innovative numerical methodologies for the simulation of nanaoscale light-matter interactions and to demonstrate their capabilities by studying challenging applications in close collaboration with physicist partners. On the methodological side, the Discontinous Galerkin (DG) family of methods is a cornerstone of our contributions, and our research directions in ATLANTIS aim at extending our former achievements on these methods in order to deal with more complex PDEs, including nonlinear problems and coupled systems stemming from multiphysics problems, relevant to the study of nanoscale wave-matter interactions. Moreover, mathematical modeling is a central activity of the team, in particular for shaping initial and boundary value problems in view of devising accurate, efficient and robust numerical methods in the presence of multiple space and time scales or/and geometrical singularities. Additional methodological topics that are considered in close collaboration with colleagues from other Inria teams or external applied mathematics research groups are model order reduction, inverse design, uncertainty analysis and high order geometry approximation. New contributions on these topics in the context of the physical problems studied in ATLANTIS will ultimately be implemented in the DIOGENeS software suite, which is a unique software plaform dedicated to computationl nanophotonics.
As a more prospective and longer term objective, we will also explore the possibility of exporting our methodological contributions to the much more recent scientific field of nanophononics that exploit the dynamics of phonons (quanta of lattice vibrations) at the nanoscale. These vibrations, occuring in a large variety of material systems, solids or liquids, can manifest as sound or heat. In contrast with electrons and photons, the launched efforts to achieve control of phonons are quite recent and the physics (and subsequent mathematical modeling) are not completely understood yet. These efforts came, as for nanophotonics, from the reduction of the size of electronic devices that opened up new possibilities for phonon propagation and interaction. By way of consequences, new thrilling perspectives to enhance the properties of nanodevices have appeared with technological applications encompassing nanoelectronics, renewable energy harvesting, nano- and optomechanics, quantum technologies, as well as medical therapy, imaging and diagnostics. The mathematical modeling of nanoscale sound and heat transport control raises several questions and it is not clear so far that it can rely on classical physics (mechanics) differential models as it is the case with nanophotonics, although the study of microscale phononic devices relies on PDE models that are relevant to our research activities.
3 Research program
3.1 Driving physical fields
Our activities ultimately materialize as innovative computational techniques for studying concrete questions and applications that are tightly linked to specific physical fields related to nanophotonics and plasmonics, and which will be considered in collaboration with physicists. These driving physical fields aim at exploiting the peculiarities of nanoscale light-matter interactions.
Quantum plasmonics. The physical phenomena involved in the deep confinement of light when interacting with matter opens a major route for novel nanoscale devices design. Indeed, the recent progress of fabrication at the nanoscale makes it possible to conceive metallic structures with increasingly large size mismatch, in which microscale devices can be characterized by sub-nanometer features 39. These advances have also allowed to achieve spatial separation between metallic elements of only few nanometers 37. At such sizes quantum effects become non-negligible, producing huge variations in the macroscopic optical response. Following this evolution, the quantum plasmonics field has emerged, and with it the possibility of building quantum-controled devices, such as single photon sources, transistors and ultra-compact circuitry at the nanoscale.
Thermoplasmonics. Plasmonic resonances can be exploited for many applications 53. In particular, the strong local field enhancement associated with the plasmonic resonances of a metallic nanostructure, together with the absorption properties of the metal, induce a photo-thermal energy conversion. Thus, in the vicinity of the nanostructure, the temperature increases. These effects, viewed as ohmic losses, have been for a long time considered as a severe drawback for the realization of efficient devices. However, the possibility to control this temperature rise with the illumination wavelength or polarization has gathered strong interest in the nano-optics community, establishing the basis of thermoplasmonics 35. By increasing temperature in their surroundings, metal nanostructures can be used as integrated heat nanosources. Decisive advances are foreseen in nanomedicine with applications in photothermal cancer therapy, nano-surgery, drug delivery, photothermal imaging, protein tracking, photoacoustic imaging, but also in nano-chemistry, optofluidics, solar and thermal energy harvesting (thermophotovoltaics).
Planar optics. Nanostructuring of matter can be tailored to shape, control wavefront and achieve unusual device operations. Recent years have seen tremendous advances in the fabrication and understanding of two-dimensional (2D) materials, giving rise to the field of planar optics. In particular, the concept of quasi-2D metasurfaces has started to develop into an exciting research area, where nanostructured surfaces are designed for novel functionalities 47- 38- 42. Metasurfaces are planar metamaterials with subwavelength thickness, consisting of single-layer or few-layer stacks of nanostructures. They can be readily fabricated using lithography and nanoprinting methods, and the ultrathin thickness in the wave propagation direction can greatly suppress the undesirable losses. Metasurfaces enable a spatially varying optical response (e.g. scattering amplitude, phase, and polarization). They mold optical wavefronts into shapes that can be designed at will, and facilitate the integration of functional materials to accomplish active control and greatly enhanced nonlinear response.
Optoelectronics and nanoelectronics. Semiconductors also play a major role in leveraging nanoscale light-matter interactions. Emission or absorption of light by a semiconductor is at the heart of optoelectronics, which is concerned with devices that source, detect or control light. Photodiodes, solar cells, light emitting diodes (LEDs), optical fibers and semiconductor lasers are some typical examples of optoelectronic devices. The attractive properties of these devices is based on their efficiency in converting light into electrical signals (or vice versa). Using a structuration with low dimensional materials and carrier-photons interaction, optoelectronics aims at improving the quality of these systems. A closeby field is nanoelectronics 54, i.e., the physical field that, while incorporating manufacturing constraints, tries to describe and understand the influence of the nanostructuration of electronic devices on their electronic properties. This area has quickly evolved with the increasing fabrication capabilities. One striking motivating example is the drastic increase of the number of transistors (of a few nanometer size) per chip on integrated circuits. At the achieved nanostructuration scales, inter-atomic forces, tunneling or quantum mechanical properties have a non-negligible impact. A full understanding of these effects is mandatory for exploiting them in the design of electronic components, thereby improving their characteristics.
3.2 Research agenda
The processes that underly the above-described physical fields raise a number of modeling challenges that motivate our research agenda:
- They exhibit multiple space and time scales;
- They are highly sensitive to exquisite geometrical features of nanostructures and matter nanostructuring;
- They impose dealing with unconventional material models;
- They may require to leave the comfortable setting of linear differential models;
- Some of them are inherently multiphysics processes.
3.2.1 Core research topics
Our research activities are organized around core theoretical and methodological topics to address the above-listed modeling challenges.
Multiscale modeling. The physical models that we consider may feature three different space scales. First, the size of the computational domain is fixed by the nanostructure under consideration and the required observables. Second, the solution wavelength depends on the operating frequency and on the light velocity in the constitutive materials. Finally, the finest scale involved corresponds to the nanostructuring length. These three space scales can differ by orders of magnitude, leading to unaffordable computational costs, if the discretization scheme must resolve the nanostructure details. We thus aim at providing multiscale numerical schemes, that can embed fine scale information into a coarser mesh. Such methodologies lead to embarrassingly parallel two-level algorithms, that are especially suited for HPC environments and produce accurate numerical approximations. These multiscale schemes will in particular be designed in the framework of MHM (Multiscale Hybrid-Mixed) formulations recently introduced in collaboration with Frédéric Valentin at LNCC, Petropolis, Brazil. In the MHM framework, the inherent upscaling procedure that is at the heart of the approach, allows to incorporate more physics in the numerical schemes themselves, as the upscaling principle is used to construct physical basis functions that resolve the fine scales. At the second level, one defines a set of boundary value problems, whose solutions call for adapted versions of classical finite element or DG methods, and yield the upscaled basis functions.
High order DG methods. Designing numerical schemes that are high order accurate on general meshes, i.e., unstructured or hybrid structured/unstructured meshes, will still represent a major objective of our core research activities in ATLANTIS. We will focus on the family of Discontinuous Galerkin (DG) methods that has been extensively developed for wave propagation problems during the last 15 years. We will investigate several variants, namely nodal DGTD for time-domain problems, and HDG (Hybridized DG) for time-domain and frequency-domain problems, with the general goal of devising, analyzing and developing extensions of these methods, in order to deal with the above-mentioned physical drivers: nonlinear features, in particular in relation with generation of higher order harmonics in electromagnetic wave interaction with nonlinear materials, and nonlinear models of electronic response in metallic and semiconductor materials; multiphysic couplings such as for instance when considering PDE models relevant to thermoplasmonics, optoelectronics and nanoelectronics. There are to date very few works promoting DG-type methods for these situations. Concerning nonlinear effects, it is worth notice that DG methods have already proved to be attractive and have been extensively developed for computational fluid dynamics, and in particular for gas dynamics. The development of a high order DG method for situations involving nonlinear materials require to address several issues such as the construction approximate Riemann solvers for the evaluation of numerical traces associated with the evaluation of boundary integral terms in DG weak forms, the formulation of positivity-preserving schemes as continuous solutions may develop nonlinear discontinuities and the definition of linearization strategies in conjunction with time-integration schemes.
Error estimation and adaptivity. While standard theory ensures the convergence of the discrete approximation to the correct solution, it does not permit to quantitatively estimate the discretization error in actual applications. As a result, the selection of the discretization parameters is often carried out by the practitioners themselves, based on their experience and hence manual approaches to guarantee a sufficient accuracy level. We aim at providing reliable measurements of the discretization error and devising a more systematic and rigorous procedure to select and adapt discretization parameters — in particular the mesh size and the discretization order — through the use of a posteriori estimators. On the one hand, we propose to design a posteriori error estimators for the wave-matter interaction problems we consider in ATLANTIS, able to provide a fully reliable error estimation. On the other hand, such estimators can be employed to drive hp-adaptive algorithms, where the mesh and discretization order are iteratively improved to fit the complicated structure of the solution.
Time integration for multiscale problems. Multiscale physical problems with complex geometries or heterogeneous media are extremely challenging for conventional numerical simulations. Adaptive mesh refinement is an attractive technique for treating such problems and will be developed in our research activities in ATLANTIS. Local mesh refinement imposes a severe stability condition on explicit time integration since the allowed maximal time step size is constrained by the smallest element in the mesh. We will focus on different ways to overcome this stability condition, especially by using implicit-explicit (IMEX) methods where a time implicit scheme is used only for the refined part of the mesh, and a time explicit scheme is used for the other part. Optimizing the CPU time requires coupling IMEX methods with hybridized DG (HDG) methods and non-conforming meshes and will be one of the main objectives of our research activities in ATLANTIS.
Dealing with complex materials. Physically relevant simulations deal with increasing levels of complexity in the geometrical and/or physical characteristics of nanostructures, as well as their interaction with light. Standard simulation methods may fail to reproduce the underlying physical phenomena, therefore motivating the search for more sophisticated light-matter interaction numerical modeling strategies. A first direction consists in refining classical linear dispersion models and we shall put a special focus on deriving a complete hierarchy of models, that will encompass standard linear models to more complex and nonlinear ones (such as Kerr-type materials, non- linear quantuum hydrodynamic theory models, etc.). One approach will rely on an accurate description of the Hamiltonian dynamics with intricate kinetic and exchange correlation energies, for different modeling purposes. A second direction will be motivated by the study of 2D materials. A major concern is centered around the choice of the modeling approach between a full costly 3D modeling and the use of equivalent boundary conditions (the so-called GSTC), that could in all generality be nonlinear. Assessing these two directions will require efficient dedicated numerical algorithms that will especially be able to tackle several types of nonlinearities and scales.
Dealing with coupled models. Several of our target physical fields are multiphysics in essence and require going beyond the sole description of the electromagnetic response. In thermoplasmonics, the various phenomena (heat transfer through light concentration, bubbles formation and dynamics) call for different kinds of governing PDEs (Maxwell, conduction, fluid dynamics). Since, in addition, these phenomena can occur in significatively different space and time scales, drawing a quite complete picture of the underlying physics is a challenging task, both in terms of modeling and numerical treatment. In the nanoelectronics field, an accurate description of the electronic properties involves including quantum effects. A coupling between Maxwell’s and Schrödinger equations (again at significantly different time and space scales) is a possible relevant scenario. In the optoelectronics field, the accurate prediction of semiconductors optical properties is a major concern. A possible strategy may require to solve both the electromagnetic and the drift-diffusion equations. In all these aforementioned examples, difficulties mainly arise both from the differences in physical nature as well as in the time/space scales at which each physical phenomenon occurs. Accurately modeling/solving their coupled interactions remains a formidable challenge.
High performance computing (HPC). HPC is transversal to almost all the other research topics considered in the team, and is concerned with both numerical algorithm design and software development. We will work toward taking advantage of fine grain massively parallel processing offered by GPUs in modern exascale architectures, by revisiting the algorithmic structure of the computationally intensive numerical kernels of the high order DG-based solvers that we develop. We will mostly rely on the lastest features of the OpenMP standard for implementing these numerical algorithms in the DIOGENeS software suite, which already offers a coarse grain parallelization based on MPI. We will also investigate task-based fine grain parallelization for dealing with load balancing issues inherent to realistic wave-matter interaction problems and that are sourced by both numerical (e.g., locally adapted interpolation order, PML layers, volumic observables, etc.) and physical (e.g., multiple material models) characteristics.
3.2.2 Complementary topics
Beside the above-discussed core reserach topics, we have also identified additional topics that are important or compulsory in view of maximizing the impact in nanophotonics or nanophononics of our core activities and methodological contributions.
Inverse design. Inverse design has emerged rather recently in nanophotonics, and is currently the subject of intense research as witnessed by several reviews 55. Artificial Intelligence (AI) techniques are also increasingly investigated within this context 63. In ATLANTIS, we will extend the modeling capabilities of the DIOGENeS software suite by using statistical learning techniques for the inverse design of nanophotonic devices. When it is linked to the simulation of a realistic 3D problem making use of one of the high order DG and HDG solvers we develop, the evaluation of a figure of merit is a costly process. Since a sufficiently large input data set of candidate designs, as required by using Deep Learning (DL), is generally not available, global optimization strategies relying on Gaussian Process (GP) models are considered in the first place. This activity will be conducted in close collaboration with researchers of the ACUMES project-team. In particular, we investigate GP-based inverse design strategies that were initially developed for optimization studies in relation with fluid flow problems 43- 44 and fluid-structure interaction problems 61.
Uncertainty analysis and quantification. The automatic inverse design of nanophotonic devices enables scientists and engineers to explore a wide design space and to maximize a device performance. However, due to the large uncertainty in the nanofabrication process, one may not be able to obtain a deterministic value of the objective, and the objective may vary dramatically with respect to a small variation in uncertain parameters. Therefore, one has to take into account the uncertainty in simulations and adopt a robust design model 48. We study this topic in close collaboration with researchers of the ACUMES project-team one one hand, and researchers at TU Braunschweig in Germany.
Numerical linear algebra. Sparse linear systems routinely appear when discretizing frequency-domain wave-matter interaction PDE problems. In the past, we have considered direct methods, as well as domain decomposition preconditioning coupled with iterative algorithms to solve such linear systems 7. In the future, we would like to further enhance the efficiency of our solvers by considering state-of-the-art linear algebra techniques such as block Krylov subspace methods 34, or low-rank compression techniques 60. We will also focus on multi-incidence problems in periodic structures, that are relevant to metagrating or metasurface design. Indeed, such problems lead to the resolution of several sparse linear systems that slightly differ from one another and could benefit from dedicated solution algorithms. We will collaborate with researchers of the HIEPACS (Inria Bordeaux-Sud Ouest) project-team to develop efficient and scalable solution strategies for such questions.
4 Application domains
Nanoscale wave-matter interactions find many applications of industrial and societal relevance. The applications discussed in this section are those that we address in the first place in the short- to medium-term. Our general goal is to impact scientific discovery and technological development in these application topics by leveraging our methodological contributions for the numerical modeling of nanoscale wave-matter interactions, and working in close collaboration with external partners either from the academic or the industrial world. Each of these applications is linked to one or more of the driving physical fields described in section 3.1 except nanoelectronics that we consider as a more prospective, hence long-term application.
4.1 Nanostructures for sunlight harvesting
Photovoltaics (PV) converts photon energy from the sun into electric energy. One of the major challenges of the PV sector is to achieve high conversion efficiencies at low cost. Indeed, the ultimate success of PV cell technology requires substantial progress in both cost reduction and efficiency improvement. An actively studied approach to simultaneously achieve both objectives is to exploit light trapping schemes. Light trapping enables solar cells absorption using an active material layer much thinner than the material intrinsic absorption length. This then reduces the amount of materials used in PV cells, cuts cell cost, facilitates mass production of these cells that are based on less abundant material and moreover can improve cell efficiency (due to better collection of photo- generated charge carriers). Enhancing the light absorption in ultrathin film silicon solar cells is thus of paramount importance for improving efficiency and reducing costs. Our activities in relation with this application field aim at precisely studying light absorption in nanostructured solar cell structures with the help of an adapted numerical procedure. We consider both the characterization of light trapping for a given texturing of material layers, and the goal-oriented inverse design of the nanostructuring.
4.2 Metasurfaces for light shaping
Metasurfaces produce abrupt changes over the scale of the free-space wavelength in the phase, amplitude and/or polarization of a light beam. Metasurfaces are generally created by assembling arrays of miniature, anisotropic light scatterers (i.e. resonators such as optical antennas). The spacing between antennas and their dimensions are much smaller than the wavelength. As a result the metasurfaces, on account of Huygens principle, are able to mould optical wavefronts into arbitrary shapes with subwavelength resolution by introducing spatial variations in the optical response of the light scatterers. Designing metasurfaces for realistic applications such as metalenses 62 is a challenging inverse problem. In this context, the ultimate goal of our activities is to develop numerical methodologies for the inverse design of large-area metasurfaces 59.
4.3 THz wave generation
Recent research on the interaction of short optical pulses with semiconductors has stimulated the development of low power terahertz (THz) radiation transmitters. The THz spectral range of electromagnetic waves (0.1 to 10 THz) is of great interest. In particular, it includes the excitation frequencies of semiconductors and dielectrics, as well as rotational and vibrational resonances of complex molecules. As a result, THz waves have many applications in areas ranging from the detection of dangerous or illicit substances and biological sensing to diagnosis and diseases treatment in medicine. The most common mecanism of THz generation is based on the use of THz photoconductive antennas (PCA), consisting of two electrodes spaced by a given gap and placed onto a semiconductor surface. The excitation of the gap by a femtosecond optical pulse induces a sharp increase of the concentration of charge carriers for a short period of time, and a THz pulse is generated. Computer simulation plays a central role in understanding and mastering these phenomena in order to improve the design of PCA devices. The numerical modeling of a general 3D PCA configuration is a challenging task. Indeed, it requires the simultaneous solution of charge transport in the semiconductor substrate and the electromagnetic wave radiation from the antenna 57- 64. The recently-introduced concept of hybrid photoconductive antennas leveraging plasmonic effects is even more challenging 50. So far, existing simulation approaches are based on the FDTD method, and are only able to deal with classical PCAs. In relation with the design of photonic devices for THz waves generation and manioulation, we intend to a multiscale numerical modeling strategy for solving the system of Maxwell equations coupled to various models of charge carrier dynamics in semiconductors.
4.4 Plasmonic nanostructures for nanoscale sensing
The propagation of light in a slit between metals is known to give rise to guided modes. When the slit is of nanometric size, plasmonic effects must be taken into account, since most of the mode propagates inside the metal. Indeed, light experiences an important slowing-down in the slit, the resulting mode being called gap plasmon. Hence, a metallic structure presenting a nanometric slit can act as a light trap, i.e. light will accumulate in a reduced space and lead to very intense, localized fields. Nanocubes are extensively studied in this context and have been shown to support such gap plasmon modes. At visible frequencies, the lossy behavior of metals will cause the progressive absorption of the trapped electromagnetic field, turning the metallic nanocubes into efficient absorbers. The frequencies at which this absorption occurs can be tuned by adjusting the dimensions of the nanocube and the spacer. Such metallic nanocubes can be used for a broad range of applications including plasmonic sensing, surface enhanced Raman scattering (SERS), metamaterials, catalysis, and bionanotechnology. We aim at devising a numerical methodology for characterizing the impact of geometrical parameters such as the dimensions of the cube, the rounding of nanocube corners or the size of the slit separating the cube and the substrate, on the overall performance of these absorbers. In practice, this leads us to address two main modeling issues. First, as the size of the slit is decreased, spatial dispersion effects 41 have to be taken into account when dealing with plasmonic structures. For this purpose, we consider a fluid model in the form of a nonlocal hydrodynamic Drude model 40, which materializes as a system of PDEs coupled to Maxwell's equations 9-8. The second issue is concerned with the assessment of geometrical uncertainties and their role in the development of spatial dispersion effects.
4.5 Plasmonic nanostructures for photothermal effects
The field of thermoplasmonics has developed an extensive toolbox to produce, control and monitor heat at the nanometer scale. Nanoparticles are promising nano-sensing and nano-manipulating tools, and recent studies yielded remarkable advances in design, synthesis, and implementation of luminescent nanoparticles. Some applications deal with bio-imaging and bio-sensing, like e.g. luminescent nanothermometers, nanoparticles capable of providing contactless thermal reading through their light emission properties 49. Also, bio-functionalized gold nanorods are promising candidates for light-induced hyperthermia 56, to cause local and selective damage in malignant tissue. At the same time, laser pulse interaction with plasmonic nanostructures can also be exploited for cell nanosurgery 36, including plasmonic enhanced cell transfection, molecular surgery and drug delivery. In parallel to all these bio-oriented applications, plasmonic nanoparticles can also be thought of as prototypic systems for understanding fundamental aspects of nanoscale material as well as light-matter interaction. Specific numerical modeling tools are essential to provide a good insight in this understanding.
5 New software and platforms
Let us describe new/updated software.
5.1 New software
5.1.1 DIOGENeS
-
Name:
DIscOntinuous GalErkin Nanoscale Solvers
-
Keywords:
High-Performance Computing, Computational electromagnetics, Discontinuous Galerkin, Computational nanophotonics
-
Functional Description:
The DIOGENeS software suite provides several tools and solvers for the numerical resolution of light-matter interactions at nanometer scales. A choice can be made between time-domain (DGTD solver) and frequency-domain (HDGFD solver) depending on the problem. The available sources, material laws and observables are very well suited to nano-optics and nano-plasmonics (interaction with metals). A parallel implementation allows to consider large problems on dedicated cluster-like architectures.
- URL:
-
Authors:
Stéphane Lanteri, Nikolai Schmitt, Alexis Gobé, Jonathan Viquerat, Guillaume Leroy
-
Contact:
Stéphane Lanteri
6 New results
6.1 Time-domain problems
6.1.1 Reduced-order modeling for time-domain electromagnetics
Participants: Stéphane Lanteri [UESTC, Chengdu, China], Kun Li [UESTC, Chengdu, China], Liang Li [UESTC, Chengdu, China].
In short, reduced order models (ROMs) are simplifications of high fidelity, complex models. They capture the behavior of these source models so that one can quickly study a system's dominant effects using minimal computational resources. During the last four years, in collaboration with researchers at the University of Electronic Science and Technology of China and the Southwestern University of Finance and Economics, Chengdu in China, we have studied reduced-order modeling for time-domain electromagnetics and nanophotonics. More precisely, we have considered the applicability of the proper orthogonal decomposition (POD) technique for the system of 3D time-domain Maxwell equations, possibly coupled to a Drude dispersion model, which is employed to describe the interaction of light with nanometer scale metallic structures. A reduced subspace with a significantly smaller dimension is constructed by a set of POD basis vectors extracted offline from snapshots that are extracted from simulations with a high order DGTD method. In our first contribution, a POD-based ROM is established by projecting (Galerkin projection) the global semi-discrete DG scheme onto the low-dimensional space 51- 52. Our most recent achievement 18 introduces a non-intrusive variant of the POD-based ROM the solution of parameterized time-domain electromagnetic scattering problems. Here, the considered parameters are the electric permittivity and the temporal variable. By using the singular value decomposition (SVD) method, the principal components of the projection coefficient matrices (also referred to as the reduced coefficient matrices) of full-order solutions onto the RB subspace are extracted. A cubic spline interpolation-based (CSI) approach is proposed to approximate the dominating time- and parameter-modes of the reduced coefficient matrices without resorting to Galerkin projection. The generation of snapshot vectors, the construction of POD basis functions and the approximation of reduced coefficient matrices based on the CSI method are completed during the offline stage. The RB solutions for new time and parameter values can be rapidly recovered via outputs from the interpolation models in the online stage. In particular, the offline and online stages of the proposed RB method, termed as the POD-CSI method, are completely decoupled, which ensures the computational validity of the method. Moreover, a surrogate error model is constructed as an efficient error estimator for the POD-CSI method.
6.1.2 Dynamic metasurface control using Deep Reinforcement Learning
Participants: Stéphane Lanteri [UESTC, Chengdu, China], Kun Li [UESTC, Chengdu, China], Liang Li [UESTC, Chengdu, China], Jonathan Viquerat [CEMEF, Mines ParisTech, Sophia Antipolis, France], Ying Zhao [UESTC, Chengdu, China].
Dynamic metasurface is an emerging concept for achieving a flexible control of electromagnetic waves. Generalized sheet transition conditions (GSTCs) can be used to model the relationship between the electromagnetic response and surface susceptibility parameters characterizing a metasurface. However, when it comes to the inverse problem of designing and controlling a metasurface in a space-time varying context based on GSTCs, the dynamic synthesis of the susceptibility parameters is a difficult and non-intuitive task. In this work, we transform the inverse problem of solving dynamic susceptibility parameters into a sequence of control problems. Based on FDTD numerical simulations, a Deep Reinforcement Learning (DRL) framework using a proximal policy optimization (PPO) algorithm and a fully connected neural network is designed to control the susceptibility parameters intelligently and efficiently, promoting the further expansion of the application range of metasurface and thus helping realize more flexible and effective control of electromagnetic waves. We provide numerical results in a one-dimensional setting to show the applicability, correctness and effectiveness of the proposed approach.
6.1.3 Stability and asymptotic properties of the linearized Hydrodynamic Drude model
Participants: Serge Nicaise [Université de Valenciennes], Claire Scheid.
We go a step further toward a better understanding of the fundamental properties of the linearized hydrodynamical model studied. This model is especially relevant for small nanoplasmonic structures (below 10 nm). Using a hydrodynamical description of the electron cloud, both retardation effects and non local spatial response are taken into account. This results in a coupled PDE system for which we study the linear response. In 58, we concentrated on establishing well posedness results combined to a theoretical and numerical stability analysis. We especially proved polynomial stability and provided optimal energy decay rate. Finally, we investigated the question of numerical stability of several explicit time integration strategies combined to a Discontinuous Galerkin spatial discretization. This year, we have also extended the theoretical results of well posedness and stability to a problem of propagation in an open metallic domain with a hole (using the same model). A publication is currently in the second reviewing step after minor revisions.
6.1.4 Toward thermoplasmonics
Participants: Yves D'Angelo, Stéphane Lanteri, Thibault Laufroy, Claire Scheid.
Although losses in metal are viewed as a serious drawback in many plasmonics experiments, ther- moplasmonics is the field of physics that tries to take advantage of the latter. Indeed, the strong field enhancement obtained in nanometallic structures lead to a localized temperature increase in its vicin- ity, leading to interesting photothermal effects. Therefore, metallic nanoparticles may be used as heat sources that can be easily integrated in various environments. This is especially appealing in the field of nanomedecine and can for example be used for diagnosis purposes or nanosurgery to cite but just a few. Due to the various scales and phenomena that come into play, accurate numerical modeling is challenging. Laser illumination first excites a plasmon oscillation (reaction of the electrons of the metal) that relaxes to a thermal equilibrium and in turn excites the metal lattice (phonons). The latter is then responsible for heating the surroundings. A relevant modeling approach thus consists in describing the electron-phonon coupling through the evolution of their respective temperature. Maxwell’s equations are then coupled to a set of coupled nonlinear hyperbolic equations for the temperatures of respectively electrons, phonons and environment. The nonlinearities and the different time scales at which each thermalization occurs make the numerical approximation of these equations quite challenging. In the context of the PhD of Thibault Laufroy, which has started in October 2020, we propose to develop a suitable numerical framework for studying thermoplasmonics. As a first step, we have reviewed the models used in thermoplasmonics that are most often based on strong or weak (nonlinear) couplings of Maxwell’s equations with nonlinear equations modeling heat transfer (hyperbolic or parabolic). We also layed the foundations of the numerical approximation framework and provided a first stability study. This activity is planed to be carried out in close collaboration with physicists or chemists specialized in the field, in particular from the Fresnel Institute in Marseille (Guillaume Baffou).
6.1.5 Corner effects in nanoplasmonics
Participants: Camille Carvalho [Applied Mathematics Department, University of California Merced, USA], Patrick Ciarlet [Inria POEMS project-team and ENSTA, Paris], Claire Scheid.
In this work, we study nanoplasmonic structures with corners (typically a diedral/triangular structure); a situation that raises a lot of issues. We focus on a lossles Drude dispersion model and propose to investigate the range of validity of the amplitude limit principle. The latter predicts the asymptotic harmonic regime of a structure that is monochromatically illuminated, which makes a frequency domain approach relevant. However, in frequency domain, several well posedness problems arise due to the presence of corners (addressed in the PhD thesis of Camille Carvalho). This should impact the validity of the limit amplitude principle and has not yet been addressed in the literature in this precise setting. Here, we combine frequency-domain and time-domain viewpoints to give a numerical answer to this question in two dimensions. We show that the limit amplitude principle does not hold for whole interval of frequencies, that are explicited using the well-posedness analysis. This work has been published in 10.
6.1.6 DGTD method for modeling EM wave propagation in a gain medium
Participants: Stéphane Descombes, Stéphane Lanteri, Cédric Legrand.
In laser physics, gain or amplification is a process where the medium transfers part of its energy to an incident electromagnetic radiation, resulting in an increase in optical power. This is the basic principle of all lasers. Quantitatively, gain is a measure of the ability of a laser medium to increase optical power. Modeling optical gain requires to study the interaction of the atomic structure of the medium with the incident electromagnetic wave. Indeed, electrons and their interactions with electromagnetic fields are important in our understanding of chemistry and physics. In the classical view, the energy of an electron orbiting an atomic nucleus is larger for orbits further from the nucleus of an atom. However, quantum mechanical effects force electrons to take on discrete positions in orbitals. Thus, electrons are found in specific energy levels of an atom. In a semiclassical setting, such transitions between atomic energy levels are generally described by the so-called rate equations (RE). These rate equations model the behavior of a gain material, and they need to be solved self-consistently with the system of Maxwell equations. So far, the resulting coupled system of Maxwell-RE equations has mostly been considered in a time-domain setting using the FDTD method for which several extensions have been proposed. In the context of the PhD of Cédric Legrand, we study an alternative numerical modeling approach by relying on our know how of the DGTD method. This year, we have proposed a first variant that extends the DGTD method introduced in 45. The method has been formulated and analyzed in the three-dimensional case, and the computer implementation is underway.
6.1.7 DGTD method for semiconductor device modeling
Participants: Stéphane Lanteri, Massimiliano Montone, Claire Scheid.
In the field of semiconductor physics modeling, charge carrier transport is the starring phenomenon that needs to be predicted in order to build a mathematical model, based on higher-level quantities (e.g. electric current and voltage), that can be practically used for device simulation. Charge carrier transport is generally described by a drift-diffusion model. This yields a system of partial differential equations (PDEs) which can be solved at two levels: (1) quasistatic approximation: the external force applied to the crystal is electrostatic, and drift-diffusion equations are coupled to a Poisson equation for the electrostatic potential. The goal is to determine the spatial distribution of carrier concentrations and the electric field (deduced from the potential); (2) fullwave model: the crystal is subject to an applied electromagnetic field, and Maxwell equations are coupled with transport equations for carrier dynamics. The goal is to determine the space-time evolution of carrier concentrations and the electromagnetic field. The quasistatic approximation is rigorous when the steady state of the semiconductor has to be calculated. For example, this could be a preliminary step to the fullwave simulation of a device that is biased prior to responding to a (time-varying) electromagnetic excitation. The fullwave model is particularly relevant to electro-optics, i.e. when light-matter interaction is investigated. Indeed, such study is essential to understanding and accurately modeling the operation of photonic devices for light generation, modulation, absorption. In the context of the PhD of Massimiliano Montone we have a designed a DGTD methd for solving the the coupled system of Maxwell equations and drift-diffusion equations in the fullwave setting. The method has been formulated in the general three-dimensional case, and a computer implementation has been realized in a two-dimensional setting.
6.1.8 Advanced modeling of nanostructured CMOS imagers based on DG methods
Participants: Jérémy Grebot [STMicroelectronics, Crolles], Stéphane Lanteri [STMicroelectronics, Crolles], Denis Rideau [STMicroelectronics, Crolles], Claire Scheid.
The exploitation of nanostructuring to improve the performance of CMOS imagers based on microlens grids is a very promising avenue. In this perspective, numerical modeling is a key component to accurately characterize the absorption properties of these complex imaging structures, which are intrinsically multiscale (from the micrometer scale of the lenses to the nanometric characteristics of the nanostructured material layers). The FDTD (Finite Difference Time-Domain) method is the solution adopted in the first instance for the simulation of the interaction of light with this type of structure. However, because FDTD is based on a Cartesian mesh, this method shows limitations when it comes to accurately account for complex geometric features such as the curvature of microlenses or the texturing of material layer surfaces. In this context of the PhD of Jérémy Grebot, our main objectives are (1) to leverage locally refined meshes with a high order DGTD method for modeling the propagation of light in a nanostructured CMOS imager and, (2) to study and optimize the impact of nanostructuring for improving light absorption. In particular, for what concern the second objective, an inverse design methodology combining a high order DGTD method with a statistical learning-based global optimization algorithm is developed for studying various nanostructuring patterns of the surface of the absorbing semiconductor material such as one- and two-dimensional gratings.
6.2 Frequency-domain problems
6.2.1 Spectral analysis of nanostructures
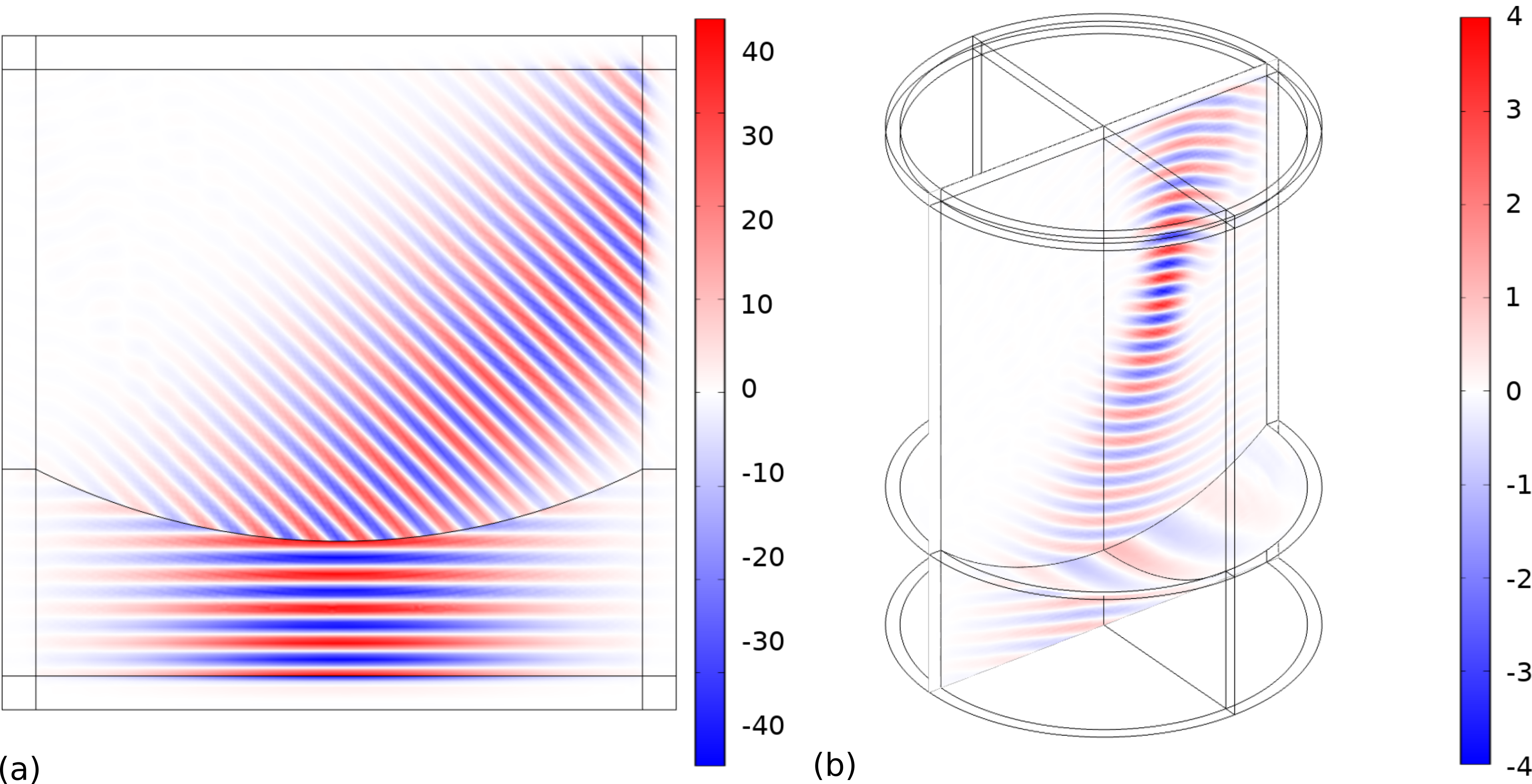
Recent research in nanophotonics shows that the optical properties of nanostructures or metasurfaces can be derived in frequency-domain through the calculation of the supported optical resonances (see Fig. 1 (a)) also called Quasi-normal modes (QNM). Optical resonant modes are modeled by a nonlinear eigenvalue problem involving Maxwell’s equations, as a consequence the research devoted to solve such eigenvalue problem is a very active research field. In this work, we propose a comparative study on two recently introduced methods to solve nonlinear eigenvalue problems. In a first step, we discretize Maxwell’s equations with a finite element method (FEM) and we employ perfectly matched layers (PML) to account for the radiation condition at infinity. FEM generate very large matrices that turn eigenvalue equations solving in tedious tasks. Thus, in a second step, we implemented two advanced contour integral methods that can handle swiftly arbitrarily large matrices: the FEAST and Beyn algorithms. We then conducted a fine study of these two algorithms and the characteristics of different integration paths in the complex plane (see Fig. 1 (b)) to derive the eigenvalues and the associated eigenvectors of cylindrical particles with semi-analytic solutions. This study will ultimately allow us to determine the fastest and most accurate method for the computation of optical resonances on particles with arbitrary geometries. Such approach will provide a very good framework upon which optimization algorithms will be implemented to conduct research on photonic structures with optimal optical properties.
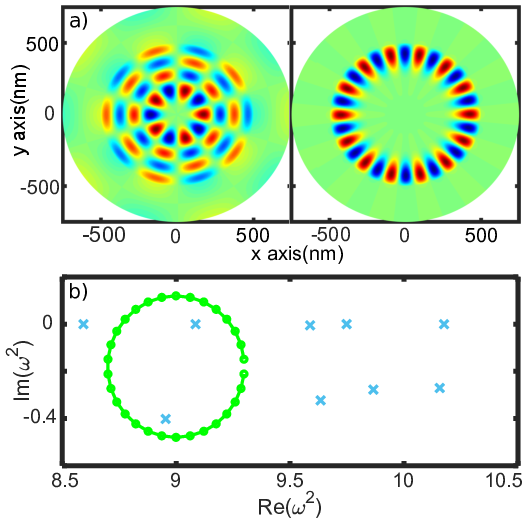
(a) Resonant modes of a cylinder made of GaAs in air. The radius of the cylinder is 500 nm the dielectric permittivity of GaAs and air are 14 and 1 respectively. The squared frequencies of the displayed eigenmodes are f1=8.95-0.4if_1=8.95-0.4i and f2=9.09-0if_2=9.09-0i, from left to right. (b) Map of the complex plane hosting the squared resonances of the structure described above. The blue crosses give the position of resonant modes and the green circle an exemple of contour to derive the modes displayed in panel (a).
6.2.2 Homogenization theory for metasurfaces
Participants: Agnès Maurel [ESPCI, Paris], Kim Pham [ENSTA, Paris], Nicolas Lebbe.
We applied the (surfacic) quasi-periodic homogeneization theory to derive equivalent transmission condition of deeply subwavelength microstructured metasurfaces. The resulting conditions are closely related to the so-called GSTC (Generalized Sheet Transition Conditions) that were originally derived in the 90s from physicial principles. Our approach provides a direct link between the coefficients of the susceptibility tensors driving surfacic polarization fields and the structural design of a microstructured metasurface. These equivalent boundary conditions are useful for numerical simulations since they reduce the complexity of the meshes at the interfaces and thus the number of degrees of freedom to be solved (see Fig. 2 (a)). The numerical implementation of these GSTCs was done both in 2D and 3D using the finite element method (FEM) in the frequency-domain (see Fig. 2 (b)). This year we have started to investigate the extension of this work in order to deal with resonant subwavelenth nanostructures, which is a prerequisite to design metasurfaces with enhanced light front control and shaping.

6.2.3 Equilibrated error estimators for high-order methods
Participants: Théohpile Chaumont-Frelet [SERENA project-team, Inria Paris], Alexandre Ern [SERENA project-team, Inria Paris], Patrick Vega [SERENA project-team, Inria Paris], Martin Vohralík [SERENA project-team, Inria Paris].
Error estimators are an important tool in the discretization of PDE problems. On the one hand, they permit to estimate the accuracy of the discrete model. On the other hand, estimators typically provide local information about the error in parts of the discretization mesh, enabling local refinement algorithms. A now well-established and widely used family of error estimators is called residual-based. These estimators have the advantage to be relatively easy to implement, and very efficient. Unfortunately, they become less accurate as the discretization order is increased. This leads in general to large error over-estimation, and decreased performance of adaptive algorithms.
In this collaboration with the SERENA project-team, we are interested in a modern family of error estimators, called equilibrated-fluxes. These estimators are slightly more costly to compute than residual-based ones, but they are much more efficient when combined with high-order discretization schemes. A key properties of such estimators is that they are p-robust, meaning that they do not suffer from an increase in the polynomial degree p employed in the discretization method. Our first key contribution in this topic that are concerned with the discretization of Helmholtz problems, has been published this year 12. Since 2020, we have focused on Maxwell's equations in the low-frequency limit. While the low-frequency limit is not directly of interest to the applications considered in the ATLANTIS project-team, it is mathematically interesting as a simplified foundation for later extension to the high-frequency case. Our first key results, where we introduce a broken patch-wise equilibrated estimators have been accepted for publication results 13. Further developments include the design of fully equilibrated estimators 32 as well as alternative equilibration strategies 27. Both of these works have been submitted for publication and are currently under review. Related theoretical results concerning approximation properties of Nédélec elements under minimal regularity have also been published 15.
6.2.4 Residual error estimators for high frequency problems
Participants: Théohpile Chaumont-Frelet, Stéphane Lanteri, Patrick Vega.
Residual error estimators are widely employed in applications to drive adaptive mesh refinement algorithms. However, most of the available current works in the literature deals with coercive problems and unfortunately, this setting does not cover high frequency wave problems, which are of central interest to the ATLANTIS project-team. This year, we started to investigate the use of residual error estimator for Maxwell's equations coupled with a linearized hydrodynamic Drude model. We covered the theoretical analysis and our two-dimensional numerical experiments are promising. This work has been accepted for publication 29. Some technical results concerning the approximability of high frequency solutions to Maxwell's equations have also been submitted 31.
6.2.5 Analysis of quasi-periodic boundary conditions
Participants: Théohpile Chaumont-Frelet, Zakaria Kassali, Stéphane Lanteri.
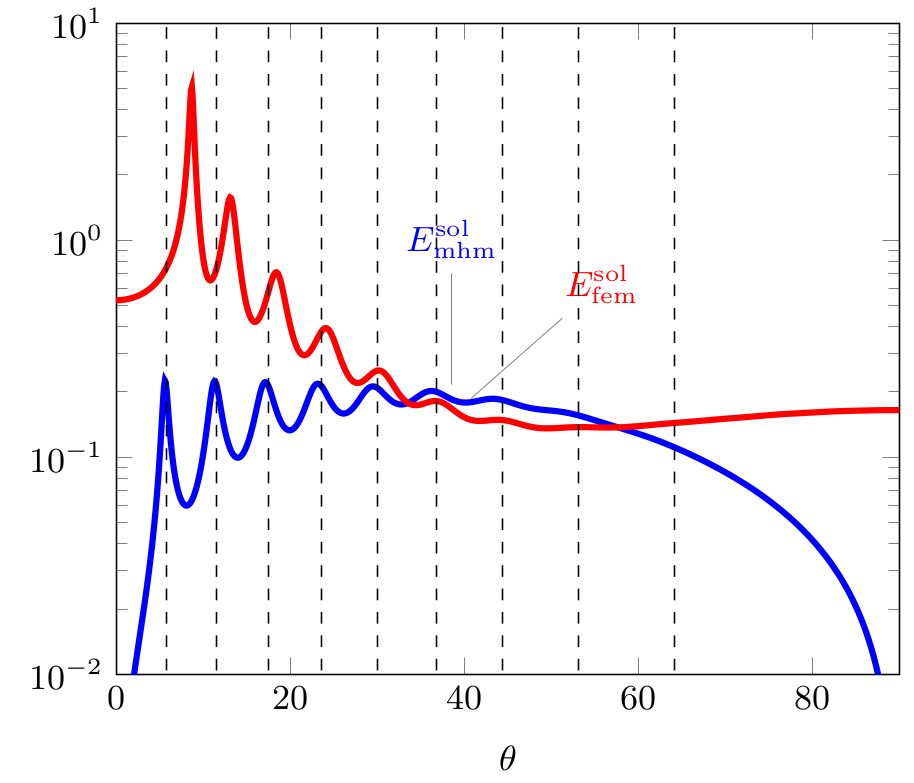
Quasi-perodidc boundary conditions arise in a number of applications considered in the team. Important examples are the simulations of solar cells and photonic crystals. These “unusual” boundary conditions correspond to an incident wave with an oblique incidence, and can strongly impact the stability and accuracy of numerical discretizations. We investigate these boundary conditions in the PhD of Zakaria Kassali. We have been able to derive sharp stability estimates for finite element discretizations involving quasi-periodicity. In particular, we have shown that a drastic accuracy loss can appear at certain angle of incidence, that mathematically correspond to “parabolically trapped” rays (see Fig. 3, blue curve). We propose to remedy this problem by using a multiscale hybrid-mixed (MHM) method (see Fig. 3, red curve). Our numerical investigations are rather promising, and current efforts are guided toward a rigorous error analysis for the MHM method, showing its robustness with respect to parabolically trapped rays.
6.2.6 Controllability methods
Participants: Théophile Chaumont-Frelet [University of Basel, Switzerland], Marcus Gröte [University of Basel, Switzerland], Stéphane Lanteri [University of Basel, Switzerland], Jet Tang [University of Basel, Switzerland].
The limiting amplitude principle predicts the asymptotic harmonic regime of a structure that is monochromatically illuminated. This makes a frequency-domain approach relevant, viewed as a long time asymptotic of a monochromatic time-domain simulation setting. Besides, it means that a time-domain solver may be used to solve frequency-domain problems, avoiding an explicit linear system solve. In this context, we investigate a modeling approach that allows to build bridges between the frequency- and time-domain approaches. This works heavily relies on the possibility of having reliable, accurate and high order discretization strategies for approximating time-domain problems. In collaboration with Marcus J. Grote and Jet Tang, University of Basel, Switzerland, we consider the use of time-domain solvers to efficiently solve frequency-domain problems in non dispersive materials. Specifically, we consider an approach called conjugate gradient controlability method that we analyze theoretically in 3D and numerically in 2D 28. We have shown that the proposed methods converges toward the time-harmonic solution, and numerical examples suggest that the method is robust in the high-frequency regime and/or in the presence of trapped waves in complex geometries.
6.2.7 Multiscale analysis of highly heterogeneous problems
Participants: Théophile Chaumont-Frelet [SERENA project-team, Inria Paris], Alexandre Ern [SERENA project-team, Inria Paris], Simon Lemaire [RAPSODI project-team, Inria Lille], Euan Spence [University of Bath, UK], Barbara Verfürth [Karlsruhe Institute for technology, Germany].
The analysis of multiscale highly heterogeneous problems where the characteristic size of the heterogeneities is much smaller than the wavelength is one of the core research topics of the ATLANTIS project-team. This year, we made three important contributions. (i) First, in collaboration with Euan Spence at University of Bath, UK, we derived frequency-explicit homogenization results for high-frequency Helmholtz problems 30. To the best of our knowledge, this work is the first contribution to rigorously established the convergence of the highly heterogeneous solution toward the homogenized one for high-frequency problems. (ii) Then, in collaboration with Alexandre Ern from the SERENA project-team and Simon Lemaire from the RAPSODI project-team, we established a bridge between two multiscale methods: the MHM and MsHHO methods 11. Specifically, we have shown that when the right-hand side is piecewise polynomial, the two methods produce the same solution, although they represent it with different degrees of freedom. We believe that this abstract result to be important, as it gives more insight about the two multiscale methods, and we hope it will provide several opportunities for improving them in the future. Finally (iii), with Barbara Verfürth at the Karlsruhe Institute for technology, Germany, we modified a mulitscale method known as LOD to handle sign-changing coefficients, which are crucial in the modeling of metamaterials 14.
6.3 Applications
6.3.1 Multiobjective statistical learning optimization of RGB metalens
Participants: Mickaël Binois [ACUMES project-team], Régis Duvigneau [ACUMES project-team], Patrice Genevet [CRHEA laboratory, Sophia Antipolis], Mahmoud Elsawy, Stéphane Lanteri.
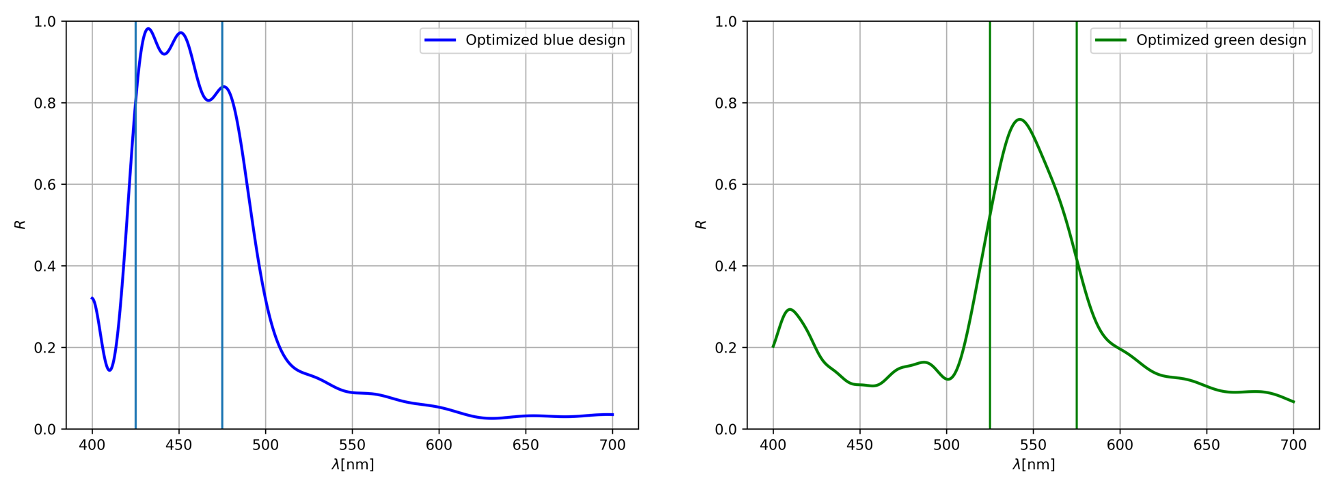
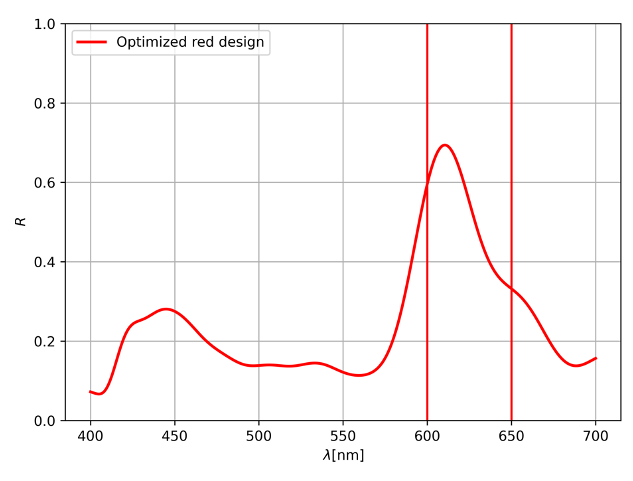
Modeling of multi-wavelength metasurfaces relies on adjusting the phase of individual nanoresonators at several wavelengths. The most widely used design procedure neglects the near-field coupling between the nanoresonators, which dramatically reduces the overall diffraction efficiency, bandwidth, numerical aperture, and device diameter. Another alternative design strategy is to combine a numerical optimization technique with fullwave simulations to mitigate this problem and optimize the entire metasurface at once. In this work, we have developed a global multiobjective optimization technique that leverages a statistical learning method to optimize RGB spherical metalenses at visible wavelengths. The optimization procedure, coupled to a fullwave solver based on a high order DGTD method, accounts for the near-field coupling between the resonators as indicated in Fig. 4 (a). High numerical aperture RGB metalenses (numerical aperture and ) of 8 and 10 m diameters are optimized with numerical average focusing efficiencies of and , respectively, and with an average focusing error of less than for the RGB colors as it is shown in Fig. 4 (c). The fabricated and experimentally characterized devices (see Fig. 4 (b)) yield and respective efficiencies. The reported performances represent the highest focusing efficiencies for high numerical aperture RGB metalenses obtained so far. The integration of multi-wavelength metasurfaces in portable and wearable electronic devices requires high performances to offer a variety of applications ranging from classical imaging to virtual and augmented reality. The manuscript has been published in one of the most prestigious scientific journals (ACS photonics) in the field of nanophotonics and metasurface 17.


6.3.2 Optimization of metasurfaces under geometrical uncertainty using statistical learning
Participants: Mickaël Binois [ACUMES project-team, Inria Sophia Antipolis-Méditerranée],, Régis Duvigneau [ACUMES project-team, Inria Sophia Antipolis-Méditerranée],, Patrice Genevet [CRHEA laboratory, Sophia Antipolis], Mahmoud Elsawy, Stéphane Lanteri.
The fabrication process of metasurface components requires dealing with very small sizes in the nanometer scale. Despite the revolutionary nanofabrication facilities developed in the past few years, uncertainties and systematic fabrication errors are usually non-negligible and are the source of efficiency degradation compared to modeling and simulation results. The performance of metasurfaces measured experimentally often discords with expected values from numerical optimization. These discrepancies are attributed to the poor tolerance of metasurface building blocks concerning fabrication uncertainties and nanoscale imperfections. Quantifying their efficiency drop according to geometry variation is crucial to improve the range of application of this technology. In this work, we have introduced a novel optimization methodology to account for the manufacturing errors related to metasurface designs. In this approach, accurate results using probabilistic surrogate models are used to reduce the number of costly numerical simulations. Our approach necessitates resolving a bi-objective optimization problem that accounts for the mean and the variance change of the efficiency under the given noise. With the UQ analysis, we obtained designs that are twice more robust than the analogous one in the deterministic case. We employ our procedure to optimize the classical beam steering metasurface made of cylindrical nanopillars as shown in Fig. 5. Our numerical results reveal that the performance of the optimized design taking into account the UQ analysis (Fig. 5 (b)) is much more robust compared to the optimized design in the deterministic case (Fig. 5 (a)). In other words, perturbing the diameters of the pillars in the deterministic case by nm dramatically reduces the overall efficiency (our target wavelength is 600 nm). Yet, the efficiency of the optimized design in the UQ case remains almost constant under the same diameter perturbations. This work has been published in Optics Express, which is a well-known open-access journal in the field of nanophotonics 16.
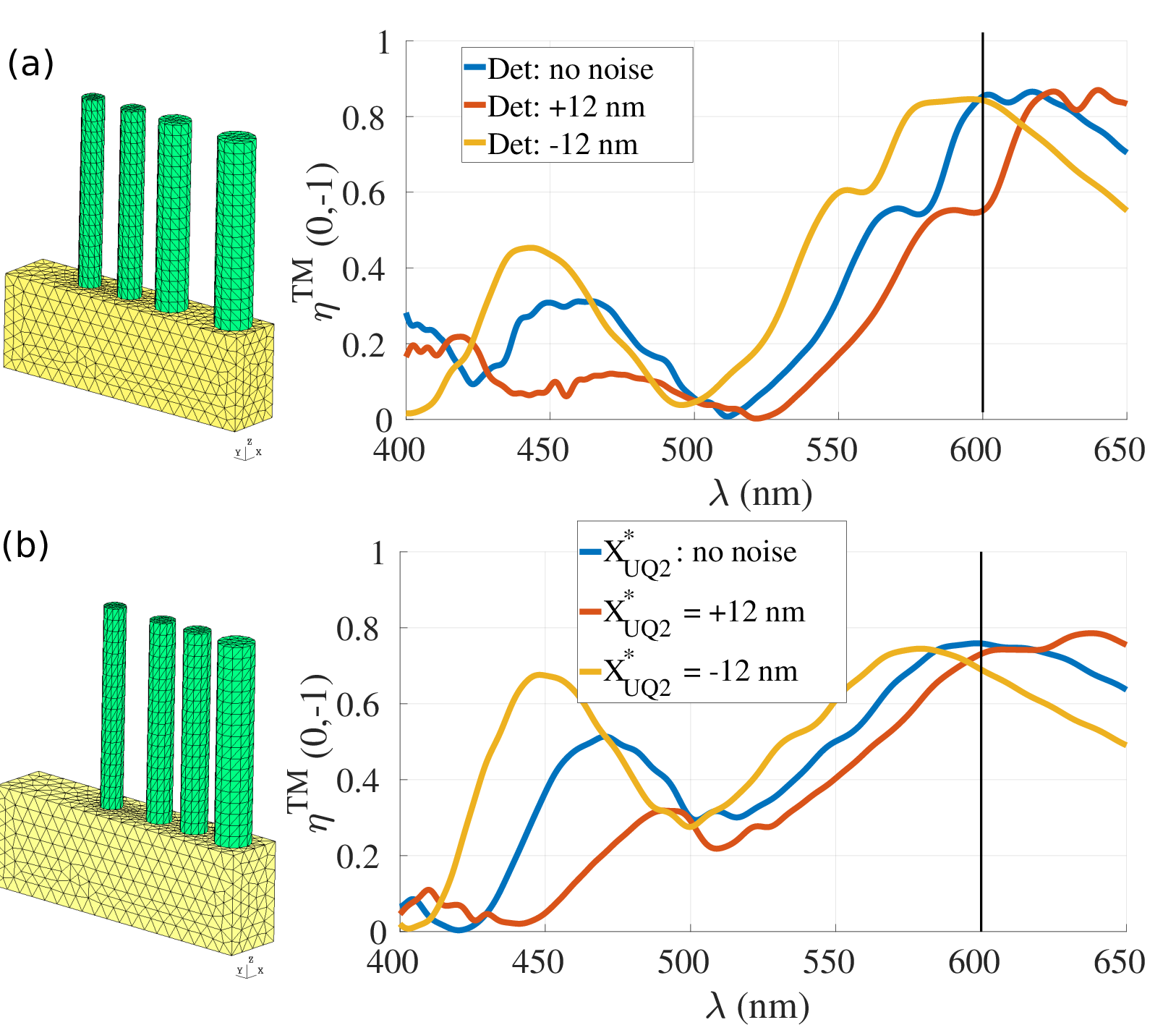
Comparison between the performance of the best design obtained in the deterministic case (a) (without taking into account the UQ) and the best design obtained from our UQ optimization methodology (b). In each case we plot the design without any noise (blue curves in each case) and trace the performance when adding ±12\pm 12 nm to the optimized diameters. The optimized geometry in each case is shown in the insets.
6.3.3 Nanoimprint color filter metasurface for imaging devices
Participants: David Grosso [Institut Matériaux Microélectronique Nanosciences de Provence (IM2NP) Aix-Marseille Université and Solnil], Marco Abbarchi [Institut Matériaux Microélectronique Nanosciences de Provence (IM2NP) Aix-Marseille Université and Solnil], Badre Kerzabi [Solnil], Mahmoud Elsawy, Alexis Gobé, Stéphane Lanteri.
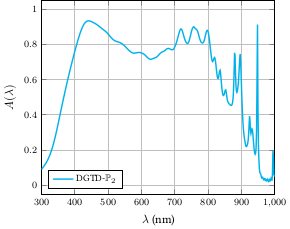
Due to the rapid growth of metasurface applications, several manufacturing techniques have been developed in the past few years. Among them, NanoImprint Lithography (NIL) has been considered as a promising fabrication possibility for metasurface. NIL can achieve features below 100 nm without the need for complex and expensive optics and light sources needed to achieve similar resolutions in advanced photolithography. Yet, despite the advantages of this fabrication procedure, it has some limitations related to the dimensions of the single nanoresonators. In other words, the fabrication constraints related to the aspect ratio (ratio between height and width) is important. Typically, a maximum aspect ratio of 2 can be considered in such a technique. In this work, together with our collaborators from IM2NP, Aix-Marseille Université and the Solnil startup who are specialists in nanoimprint technology, we aim at optimizing a low cost colour filtering metasurface for imaging applications taking into account all the fabrication constraints. The main goal of this study is to deploy our optimization methodology to maximize the efficiency of such metasurface configuration. Various shapes with different physical mechanisms have been investigated. Fig. 6 illustrates one of the optimized designs based on cylindrical nanopillars (see caption for more details). It is worth mentioning that in this study various shapes have been optimized and studied numerically, yet, as a starting point for the fabrication, the cylindrical pillars will be considered as a first illustration for the nanoimprint fabrication.
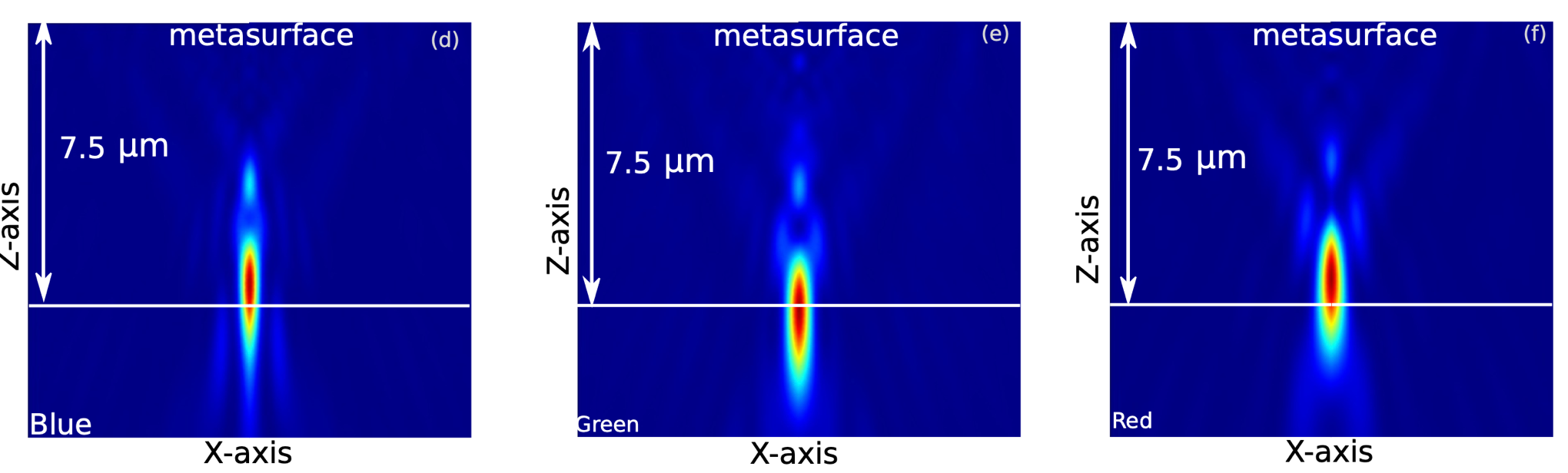
6.3.4 Simulation of 3D conformal metasurfaces using GSTCs
Participants: Sandeep Yadav Golla [CRHEA laboratory, Sophia Antipolis], Patrice Genevet [CRHEA laboratory, Sophia Antipolis], Nicolas Lebbe.
The development of metamaterials and the associated technologies have exploited transformation optics and its analogy with light propagation in curved space to manipulate light in arbitrary ways. In this collaboration, we addressed the problem of metasurface tranmission conditions at conformal interfaces using Generalized Sheet Transitions Conditions (C-GSTCs) derived following pioneering works of Idemen for planar interfaces using surfacic Dirac distributions. We also adapted the classical inversion procedure used in planar GSTCs to retrieve the susceptibilities to the conformal case by restricting the tensors values to their sole tangential components. These C-GSTCs were first implemented in both 2D and 3D using the FEM (see Fig. 17) and then used to study the impact of the interface geometry on different types of aberrations for metalenses.
6.3.5 Optimization of light trapping in nanostructured solar cells
Participants: Stéphane Collin [Sunlit team, C2N-CNRS, Paris-Saclay], Alexis Gobé, Stéphane Lanteri.
There is significant recent interest in designing ultrathin crystalline silicon solar cells with active layer thickness of a few micrometers. Efficient light absorption in such thin films requires both broadband antireflection coatings and effective light trapping techniques, which often have different design considerations. In collaboration with physicists from the Sunlit team at C2N-CNRS, We exploit statistical learning methods for the inverse design of material nanostructuring with the goal of optimizing light trapping properties of ultraphin solar cells. This objective is challenging because the underlying electromagnetic wave problems exhibit multiple resonances, while the geometrical settings are non-trivial. Such multi-resonant solar cell structures are attractive for maximizing light absorption for the full solar light spectrum as illustrated in Fig. 8. We exploit statistical learning methods for the inverse design of material nanostructuring with the goal of optimizing light trapping properties of ultraphin solar cells. This study is conducted in collaboration with the Sunlit headed by Stéphane Collin at the Center for Nanoscience and Nanotechnology (C2N, CNRS).

|
6.3.6 Plasmonic sensing with nanocubes
Participants: Antoine Moreau [Institut Pascal, Clermont-Ferrand], Stéphane Lanteri, Guillaume Leroy, Claire Scheid.
The propagation of light in a slit between metals is known to give rise to guided modes. When the slit is of nanometric size, plasmonic effects must be taken into account, since most of the mode propagates inside the metal. Indeed, light experiences an important slowing-down in the slit, the resulting mode being called gap-plasmon. Hence, a metallic structure presenting a nanometric slit can act as a light trap, i.e. light will accumulate in a reduced space and lead to very intense, localized fields. We study the generation of gap plasmons by various configurations of silver nanocubes separated from a gold substrate by a dielectric layer, thus forming a narrow slit under the cube. When excited from above, this configuration is able to support gap-plasmon modes which, once trapped, will keep bouncing back and forth inside the cavity. We exploit statistical learning methods for the goal-oriented inverse design of cube size, dielectric and gold layer thickness, as well as gap size between cubes in a dimer configuration. This study is conducted in collaboration with Antoine Moreau at Institut Pascal (CNRS).

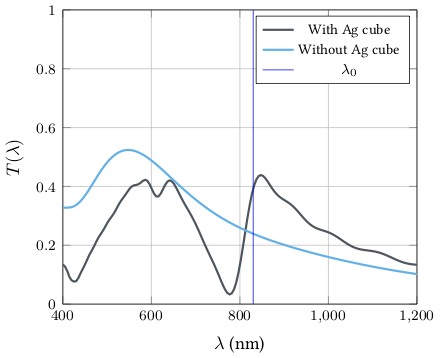
|
6.3.7 Multiple scattering in random media
Participants: Stéphane Descombes [INPHYNI laboratory, Sophia Antipolis], Stéphane Lanteri [INPHYNI laboratory, Sophia Antipolis], Guillaume Leroy [INPHYNI laboratory, Sophia Antipolis], Cédric Legrand [INPHYNI laboratory, Sophia Antipolis], Gian Luca Lippi [INPHYNI laboratory, Sophia Antipolis].
Fluorescence signals emitted by probes, used to characterize the expression of biological markers in tissues or cells, can be very hard to detect due to a small amount of molecules of interest (proteins, nucleic sequences), to specific genes expressed at the cellular level, or to the limited number of cells expressing these markers in an organ or a tissue. Access to information coming from weaker emitters can only come from strengthening the signal, since electronic post-amplification raises the noise floor as well. While costly and molecule-specific biochemical processes are being developed for this purpose, a new mechanism based on the simultaneous action of stimulated emission and multiple scattering, induced by nanoparticles suspended in the sample, has been recently demonstrated to effectively amplify weak fluorescence signals. A precise assessment of the signal fluorescence amplification that can be achieved by such a scattering medium requires a electromagnetic wave propagation modeling approach capable of accurately and efficiently coping with multiple space and time scales, as well as with non-trivial geometrical features (shape and topological organization of scatterers in the medium). In the context of a collaboration with physicists from the Institut de Physique de Nice INPHYNI (Gian Luca Lippi from the complex photonic systems and materials group), we initiated this year a study on the simultaneous action of stimulated emission and multiple scattering by randomly distributed nanospheres in a bulk medium. From the numerical modeling point of view, or short term goal is to develop a time-domain numerical methodology for the simulation of random lasing in a gain medium.

|
7 Bilateral contracts and grants with industry
7.1 Bilateral contracts with industry
Nom: NANOMULTIX - Numerical study of multispectral sensors
Participants: Mahmoud Elsawy, Alexis Gobé, Guillaume Leroy, Stéphane Lanteri.
- Duration: Jan 2021-Sep 2021
- Local coordinator: Stéphane Lanteri
- Participants: Abbarchi, David Grosso and Badre Kerzabi [Solnil, Marseille]
- Solnil is a startup company launched in 2020. Its activities are concerned with the design, development, operation and provision of products, hardware, software services, automatons or complex machines, implementing innovative technologies resulting from research on processes for the nano-fabrication of sol-gel materials and in particular, the direct nano-printing of such materials for various applications in the fields of optics and optoelectronics. Solnil carries out research and development operations, as well as scientific and technical studies. The company's ambition is to exploit and industrialize a low-cost nano-fabrication process for optical applications. This process is an evolution of an existing technology, referred as nanoimprinting, which can be described as a molding process at sub-micrometer resolutions. The industrial applications of nanoimprinting are numerous today numerous: 3D measurements for recognition, DNA sequencing augmented reality glasses, anti-reflection films, LED sources etc. In the present project, which a first step toward a longer term partnership, we reort to numerical modeling for the optimal design of metasurface-based multispectral sensors.
Nom: DGTD solvers for semiconductor device modeling
Participants: Mahmoud Elsawy, Massimiliano Montone, Claire Scheid.
- Duration: Nov 2019-Jan 2023
- Local coordinator: Stéphane Lanteri
- Participants: Eric Guichard [Silvaco Inc., Santa Clara, USA], Massimiliano Montone, Claire Scheid, Slim Chourou [Silvaco Inc., Santa Clara, USA], Mark Townsend [Silvaco Inc., Santa Clara, USA]
- This contract with the TCAD division of Silvaco Inc. is closely linked to the PhD project of Massimiliano Montone and is concerned with the numerical modeling of semiconductor-based photonic devices using high order DGTD methods. More precisely, the main methodological objective of the PhD project is to design, analyze and develop DG-based approaches for solving the system of time-domain Maxwell equations coupled to the unsteady drift-diffusion equations in 3D. On the application side, in close collaboration with Silvaco Inc., microLEDs and CMOS image sensors will constitute the driving technologies for the application of the methodological developments achieved in the PhD work; moreover, Nanostructured solar cells and photoconductive antennas (including hybrid photoconductive antennas leveraging plasmonic effects) will serve as more prospective applications that will possibly be considered in collaboration with physicists from academic groups, which are partners of the ATLANTIS project-team.
7.2 Grants with industry
Nom: DGTD solvers for modeling light absorption in CMOS imagers
Participants: Alexis Gobé, Jérémy Grebot, Guillaume Leroy, Stéphane Lanteri.
- Duration: Jan 2019-Dec 2022
- Local coordinator: Stéphane Lanteri
- Participants: Marios Barlas [STMicroelectronics, Crolles], Alexis Gobé, Valentin Goblot [STMicroelectronics, Crolles], Jérémy Grebot, Denis Rideau [STMicroelectronics, Crolles], Guillaume Leroy, Claire Scheid
- This grant is part of the Nano 2022 IPCEI (Important Projects of Common European Interest) program, which involves several european industrial groups and companies in the microelectronics field. The team is involved in a subproject conducted in close collaboration with STMicrolectronics (CMOS Imagers division of the Technology for Optical Sensors department) in Crolles. The exploitation of nanostructuring to improve the performance of CMOS imagers based on microlens grids is a very promising path. In this perspective, numerical modeling is a key component to accurately characterize the absorption properties of these complex imager structures that are intrinsically multiscale (from the micrometer scale of lenses to the nanometric characteristics of nanostructured layers of materials). The FDTD method (for Finite Difference Time-Domain) is the solution adopted in the first instance for the numerical simulation of the interaction of light with this type of structure. However, because it is based on a Cartesian mesh (mostly uniform structured mesh), this method has limitations when it comes to accurately account for complex geometrical features such as microlens curvature or texturing of material layer surfaces. In this context, the main objective of our study is to take advantage of the possibilities offered by the DGTD method with realistic geometric models of complex imager structures based on locally refined tetrahedral meshes.
8 Partnerships and cooperations
8.1 International initiatives
8.1.1 STIC/MATH/CLIMAT AmSud project
EOLIS
-
Title:
Efficient Off-LIne numerical Strategies for multi-query problems
-
Partner Institution(s):
- LNCC, Brazil
- Universidad de Concepción, Chile (UdeC), Chile
- Universidad Católica de la Santísima Concepción (USCS), Chile
- Inria, Fance
-
Date/Duration:
Jan 2021-Dec 2022
-
Additionnal info/keywords:
This project is concerned with the design, anlysis and implementation of novel preprocessing and postprocessing strategies for solving multi-query partial differential equation problems with finite element methods. By multi-query, we mean applications, such as inverse problems, optimal design or uncertainty quantification, that require a set of solution to the related PDE problems with different parameters. Instead of focusing on one particular PDE solve, we strive to reduce the computational cost for the whole set of PDE problems and focus on three main strategies: -adaptivity, multiscale modelling, and preconditioning. The design of nanostructures for light manipulation and the quantification of uncertainties associated with media fields on fluid flows will be the main benchmarks we consider.
is concerned with the design and development of numerical methodlogies for dealing efficiently and accurately with multi-query problems that involve the solution of a parametrized boundary value problem (BVP) for a set of parameters. Notable examples include parameter identification, optimal design and uncertainty quantification, each having concrete applications.
8.2 International research visitors
8.2.1 Visits of international scientists
Inria International Chair
Frédéric Valentin from LNCC, Petropolis, Brasil, is holding an Inria International Chair from January 2018 until May 2023. He is currently visiting the team (since October 2021 and until June 2022).
8.3 European initiatives
8.3.1 FP7 & H2020 projects
RISC2 (A network for supporting the coordination of High-Performance Computing research between Europe and Latin America)
Participants: Luc Giraud [HIEPACS project-team, Inria Bordeaux - Sud-Ouest], Stéphane Lanteri [ZENITH project-team], Patrick Valduriez [ZENITH project-team].
- Type: H2020 (Coordinated Support Action)
- Link.
- Duration: Jan 2021 - Jun 2023
- Coordinator: Barcelona Supercomputing Center
- Partners:
- Forschungzentrum Julich GMBH (Germany)
- Inria (France)
- Bull SAS (France)
- INESC TEC (Portugal)
- Universidade de Coimbra (Portugal)
- CIEMAT (Spain)
- CINECA (Italy)
- Universidad de Buenos Aires (Argentina)
- Universidad Industrial de Santander (Columbia)
- Universidad de le Republica (Uruguay)
- Laboratorio Nacional de Computacao Cientifica (Brazil)
- Centro de Investigacion y de Estudios Avanzados del Instituto Politecnico Nacional (Mexico)
- Universidad de Chile (Chile)
- Fundacao Coordenacao de Projetos Pesquisas e Estudos Tecnologicos COPPETEC (Brazil)
- Fundacion Centro de Alta Tecnologia (Costa Rica)
- Inria contact: Stéphane Lanteri
- Recent advances in AI and the Internet of things allow high performance computing (HPC) to surpass its limited use in science and defence and extend its benefits to industry, healthcare and the economy. Since all regions intensely invest in HPC, coordination and capacity sharing are needed. The EU-funded RISC2 project connects eight important European HPC actors with the main HPC actors from Argentina, Brazil, Chile, Colombia, Costa Rica, Mexico and Uruguay to enhance cooperation between their research and industrial communities on HPC application and infrastructure development. The project will deliver a cooperation roadmap addressing policymakers and the scientific and industrial communities to identify central application areas, HPC infrastructure and policy needs.
EPEEC (European joint effort toward a highly productive programming environment for heterogeneous exascale computing)
Participants: Alexis Gobé, Stéphane Lanteri.
- Type: H2020
- Link
- Duration: Oct 2018 - Sep 2021
- Coordinator: Barcelona Supercomputing Center
- Partner: Barcelona Supercomputing Center (Spain)
- Coordinator: CEA
- Partners:
- Fraunhofer–Gesellschaft (Germany)
- CINECA (Italy)
- IMEC (Blegium)
- INESC ID (Portugal)
- Appentra Solutions (Spain)
- Eta Scale (Sweden)
- Uppsala University (Sweden)
- Inria (France)
- Cerfacs (France)
- Inria contact: Stéphane Lanteri
- EPEEC’s main goal is to develop and deploy a production-ready parallel programming environment that turns upcoming overwhelmingly-heterogeneous exascale supercomputers into manageable platforms for domain application developers. The consortium will significantly advance and integrate existing state-of-the-art components based on European technology (programming models, runtime systems, and tools) with key features enabling 3 overarching objectives: high coding productivity, high performance, and energy awareness. An automatic generator of compiler directives will provide outstanding coding productivity from the very beginning of the application developing/porting process. Developers will be able to leverage either shared memory or distributed-shared memory programming flavours, and code in their preferred language: C, Fortran, or C++. EPEEC will ensure the composability and interoperability of its programming models and runtimes, which will incorporate specific features to handle data-intensive and extreme-data applications. Enhanced leading-edge performance tools will offer integral profiling, performance prediction, and visualisation of traces. Five applications representative of different relevant scientific domains will serve as part of a strong inter-disciplinary co-design approach and as technology demonstrators. EPEEC exploits results from past FET projects that led to the cutting-edge software components it builds upon, and pursues influencing the most relevant parallel programming standardisation bodies.
PRACE 6IP (PRACE Sixth Implementation Phase)
Participants: Emmanuel Agullo [HIEPACS project-team, Inria Bordeaux - Sud-Ouest], Alexis Gobé [HIEPACS project-team, Inria Bordeaux - Sud-Ouest], Luc Giraud [HIEPACS project-team, Inria Bordeaux - Sud-Ouest], Stéphane Lanteri.
- Type: H2020
- Link
- Duration: May 2019 - Dec 2021
- Partners
- Inria contact: Stéphane Lanteri
- The mission of PRACE (Partnership for Advanced Computing in Europe) is to enable high-impact scientific discovery and engineering research and development across all disciplines to enhance European competitiveness for the benefit of society. PRACE seeks to realise this mission by offering world class computing and data management resources and services through a peer review process. PRACE also seeks to strengthen the European users of HPC in industry through various initiatives. PRACE has a strong interest in improving energy efficiency of computing systems and reducing their environmental impact. The objectives of PRACE-6IP are to build on and seamlessly continue the successes of PRACE and start new innovative and collaborative activities proposed by the consortium. These include: assisting the development of PRACE 2; strengthening the internationally recognised PRACE brand; continuing and extend advanced training which so far provided more than 36 400 person·training days; preparing strategies and best practices towards Exascale computing, work on forward-looking SW solutions; coordinating and enhancing the operation of the multi-tier HPC systems and services; and supporting users to exploit massively parallel systems and novel architectures. The activities are designed to increase Europe's research and innovation potential especially through: seamless and efficient Tier-0 services and a pan-European HPC ecosystem including national capabilities; promoting take-up by industry and new communities and special offers to SMEs; assistance to PRACE 2 development; proposing strategies for deployment of leadership systems; collaborating with the ETP4HPC, CoEs and other European and international organisations on future architectures, training, application support and policies.
8.4 National initiatives
8.4.1 ANR project
OPERA (Adpative planar optics)
Participants: Emmanuel Agullo [HIEPACS project-team, Inria Bordeaux - Sud-Ouest], Régis Duvigneau [ACUMES project-team], Mahmoud Elsawy [CRHEA laboratory, Sophia Antipolis], Patrice Genevet [CRHEA laboratory, Sophia Antipolis], Luc Giraud [HIEPACS project-team, Inria Bordeaux - Sud-Ouest], Stéphane Lanteri.
- Type: ANR ASTRID Maturation
- See also: www-sop.inria.fr/nachos/opera
- Duration: Apr 2019 to Sep 2022
- Coordinator: Inria
- Partner: CRHEA laboratory in Sophia Antipolis and NAPA Technologies in Archamps
- Inria contact: Stéphane Lanteri
- Abstract: In the OPERA project, we are investigating and optimizing the properties of planar photonic devices based on metasurfaces using numerical modelling. The scientific and technical activities that constitute the project work programme are organized around 4 main workpackages. The numerical characterization of the optical properties of planar devices based on metasurfaces, as well as their optimization are at the heart of the activities and objectives of two horizontal (transversal) workpackages. These numerical methodologies will be integrated into the DIOGENeS software framework that will eventually integrates (1) discontinuous Galerkin-type methods that have been tested over the past 10 years for the discretization of Maxwell equations in time and frequency regimes, mainly for applications in the microwave band, (2) parallel resolution algorithms for sparse linear systems based on the latest developments in numerical linear algebra, (3) modern optimization techniques based on learning and metamodeling methods and (4) software components adapted to modern high performance computing architectures. Two vertical workpackages complete this program. One of them aims to demonstrate the contributions of methodological developments and numerical tools resulting from transversal workpackages through their application to diffusion/radiation control by passive planar devices. The other, more prospective, concerns the study of basic building blocks for the realization of adaptive planar devices.
9 Dissemination
9.1 Promoting scientific activities
9.1.1 Scientific events: organisation
Member of the organizing committees
- Théophile Chaumont-Frelet co-organized a minisymposium at ICOSAHOM 2020/2021 with Alexandre Ern (SERENA project-team) and Simon Lemaire (RAPSODI project-team)
- Stéphane Lanteri co-organized a special session at META 2020/2021 with Patrice Genevet (CNRS-CRHEA)
- Stéphane Descombes: member of the organizing committee (and head in 2021) of the SophI.A Summit conference in Sophia Antipolis (around 500 participants each year)
9.1.2 Journal
Reviewer - reviewing activities
- Stéphane Lanteri: Journal of Computational Physics
- Théophile Chaumont-Frelet: IMA Journal of Numerical Analysis, SIAM Journal on Numerical Analysis, Mathematics of Computation
- Claire Scheid: IMA Journal of Numerical Analysis
9.2 Teaching - Supervision - Juries
9.2.1 Teaching
- Claire Scheid, Analyse Fonctionnelle, Practical works, Master 1 MPA, 18 h, Université Côte d'Azur
- Claire Scheid, Optimisation et éléments finis, Master 1 MPA and IM, 54 h, Université Côte d'Azur
- Claire Scheid, Option Modélisation, Lectures and practical works, Master 2 Agrégation, 43 h, Université Côte d'Azur
- Yves D'Angelo, Modélisation Simulation Numérique, Master 1 IM/MPA, 50 h, Université Côte d'Azur
- Yves D'Angelo, Modélisation de la Turbulence dans l'Industrie, Master 1 MPA and IM, 16 h, Université Côte d'Azur
- Stéphane Descombes, Introduction to partial differential equations Master 1 MPA and IM, 30 h, Université Côte d'Azur
- Stéphane Descombes, Principal component analysis and supervised analysis, Master 2 SIRIS, Université Côte d'Azur
- Stéphane Descombes, Mathematics practical works, L1 SocioEco, 18 h, Université Côte d'Azur
- Engineering: Stéphane Lanteri, High performance scientific computing, 24 h, MAM5, Polytech Nice Sophia - Université Côte d'Azur
9.2.2 Supervision
- PhD in progress: Jérémy Grebot, Advanced modeling of nanostructured CMOS imagers, Stéphane Lanteri and Claire Scheid
- PhD in progress: Zakaria Kassali, Multiscale finite element simulations applied to the design of photovoltaic cells, Théophile Chaumont-Frelet and Stéphane Lanteri
- PhD in progress: Cédric Legrand, High order finite element type solvers for modeling gain media, Stéphane Descombes and Stéphane Lanteri
- PhD in progress: Thibault Laufroy, High order finite element type solvers for thermoplamonics, Yves d'Angelo and Claire Scheid
- PhD in progress: Massimiliano Montone, High order finite element type solvers for the coupled Maxwell-semiconductor equations in the time-domain, Stéphane Lanteri and Claire Scheid
9.2.3 Juries
- Claire Scheid, examiner, Joe Alhelou, Institut de Mathématiques de Toulouse, Université de Toulouse, France, November 2021
- Stéphane Lanteri, examiner, Claire Scheid, HDR defense, Université Côte d'Azur, December 2021
- Stéphane Lanteri, reviewer, Kassem Asfour, Université de Toulouse, France, November 2021
- Stéphane Lanteri, examiner, Sandeep Yadav, Golla, CRHEA, Université Côte d'Azur, November 2021
- Stéphane Lanteri, examiner, Anthony Gourdin, Université de Montpellier, May 2021
9.2.4 Internal or external Inria responsibilities
- Yves D'Angelo: head of the J.A. Dieudonné Mathematics Laboratory
- Stéphane Descombes: director of the “Maison de la Modélisation, de la Simulation et des Interactions” (MSI) at Université Côte d'Azur
- Stéphane Lanteri: member of the Project-team Committee's Bureau of the Inria research center at Université Côte d'Azur
- Stéphane Lanteri: member of the Scientific Committee of Cerfacs
- Stéphane Lanteri: member of the Scientific Committee of the Wavecomplexity initiative at Université Côte d'Azur
- Stéphane Lanteri: member of the “Comité de Chercheurs Calculant au CINES”
10 Scientific production
10.1 Major publications
- 1 unpublishedOn the derivation of guaranteed and p-robust a posteriori error estimates for the Helmholtz equation.August 2020, working paper or preprint
- 2 articleLocally implicit discontinuous Galerkin time domain method for electromagnetic wave propagation in dispersive media applied to numerical dosimetry in biological tissues.SIAM Journal on Scientific Computing3852016, A2611-A2633
- 3 articleTemporal convergence analysis of a locally implicit discontinuous galerkin time domain method for electromagnetic wave propagation in dispersive media.Journal of Computational and Applied Mathematics316May 2017, 122--132
- 4 articleGlobal optimization of metasurface designs using statistical learning methods.Scientific Reports91November 2019
- 5 articleA reduced-order discontinuous Galerkin method based on a Krylov subspace technique in nanophotonics.Applied Mathematics and Computation358October 2019, 128-145
- 6 articlePOD-based model order reduction with an adaptive snapshot selection for a discontinuous Galerkin approximation of the time-domain Maxwell's equations.Journal of Computational Physics396November 2019, 106-128
- 7 articleA hybridizable discontinuous Galerkin method combined to a Schwarz algorithm for the solution of 3d time-harmonic Maxwell's equations.Journal of Computational Physics256January 2014, 563-581
- 8 articleA DGTD method for the numerical modeling of the interaction of light with nanometer scale metallic structures taking into account non-local dispersion effects.Journal of Computational Physics316July 2016
- 9 articleSimulation of three-dimensional nanoscale light interaction with spatially dispersive metals using a high order curvilinear DGTD method.Journal of Computational Physics373November 2018, 210-229
10.2 Publications of the year
International journals
Conferences without proceedings
Reports & preprints
10.3 Cited publications
- 34 articleBlock GMRES method with ineact breackdowns and deflated restarting.SIAM J. Matrix Anal. Appl.3542014, 1625--1261
- 35 bookThermoplasmonics.Cambridge University Press2017
- 36 articlePlasmonics for pulsed-laser cell nanosurgery: fundamentals and applications.J. Photochem. Photobiol. C: Photochem. Rev.172013, 26--49
- 37 articleAtomic layer lithography of wafer-scale nanogap arrays for extreme confinement of electromagnetic waves.Nature Comm.42013, 2361
- 38 articleA review of metasurfaces: physics and applications.Rep. Progr. Phys.7972016, 076401
- 39 articleProbing the ultimate limits of plasmonic enhancement.Science33760982012, 1072--1074
- 40 articleHydrodynamic model for plasmonics: a macroscopic approach to a microscopic problem.Chemphyschem1462013, 1109--1116
- 41 articleInfluence of spatial dispersion in metals on the optical response of deeply subwavelength slit arrays.Phys. Rev. B9342016, 045413
- 42 articleGradient metasurfaces: a review of fundamentals and applications.Rep. Progr. Phys.8122018, 026401
- 43 articleKriging-based optimization applied to flow control.Int. J. Num. Fluids692012, 1701--1714
- 44 articleComparison of turbulence closures for optimized active control.Comput. Fluids1242016, 67--77
- 45 articleConvergence and stability of a discontinuous Galerkin time-domain method for the 3D heterogeneous Maxwell equations on unstructured meshes.ESAIM: Math. Model. Num. Anal.3962005, 1149--1176
- 46 bookIntroduction to nanophotonics.Cambridge University Press2010
- 47 articleHolographic optical metasurfaces: a review of current progress.Rep. Progr. Phys.7822015, 024401
- 48 articleUncertainty quantification guided robust design for nanoparticles morphology.Comp. Meth. Appl. Mech. Engrg.893362018, 578--593
- 49 articleLuminescence nanothermometry.Nanoscale4152012, 4301-4326
- 50 articleEnhancement of terahertz photoconductive antenna operation by optical nanoantennas.Laser Phot. Rev.1112017, 1600199
- 51 articleA reduced-order DG formulation based on POD method for the time-domain Maxwell’s equations in dispersive media.J. Comput. Appl. Math.3362018, 249--266
- 52 articlePOD-based model order reduction with an adaptive snapshot selection for a discontinuous Galerkin approximation of the time-domain Maxwell's equations.J. Comput. Phys.3962019, 106--128
- 53 bookPlasmonics - Fundamentals and applications.Springer2007
- 54 bookIntroduction to nanoelectronics.Cambridge University Press2012
- 55 articleInverse design in nanophotonics.Nat. Phot.122018, 659--670
- 56 articleOptimum morphology of gold nanorods for light-induced hyperthermia.Nanoscale1052018, 2632--2638
- 57 articleTime-domain numerical modeling of THz photoconductive antennas.IEEE Trans. Terah. Sci. Technol.442014, 490--500
- 58 articleStability and asymptotic properties of a linearized hydrodynamic medium model for dispersive media in nanophotonics.Comput. Math. Applic.79122020, 3462--3494
- 59 articleInverse design of large-area metasurfaces.Opt. Expr.26262018, 33732--33747
- 60 articleSparse supernodal solver using block low-rank compression: design performance and analysis.J. Comp. Sci.272018, 255--270
- 61 articleEfficient optimization procedure in non-linear fluid-structure interaction problem: application to mainsail trimming in upwind conditions.J. Fluids and Struct.692017, 209--231
- 62 articleLarge area metalenses: design, characterization, and mass manufacturing.Opt. Expr.262018, 1573--1585
- 63 articleIntelligent nanophotonics: merging photonics and artificial intelligence at the nanoscale.Nanophot.832019, Open AccessURL: https://doi.org/10.1515/nanoph-2018-0183
- 64 articleContribution assessment of antenna structure and in-gap photocurrent in terahertz radiation of photoconductive antenna.J. Appl. Phys.1242018, 053107

