4.1.1 Deep geothermal energy

Obtaining accurate images of natural reservoirs is still critical for their management and exploitation and seismic imaging is an efficient tool (see 83, 81 and their references therein. One example is with deep geothermal energy which requires precise imaging of deep fractured reservoirs filled with geothermal fluids. Standard seismic imaging is based upon inverting mechanical waves which have difficulties to detect them, whereas electromagnetic waves are more sensitive. We see here a clear interest of coupling seismic with electromagnetic methods and this is what Makutu is developing with the CHICkPEA project. This is a multidisciplinary project involving experimental geophysicists from UPPA, members of the LFCR (Laboratory of Complex Fluids and their Reservoirs) and Steve Pride, professor at the University of Berkeley, who developed the theory describing the coupling between seismic waves and electromagnetic waves called seismoelectric effects 90. CHICkPEA started in 2018 and it was scheduled to be completed at the end of 2021. However, beginning of 2021, the team will be involved in a new project SEE4GEO funded by ADEME, in the framework of Geothermica call. Hence CHICkPEA project will continue as long as SEE4GEO, i.e. until the end of 2023. The project belongs with E2S (Energy Environment Solutions) program of UPPA which steers the actions of the University labeled I-Site within the framework of the Investment Plan for the Future.

4.1.2 Shallow geothermal energy

Regarding shallow geothermal energy, Makutu has started a collaboration with RealTimeSeismic SME in the framework of the FEDER-Poctefa Pixil project in order to use surface waves for a better imaging of shallow reservoirs. This project goes further in the work carried out on the inversion of seismic waves. Surface waves have long been considered as noise in seismograms because they were used to study the subsurface at depths. In shallow geothermal energy, surface waves contain interesting information on the first layers of the subsurface. Inverting them is a real problem because the surface waves are of high amplitude while propagating slowly. They therefore pose difficulties for multi-frequency optimization methods. The analysis of surface wave properties derives from the analysis of elastic wave propagation in horizontally stratified media 97, 79, 82. Consequently, current surface inversion derives 1D property profiles from dispersion curves picked in the frequency-wavenumber domain 94. Most of the available methods are limited to only inverting the fundamental modes, while lateral variations are difficult to handle with this approach. To overcome these limitations, the academia community has recently started to apply Full Waveform Inversion to this specific problem 92, 85.

4.1.3 CO2 injection

The reduction of greenhouse gases in the atmosphere is a societal topic of the utmost importance, with the Paris Agreement setting ambitious goals for many countries. One fundamental pillar of greenhouse emission management is Carbon Capture Utilisation and Storage (CCUS) 98. With this strategy, carbon dioxide produced on- or off-site is sequestered and injected into depleted reservoirs, thus offsetting an important portion of current CO2 emissions. The successful and safe implementation of this strategy requires the prediction, monitoring and surveillance of stored CO2 over long periods, which presents significant challenges in terms of seismic acquisition, seismic inversion and numerical simulation. These tools, coupled with state-of-the-art flow simulations, are vital in order to support the injection operations with vital real-time and long-term information. Moreover, specific challenges related to the physics of injected CO2, such as viscosity, temperature and multi-phase fluid conditions push to the limits our current numerical models, and require ambitious new multi-physics simulations to support safe and cost-effective CO2 injection operations. For example, some recent publications like 93, 101 have shown that the combination of CO2-brine flow with wave propagation provides efficient simulations for the monitoring of sequestered CO2. Makutu proposes to develop numerical methods for this new application, in collaboration with Total, in the framework of the research agreement DIP (Depth Imaging Partnership).

4.3.1 Modeling

Although the playing context should always be the final reference, some aspects of the behavior of a wind instrument can be firstly characterized by its entry impedance which quantifies the Dirichlet-to-Neumann map of the wave propagation in the pipe in the harmonic domain. This impedance can be both measured  73, 66 and computed with simulations based on accurate and concise models of the pipe  91, 6099, 67. A more realistic approach accounts for the embouchure  78, 60, 53, 54, 100, which is modeled as a nonlinear oscillator coupled with the pressure and acoustic velocity at the entry of the pipe, allowing to predict the sound qualities. Mathematical properties of the underlying models are not yet totally understood, and adequate models still need to be developed. This is particularly true when accounting for dissipation phenomena, junctions of pipes, pipe porosity and rugosity, embouchures...

To reproduce the sound of instruments, time-dependent models are more suitable. Here, nonlinear lumped elements induce an “auto-oscillatory” behavior of the instrument. The models currently available in the literature are meant to reproduce viscothermal effects, pipe junctions, pipe radiation, lips oscillation, etc. They do not necessarily possess adequate mathematical properties to ensure stable simulations and they should be improved using asymptotic analysis methods or Lagrangian formalism.

4.3.2 Numerical methods

As far as numerical developments are concerned, the accuracy of the calculations is essential. Indeed, for some aspects like the sounding frequency, a deviation of 1% between the predictions and the observations is unacceptable. Moreover, contrary to what the team is used to do for geophysics or astrophysics thanks to HPC, numerical methods for acoustical musics must be frugal to be run on personal computers by acousticians and makers. Makutu  has a wide range of numerical methods that have been implemented in its codes for linear problems. New numerical schemes will have to be implemented to take into account the non-linearities of time-dependent models.

4.3.3 Virtual workshop

Beyond the idea of mathematically modeling musical instruments, Makutu  wishes to develop a virtual workshop whose vocation will be twofold: (i) support the manufacturers to design new instruments; (ii) recreate the sound of old and historical instruments. To implement this idea, we propose to elaborate optimization techniques that are well-known in the team to define optimal geometries to meet given specifications. This can be used to reconstruct existing instruments from acoustic measurement or to design new instruments by fixing relevant quantitative objective which is a research activity by its own 68. Behind the idea of the virtual workshop is also the intention to hear the instruments, from the knowledge of their shape and playing regime. For that purpose, time-domain models are essential.

6.1.1 OpenWind

• Name:
  Open Wind Instrument Design
• Keywords:
  Wave propagation, Inverse problem, Experimental mechanics, Time Domain, Physical simulation
• Scientific Description:
  Implementation of first order finite elements for wind musical instrument simulation. Implementation of the Full Waveform inversion method for wind musical instrument inversion. Implementation of energy consistent numerical schemes for time domain simulation of reed-type wind musical instrument.
• Functional Description:
  Simulation and inversion of wind musical instruments using one-dimensional finite element method with tonholes or valves and fingering chart. The software has three functionnalities. First, the software takes the shape of a wind instrument and computes the acoustical response (answer to a given frequential excitation). Second, the software takes the instrument shape and the control parameters of a musician, and computes the produced sound and the time evolution of many acoustical quantities. Last, the software takes a measured acoustical response and computes the corresponding instrument geometry (inner bore and tone holes parameters).
• Release Contributions:
  Addition of valves (pistons) for brass instruments and their activations with a fingering chart. New thermo-viscous losses model within conical parts.
• URL:
  https://­openwind.­inria.­fr
• Publications:
  hal-02984478, hal-02996142, hal-03132474, hal-02917351, hal-02432750, hal-02019515, hal-03231946, hal-03328715, hal-01963674
• Contact:
  Juliette Chabassier
• Participants:
  Juliette Chabassier, Augustin Ernoult, Alexis Thibault, Robin Tournemenne, Olivier Geber, Guillaume Castera, Tobias Van Baarsel

6.1.2 Hou10ni

• Keywords:
  2D, 3D, Elastodynamic equations, Acoustic equation, Elastoacoustic, Frequency Domain, Time Domain, Discontinuous Galerkin
• Scientific Description:
  Hou10ni simulates acoustic and elastic wave propagation in time domain and in harmonic domain, in 2D and in 3D. It is also able to model elasto acoustic coupling. It is based on the second order formulation of the wave equation and the space discretization is achieved using Interior Penalty Discontinuous Galerkin Method. Recently, the harmonic domain solver has been extended to handle Hybridizable Discontinuous Galerkin Methods.
• Functional Description:
  This software simulates the propagation of waves in heterogeneous 2D and 3D media in time-domain and in frequency domain. It is based on an Interior Penalty Discontinuous Galerkin Method (IPDGM) and allows for the use of meshes composed of cells of various order (p-adaptivity in space).
• News of the Year:
  In 2021, we have implemented the 3D seismoelectomagnetic equations (poroelastic+electromagnetic) for the HDG formulation and started comparison with laboratory experiments.
• URL:
  https://­team.­inria.­fr/­magique3d/­software/­hou10ni/
• Publications:
  hal-01513597, hal-01957131, hal-01388195, hal-01972134, hal-01957147, hal-02152117, hal-02486942, hal-02408315, hal-02911686, hal-03464413v1, tel-03442300v1, tel-03014772, hal-01656440, hal-01662677, hal-01623953, hal-01623952, hal-01513597, hal-01519168, hal-01254194, hal-01400663, hal-01400656, hal-01400643, hal-01313013, hal-01303391, hal-01408981, tel-01304349, hal-01184090, hal-01223344, hal-01207897, hal-01184111, hal-01184110, hal-01184107, hal-01207906, hal-01184104, hal-01207886, hal-01176854, hal-01408705, hal-01408700, tel-01292824, hal-01656440, hal-00931852, hal-01096390, hal-01096392, hal-01096385, hal-01096324, hal-01096318, tel-01133713, tel-00880628
• Contact:
  Julien Diaz
• Participants:
  Conrad Hillairet, Elodie Estecahandy, Julien Diaz, Lionel Boillot, Marie Bonnasse, Marc Fuentes, Rose-Cloé Meyer, Vinduja Vasanthan

6.1.3 haven

• Name:
  time-HArmonic waVe modEling and INversion using Hybridizable Discontinuous Galerkin Discretization
• Keywords:
  Inverse problem, Wave Equations, Geophysics, Helioseismology, Discontinuous Galerkin, MPI, Large scale, Wave propagation, 3D, 2D
• Scientific Description:
  Many applications such as seismic and medical imaging, material sciences, or helioseismology and planetary science, aim to reconstruct properties of a non directly accessible or non-visible interior. For this purpose, they rely on waves whose propagation through a medium interrelates with the physical properties (density, sound speed, etc.) of this medium. Haven is a software designed to perform imaging with waves, following an algorithm that comprises of two main stages: In the data acquisition stage, the medium response to probing waves is recorded (e.g., seismic waves from Earthquakes recorded by ground network). In the second stage, we rely on a reconstruction procedure which iteratively updates an initial model of physical parameters, so that numerical simulations approach the measurements. This procedure is employed, for instance, for seismic (reconstruction of subsurface layers) and medical (disease diagnostic) imaging.
• Functional Description:
  The software solves time-harmonic forward and inverse wave problems for acoustic and elastic media using the Hybridizable Discontinuous Galerkin method for discretization. It combines MPI and OpenMP parallelism to solve large-scale applications such as Earth's imaging and helioseismology.
• News of the Year:
  - Several choices of visco-acoustic and visco-elastic models have been added for the propagation, - New propagators dedicated to helioseismology have been implemented
• URL:
  https://­ffaucher.­gitlab.­io/­hawen-website/
• Publications:
  hal-03406861, hal-02982650, hal-03101659, hal-03101642, hal-02982619
• Contact:
  Florian Faucher
• Participant:
  Florian Faucher

6.1.4 MONTJOIE

• Keywords:
  High order finite elements, Edge elements, Aeroacoustics, High order time schemes
• Scientific Description:
  Montjoie is designed for the efficient solution of time-domain and time-harmonic linear partial differential equations using high-order finite element methods. This code is mainly written for quadrilateral/hexahedral finite elements, partial implementations of triangular/tetrahedral elements are provided. The equations solved by this code, come from the ”wave propagation” problems, particularly acoustic, electromagnetic, aeroacoustic, elastodynamic problems.
• Functional Description:
  Montjoie is a code that provides a C++ framework for solving partial differential equations on unstructured meshes with finite element-like methods (continuous finite element, discontinuous Galerkin formulation, edge elements and facet elements). The handling of mixed elements (tetrahedra, prisms, pyramids and hexahedra) has been implemented for these different types of finite elements methods. Several applications are currently available : wave equation, elastodynamics, aeroacoustics, Maxwell's equations.
• URL:
  https://­gitlab.­inria.­fr/­durufle/­montjoie
• Contact:
  Marc Durufle
• Participants:
  Juliette Chabassier, Marc Durufle, Morgane Bergot

6.1.5 GEOSX

• Keywords:
  Physical simulation, Multiphysics modelling
• Functional Description:
  GEOSX is an open-source, multiphysics simulator developed cooperatively by Lawrence Livermore National Laboratory, Stanford University, and TotalEnergies. Its goal is to open up new horizons in modeling carbon storage and other subsurface energy systems. This includes: - taking advantage of the ongoing revolution in high-performance computing hardware, which is enabling orders-of-magnitude gains in performance, but also forcing a fundamental rethink of our software designs, - enriching the physics used in industrial simulations, allowing complex fluid flow, thermal, and geomechanical effects to be handled in a seamless manner, - developing highly-scalable algorithms for solving these coupled systems, - and improving workflows for modeling faults, fractures, and complex geologic formations. Inria contributes to the seismic wave propagators of GEOSX, and to its python interface. Inria also contributes advanced workflows for seismic inversion, and CO2 storage an monitoring.
• News of the Year:
  Project-team Makutu has contributed to the integration of seismic propagators based on Q1 SEM (spectral element method), and to the development of the python interface (pyGEOSX), with the goal of later using it to integrate a complete seismic inversion workflow via full waveform inversion (FWI). These developments will carry on and expand in 2022.
• URL:
  http://­www.­geosx.­org/
• Contact:
  Randolph Settgast

6.1.6 Gar6more2D

• Keywords:
  Validation, Wave propagation
• Functional Description:
  This code computes the analytical solution of problems of waves propagation in two layered 3D media such as- acoustic/acoustic- acoustic/elastodynamic- acoustic/porous- porous/porous,based on the Cagniard-de Hoop method.
• News of the Year:
  In the framework of collaboration with Peter Moczo (Comenius University Bratislava and Slovak Academy of Sciences), David Gregor, Josef Kriztek, Miriam Kristekova (Comenius University Bratislava), Arnaud Mesgouez, Gaëlle Lefeuve-Mesgouez (Inrae, Avignon University) and Christina Morency (Laurence Livermore National Laboratory) and we have implemented the coupling between elastic and poroelastic media. The obtained results are presented in an article of Geophysical Journal Internation 16.
• URL:
  https://­gitlab.­inria.­fr/­jdiaz/­gar6more2d
• Publications:
  inria-00274136, inria-00404224, inria-00305395, gregor:hal-03471065
• Contact:
  Julien Diaz
• Participants:
  Abdelaâziz Ezziani, Julien Diaz
• Partner:
  Université de Pau et des Pays de l'Adour

7.1.1 Comparative study of French and German bassoons

Participants: Augustin Ernoult.

The bassoon is a woodwind instrument with side holes, and it is played with a double cane reed. Today, the French and German models coexist in the orchestra, with a predominance of the German model. They differ mainly by the number and the location of the holes and by the shape of the reed. The resulting timbre is slightly different, the sound of the French bassoon being often described as more “nasal” than the one of its German cousin. The aim of this work is to understand the relation between the geometric differences and the sound differences by comparing these two instruments through a series of measurements and simulations. The main part of this study as been carried out with Timo Grothe in the Erich Thienhaus Institute (ETI) in the “Hochscule für Musik” of Detmold in Germany during the research stay of Augustin Ernoult 9.2.1.

To begin, the internal geometries of one bassoon of each type have been measured with split probes (main bore and side holes). Thanks to this knowledge, the acoustic response of these instruments have been computed with the software Openwind 6.1.1. The results of these simulations and in particular their input impedance for each fingering have been compared with acoustic measurement to validate both geometric measurements and the acoustic models. To compare their sound, the two instruments have been played by the same professional player and recorded with a high quality set-up from ETI. A second set of sound recording have been carried out with an artificial mouth designed by T. Grothe allowing the fine control and the measurement of all the “player's parameters”. This knowledge has been used to compute the sound by using the temporal simulation of Openwind and compare the predicted and measured sounds.

The sound from the different measurement have been then processed to highlight the difference between the two instruments. This supposes to define specific sound descriptors. The simulations give us the possibility to vary independently each geometric parameters and observe which sound characteristic is modified. We developed phenomenological models acoustically informed to relate some geometric parameters (such has the chimney height of side holes) to the timbre signature.

The results of this study will be presented in two conference in 2022 (German and French Acoustic Congress) and should lead to the publication of two papers currently in redaction.

7.1.2 Benchmark study on wind instrument models

Participants: Juliette Chabassier, Augustin Ernoult.

In the musical acoustic community, the wave propagation within the body of the instrument and the radiation at the openings are generally computed by using light models (1D for the propagation and equivalent boundary condition at the opening). These models are obtained with a large variety of process (asymptotic studies, phenomenological models from measurement or more complex numerical simulations, etc.). For some geometric configurations, no consensus exist on which solution which must be used to correctly model a given wind instrument.

In the aim of developing a numerical tool to support the manufacture of wind instrument, a collaborative study has been started in June 2021. About thirty benchmark cases have been designed to cover the most of the geometric configurations encountered within the existing wind instruments. Each participant compute the acoustic response of these configurations with its own model, then the data are shared to be analyzed together. In parrallel of this numerical work, an experimental work has been started in December. Some of these benchmark geometries are build by the Itemm (Institut Technique Européen des Métiers de la Musique) and their acoustic characteristic s will be measured by several participants to be compared to the numeric data.

This work is done in collaboration with about ten french research teams (from academic and private institution) and conducted by Augustin Ernoult. Preliminary results will be presented at the French Congress of Acoustic in April 2022.

7.1.3 Pulsed-laser source characterization in laboratory seismic experiments

Participants: Julien Diaz, Chengyi Shen.

This work was published in Geomechanics and Geophysics for Geo-Energy and Geo-Resources 17, in collaboration with Clarisse Bordes, Daniel Brito, Federico Sanjuan (LFCR, UPPA), and Stéphane Garambois (ISterre).

7.1.4 Analytical solutions for elasto/poroelastic coupling

Participants: Julien Diaz.

7.1.5 Quantitative Analysis of Seismic Waves with Computational and Laboratory-Scale Experiments.

Participants: Florian Faucher.

We perform a quantitative analysis of the attenuation for seismic waves, with both numerical and physical experiments. We compare different rheologic models of attenuation and use body and surface waves propagation to infer their parameters. We first rely on numerical simulations, separating the body and surface waves and applying corrections of the geometrical spreading to focus on the attenuation behavior. This leads to a precise step-by-step strategy to retrieve the attenuation parameters from the wave field solutions. The computational study is supplemented with experimental acquisitions where we consider different samples. We design a dedicated setup using various Piezoelectric Transducers acting as seismic sources, and a moving Laser-Doppler Vibrometer as receivers for accurate non-contact time-domain measurements. Here, the main difficulty comes from the faces of the samples which cause wave conversions and reflections that appear strongly in the measurements.

This is the subject of Marine Deheuvels Ph.D. at UPPA, she is supervised by Daniel Brito (UPPA) and Florian Faucher. This work has been presented at the conference AGU-SEG, 25.

7.2.1 Viscothermal phenomena in wind musical instruments: modeling and simulation

Participants: Juliette Chabassier, Alexis Thibault.

For the purpose of virtual prototyping, the high-precision calculation of the acoustic propagation in a conical musical instrument - such as the bassoon or the oboe - must include dissipative phenomena due to air viscosity and thermal diffusion. These boundary layer phenomena can be represented by complex coefficients in 1D models. However, the expressions commonly used for these coefficients come from calculations carried out in cylinders. The aim of this work is to understand the variation of these dissipative phenomena with the conicity of the pipe. This work has been done in collaboration with Thomas Hélie and Henri Boutin (STMS, UMR9912, CNRS-SU-IRCAM), was presented in 38, and a journal publication is upcoming.

In addition, we compared these results to simulations from models directly posed in three dimensions, based on various simplifying hypotheses. These comparisons provide a quantitative estimate of the errors made by the different models and reveals a hierarchy of the underlying assumptions. This work has been published in a research report 63.

Time domain simulation of these phenomena is non-trivial due to the memory effect they exhibit. A new model directly based on knowledge of the geometry of the instrument, accompanied by a second-order energy-preserving discretization scheme was published in 18

7.2.2 Modeling the influence of wall porosity and roughness on acoustic propagation in wind musical instruments

Participants: Juliette Chabassier, Alexis Thibault.

In wind instruments, the geometric irregularities at the surface of the inner wall, as well as its possible porous structure, have previously been shown to influence the acoustic propagation within the bore of the instrument. An investigative work has been started in order to write models of acoustic propagation in the instrument which take into account wall porosity and/or roughness. As the base equations are the same as in the study of viscothermal phenomena, some of the expertise and concepts deployed in that previous work can be applied there. The tools used stem from the theory of periodic homogenization and asymptotic analysis. The aim of this work is to allow simulations in time of instruments with porous and/or rough walls. Simulations of an instrument with a basic model of wall roughness have already been achieved.

This work has been done in collaboration with Thomas Hélie and Henri Boutin (STMS, UMR9912, CNRS-SU-IRCAM).

7.2.3 Simulation of the piano touch

Participants: Juliette Chabassier, Guillaume Castera.

In this PhD work, we develop physical models for the piano to understand the real influence of the pianist on the sound. Mechanical models [iMMC] will be paired with vibro-acoustical models [INRIA] to analyse the differences in sound depending on the pianist’s touch. We are currently working on convergence analysis of auxiliary variable based schemes to solve the non-linear string equation in the most efficient way. We are implementing the algorithms in python to analyse the influence of multiple parameters on the precision of the results. A three-weeks scientific visit in iMMC laboratory in Belgium allowed to pursue last year's work on friction modeling between the hammer and the string. Also first simulations were made with inria's MONTJOIE software and gave encouraging results. We directly used the force applied by the action on the hammer calculated by iMMC's Robotran software as an input.

This work has been done in collaboration with Paul Fisette and Sébastien Timmermans, université catholique de Louvain, Belgique. Preliminary results on the numerical scheme have been presented at ICOSAHOM 23.

7.2.4 Low-order Prandtl-Glauert-Lorentz based Absorbing Boundary Conditions for solving the convected Helmholtz equation with Discontinuous Galerkin methods

Participants: Hélène Barucq, Nathan Rouxelin, Sébastien Tordeux.

7.2.5 Time domain analysis and localization of a non-local PML for dispersive wave equations

Participants: Julien Diaz.

This is a joint work with Nacera Baara and Mounir Tlemcani (Oran University). It has been publised in JPC 9.

7.2.6 Physical based synthesis of heritage wind musical instruments

Participants: Juliette Chabassier, Augustin Ernoult, Tobias Van Baarsel.

During this second and last year of the project SYSIMPA 9.4.1, the work was aimed at finalising the study of the geometry of the wind instruments. First, uncertainties around the mouthpiece remained, due to the relative thickness of the metal walls of the main pipe that caused important biases in the measurement based on X-ray technology. This has been addressed by using a silicone moulding of the bore of the mouthpiece. This was done at the Cité de la Musique in Paris. The moulding offers a precise measurement of the inner radius which satisfyingly completes and validates the measurements done based on X-ray tomography.

The measurements based on X-ray tomography done in the first year had to be processed in order to be used in impedance / sound synthesis. The raw geometries yielded by the measurements need to be simplified in fewer simple elements for the sake of computational cost. This has been done using two optimisations: a first one based only on the geometry, so that the simplification is as close as possible to the original measurement. This step needs some human input as the measurements include either local aberrations due to X-ray artefacts, or are simply very noisy. The second optimisation is based on the input impedance of the air column. The input impedance of the simplified geometry is computed and compared to the input impedance measured experimentally on the original instrument at the Cité de la Musique. An optimisation algorithm is then used to slightly modify the geometry so that it minimises the difference between measured and computed input impedances. The result is a simplified geometry that accurately represents the original instrument, from the geometrical and the acoustic point of view.

Temporal simulations (i.e., sound synthesis) have been performed using such a geometry. The parameters for the excitator are tricky to find, and work still needs to be done in order to fully grasp the complexity of the phenomenon. However, a set of simple parameters has been found for which a sound can be synthesised. Based on the natural resonant frequencies of the instrument, the natural scale (for the first impedance peaks, i.e., the first notes of the harmonic series) has been synthesised.

The methodology around the geometry of the instrument has been done for each of the ten selected instruments from the Besson corpus. A simplified version is from now on available for each instrument, and can be used for impedance or sound simulation.

One instrument (labeled E0925 in the archives) has been selected for the making of a physical copy. Instrument-maker Jérôme Wiss was assigned to this task. The instrument should be finished in january 2022. Impedance measurements of this copy will be done at the Cité de la Musique for comparison with the original.

This work will be presented in the French Congress of Acoustics in April and an article is being written.

7.2.7 Outgoing modal solutions for Galbrun's equation in helioseismology.

Participants: Hélène Barucq, Florian Faucher, Ha Pham.

We construct modal outgoing Green's kernels for the simplified Galbrun's equation under spherical symmetry, in the context of helioseismology. The coefficients of the equation are C² functions representing the solar interior model S, complemented with an isothermal atmospheric model. We solve the equation in vectorial spherical harmonics basis to obtain modal equations for the different components of the unknown wave motions. These equations are then decoupled and written in Schrödinger form, whose coefficients are shown to be C² apart from at most two regular singular points, and to decay like a Coulomb potential at infinity. These properties allow us to construct an outgoing Green's kernel for each spherical mode. We also compute asymptotic expansions of coefficients up to order r-³ as r tends to infinity, and show numerically that their accuracy is improved by including the contribution from the gravity although this term is of order r-³.

This is a joint work with Laurent Gizon and Damien Fournier of the Max Plank Institute for Solar system research in Göttingen (MPS), supported by the INRIA associated team ANTS between Makutu and MPS. It is published in Journal of differential Equations, 13. Some of the results of barucq:hal-03406855v1 have been presented at SIAM Conference on Mathematical & Computational Issues in the Geosciences (GS21), June 21–24, 2021, Virtual Conference, see , see 22. It has also been presented at the ANTS Workshop on helioseismology, 27.

7.2.8 Non-perturbative computation of normal modes for rotating gas giant planets.

Participants: Florian Faucher.

The Cassini spacecraft detected waves in Saturn's rings caused by Lindland and vertical resonances between ring particles and normal modes excited in the planet's interior. The detection of these waves potentially provides us with a novel way to constrain Saturn's interior structure in the same way helioseismological measurements improved our understanding of the Sun. Perturbation theory along with internal structure modeling has been used to infer the properties of Saturn’s interior from the measurements of normal mode frequencies. However, due to the measurements’ high precision and Saturn’s rapid rotation, the perturbation theory-based approach does not allow one to fully exploit the normal modes dataset. we circumvent perturbative methods altogether and, instead, implement a non-perturbative approach for computing both the hydrostatic equilibrium structure of a planet and its acoustic normal mode spectrum. We use the Concentric Maclaurin Spheroids (CMS) method to compute the planet's interior structure in hydrostatic equilibrium that results from gravitational and centrifugal forces. We model gas giant interior using a polytropic equation of state (EOS) with a unity polytropic index. With this simplified EOS, pressure scales as density squared.

This is a joint work with Anton Ermakov (Space Sciences Lab, UC Berkeley), Burkhard Militzer (UC Berkeley) and Maarten V. de Hoop (Rice University). This work was presented at conference AGU fall meeting.

7.2.9 Modeling and numerical simulation of the propagation of electromagnetic waves in offshore cables: applications to the environmental impact on the marine ecosystem

Participants: Hélène Barucq, Marc Duruflé, Augustin Leclerc.

This work is part of a PhD program which started in October 2021. The topic has been proposed by the SME Kraken Subsea and is now the heart of a collaboration between this SME, Makutu and INSA Rouen with Christian Gout and Antoine Thonnoir.

7.3.1 Construction and convergence analysis of conservative second order local time discretisation for linear wave equations

Participants: Juliette Chabassier.

In this work we present and analyse a time discretisation strategy for linear wave equations t hat aims at using locally in space the most adapted time discretisation among a family of implicit or explicit centered second order schemes. The proposed family of schemes is adapted to domain decomposition methods such as the mortar element method. They correspond in that case to local implicit schemes and to local time stepping. We show that, if some regularity properties of the solution are satisfied and if the time step verifies a stability condition, then the family of proposed time discretisations provides, in a strong norm, second order space-time convergence. Finally, we provide 1D and 2D numerical illustrations that confirm the obtained theoretical results and we compare our approach on 1D test cases to other existing local time stepping strategies for wave equations.

This is a joint work with Sébastien Imperiale (M3DISIM Inria Saclay). It has been publised in M2AN 14.

7.3.2 Tent Pitcher algorithm for space-time integration of wave problems

Participants: Hélène Barucq, Julien Diaz, Vinduja Vasanthan.

In order to develop a method in time domain for wave problems that can combine sufficient flexibility to accommodate geophysical heterogeneities, rapidity of the algorithm, and precision of the solution, we consider Trefftz-DG methods. In Trefftz spaces, the basis functions are defined as elementwise solutions. The approximation space includes thus the Physics of the problem, which contributes to accuracy, a lower number of degrees of freedom and less numerical dispersion. Moreover, it is composed of Discontinuous Galerkin functions as the continuity at the interfaces of the elements cannot be imposed strongly. The resulting Trefftz-DG variational formulation is then applied on the boundaries of each cell of the mesh of the domain of interest with appropriate transmission conditions between the cells. However, the major drawback of this method is that it leads to an implicit scheme, which requires to solve a huge linear system. To overcome this difficulty, our Trefftz-DG formulation is implemented with the Tent-Pitching algorithm, which allows us to construct a causal mesh, hence leading to a locally-implicit scheme.

We have developed a Trefftz-DG framework for solving the acoustic wave equation using Tent-Pitching algorithms. During this past year, we worked along three lines of research : improving the meshing process, parallelizing the code and developing absorbing boundary conditions.

One important aspect of numerical modeling is the mesh, which should fit the domain as perfectly as possible, as to obtain the most accurate results. We extended the existing code to unstructured meshes, which led to a modified Tent-Pitching algorithm. In order to gain time during the execution of the code, parallelization is an essential tool. We propose a parallel version of our Trefftz-DG framework, which is carried out with the MPI library. The most interesting boundary conditions are the absorbing ones, allowing us to work in bounded domains with the same characteristics as an unbounded one, avoiding reflections at the interfaces. We developed a new Trefftz-DG method with Perfectly Matched Layers and non-polynomial type basis functions : Green’s functions.

This work has been done in the framework of DIP in collaboration with Henri Calandra and has been presented in several congresses, such as ECCOMAS 20 (jan. 2021), ICOSAHOM 39(jul. 2021) and MATHIAS DAYS 40 (oct. 2021).

7.3.3 Unstructured Isogeometric Analysis with Applications to Seismic Wave Propagation

Participants: Hélène Barucq, Julien Diaz, Stefano Frambati.

This is the topic of the PhD of Stefano Frambati, defended in december 2021 41, in the framework of DIP. The construction of the spline spaces is presented in JCAM 11 This is a collaboration with Henri Calandra.

7.4.1 Trefftz methods for Maxwell equations

Participants: Margot Sirdey, Sébastien Tordeux.

7.4.2 Discontinuous Galerkin Method based on Riemann fluxes for the time domain Maxwell System

Participants: Margot Sirdey, Sébastien Tordeux.

7.4.3 Iterative Trefftz methods for anisotropic acoustic problems equations

Participants: Hélène Barucq, Ibrahima Djiba, Sébastien Tordeux.

7.4.4 A HDG framework for convected Helmholtz equation.

Participants: Hélène Barucq, Nathan Rouxelin, Sébastien Tordeux.

7.4.5 Hybridizable Discontinuous Galerkin method for time-harmonic anisotropic poroelasticity in two dimensions.

Participants: Hélène Barucq, Julien Diaz, Ha Pham, Rose-Cloé Meyer.

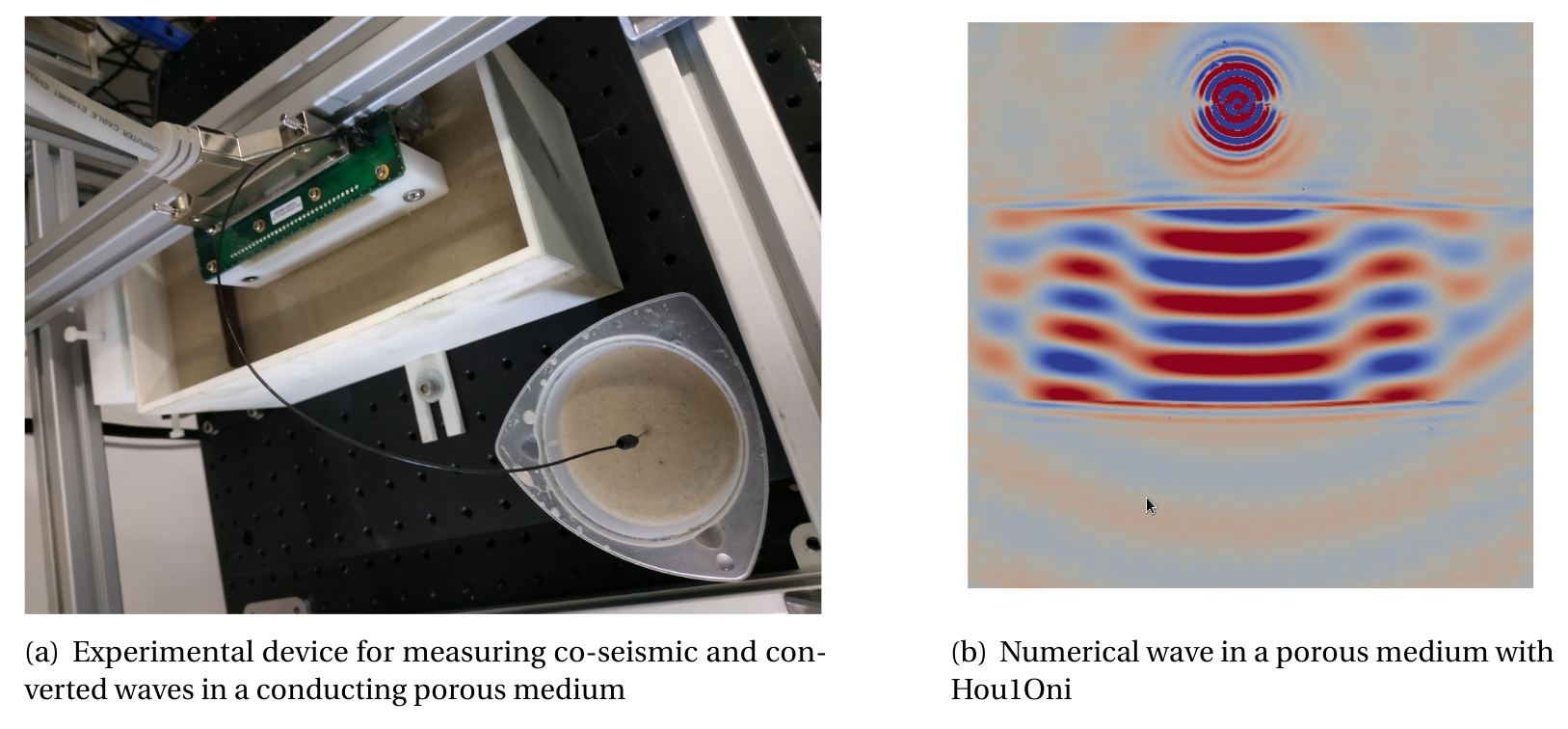
We consider the time-harmonic waves propagation in conducting poroelastic media. In poroelastic materials, whichare composed of an elastic solid frame and pores filled with fluid, wave propagation is described by Biot’s model. Ingeophysical media, due to the polarization of the fluid in the pores, one can observe the creation of elecromagneticwaves and even conversions between electromagnetic and seismic fields. They are the electrokinetic effects and aremodeled using Pride’s equations, a coupling between Maxwell’s and Biot’s equations. The electrokinetic couplinghas been observed in natural geophysical media both in laboratory and on the field. The converted waves are veryinteresting because they are heavily sensitive to the medium properties, and the seismoelectric conversions could forexample help to locate interfaces in the material that seismic waves cannot detect. The characterization of poroelasticor conducting poroelastic media is complex and involves many physical parameters, some of which depend non-linearlyon the frequencies. In addition, the seismic and electromagnetic speeds are significantly different, which is complicatedto handle for time domain simulations. Hence, we have chosen to solve the equations in the frequency domain andto use a Fourier transform to generate the seismograms in time domain. The main drawback to this is that we mustinvert one global linear system for each frequency, and this has a large computational cost because of the complexityof the equations and hence the high number of unknowns. In this work, we focus on the development and validation ofa Hybridizable Discontinuous Galerkin method for Pride’s model in the frequency regime. We validate the numericalmethod in two dimensions in circular geometry thanks to analytical solutions that we have constructed. Using theseanalytical solutions, we show in particular that the numerical method has an optimal order of convergence. In addition,to extend the method to infinite domains, we propose new radiation boundary condition for poroelastic equations andelectrokinetic equations. We have also implemented Perfectly Matched Layers and we have compared the performancesof the two methods. Finally, we show that the code we have developed is capable of modeling electrokinetic conversionsin the time domain. It is worth noting that we also provide details on the development of a HDG formulation for theporoelastic equations both in 2D and 3D.

This work is the topic of the PhD of Rose-Close Meyer, which has been funded by E2S-UPPA in the framework of the scientific challenge Chickpea. It has been defended in Septembre 2021 43. The HDG method for poroelastic material is presented in IJNME 12.

7.4.6 Local strategies for improving the conditioning of the plane-wave Ultra-Weak Variational Formulation

Participants: Hélène Barucq, Julien Diaz, Sébastien Tordeux.

This is a joint work with Abderrahmane Bendali (Insa Toulouse). It has been published in JCP 10.

7.4.7 Efficient computation of modal Green's kernels for vectorial equations in helioseismology under spherical symmetry.

Participants: Hélène Barucq, Florian Faucher, Ha Pham.

We investigate the numerical computation of physical modal Green's kernels for the time-harmonic Galbrun's equation in helioseismology under spherical symmetry. These kernels are the coefficients of the 3D Green's kernels in the vector spherical harmonic expansion. In a previous work, we have characterized the physical kernels for the isothermal radial solar background model S-AtmoI and provide their well-posedness results. Here, we provide an algorithm to compute efficiently these kernels for all receiver and source positions in a region of interest and develop the technical ingredients for its implementation.

The kernels are built from the solution of a scalar wave equation for the radial displacement. The solution and its derivative which are both necessary to assemble the Green's kernel are obtained by solving a first-order system using the HDG method. This approach extends previous works considering a scalar wave equation and allows to model not only the pressure modes but also the surface and internal gravity waves. While being physically more interesting, this problem raises additional numerical difficulties. In particular, the solution of the Schrödinger equation for the radial displacement is singular without attenuation and it is thus preferable to solve the original equation. Moreover, for low frequencies and high-modes, the potential switches sign in the atmosphere which requires the position of the artificial boundary to be further away from the solar surface in order to capture the correct physical solution.

This is a joint work with Laurent Gizon and Damien Fournier of the Max Plank Institute for Solar system research in Göttingen (MPS), supported by the INRIA associated team ANTS between Makutu and MPS. A research report of 86 pages is available, 45.

7.4.8 Efficient computation of modal scalar Green's kernels with application to helioseismology.

Participants: Hélène Barucq, Florian Faucher, Ha Pham.

The computation of Green's kernels occupies an important place in terrestrial and extraterrestrial seismology which aim to reconstruct the interior from data on the surface. Currently, time-distance helioseismology relies on single-source computations which use Green's kernel with source s at fixed height. However, observed solar oscillations should be considered as an average over all depths weighted by transparency. This requires information of the ‘full’ Green's kernel, i.e., in the entire discretized region. Our algorithm solves for two regular solutions and the full kernel is assembled from these two simulations. It is thus independent of source position, in contrast to classical approaches which require different discretization as the source height varies. Additionally, the behavior of the kernel at the source position is described analytically and circumvents dealing with a singular source problem.

This is a joint work with Laurent Gizon and Damien Fournier of the Max Plank Institute for Solar system research in Göttingen (MPS), supported by the INRIA associated team ANTS between Makutu and MPS. It is the continuation of our work published in SIAM, 58, It has been presented at AGU Fall meeting, 26, the SIAM Conference on Mathematical & Computational Issues in the Geosciences, 22, and the ANTS Workshop on helioseismology, 28.

7.4.9 Recent work on sparse direct solvers to exploit numerical and structural properties of HDG discretizations.

Participants: Florian Faucher.

Efficient numerical simulation of wave propagation phenomena is needed in several applications such as seismic imaging, non-destructive testing, or helioseismology. We consider time-harmonic waves and the hybridizable discontinuous Galerkin discretization method, for which the efficiency of the wave propagator relies on the performance of finding the solution of systems of sparse linear equations. These systems have multiple sparse right-hand sides associated with several sources. This motivates the use of direct solvers which can efficiently reuse the LU factors to compute the solution of multiple right-hand sides at the cost however of a high memory footprint. Fortunately, matrices arising from the discretization of partial differential equations have been shown to have a low-rank property and the Block-Low Rank (BLR) format has been used to design fast direct solvers with reduced asymptotic complexity. In the context of 3D frequency-domain wave equations, the BLR factorization in 32-bit single precision arithmetic has been shown to provide accurate enough solutions. We further exploit lower precision formats (such as 24 and 16 bit arithmetics) in the representation of BLR blocks, while preserving a satisfactory accuracy. This allows us to reduce the memory footprint of the solver by further compressing both the LU factor matrices and the working space without affecting the precision of the solution. The performance are analyzed based upon hawen software, 71.

This is a joint work with the consortium MUMPS Technologies, in particular Patrick Amestoy (ENS Lyon), Jean-Yves L'Excellent (ENS Lyon), Théo Mary (Sorbonne University) and Chiara Puglisi. This work has been presented at ECCOMAS Conference and a preprint is being written. Some results regarding helioseismology have been presented at the ANTS Workshop on helioseismology, 29.

7.5.1 Full waveform inversion for bore reconstruction of woodwind-like instruments

Participants: Juliette Chabassier, Augustin Ernoult.

The internal geometry of a wind instrument can be estimated from acoustic measurements. For woodwind instruments, this involves characterizing the inner shape (bore) but also the side holes (dimensions and location). In this study, the geometric parameters are recovered by a gradient-based optimization process, which minimizes the deviation between simulated and measured linear acoustic responses of the resonator for several fingerings through an observable function. The acoustic fields are computed by solving a linear system resulting from the 1D spectral finite elements spatial discretization of the wave propagation equations (including thermo-viscous effects, radiation and side holes). The “full waveform inversion” (FWI) technique exploits the fact that the gradient of the cost function can be computed by solving the same linear system as that of the direct problem but with a different source term. The gradient is computed with better accuracy and less additional cost than with finite-difference. The dependence of the cost function on the choice of the observed quantity, the frequency range and the fingerings used, is first analyzed. Then, the FWI is used to reconstruct, from measured impedances, an elementary instrument with 14 design variables. The results, obtained in about 1 minute on a laptop, are in excellent agreement with the direct geometric measurements.

This work has been done in collaboration with Samuel Rodriguez from I2M (University of Bordeaux, CNRS, Arts et Métier, Bordeaux INP) and the manufacturer Augustin Humeau from Humeau Factory. It has been published in 15.

7.5.2 Full Waveform Inversion on Seismic Data including Surface Waves.

Participants: Hélène Barucq, Julien Diaz, Florian Faucher, Chengyi Shen.

7.5.3 Time-Domain Full Waveform Inversion using advanced Discontinuous Galerkin methods

Participants: Hélène Barucq, Julien Diaz, Pierre Jacquet.

This is the topic of the PhD of Pierre Jacquet, defended in february 2021 42, in the framework of DIP. This is a collaboration with Henri Calandra.

7.5.4 Comparison of Fourier Reconstruction and Full Waveform Inversion for diffraction tomography.

Participants: Florian Faucher.

We study the mathematical imaging problem of diffraction tomography which is an inverse scattering technique used to find material properties of an object by illuminating it with probing waves and recording the scattered waves.Conventional DT relies on the Fourier diffraction theorem, which is applicable under the condition of weak scattering. However, if the object has high contrasts or is too large compared to the wavelength, it tends to produce multiple scattering, which complicates the reconstruction. We give a survey on diffraction tomography and compare the reconstruction of low and high contrast objects. We also implement and compare the reconstruction using the full waveform inversion method which, contrary to the Born and Rytov approximations, works with the total field and is more robust to multiple scattering.

This is a joint work with Clemens Kirisits (University of Vienna), Michael Quellmalz (TU Berlin), Otmar Scherzer (University of Vienna) and Eric Setterqvist (RICAM). A preprint is available online: 70.

7.5.5 Visco-acoustic ultrasound imaging allowing for attenuation model error.

Participants: Florian Faucher.

We perform ultrasound imaging in visco-acoustic media for the recovery of biological tissues and identification of embedded bodies. The attenuation of waves is encoded via different models in the propagation and we review the corresponding time-harmonic formulations to compare the resulting wave fields. For the reconstruction of the physical properties of the media, we use the full wave model for the propagation, and rely on a minimization algorithm to iteratively update the parameters. Firstly, we investigate the robustness of the algorithm with respect to model error, that is, when a different attenuation model is used between the data generation and the inversion. Secondly, to handle data-sets with multiple reflections generated by wall boundaries around the domain, we perform inversion using complex frequencies, and show that it offers a robust framework that alleviate the difficulties. To illustrate the performance of the algorithm, we carry out experiments with the imaging of a synthetic breast sample that contains an inclusion of sharp contrast in two and three dimensions, where the latter also serves to demonstrate the numerical feasibility in large-scale configuration.

This is a joint work with Otmar Scherzer (University of Vienna).

9.1.1 Inria associate team not involved in an IIL or an international program

9.1.2 SEE4GEO project as part of European GEOTHERMICA initiative

SEE4GEO is for SeismoElectric Effects for GEOthermal resource assessment and monitoring. Information on the project is available here. The project is lead by Christina Morency, LLNL (Lawrence Livermore National Laboratory, US department of Energy). Makutu's is involved in the project as a joint team with UPPA (University of Pau and Pays de l'Adour).

9.2.1 Visits to international teams

9.3.1 FP7 & H2020 projects

9.3.2 Other european programs/initiatives

9.4.1 SYSIMPA

• Title:
  Synthèse Sonore des Instruments de Musique du PAtrimoine
• Durations
  January 2020 – January 2022
• Coordinator:
  Inria
• Partners:
  • Cité de la Musique-Philharmonie de Paris (CM-P)
  • Institut Technologique Européen des Métiers de la Musique (ITEMM) in le Mans
  • Centre Culturel et de Restauration des Musées de France (C2RMF)
• Summary:
  This project aims at studying ancient music wind instruments (in this case, natural trumpets from around 1900) kept in the museum of CM-P using imaging technologies available at C2RMF and numerical methods developped at INRIA. The people involved in this project are : Tobias van Baarsel, Juliette Chabassier and Augustin Ernoult (INRIA) ; Romain Viala (ITEMM) ; Clotilde Boust and Elsa Lambert (C2RMF) ; Thierry Maniguet, Marguerite Jossic, Rodolphe Bailly, Cécile Cecconi and Sebastian Kirsch (CM-P).
• Inria contact:
  Tobias Van Baarsel

9.4.2 Depth Imaging Partnership

Magique-3D maintains active collaborations with Total. In the context of Depth Imaging, Magique-3D coordinates research activities dealing with the development of high-performance numerical methods for solving wave equations in complex media. This project has involved 2 other Inria Team-Projects (Hiepacs and Nachos) which have complementary skills in mathematics, computing and in geophysics. DIP is fully funded by Total by the way of an outline agreement with Inria .

The third phase of DIP began in 2019. Aurélien Citrain has been hired as engineer to work on the DIP platform. More than 10 PhD students have defended their PhD since the creation of DIP and most of them are now post-doctoral researchers or engineers in Europe. DIP is currently employing 3 PhD students.

9.5.1 Project supported by Conseil Régional d'Aquitaine

• Title:
  Revival.
• Coordinator:
  Juliette Chabassier
• Other partners:
  Univ Bordeaux, Univ Montreal (Canada), Univ Cath. Louvain (Belgium)
• Summary:
  The objective is to develop numerical tools for the virtual restoration of heritage instruments.
• Financing:
  This project is supported by the Conseil Régional d'Aquitaine, for a duration of 2 years and has funded the postdoctoral position of Tobias van Baarsel since Feb 2019.

10.1.1 Scientific events: organisation

10.1.2 Journal

Members of Makutu are regular reviewers for the following journals:

• Mathematics of Computation
• Numerical Methods in Engineering
• SIAM Journal on Scientific Computing
• ESAIM: Mathematical Modelling and Numerical Analysis
• Geophysical Journal International
• International Journal on Geomathematics
• Journal of Computational Physics
• International Journal for Numerical Methods in Engineering
• SIAM Journal on Scientific Computing
• SIAM Journal on Numerical Analysis
• SIAM journal on Applied Mathematics
• Inverse Problems
• Inverse Problems in Science & Engineering
• Journal of Acoustical Society of America
• Journal of Sound and Vibration
• Geophysics
• Journal of Inverse and Ill-Posed Problems
• Journal of Mathematical Imaging and Vision

10.1.3 Invited talks

Juliette Chabassier has been keynote speaker in the Journées d'Informatique Musicale 24.

10.1.4 Seminars

• Augustin Ernoult presented two seminars in the Erich Thienhaus Institute, Hochschule für Musik, Detmold, Germany, in the context of his research stay 9.2.1:
  • "Modeling sound production in the recorder", 28/06/2021
  • "Bassoon and Basson, an acoustical comparison", 11/10/2021
• Florian Faucher presented in the Mathematics & Statistics MACSI Seminar at the University of Limerick “Quantitative seismic imaging using recent sensing technology with Full Reciproity-gap Waveform Inversion”, in March 2021.

10.1.5 Leadership within the scientific community

• Augustin Ernoult is elected member of the "Groupe spécialisé d'acoustique musicale" (Gsam) of the french acoustical society.
• Hélène Barucq is member of the committee appointed by the Hcéres to carry out a national synthesis of the evaluations of French Mathematics

10.1.6 Scientific expertise

• Since 2017, Hélène Barucq is chairwoman of a committee which evaluates research projects in Mathematics, Computer Science, Electronics and Optics to be funded by the Regional Council New Aquitaine.
• Since 2018, Hélène Barucq is scientific officer for E2S project. She participates in the evaluation of each E2S call. She is also member of a committee having in charge the recruitment of non permanent researchers for E2S.
• Hélène Barucq acts as a scientific expert for the FWO (Research Foundation - Flanders.
• Hélène Barucq is member of the steering committee of CATIE.

10.1.7 Research administration

• Augustin Ernoult is member of the Center Commitee of Inria Bordeaux Sud-Ouest.
• Hélène Barucq is appointed member of the scientific board of the LMA2S (Laboratory of Mathematics Applied to Aeronautics and Space) created to federate the activities in Applied Mathematics which are carried out in seven departments of the ONERA.
• Julien Diaz is elected member of the Inria Technical Committee and of the Inria Administrative Board. He is appointed member of the Bureau du Comité des Projets (BCP) of Inria Bordeaux Sud-Ouest. Since 2018, he has been the head of the Mescal team of LMAP.
• Rose-Cloé Meyer is elected member of Laboratory Commitee of LMAP.
• Juliette Chabassier is member of the Research Position Commission of Inria Bordeaux Sud-Ouest.

10.2.1 Supervision

• PhD: Pierre Jacquet, Time domain Full Waveform Inversion involving hybrids numerical method to characterize elasto-acoustic media, February 25th, Hélène Barucq and Julien Diaz.
• PhD: Rose-Cloé Meyer, Modeling of conducting poro-elastic media using advanced numerical methods, Université de Pau et des Pays de l'Adour, September 30th, Hélène Barucq, Julien Diaz and Ha Howard Faucher.
• PhD: Nathan Rouxelin, Advanced numerical modeling of acoustic waves propagating below the surface of the Sun, Université de Pau et des Pays de l'Adour, December 10th, Hélène Barucq and Sébastien Tordeux.
• PhD: Stefano Frambati, Isogeometric analysis of sharp boundaries in full waveform inversion, December 13th, Hélène Barucq and Julien Diaz.
• PhD in progress: Victor Martins Gomez, Experimental characterization and modeling of seismo-electromagnetic waves, Université de Pau et des Pays de l'Adour, October 2018, Hélène Barucq and Daniel Brito (LFCR).
• PhD in progress : Margot Sirdey, Méthode de Trefftz pour l'électromagnétisme, October 2019, Sébastien Tordeux and Sébastien Pernet (Onera).
• PhD in progress : Vinduja Vasanthan, Tent Pitcher algorithm for space-time integration of wave problems, October 2019, Hélène Barucq and Julien Diaz.
• PhD in progress : Alexis Thibault, Modeling and simulation of wind musical instruments, October 2020, Juliette Chabassier and Thomas Hélie (IRCAM).
• PhD in progress : Guillaume Castera, Modeling and simulation of the piano touch, October 2020, Juliette Chabassier and Paul Fisette (Louvain Cath. Univ., Belgium).
• PhD in progress: Nicolas Victorion, Numerical schemes assisted with Machine Learning for solving time-dependent seismic wave problems, October 2021, Hélène Barucq, Florian Faucher and Emmanuel Franck (Inria Nancy Grand-Est, Tonus).
• PhD in progress: Arjeta Heta, Advanced numerical schemes to model seismoelectric effects and improve characterization of geological reservoirs, September 2021, Hélène Barucq and Julien Diaz.
• PhD in progress: Ibrahima Djiba, Trefftz domain decomposition method for wave propagation in geophysics, October 2021, Hélène Barucq et Sébastien Tordeux.
• PhD in progress: Marine Deheuvels, from quantitative analysis of wave amplitudes to full waveform inversion, October 2020, Daniel Brito (UPPA) et Florian Faucher

10.2.2 Juries

• Hélène Barucq: Hajer Methenni, Mathematical and numerical modeling for the simulation of ultrasonic integrated health monitoring of laminated composite plates, March 26th 2021, Reviewer
• Hélène Barucq: Paul Stocker (Vienna University, Austria), Space-time finite elment methods, July 1rst 2021, Reviewer
• Hélène Barucq: Adèle Veilleux (ISAE, Université Fédérale Toulouse Midi-Pyrénées), Extension of the Spectral Difference method to simplex cells and hybrid grids, March 24th 2021, Committee chair
• Hélène Barucq: Milad Farshad (Mines ParisTech, PSL, Université Paris), Multi-parameter seismic linear waveform imaging, December 9th 2021
• Hélène Barucq and Sébastien Tordeux: Ronan Cranny (ISAE, Université Fédérale Toulouse Midi-Pyrénées), Study of a hybrid finite volume - finite difference method and of a skewed thin wire model for electromagnetic compatibility applications, December 17th 2021, Examiner
• Hélène Barucq: Ibtissem Zaafrani (Université de Lorraine), Dynamics and stabilization of a cold magnetic plasma, December 19th 2021, Reviewer
• Julien Diaz : Alexandre Goupy (Université de Paris Saclay), Une approche spectrale de la métamodélisation multi-échelle appliquée à la propagation acoustique, March 26th 2021, Reviewer
• Juliette Chabassier : Pierre Carré (IRCAM) : Méthodes numériques d’inspiration géométrique pour la synthèse sonore par modèle physique; application à un modèle de corde géométriquement exact, Dec 21st, 2021, Examiner
• Juliette Chabassier : Nathan Rouxelin (Inria), Méthodes numériques mixtes condensées pour l’étude de la propagation des ondes acoustiques en écoulement. Applications en héliosismologie, Dec 10th2021, Examiner
• Juliette Chabassier : Hajer Methenni, Mathematical and numerical modeling for the simulation of ultrasonic integrated health monitoring of laminated composite plates, March 26th 2021, Examiner
• Florian Faucher : Nathan Rouxelin (Inria), Méthodes numériques mixtes condensées pour l’étude de la propagation des ondes acoustiques en écoulement. Applications en héliosismologie, Dec 10th2021, Examiner

10.3.1 Articles and contents

• An article in Germann has been published by the radio "Deutschlandfunk" on 12.07.2021. It is devoted to the research project 9.2.1 that Augustin Ernoult carried out on German and French bassoons in collaboration with Timo Grothe during his research stay in Germany. See here.

10.3.2 Interventions

• Tobias Van Baarsel participated to the Musicora exhibition in Paris, on the 19th-21th of Novemebr 2021. He gave a public talk to present the project SYSIMPA 9.4.1 and the numerical restoration of an historical trumpet.

International journals

• 9 articleN.Nacéra Baara, J.Julien Diaz and M.Mounir Tlemcani. Time domain analysis and localization of a non-local PML for dispersive wave equations.Journal of Computational Physics445November 2021, 110638
  HALDOIback to text
• 10 articleH.Hélène Barucq, A.Abderrahmane Bendali, J.Julien Diaz and S.Sébastien Tordeux. Local strategies for improving the conditioning of the plane-wave Ultra-Weak Variational Formulation.Journal of Computational Physics441September 2021, 110449
  HALDOIback to text
• 11 articleH.Hélène Barucq, H.Henri Calandra, J.Julien Diaz and S.Stefano Frambati. Polynomial-reproducing spline spaces from fine zonotopal tilings.Journal of Computational and Applied Mathematics402March 2022, 113812
  HALDOIback to text
• 12 articleH.Hélène Barucq, J.Julien Diaz, R.-C.Rose-Cloé Meyer and H.Ha Pham. Implementation of Hybridizable Discontinuous Galerkin method for time-harmonic anisotropic poroelasticity in two dimensions..International Journal for Numerical Methods in Engineering2021
  HALback to text
• 13 articleH.Hélène Barucq, F.Florian Faucher, D.Damien Fournier, L.Laurent Gizon and H.Ha Pham. Outgoing modal solutions for Galbrun's equation in helioseismology.Journal of Differential Equations286June 2021, 494-530
  HALDOIback to text
• 14 articleJ.Juliette Chabassier and S.Sébastien Imperiale. Construction and convergence analysis of conservative second order local time discretisation for linear wave equations.ESAIM: Mathematical Modelling and Numerical Analysis554July 2021, 1507-1543
  HALDOIback to text
• 15 articleA.Augustin Ernoult, J.Juliette Chabassier, S.Samuel Rodriguez and A.Augustin Humeau. Full waveform inversion for bore reconstruction of woodwind-like instruments.Acta AcusticaNovember 2021
  HALDOIback to text
• 16 articleD.David Gregor, P.Peter Moczo, J.Jozef Kristek, A.Arnaud Mesgouez, G.Gaëlle Lefeuve-Mesgouez, C.Christina Morency, J.Julien Diaz and M.Miriam Kristekova. Seismic waves in medium with poroelastic/elastic interfaces: a two-dimensional P-SV finite-difference modelling.Geophysical Journal International2281January 2022, 551-588
  HALDOIback to textback to text
• 17 articleC. D.Chengyi D Shen, D.Daniel Brito, J.Julien Diaz, F.Federico Sanjuan, C.Clarisse Bordes and S.Stéphane Garambois. Pulsed-laser source characterization in laboratory seismic experiments.Geomechanics and Geophysics for Geo-Energy and Geo-Resources81February 2022
  HALDOIback to text
• 18 articleA.Alexis Thibault and J.Juliette Chabassier. Dissipative time-domain one-dimensional model for viscothermal acoustic propagation in wind instruments..Journal of the Acoustical Society of America1502August 2021, 1165-1175
  HALDOIback to text
• 19 articleA.Alexis Thibault, L.Lénaïc Chizat, C.Charles Dossal and N.Nicolas Papadakis. Overrelaxed Sinkhorn-Knopp Algorithm for Regularized Optimal Transport.Algorithms1452021
  HALDOI

International peer-reviewed conferences

• 20 inproceedingsV.Vinduja Vasanthan, H.Hélène Barucq, H.Henri Calandra and J.Julien Diaz. Spacetime Trefftz-DG formulation for Wave Propagation using Tent-Pitching Meshes.Eccomas Congress 2020 & WCCM 2020 - European Congress on Computational Methods in Applied Sciences and Engineering & 14th World Congress in Computational MechanicsParis, FranceJanuary 2021
  HALback to text

Conferences without proceedings

• 21 inproceedingsH.Hélène Barucq, H.Henri Calandra, J.Julien Diaz and S.Stefano Frambati. Unlocking the power of unstructured Isogeometric Analysis: a unified framework and some applications to wave propagation and elasticity.IX International Conference on Isogeometric AnalysisLyon, FranceSeptember 2021
  HAL
• 22 inproceedingsH.Hélène Barucq, F.Florian Faucher, H.Ha Pham, L.Laurent Gizon and D.Damien Fournier. Outgoing solutions and radiation conditions of the scalar wave equation in helioseismology..SIAM Conference on Mathematical & Computational Issues in the Geosciences 2021Milan, ItalyJune 2021
  HALback to textback to text
• 23 inproceedingsG.Guillaume Castera and J.Juliette Chabassier. Energy-preserving IEQ and SAV schemes applied to piano string.ICOSAHOMVienna, AustriaJuly 2021
  HALback to text
• 24 inproceedingsJ.Juliette Chabassier. Modéliser et simuler les instruments de musique.Journées d'informatique musicale 2021visio, FranceJuly 2021
  HALback to text
• 25 inproceedingsM.Marine Deheuvels, F.Florian Faucher and D.Daniel Brito. Quantitative Analysis of Seismic Waves with Computational and Laboratory-Scale Experiments.AGU-SEG meeting 2021online, FranceSeptember 2021
  HALback to text
• 26 inproceedingsF.Florian Faucher, H.Hélène Barucq, H.Ha Pham, L.Laurent Gizon and D.Damien Fournier. Efficient computation of modal Green’s kernels with application to helioseismology.AGU Fall Meeting 2021New Orleans, United StatesDecember 2021
  HALback to text
• 27 inproceedingsF.Florian Faucher, H.Hélène Barucq, H.Ha Pham, L.Laurent Gizon and D.Damien Fournier. Vectorial problem with 1D background model: Spherical harmonics decomposition.ANTS Workshop on Computational Helioseismology 2021Gottingen, GermanyMarch 2021
  HALback to text
• 28 inproceedingsF.Florian Faucher. Full waveform inversions in helioseismology.ANTS Workshop on Computational Helioseismology 2021Gottingen, GermanyMarch 2021
  HALback to text
• 29 inproceedingsF.Florian Faucher. Introduction to hawen for time-harmonic modeling using HDG, towards global Sun simulations.ANTS Workshop on Computational HelioseismologyGottingen, GermanyMarch 2021
  HALback to text
• 30 inproceedingsJ.Justine Galet, A.Antoine Rousseau, H.Hervé Rivano and J.Juliette Chabassier. Des outils de médiation innovants inspirés par des travaux de recherche : 3 exemples.Science and You 2021 - Colloque international Science&YouMetz, FranceNovember 2021
  HALback to text
• 31 inproceedingsV.Victor Martins Gomes, D.Daniel Brito, S.Stéphane Garambois, C.Clarisse Bordes and H.Hélène Barucq. Experiments and Modelling of Seismoelectrics in the Ultrasonic Range: A Comparison With Electrokinetic Theory.NSG2021 27th European Meeting of Environmental and Engineering GeophysicsNSG2021 27th European Meeting of Environmental and Engineering Geophysics[Hybrid], FranceEuropean Association of Geoscientists & EngineersAugust 2021, 1-5
  HALDOI
• 32 inproceedingsC.Christina Morency, E.Eric Matzel, N.Niels Grobbe, D.Daniel Brito, C.Clarisse Bordes, M.Mathieu Bellanger, M.Mathieu Auxiètre, K.Kirsti Midttømme, W.Walter Wheeler, H.Hélène Barucq, J.Julien Diaz and B.Bjarte Fagerås. Seismoelectric effects for geothermal resources assessment and monitoring.IMAGE 2021 - Society of Exploration Geophysicists Annual Meeting and ExpositionDenver, United StatesSeptember 2021
  HAL
• 33 inproceedingsS.Sebastien Pernet, M.Margot Sirdey and S.Sébastien Tordeux. Trefftz method for electromagnetic wave simulation in three dimensions.ICOSAHOM 2020Vienna, FranceJune 2021
  HALback to text
• 34 inproceedingsS.Sebastien Pernet, M.Margot Sirdey and S.Sébastien Tordeux. Trefftz method for three-dimensional electromagnetic wave simulation.SMAI 2021La Grande-Motte, FranceJune 2021
  HALback to text
• 35 inproceedingsN.Nathan Rouxelin, H.Hélène Barucq and S.Sébastien Tordeux. Convergence analysis of HDG formulations for the convected Helmholtz equation.ICOSAHOM 2021 – International conference on spectral and high-order methods 2021Vienna (Online), AustriaApril 2021
  HALback to text
• 36 inproceedingsN.Nathan Rouxelin, H.Hélène Barucq and S.Sébastien Tordeux. Numerical simulation of aeroacoustic wave propagation in the Sun.WCCM ECCOMAS 2020 - 14th World Congress on Computational MechanicsParis (online), FranceJanuary 2021
  HALback to text
• 37 inproceedingsC.Chengyi Shen, D.Daniel Brito, J.Julien Diaz, C.Clarisse Bordes, J.Jean Virieux and S.Stephane Garambois. Tomographic imaging of a carbonate core using different ultrasonic seismic sources at the laboratory scale : piezoelectric vs point-like pulsed-laser.IMAGE 2021 - Society of Exploration Geophysicists Annual Meeting and ExpositionDenver, United StatesSeptember 2021
  HAL
• 38 inproceedingsA.Alexis Thibault, J.Juliette Chabassier, H.Henri Boutin and T.Thomas Hélie. A low reduced frequency model for viscothermal wave propagation in conical tubes of arbitrary cross-section.ICSV27 - 27th International Congress on Sound and VibrationPrague, Czech RepublicJuly 2021
  HALback to text
• 39 inproceedingsV.Vinduja Vasanthan, H.Hélène Barucq, H.Henri Calandra and J.Julien Diaz. PML applied to spacetime Trefftz-DG numerical formulation for the acoustic wave equation.Icosahom 2020 - International Conference on Spectral and High-Order MethodsVienna, AustriaJuly 2021
  HALback to text
• 40 inproceedingsV.Vinduja Vasanthan, H.Hélène Barucq, H.Henri Calandra and J.Julien Diaz. PML applied to spacetime Trefftz-DG numerical formulation for the elastic wave equation.Mathias Days 2021Paris, FranceOctober 2021
  HALback to text

Doctoral dissertations and habilitation theses

• 41 thesisS.Stefano Frambati. Unstructured Isogeometric Analysis with Applications to Seismic Wave Propagation.Université de Pau et des Pays de l'AdourDecember 2021
  HALback to text
• 42 thesisP.Pierre Jacquet. Time-Domain Full Waveform Inversion using advanced Discontinuous Galerkin method.Université de Pau et des Pays de l'AdourFebruary 2021
  HALback to text
• 43 thesisR.-C.Rose-Cloé Meyer. Mathematical modeling and simulation of waves in conducting poroelastic media using HDG method.Université de Pau et des Pays de l'AdourSeptember 2021
  HALback to text
• 44 thesisN.Nathan Rouxelin. Mixed condensed numerical methods for convected wave propagation. Applications in helioseismology.Universite de Pau et des Pays de l'Adour

Reports & preprints

• 45 reportH.Hélène Barucq, F.Florian Faucher, D.Damien Fournier, L.Laurent Gizon and H.Ha Pham. Efficient computation of modal Green's kernels for vectorial equations in helioseismology under spherical symmetry.RR-9433Inria Bordeaux - Sud OuestOctober 2021, 86
  HALback to text
• 46 reportH.Hélène Barucq, N.Nathan Rouxelin and S.Sébastien Tordeux. HDG and HDG+ methods for harmonic wave problems with convection.RR-9410Inria Bordeaux - Sud-Ouest; LMAP UMR CNRS 5142; Université de Pau et des Pays de l'Adour (UPPA), Pau, FRA.June 2021
  HALback to text
• 47 miscH.Hélène Barucq, N.Nathan Rouxelin and S.Sébastien Tordeux. Prandtl-Glauert-Lorentz based Absorbing Boundary Conditions for the convected Helmholtz equation.July 2021
  HALback to text
• 48 reportS.Sebastien Pernet, N.Nadezhda Serdiuk, M.Margot Sirdey and S.Sébastien Tordeux. Discontinuous Galerkin Method based on Riemann fluxes for the time domain Maxwell System.RR-9431INRIA Bordeaux - Sud-OuestOctober 2021, 55
  HALback to text

Scientific popularization

• 49 inproceedingsJ.Justine Galet, A.Antoine Rousseau, H.Hervé Rivano and J.Juliette Chabassier. Des outils de médiation innovants inspirés par des travaux de recherche : 3 exemples.Science and You 2021 - Colloque international Science&YouMetz, FranceNovember 2021
  HALback to text