Keywords
Computer Science and Digital Science
- A5.9.2. Estimation, modeling
- A6.4.1. Deterministic control
- A6.4.4. Stability and Stabilization
- A6.4.5. Control of distributed parameter systems
- A9.5. Robotics
Other Research Topics and Application Domains
- B1.1.8. Mathematical biology
- B2.1. Well being
- B5.6. Robotic systems
- B7.2.1. Smart vehicles
1 Team members, visitors, external collaborators
Research Scientists
- Denis Efimov [Team leader, Inria, Senior Researcher, HDR]
- Leonid Fridman [Inria, Advanced Research Position, from Jun 2021 until Jul 2021, HDR]
- Andrey Polyakov [Inria, Researcher, HDR]
- Rosane Ushirobira [Inria, Researcher, HDR]
Faculty Member
- Jean-Pierre Richard [École centrale de Lille, Professor]
Post-Doctoral Fellows
- Jesus Mendoza Avila [Inria, Dec 2021]
- Gabriele Perozzi [Inria, until Aug 2021]
PhD Students
- Alex Dos Reis De Souza [Inria, until Sep 2021]
- Anatolii Khalin [Inria]
- Min Li [China Scholarship Council, from Oct 2021]
- Wenjie Mei [China Scholarship Council]
- Artem Nekhoroshikh [École centrale de Lille]
- Quentin Voortman [Université de technologie d'Eindhoven - Pays bas, until Feb 2021]
- Yu Zhou [China Scholarship Council]
Technical Staff
- Fiodar Hancharou [Inria, Engineer, until Jul 2021]
Interns and Apprentices
- Benjamin Maerten [Inria, from May 2021 until Aug 2021]
Administrative Assistant
- Amelie Supervielle [Inria]
Visiting Scientists
- Romeo Falcon Prado [Conseil national des sciences et de la technologie - Mexique, from Nov 2021]
- Xinxin Han [China Scholarship Council, from Nov 2021]
External Collaborator
- Gerald Dherbomez [CNRS]
2 Overall objectives
Valse team studies the estimation and control problems arising in the analysis, and the design of distributed, uncertain and interconnected dynamical systems:
- Using the concepts of finite-time/fixed-time/hyperexponential convergence and stability, the main idea is to separate and hierarchize in time the control and estimation processes, which are distributed in space. This greatly simplifies their analysis and the design for large-scale solutions.
- The main areas of investigation and application are Internet of things and cyber-physical systems.
- The team aims to draw up algorithms for decentralized finite-time control and estimation. The methodology to be developed includes extensions of the theory of homogeneous systems and of finite-time/fixed-time/hyperexponential convergence and stability notions. Particular attention is given to applications in real-world scenarios.
- It is a joint proposal with the CNRS CRIStAL UMR 9189 (Centrale Lille and Université de Lille).
3 Research program
The Valse team works in the domains of control science: dynamical systems, stability analysis, estimation, and automatic control. Our developments are focused on the theoretical and applied aspects related to control and estimation of large-scale multi-sensor and multi-actuator systems based on the use of the theories of finite-time/fixed-time/hyperexponential convergence and homogeneous systems. The Lyapunov function method and other methods of analysis of dynamical systems form a basis for the studies in the Valse team.
The key idea of the research program for the team is that a fast (non-asymptotic) convergence of the regulation and estimation errors increases the reliability of intelligent distributed actuators and sensors in complex scenarios, such as interconnected cyber-physical systems (CPSs).
The expertise of Valse's members in theoretical developments of control and estimation theory (finite-time control and estimation algorithms in centralized context 68, 54, 65, 64, 61, homogeneity framework for differential equations 69, 56, 55, 57, 59, 70, 66, time-delay systems 58, 60, 73, distributed systems 67 and algebraic-based methods for estimation 71, 72) is an essential ingredient to achieve our objective.
The generic chart of different goals and tasks included in the scientific work program of Valse and interrelations between them are presented in Fig. 1. We have selected three main objectives to pursue with the related tasks to fulfill:
- The first objective is to design control and estimation solutions for CPS and IoT, which is the principal aim of Valse. It will contain the main outcomes of our research.
- The second objective is more theoretical, which is needed to make the basement for our design and analysis parts in the previous goal.
- The third objective deals with applications, which will drive the team and motivate the theoretical studies and selected design performances.
All these objectives are interconnected: from a particular problem in an IoT application, it is planned to design control or estimation algorithms, leading to the development of theoretical tools; and vice versa, a new theoretical advance can provide a possibility for the development of novel tools, which can be used in applications.
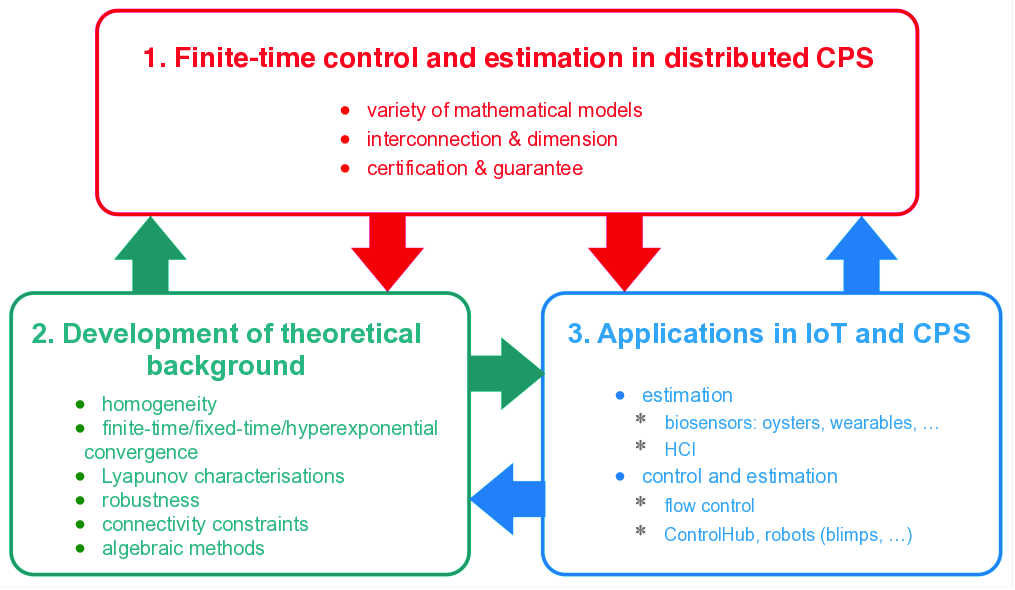
Structure of the objectives and tasks treated in Valse
Structure of the objectives and tasks treated in Valse
To explain our motivation: why use finite-time? Applying any method for control/estimation has a price in terms of its advantages and disadvantages. There is no universal framework that is the best always and everywhere. Finite-time may appear as a luxurious property for a physical system, requiring nonlinear tools. Of course, if an asymptotic convergence and a linear model are enough for solving a given problem, then there is no reason to develop something else. However, most of the present problems in CPS and IoT are nonlinear (i.e. they have various local behaviors that cannot be collected in only one linear model). Design and analysis of various local linearized models and solutions are luxurious, too. The theory of homogeneity can go beyond linearity, offering many new features while not appearing as severe as other nonlinear tools and having almost all hints of the linear framework. Suppose that, thanks to the homogeneity theory, finite-time/fixed-time can be obtained with little difficulty while adding the bonuses of stronger robustness and faster convergence compared to the linear case? We are convinced that the price of going beyond linear control and estimation can be strongly dropped down by maturing the theory of homogeneity and finite/fixed-time convergence. We are also convinced that it will be compensated in terms of robustness and speed, which can be demanded in the new areas of application as IoT, for example.
4 Application domains
An objective of the team is the application of the developed control and estimation algorithms for different scenarios in IoT or CPSs. The participation in various potential applications allows Valse team to better understand the features of CPSs and their required performances, and to properly formulate the control and estimation problems that have to be solved. Here is a list of ongoing and potential applications addressed in the team:
- smart bivalve-based biosensor for water quality monitoring (ANR project
WaQMoS, the developed
sensor is shown in Fig. 2): in living beings,
the presence of persistent external perturbations may be difficult
to measure, and important model uncertainties render the application
of conventional techniques complicated; another issue for estimation
is the consensus-seeking between animals for a contamination detection 52;

Figure 2: The valvometer used in the ANR project WaQMoS
- control and estimation for flying vehicles, e.g. quadrotors
or blimps given in Fig. 3: the nonlinearity of the model and its
uncertainty coupled with important aerodynamic perturbations have
to be compensated by fast (finite- or fixed-time) and robust control
and estimation algorithms;

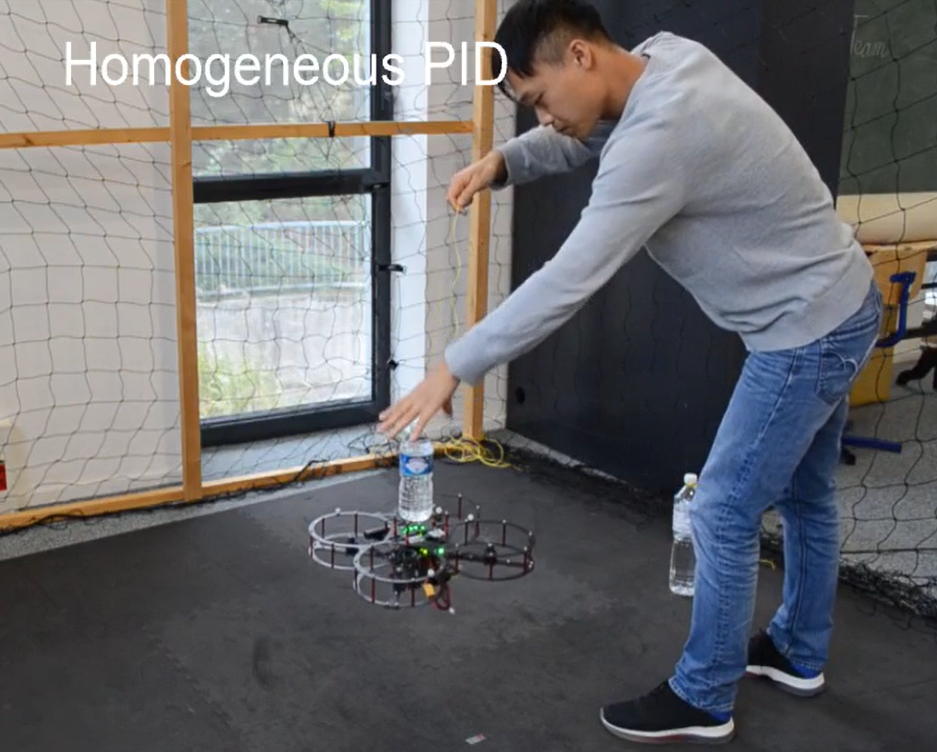
Figure 3: Blimp and quadrotor robots
- human behavior modeling and identification with the posterior design of algorithms for human-computer interaction (with the Inria team LOKI): robust finite-time differentiators demonstrate good estimation capabilities needed for prediction in this application 72, 53;
- human physiological characteristics estimation (like emotion detection, galvanic skin response filtering, fatigue evaluation in collaborations with Neotrope and Ellcie Healthy): intelligent robust filtering and finite-time distributed estimation are key features in these scenarios;
- path planning for autonomous vehicles taking into account the behavior of humans (with the Inria team SCOOL): application of interval and finite-time adaptive estimation and prediction techniques allows for treating the uncertainty of the environment by reducing the computational complexity of reinforcement learning 631;
- flow control (in the framework of ContrATech subprogram of CPER ELSAT,
see also 62): the case of control and
estimation of a distributed-parameter system with very fast and uncertain
dynamics, where finite-time solutions developed by Valse are necessary (an example of results is given in Fig. 4);

Figure 4: Particle Image Velocimetry on flow control for an Ahmed body (LAMIH wind tunnel)
- control of synthetic microbial communities (in the framework of IPL COSY): here again, the problem is an important uncertainty of the model, which can be handled by robust sliding mode control algorithms, or by applying adaptive finite-time estimation and identification tools;
It is worth highlighting a widespread distribution of various scientific domains in the list of applications for the team given above. Such an interdisciplinarity for Valse is unsurprising since the control theory is a science of systems whose interest today is, by nature, to interface with other disciplines and their fields of application. This is also well aligned with the domain of CPSs, which by its origin requires multidisciplinary competencies.
5 Highlights of the year
- A big survey on the theory of finite/fixed-time convergent and homogeneous systems has appeared in 20.
- A survey on digital implementation of sliding-mode control via the implicit method that preserves the finite-time convergence rates in discretization has been published in 14.
- ControlHub is open for testing.
5.1 Awards
- Abertis Prize, Best Doctoral Thesis on Road Safety, 2021, E. Leurent
- Best PhD Award CNRS (GdR MACS & Club EEA), 2021, E. Leurent
6 New software and platforms
ControlHub is the platform for rapid prototyping of control algorithms for CPSs. It allows an end-user to have remote access to real experimental setups to validate estimation and control algorithms (developed by the user in Matlab or C/C++). The generic structure of the ControlHub platform is shown in Fig. 5. The platform's role is to connect the experimental setups and web server, which is realizing a gate to the platform. The experimentation server must be installed for each setup to allow remote access. The web server federates the access to the platform and manages the resources over the web. Thanks to features of the already existing WebLab-Deusto software, it is possible to manage multiple user accounts and simultaneous permissions and supervise the online experiments automatically (e.g., to add a new control/estimation experiment and integrate it into the framework). The ControlHub platform is available now for Inria users: ControlHub
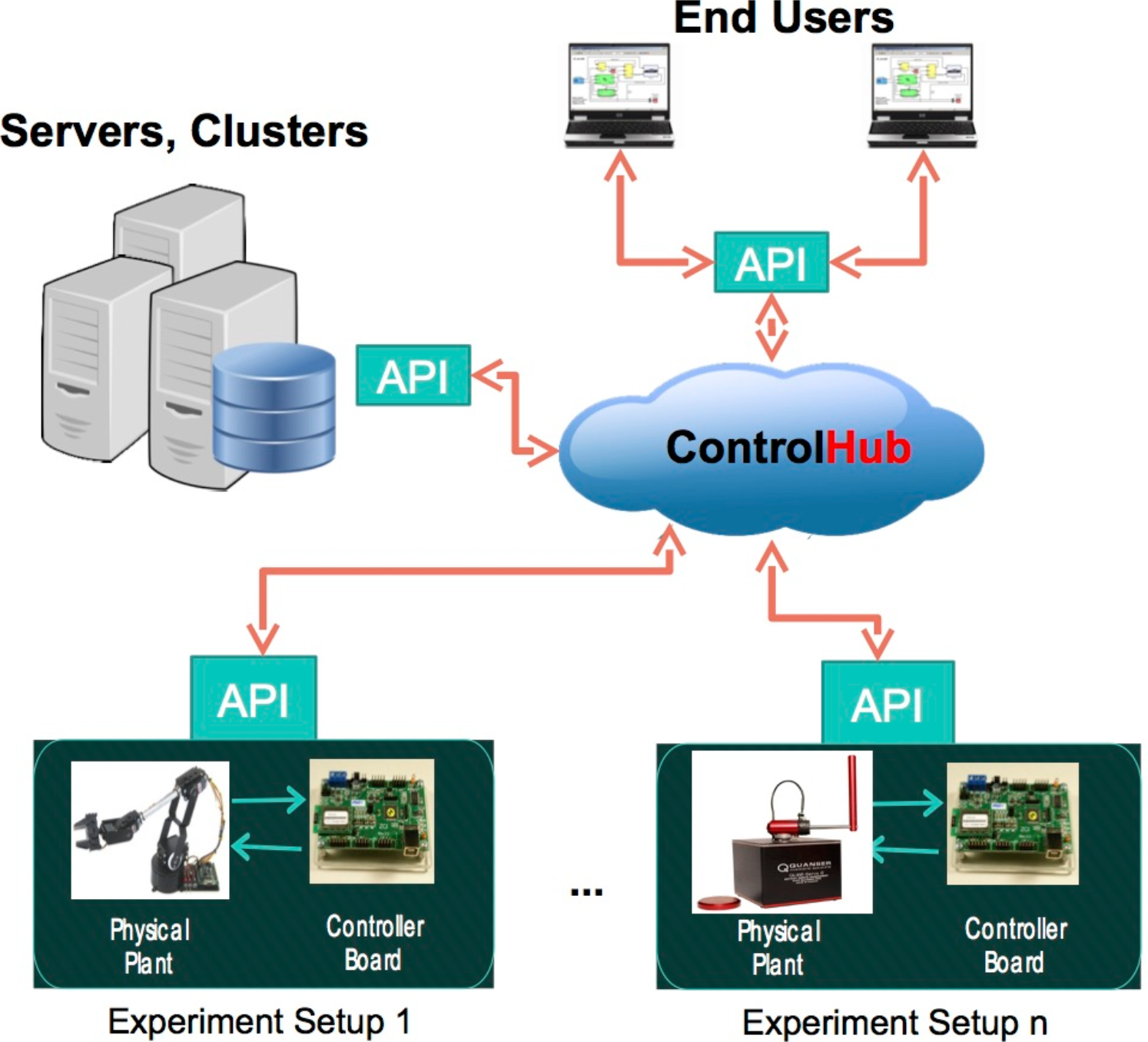
Structure of ControlHub platform
Structure of ControlHub platform
7 New results
7.1 Interval prediction for COVID-19 outbreak
Participants: Denis Efimov, Rosane Ushirobira.
In 22, a new version of the well-known epidemic mathematical SEIR model is used to analyze the pandemic course of COVID-19 in eight different countries. One of the proposed model’s improvements is to reflect the societal feedback on the disease and confinement features. The SEIR model parameters are allowed to be time-varying, and the ranges of their values are identified by using publicly available data for France, Italy, Spain, Germany, Brazil, Russia, New York State (US), and China. The identified model is then applied to predict the SARS-CoV-2 virus propagation under various conditions of confinement. For this purpose, an interval predictor is designed, allowing variations and uncertainties in the model parameters to be considered. The code and the utilized data are available on Github.7.2 Distributed systems
Participants: Denis Efimov, Jean-Pierre Richard, Rosane Ushirobira.
Many systems in the natural and physical world often work in unison with similar other systems. This process of simultaneous operation is known as synchronization. Due to this phenomenon's importance in the past few decades, extensive research efforts have been made. However, many existing results consider that the systems are identical and/or linear time-invariant, while practical systems are often nonlinear and nonidentical for various reasons.The paper 7 summarizes some recent results on the synchronization of heterogeneous nonlinear systems. First, the results on the synchronization of a particular class of robustly stable nonlinear systems are presented. Then, these results are applied to an example model known as the Brockett oscillator. Finally, using the Brockett oscillator as a common dynamics, output oscillatory synchronization results are given for heterogeneous nonlinear systems of relative degree 2 or higher. An application example of a Brockett oscillator for power-grid synchronization is also presented.
In 32, we provide novel conditions for stability analysis of aperiodically sampled nonlinear control systems subjected to time-varying delay. The proposed approach can also deal with cases in which delay is larger than the sampling interval. It is applicable to a general class of nonlinear systems and provides sufficient criteria for stability that aid in making trade-offs between control performance and the bounds on sampling interval and delay. As a stepping stone, a preliminary and generic result based on dissipativity, is introduced to analyse the exponential stability of a class of feedback-interconnected systems. The paper 33 deals with exponential stability analysis of decentralized, sampled-data, linear time-invariant systems with asynchronous sensors and actuators. We consider the case where each controller in the decentralized setting has its own sampling and actuation frequency, which translates to asynchrony between sensors and actuators. Additionally, asynchrony may be induced by delays between the sampling instants and actuation update instants as relevant in a networked context. By characterizing the properties of the operators using small-gain type integral quadratic constraints, we provide criteria for exponential stability of the asynchronous, decentralized state-space models. This group of results is obtained in collaboration with SHOC team of CRIStAL lab of CNRS.
7.3 Consistent discretisation of homogeneous systems
Participants: Andrey Polyakov.
The paper 15 deals with the practical implementation of implicit homogeneous controllers (IHCs) for linearized mechanical systems. The control design includes the methodology to get gains of the IHC based on the linearized approximation of the system. If the approximation error enforced by the linearization is vanishing with the state, locally measurable and bounded, the IHC can lead the state to the origin in finite-time. This IHC control allows accelerating the convergence rate of the states. A semi-explicit algorithm is provided to exert the digital implementation of the controller. The application of the bisection method estimates the controller gain ensuring the finite-time convergence of the state to the origin. A complementary analysis provides a simplified algorithm with a reduced number of computation stages, but equally efficient for gain estimation. The proposed IHC is applied to a rotary inverted pendulum QUBE™ Servo 2 platform of Quanser®.The goal of 42 is to investigate if it is possible to upgrade a given linear controller to a sliding mode one with an improvement of the control performance. Starting from a given linear controller, a design procedure for a sliding mode control having better performance than the linear one, is proposed. If the system has disturbances, which is always the case in experiments, the upgraded sliding mode control brings also a better robustness with respect to the given linear robust controller. The main idea is to divide the state-space into two areas, introducing a design parameter which separates the area of the linear control from the area of the sliding mode control. Some issues related to the chattering reduction are discussed. The control scheme's efficiency is demonstrated experimentally on a rotary inverted pendulum. The experimental results demonstrate the effectiveness of the obtained controls, and show an improvement with respect to the given linear proportional control.
A survey on this subject can be found in 14. The latter works are done in collaboration with TRIPOP team of Inria.
7.4 Chemostat control and estimation
Participants: Denis Efimov, Andrey Polyakov.
In 17, the problem of state estimation of a microbial consortium is discussed. This microbial consortium is composed of two strains, called producer and cleaner, in continuous culture. The producer strain grows by consuming glucose, while the cleaner grows by consuming the acetate excreted by the producer and toxifies the environment. Considering two available measurements, the total biomass and a fluorescent reporter, an observability analysis is cast and state estimators are proposed for each state component of this system. Although the system is shown to be detectable using solely the total biomass as measurement, the advantage of measuring the fluorescent reporter on the observer design is highlighted.7.5 Analysis and design of homogeneous and finite-time stable systems
Participants: Denis Efimov, Andrey Polyakov, Rosane Ushirobira.
In 1, fixed-time and finite-time stability properties of nonlinear systems are investigated using the introduced notions of sub-and sup-homogeneity. These concepts allow the systems to be analyzed using the homogeneity-based ideas and tools, even if they do not admit homogeneous approximations. It is demonstrated that finite-time and fixed-time stability properties can be established using homogeneity degree of sub-and sup-homogeneous extensions.The aim of 11 is to design a non-parametric identifier for homogeneous systems based on a class of artificial neural networks with continuous dynamics. The identification algorithm is developed for input-affine systems with uncertain gains and diverse degrees of homogeneity. One of the main contributions of this study is the extension of the universal approximation property of neural networks for continuous homogeneous systems. Another contribution is the development of a differential non-parametric identifier based on the novel concept of homogeneous neural networks. The adjustment laws for the weights are obtained from a Lyapunov stability analysis taking homogeneity properties of the system into account. The ultimate boundedness of the origin for the identification error is demonstrated using the persistent excitation condition. The effectiveness of the proposed identifier is verified by the simulation of the three-tank homogeneous model. In this example, the proposed identification scheme is compared with a classical ANN identifier, and we present a statistical analysis of such comparison.
The paper 18 is devoted to stability analysis of discrete-time time-delay systems, obtained after explicit Euler discretization of (locally) homogeneous continuous-time models. The results are derived by applying the Lyapunov-Krasovskii theory. A generic structure of the functional is given that suits for any homogeneous system of non-zero degree (and it can also be used for any dynamics admitting a homogeneous approximation). The obtained conditions are utilized to demonstrate stability for a discretized delayed locally homogeneous planar system with negative and positive degrees.
The paper 27 deals with the analytic and numeric design of a Lyapunov function for homogeneous and discontinuous systems. First, the presented converse theorems provide two analytical expressions of homogeneous and locally Lipschitz continuous Lyapunov functions for homogeneous discontinuous systems of negative homogeneity degree, generalizing classical results. Second, a methodology for the numerical construction of those Lyapunov functions is extended to the class of systems under consideration. Finally, the developed theory is applied to the numerical design of a Lyapunov function for some Higher-Order Sliding Mode (HOSM) algorithms.
In 28, continuous and discontinuous integral controllers are designed for a large class of nonlinear systems, which are (partially) feedback linearizable. These controllers of arbitrary positive or negative degree of homogeneity are derived by combining a Lyapunov function obtained from the Implicit Lyapunov Function (ILF) method with some extra explicit terms. Discontinuous integral controllers are able to stabilize an equilibrium or track a time-varying signal in finite time, while rejecting vanishing uncertainties and non-vanishing Lipschitz matching perturbations. Continuous integral controllers achieve asymptotic stabilization despite non-vanishing constant perturbations in finite-time, exponentially or nearly fixed-time for negative, zero or positive homogeneity degree, respectively.
A procedure of upgrading a linear observer to homogeneous (nonlinear) one is proposed and validated by experiment in 35. The nonlinear observer design is based on the the concept of a linear geometric homogeneity. The simple procedure developed to apply the homogeneous nonlinear observer is based on the parameters provided by the linear observer, which is potential for many applications to improve their performance. A saturation function is introduced to guaranteed the improvement of estimation quality. Finally the theoretical results are confirmed by the QDrone platform of Quanser®. The work in this direction is done in collaboration with DEFROST team of Inria.
A survey on finite/fixed-time stable and homogeneous systems, with related control and estimation tools, is presented in 20.
7.6 Adaptive control and estimation
Participants: Denis Efimov.
In 24, a robust nonlinear Model Reference Adaptive Control (MRAC) is proposed for disturbed linear systems, i.e., linear systems with parameter uncertainties, and external time-dependent perturbations or nonlinear unmodeled dynamics matched with the control input. The proposed nonlinear control law is composed of two nonlinear adaptive gains. Such adaptive gains allow the control to counteract the effects of some perturbations and nonlinear unmodeled dynamics ensuring asymptotic convergence of the tracking error to zero, and the boundedness of the adaptive gains. The nonlinear controller synthesis is given by a constructive method based on the solution of Linear Matrix Inequalities (LMIs).A high-order sliding-mode adaptive observer is proposed in 31 to solve the problem of adaptive estimation, i.e., the simultaneous estimation of the state and parameters, for a class of uncertain nonlinear systems in the presence of external disturbances, that does not need to satisfy a relative degree condition equal to one. This approach is based on a HOSM observer and a nonlinear parameter identification algorithm. The practical, global and uniform asymptotic stability of the adaptive estimation error, despite the external disturbances, is guaranteed through the small-gain theorem. The convergence proofs are developed based on Lyapunov and input-to-state stability theories.
7.7 Model for pointing tasks with computer mouse
Participants: Denis Efimov, Rosane Ushirobira.
The paper 25 proposes a new simplified pointing model as a feedback-based dynamical system, including both human and computer sides of the process. It takes into account the commutation between the correction and ballistic phases in pointing tasks. We use the mouse position increment signal from noisy experimental data to achieve our main objectives: to estimate the model parameters online and predict the task endpoint. Some estimation tools and validation results, applying linear regression techniques on the experimental data are presented. We also compare with a similar prediction algorithm to show the potential of our algorithm's implementation. This work is done in collaboration with the LOKI team of Inria.7.8 Control of PDEs
Participants: Andrey Polyakov.
In 29, the Input-to-State Stability (ISS) of homogeneous evolution equations in Banach spaces with unbounded linear operators and locally Lipschitz nonlinearities in the right-hand sides is studied. A new homogeneous converse Lyapunov theorem is presented. Similarly to finite-dimensional models, it is shown that the uniform asymptotic stability of an unperturbed homogeneous evolution equation implies its ISS with respect to homogeneously involved exogenous inputs.The existence of a locally Lipschitz continuous homogeneous Lyapunov function is proven for a class of asymptotically stable homogeneous infinite dimensional systems with unbounded nonlinear operators in 5.
7.9 Control and estimation for Persidskii systems
Participants: Denis Efimov, Rosane Ushirobira.
Generalized Persidskii systems represent the dynamics described by the superposition of a linear part with multiple sector nonlinearities and exogenous perturbations. They can be used to model many physical and engineering phenomena.The conditions of (integral) ISS and input-output-to-state stability are established for a class of generalized Persidskii systems in 19. The proposed conditions are formulated using LMIs. Based on these results, the convergence conditions are derived for discretizations of this class of models obtained by the explicit and implicit Euler methods. The proposed theory is finally applied to design a robust stabilization control.
For a class of generalized Persidskii systems, the conditions of practical stability, instability, and oscillatory behavior in the sense of Yakubovich are established in 34. For this purpose, the conditions of local instability at the origin and global boundedness of solutions (practical ISS) are developed in the form of LMIs. The proposed theory is applied to investigate robustness to unmodeled dynamics of nonlinear feedback controls in linear systems and determine the presence of oscillations in the models of neurons.
In 4, we study a class of generalized Persidskii systems with external disturbances and establish conditions, in the form of LMIs, for input-to-output stability (IOS) and robust synchronization for these systems. We apply the obtained results to the robust control design for synchronizing linear systems and the synchronization of Hindmarsh-Rose models of neurons.
The convergence conditions for a class of generalized Persidskii systems and their discretized dynamics are introduced in 26, which can be checked through linear (matrix) inequalities. The case of almost periodic convergence for this class of systems with almost periodic input is also studied. The proposed results are applied to a Lotka-Volterra model and an opinion dynamics.
In the article 8, the stability of switched time-delay Persidskii systems is studied. Both cases of synchronous and asynchronous commutation are considered. A comparison of stability conditions obtained with Lyapunov–Razumikhin and Lyapunov–Krasovskii approaches is provided. The developed theory is applied to the stability analysis of mechanical systems with linear velocity forces and switched nonlinear positional ones.
7.10 Design and analysis in switched systems
Participants: Denis Efimov.
The paper 23 investigates fixed-time and finite-time stability of switched nonlinear time-varying time-delayed systems. It is shown that by assuming these kinds of stability for each subsystem in the family, similar properties can be ensured for the whole system under commutation with a sufficiently big (average) dwell time. The estimates on admissible dwell-time values are derived. Compared with existing results, the novelties of the obtained results lie in that: (i) A class of Lyapunov–Krasovskii functionals with indefinite derivative is constructed for switched nonlinear time-delay systems, (ii) Average dwell time switching laws are designed for the fixed-time and finite-time stability of the systems, and (iii) The presented approach has potential applications in fixed-time, and finite-time stability of other hybrid systems.In 9, stability analysis for a class of switched nonlinear time-delay systems is performed by applying Lyapunov-Krasovskii and Lyapunov-Razumikhin approaches. It is assumed that each subsystem in the family is homogeneous (of positive or negative degree) and asymptotically stable in the delay-free setting. The cases of the existence of a common or multiple Lyapunov-Krasovskii functionals and a common Lyapunov-Razumikhin function are explored. The scenarios with synchronous and asynchronous switching are considered, and it is demonstrated that depending on the kind of commutation, one of the frameworks for stability analysis outperforms another, but finally leads to similar restrictions for both types of switching (despite the asynchronous one seems to be more demanded). The obtained results are applied to mechanical systems having restoring forces with real-valued powers.
We consider the problem of state estimation for a parameter-varying system that is unobservable for some values of time-varying parameters in 10. The set of parameter values is divided into a finite number of subsets for which the system is observable, and a switched observer is proposed. A dwell-time condition is defined that ensures exponential convergence. The stability conditions are formulated in the form of LMIs, which can be used for gains tuning. The obtained theoretical results are supported with an illustrative example and experimental studies for a reaction-wheel pendulum testbench (see the related video on our site).
7.11 Analysis and design for time-delay systems
Participants: Denis Efimov, Rosane Ushirobira.
In the papers 13, 12 we address the problem of adaptive state observation of affine-in-the-states time-varying systems with delayed measurements and unknown parameters. The case with known parameters has been studied by many researchers where, similarly to the approach adopted here, the system is treated as a linear time-varying system. We show that the parameter estimation-based observer (PEBO) design provides a straightforward solution for the unknown parameter case. Moreover, when PEBO is combined with the dynamic regressor extension and mixing (DREM) estimation technique, the estimated state converges in fixed-time with extremely weak excitation assumptions.The paper 3 provides the formulae connecting the Laguerre spectrum of the output signal of a linear discrete-time time-invariant system to the Laguerre spectrum of its input signal and presents a standard state-space system description. The Laguerre domain system representation is meaningful when the input signal is square-summable and the system is stable. A particular type of polynomials arising in the output spectrum evaluation due to the presence of time delay is defined, and key properties of these are investigated. The polynomials are characterized by a three-term recurrence relation that facilitates their numerically reliable calculation.
7.12 MPC with interval tools
Participants: Denis Efimov.
The work 30 addresses the problem of robust output feedback model predictive control (MPC) for discrete-time, constrained linear systems corrupted by (bounded) state and measurement disturbances. Using the available information on measurements and uncertainty bounds, the objective is to stabilize such a system while robustly respecting the imposed constraints on state and control. To this end, interval observer and predictor with guaranteed performance are incorporated into the classic MPC scheme. This new approach offers advantages such as enlarged feasible regions for the optimal control problem, low computational burden, and ease of design. An extension of these results to time-delay systems is presented in 21.In 2, the problem of robust output feedback MPC for discrete-time, constrained, linear parameter-varying systems subject to (bounded) state and measurement disturbances is studied. The vector of scheduling parameters is assumed to be an unmeasurable signal taking values in a given compact set. The proposed controller incorporates an interval observer that uses the available measurement to update the set-membership estimation of the states, and an interval predictor, used in the prediction step of the MPC algorithm. The resulting MPC scheme guarantees recursive feasibility, constraint satisfaction, and ISS in the terminal set. Furthermore, this novel algorithm shows low computation complexity and ease of implementation (similar to conventional MPC schemes).
7.13 Applications
Participants: Denis Efimov, Jean-Pierre Richard, Rosane Ushirobira.
1)
Fast and accurate frequency estimation of an unbalanced three-phase power system in the presence of measurement offset is a challenging task. A simple to implement and tune method is proposed in 6 through a delay-based linear regression framework. Using two challenging scenarios, we verify the performance of our procedure in the presence of severe grid abnormalities, including voltage unbalance, harmonics, frequency jump, and DC offset. Comparative hardware-in-the-loop experimental results demonstrate the suitability of the proposed approach.
2)
According to the World Health Organization, a child dies from malaria every two minutes worldwide. When prescribing antimalarial drugs, a challenging problem for physicians consists in estimating sequestered parasites Plasmodium falciparum populations from noisy measurements of circulating parasite concentrations while handling uncertainty. In 16, we design an interval state estimator for uncertain models of malaria patients. We consider the ubiquitous case in which only intervals of admissible values are available for all parameters in the model except for infection rates, whose bounding values are unavailable. Furthermore, we compute optimal gains for the interval observer by using linear programming to minimize the estimated interval width. We test the observer's efficiency in simulation for a model and real measured data collected by the US Public Health Service at the National Institutes of Health laboratories in Columbia, South Carolina, and Milledgeville, Georgia.
3)
The paper 37 deals with the problem of robust blood glucose regulation in critically ill patients affected by type 1 diabetes mellitus. The blood glucose measurement and insulin infusion are intravenous. The proposed algorithm regulates blood glucose and keeps it in the normoglycemia range, i.e., 70–180 mg/dl. To this aim, a control law is proposed based on a nonlinear MRAC approach. The algorithm is composed of nonlinear adaptive gains that ensure convergence to zero of the regulation error. The approach is validated in the UVA/Padova metabolic simulator for ten in silico adult patients with unannounced meals.
4)
In 44, we consider the problem of remote observation of a unicycle-type mobile robot through a data rate constrained communication channel, which can only send a limited number of bits per unit of time. The objective is to reconstruct estimates of the state of the robot at the remote location through the messages that are sent. The design of the communication protocol should ensure that the maximum observation error is bounded whilst using as few bits per unit of time as possible. An event-triggered observation scheme is developed specifically for the unicycle-type robot. This observer is tested through experiments on Turtlebots. The experiments show that the event-triggered scheme is very efficient at reducing the average number of required communications.
8 Bilateral contracts and grants with industry
8.1 Bilateral contracts with industry
Participants: Denis Efimov, Rosane Ushirobira.
- 52Hz is a startup (Brest, France) that develops an underwater communication device for divers. The goal of this contract was to develop an intelligent filtering algorithm that compensates for the voice deformation during underwater vocal communication through the device. The code for the filter was written in Matlab, with further help for its adaptation in Python and C.
9 Partnerships and cooperations
9.1 International initiatives
9.1.1 Participation in other International Programs
Participants: Denis Efimov, Andrey Polyakov, Rosane Ushirobira.
Acronym:
ECOS-Nord AICAMR
-
Title:
Artificial Intelligence-based Control Approaches for Multiple Mobile Robots
-
Partner Institution(s):
- Tecnológico Nacional de México, Torreon, Mexico
-
Date/Duration:
2021 – 2023
-
Additional info/keywords:
This project is focused on studying several tracking tasks for autonomous mobile robot systems, particularly unmanned aerial vehicles and wheeled mobile robots. This project aims to develop robust control and navigation schemes by combining the methods of artificial intelligence and control theory.
Acronym:
Inria Northern European Associate Team WeCare
-
Title:
Efficient Estimation and Control Algorithms in Wearable Devices for Health and Care
-
Partner Institution(s):
- Uppsala University, Sweden
-
Date/Duration:
2018 – 2022
-
Additional info/keywords:
This collaborative project aims to combine Control Theory and Differential Algebra methods to design effective algorithms for control, detection, and estimation in human wearable devices or human medical and well-being applications.
9.2 International research visitors
9.2.1 Visits of international scientists
Inria International Chair
- L. Fridman, June–July, 2021
Other international visits to the team
Xinxin Han
-
Status
PhD
-
Institution of origin:
HIT
-
Country:
China
-
Dates:
11/2021–10/2022
-
Context of the visit:
China Scholarship Council
-
Mobility program/type of mobility:
internship
Romeo Falcon Prado
-
Status
PhD
-
Institution of origin:
Tecnológico Nacional de México, Torreon
-
Country:
Mexico
-
Dates:
11/2021–12/2021
-
Context of the visit:
ECOS-Nord AICAMR
-
Mobility program/type of mobility:
research stay
9.2.2 Visits to international teams
Research stays abroad
Andrey Polyakov
-
Visited institution:
Tecnológico Nacional de México, Torreon
-
Country:
Mexico
-
Dates:
11/2021
-
Context of the visit:
ECOS-Nord AICAMR
-
Mobility program/type of mobility:
research stay
Yu Zhou
-
Visited institution:
Tecnológico Nacional de México, Torreon
-
Country:
Mexico
-
Dates:
11/2021–12/2021
-
Context of the visit:
ECOS-Nord AICAMR
-
Mobility program/type of mobility:
research stay
9.3 National initiatives
9.3.1 ANR
- Digitslid (Digital set-valued and homogeneous sliding mode control and differentiators: the implicit approach), coordinator B. Brogliato (Inria, Grenoble)
- Finite4SoS (Finite Time Control and Estimation for System of Systems), coordinator W. Perruquetti (École Centrale de Lille)
- WaQMoS (Coastal waters quality surveillance using bivalve mollusk-based sensors), coordinator D. Efimov (Inria, Lille)
- SyNPiD (Synchronization in power networks with periodic dynamics), coordinators D. Efimov (Inria, France) and J. Schiffer (Brandenburg University of Technology Cottbus-Senftenberg, Germany)
9.3.2 Inria project labs
The team participates in IPL COSY (real-time COntrol of SYnthetic microbial communities), coordinator E. Cinquemani (Inria, Grenoble).
10 Dissemination
10.1 Promoting scientific activities
10.1.1 Scientific events: selection
Member of the conference program committees
- 2022, IFAC ALCOS, Casablanca, Morocco, D. Efimov
- 2021, IEEE SysTol, Saint-Rafaël, France, D. Efimov
Reviewer
The members of the team participate in reviewing of the major international conferences: IEEE CDC, ECC
10.1.2 Journal
Member of the editorial boards
- Associate Editor, Asian Journal of Control, D. Efimov, R. Ushirobira
- Associate Editor, Automatica, D. Efimov
- Associate Editor, Trends in Computational and Applied Mathematics, R. Ushirobira
- Associate Editor, IEEE Transactions on Automatic Control, D. Efimov
- Associate Editor, IFAC Journal on Nonlinear Analysis: Hybrid Systems, D. Efimov
- Associate Editor, Automation and Remote Control, A. Polyakov
Reviewer - reviewing activities
The members of the team participate in reviewing of the major international journlas: IEEE TAC, Automatica, IJRNC, IJC, EJC, SIAM CON
10.1.3 Scientific expertise
- Member of IFAC TC 1.2. Adaptive and Learning Systems, D. Efimov
- Publication vice-chair of IFAC TC 9.2. Systems and Control for Societal Impact, D. Efimov
- Executive committee member, IEEE CSS Technical Committee on Variable Structure and Sliding Mode Control, D. Efimov
- Co-chair of European Ph.D. Award on Control for Complex and Heterogeneous Systems, D. Efimov
- Member of IFAC TC 2.3 Nonlinear Control Systems, A. Polyakov
10.1.4 Research administration
- Member of the operation committee of CRNS FR TTM, A. Polyakov
- Member of the CIMPA Executive team, Scientific Officer, R. Ushirobira
10.2 Teaching - Supervision - Juries
10.2.1 Teaching
- Master: J.-P. Richard, Dynamical systems, 10h, M2, Université de Lille
- Licence: R. Ushirobira, Basic courses in Linear algebra and Calculus, 71h, L3, Polytech Lille
- Master: D. Efimov, Dynamical systems, 17h, M2, Université de Lille
- Licence: D. Efimov, Estimation for engineers, 24h, L3, Centrale Lille
10.2.2 Supervision
- Ph.D. in progress: Min Li, since 10/21, A. Polyakov (50%)
- Ph.D. in progress: Yu Zhou, A. Polyakov (50%)
- Ph.D. in progress: Anatolii Khalin, since 01/10/19, R. Ushirobira, D. Efimov, G. Casiez
- Ph.D. in progress: Wenjie Mei, since 01/10/19, D. Efimov, R. Ushirobira
- Ph.D. in progress: Artem Nekhoroshikh, D. Efimov (50%), A. Polyakov (40%)
- Ph.D. defended: Alex dos Reis de Souza. Defended 09/21, D. Efimov (50%), A. Polyakov (40%)
- Ph.D. defended: Quentin Voortman. Defended 02/21, J-P. Richard (10%), D. Efimov (30%)
10.2.3 Juries
The members of the team participate in juries on different levels, such as PhD/HdR or CR/MCF recruitment.
10.3 Popularization
-
Médiation
R. Ushirobira is in charge of the scientific mediation mission of the center
10.3.1 Articles and contents
-
The Conversation
A. Reis wrote an article entitled Microbes can produce food, fuel and medicine - here's how maths can make them even more useful around his Ph.D. thesis in The Conversation, July 2021
10.3.2 Interventions
-
JSI2021
A talk On oysters and sustainable digital was given in June 2021, by R. Ushirobira in the Journées Scientifiques d'Inria, an internal Inria scientific meeting
-
RJMI
A RJMI meeting (Meeting for Mathematicians and Computer Scientists Young Girls) was organized by R. Ushirobira in October 2021. In this two-days meeting, the students attend conferences and work on a particular project
-
CHICHE
In March and December 2021, R. Ushirobira presented five talks to 1st year high-school students at the Lycée Queneau (Villeneuve d'Ascq, France), within the French national CHICHE framework: through this nationwide operation, the aim is to encourage students' taste for digital sciences through meeting with scientists who illustrate “research” in this field and its impact for society pedagogically and attractively
11 Scientific production
11.1 Major publications
- 1 articleOn finite/fixed-time stability analysis based on sup-and sub-homogeneous extensions.Systems and Control Letters150April 2021
- 2 articleRobust output feedback MPC for LPV systems using interval observers.IEEE Transactions on Automatic ControlJuly 2021
- 3 articleLinear time-invariant discrete delay systems in Laguerre domain.IEEE Transactions on Automatic Control2021
- 4 articleOn input-to-output stability and robust synchronization of generalized Persidskii systems.IEEE Transactions on Automatic Control2021
- 5 articleInput-to-State Stability of Homogeneous Infinite Dimensional Systems with Locally Lipschitz Nonlinearities.Automatica2021
11.2 Publications of the year
International journals
International peer-reviewed conferences
Reports & preprints
11.3 Cited publications
- 52 articleVelocity estimation of valve movement in oysters for water quality surveillance.IFAC-PapersOnLine482015, 333--338
- 53 conferenceFrequency Domain Forecasting Approach for Latency Reduction in Direct Human-Computer Interaction.Proc. 56th IEEE Conference on Decision and Control (CDC)Melbourne2017
- 54 articleRobust finite-time output feedback stabilization of the double integrator.International Journal of Control8832015, 451--460
- 55 articleOn Homogeneity and Its Application in Sliding Mode.Int. J. Franklin Institute35142014, 1866--1901
- 56 articleVerification of ISS, iISS and IOSS properties applying weighted homogeneity.Systems & Control Letters622013, 1159--1167
- 57 articleOn conditions of oscillations and multi-homogeneity.Mathematics of Control, Signals, and Systems2812015, 1--37URL: http://dx.doi.org/10.1007/s00498-015-0157-y
- 58 articleDevelopment of Homogeneity Concept For Time-Delay Systems.SIAM Journal on Optimization and Control5232014, 1403--1808
- 59 articleRealization and Discretization of Asymptotically Stable Homogeneous Systems.IEEE Trans. Automatic Control62112017, 5962--5969
- 60 articleWeighted Homogeneity for Time-Delay Systems: Finite-Time and Independent of Delay Stability.IEEE Trans. Automatic Control6112016, 210--215
- 61 articleBoundary time-varying feedbacks for fixed-time stabilization of constant-parameter reaction-diffusion systems.Automatica1032019, 398--407URL: https://doi.org/10.1016/j.automatica.2019.02.013
- 62 articleSISO model-based control of separated flows: Sliding mode and optimal control approaches.International Journal of Robust and Nonlinear Control27182017, 5008-5027
- 63 conferenceInterval Prediction for Continuous-Time Systems with Parametric Uncertainties.Proc. 58th IEEE Conference on Decision and Control (CDC)Nice2019
- 64 articleFinite-time and Fixed-time Observer Design: Implicit Lyapunov function approach.Automatica8712018, 52-60
- 65 articleOn Homogeneous Finite-Time Control for Linear Evolution Equation in Hilbert Space.IEEE Transactions on Automatic Control2018
- 66 articleConsistent Discretization of Finite-time and Fixed-time Stable Systems.SIAM Journal on Optimization and Control5712019, 78--103
- 67 articleOn Homogeneous Distributed Parameter Systems.IEEE Trans. Automatic Control61112016, 3657--3662
- 68 articleFinite-time and fixed-time stabilization: Implicit Lyapunov function approach.Automatica512015, 332-340
- 69 articleNonlinear feedback design for fixed-time stabilization of linear control systems.IEEE Transactions on Automatic Control57(8)2012, 2106-2110
- 70 articleTime-Varying Parameter Identification Algorithms: Finite and Fixed-Time Convergence.IEEE Transactions on Automatic Control6272017, 3671--3678URL: https://dx.doi.org/10.1109/TAC.2017.2673413
- 71 conferenceEstimating the infection rate of a SIR epidemic model via differential elimination.Proceedings of ECCNaples2019
- 72 conferenceA forecasting algorithm for latency compensation in indirect human-computer interactions.Proceedings of ECCAlborg2016, 1081--1086
- 73 articleA note on delay robustness for homogeneous systems with negative degree.Automatica7952017, 178--184

