2023Activity reportProject-TeamQUANTIC

2.1 Overall objectives

The research activities of QUANTIC team lie at the border between theoretical and experimental efforts in the emerging field of quantum systems engineering. Our research topics are in direct continuation of a historic research theme of INRIA, classical automatic control, while opening completely new perspectives toward quantum control: by developing a new mathematical system theory for quantum circuits, we will realize the components of a future quantum information processing unit.

One of the unique features of our team concerns the large spectrum of our subjects going from the mathematical analysis of the physical systems (development of systematic mathematical methods for control and estimation of quantum systems), and the numerical analysis of the proposed solutions, to the experimental implementation of the quantum circuits based on these solutions. This is made possible by the constant and profound interaction between the applied mathematicians and the physicists in the group. Indeed, this close collaboration has already brought a significant acceleration in our research efforts. In a long run, this synergy should lead to a deeper understanding of the physical phenomena behind these emerging technologies and the development of new research directions within the field of quantum information processing.

Towards this ultimate task of practical quantum digital systems, the approach of the QUANTIC team is complementary to the one taken by teams with expertise in quantum algorithms. Indeed, we start from the specific controls that can be realistically applied on physical systems, to propose designs which combine them into hardware shortcuts implementing robust behaviors useful for quantum information processing. Whenever a significant new element of quantum engineering architecture is developed, the initial motivation is to prove an enabling technology with major impact for the groups working one abstraction layer higher: on quantum algorithms but also on e.g. secure communication and metrology applications.

3.1 Hardware-efficient quantum information processing

In this scientific program, we will explore various theoretical and experimental issues concerning protection and manipulation of quantum information. Indeed, the next, critical stage in the development of Quantum Information Processing (QIP) is most certainly the active quantum error correction (QEC). Through this stage one designs, possibly using many physical qubits, an encoded logical qubit which is protected against major decoherence channels and hence admits a significantly longer effective coherence time than a physical qubit. Reliable (fault-tolerant) computation with protected logical qubits usually comes at the expense of a significant overhead in the hardware (up to thousands of physical qubits per logical qubit). Each of the involved physical qubits still needs to satisfy the best achievable properties (coherence times, coupling strengths and tunability). More remarkably, one needs to avoid undesired interactions between various subsystems. This is going to be a major difficulty for qubits on a single chip.

The usual approach for the realization of QEC is to use many qubits to obtain a larger Hilbert space of the qubit register  109, 113. By redundantly encoding quantum information in this Hilbert space of larger dimension one make the QEC tractable: different error channels lead to distinguishable error syndromes. There are two major drawbacks in using multi-qubit registers. The first, fundamental, drawback is that with each added physical qubit, several new decoherence channels are added. Because of the exponential increase of the Hilbert's space dimension versus the linear increase in the number of decay channels, using enough qubits, one is able to eventually protect quantum information against decoherence. However, multiplying the number of possible errors, this requires measuring more error syndromes. Note furthermore that, in general, some of these new decoherence channels can lead to correlated action on many qubits and this needs to be taken into account with extra care: in particular, such kind of non-local error channels are problematic for surface codes. The second, more practical, drawback is that it is still extremely challenging to build a register of more than on the order of 10 qubits where each of the qubits is required to satisfy near the best achieved properties: these properties include the coherence time, the coupling strengths and the tunability. Indeed, building such a register is not merely only a fabrication task but rather, one requirers to look for architectures such that, each individual qubit can be addressed and controlled independently from the others. One is also required to make sure that all the noise channels are well-controlled and uncorrelated for the QEC to be effective.

We have recently introduced a new paradigm for encoding and protecting quantum information in a quantum harmonic oscillator (e.g. a high-Q mode of a 3D superconducting cavity) instead of a multi-qubit register  81. The infinite dimensional Hilbert space of such a system can be used to redundantly encode quantum information. The power of this idea lies in the fact that the dominant decoherence channel in a cavity is photon damping, and no more decay channels are added if we increase the number of photons we insert in the cavity. Hence, only a single error syndrome needs to be measured to identify if an error has occurred or not. Indeed, we are convinced that most early proposals on continuous variable QIP  78, 71 could be revisited taking into account the design flexibilities of Quantum Superconducting Circuits (QSC) and the new coupling regimes that are provided by these systems. In particular, we have illustrated that coupling a qubit to the cavity mode in the strong dispersive regime provides an important controllability over the Hilbert space of the cavity mode  80. Through a recent experimental work  120, we benefit from this controllability to prepare superpositions of quasi-orthogonal coherent states, also known as Schrödinger cat states.

In this Scheme, the logical qubit is encoded in a four-component Schrödinger cat state. Continuous quantum non-demolition (QND) monitoring of a single physical observable, consisting of photon number parity, enables then the tractability of single photon jumps. We obtain therefore a first-order quantum error correcting code using only a single high-Q cavity mode (for the storage of quantum information), a single qubit (providing the non-linearity needed for controllability) and a single low-Q cavity mode (for reading out the error syndrome). An earlier experiment on such QND photon-number parity measurements  114 has recently led to a first experimental realization of a full quantum error correcting code improving the coherence time of quantum information 7. As shown in Figure 1, this leads to a significant hardware economy for realization of a protected logical qubit. Our goal here is to push these ideas towards a reliable and hardware-efficient paradigm for universal quantum computation.

$$

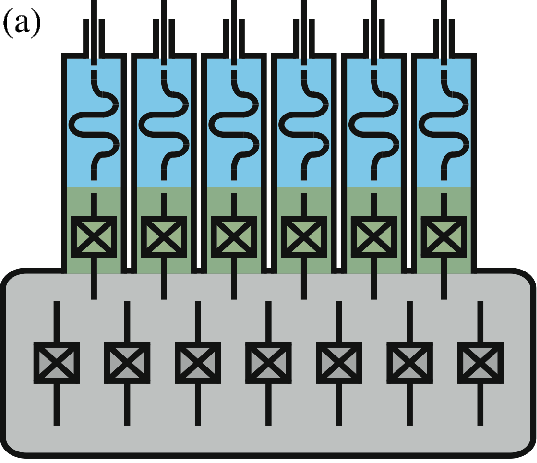
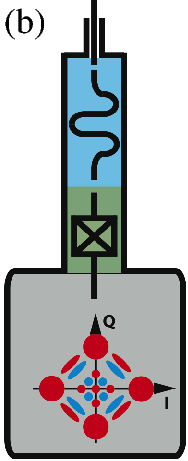
3.2 Reservoir (dissipation) engineering and autonomous stabilization of quantum systems

Being at the heart of any QEC protocol, the concept of feedback is central for the protection of quantum information, enabling many-qubit quantum computation or long-distance quantum communication. However, such a closed-loop control which requires a real-time and continuous measurement of the quantum system has been for long considered as counter-intuitive or even impossible. This thought was mainly caused by properties of quantum measurements: any measurement implies an instantaneous strong perturbation to the system's state. The concept of quantum non-demolition (QND) measurement has played a crucial role in understanding and resolving this difficulty   51. In the context of cavity quantum electro-dynamics (cavity QED) with Rydberg atoms  74, a first experiment on continuous QND measurements of the number of microwave photons was performed by the group at Laboratoire Kastler-Brossel (ENS)  72. Later on, this ability of performing continuous measurements allowed the same group to realize the first continuous quantum feedback protocol stabilizing highly non-classical states of the microwave field in the cavity, the so-called photon number states 9 (this ground-breaking work was mentioned in the Nobel prize attributed to Serge Haroche). The QUANTIC team contributed to the theoretical work behind this experiment  62, 40, 112, 42. These contributions include the development and optimization of the quantum filters taking into account the quantum measurement back-action and various measurement noises and uncertainties, the development of a feedback law based on control Lyapunov techniques, and the compensation of the feedback delay.

In the context of circuit quantum electrodynamics (circuit QED)  60, recent advances in quantum-limited amplifiers  102, 117 have opened doors to high-fidelity non-demolition measurements and real-time feedback for superconducting qubits  75. This ability to perform high-fidelity non-demolition measurements of a quantum signal has very recently led to quantum feedback experiments with quantum superconducting circuits  117, 101, 53. Here again, the QUANTIC team has participated to one of the first experiments in the field where the control objective is to track a dynamical trajectory of a single qubit rather than stabilizing a stationary state. Such quantum trajectory tracking could be further explored to achieve metrological goals such as the stabilization of the amplitude of a microwave drive  89.

While all this progress has led to a strong optimism about the possibility to perform active protection of quantum information against decoherence, the rather short dynamical time scales of these systems limit, to a great amount, the complexity of the feedback strategies that could be employed. Indeed, in such measurement-based feedback protocols, the time-consuming data acquisition and post-treatment of the output signal leads to an important latency in the feedback procedure.

The reservoir (dissipation) engineering  96 and the closely related coherent feedback  86 are considered as alternative approaches circumventing the necessity of a real-time data acquisition, signal processing and feedback calculations. In the context of quantum information, the decoherence, caused by the coupling of a system to uncontrolled external degrees of freedom, is generally considered as the main obstacle to synthesize quantum states and to observe quantum effects. Paradoxically, it is possible to intentionally engineer a particular coupling to a reservoir in the aim of maintaining the coherence of some particular quantum states. In a general viewpoint, these approaches could be understood in the following manner: by coupling the quantum system to be stabilized to a strongly dissipative ancillary quantum system, one evacuates the entropy of the main system through the dissipation of the ancillary one. By building the feedback loop into the Hamiltonian, this type of autonomous feedback obviates the need for a complicated external control loop to correct errors. On the experimental side, such autonomous feedback techniques have been used for qubit reset  70, single-qubit state stabilization  90, and the creation  45 and stabilization  79, 85, 108 of states of multipartite quantum systems.

Such reservoir engineering techniques could be widely revisited exploring the flexibility in the Hamiltonian design for QSC. We have recently developed theoretical proposals leading to extremely efficient, and simple to implement, stabilization schemes for systems consisting of a single, two or three qubits  70, 83, 58, 61. The experimental results based on these protocols have illustrated the efficiency of the approach  70, 108. Through these experiments, we exploit the strong dispersive interaction  106 between superconducting qubits and a single low-Q cavity mode playing the role of a dissipative reservoir. Applying continuous-wave (cw) microwave drives with well-chosen fixed frequencies, amplitudes, and phases, we engineer an effective interaction Hamiltonian which evacuates the entropy of the system interacting with a noisy environment: by driving the qubits and cavity with continuous-wave drives, we induce an autonomous feedback loop which corrects the state of the qubits every time it decays out of the desired target state. The schemes are robust against small variations of the control parameters (drives amplitudes and phase) and require only some basic calibration. Finally, by avoiding resonant interactions between the qubits and the low-Q cavity mode, the qubits remain protected against the Purcell effect, which would reduce the coherence times. We have also investigated both theoretically and experimentally the autonomous stabilization of non-classical states (such as Schrodinger cat states and Fock states) of microwave field confined in a high-Q cavity mode  104, 766, 4.

3.3 System theory for quantum information processing

In parallel and in strong interactions with the above experimental goals, we develop systematic mathematical methods for dynamical analysis, control and estimation of composite and open quantum systems. These systems are built with several quantum subsystems whose irreversible dynamics results from measurements and/or decoherence. A special attention is given to spin/spring systems made with qubits and harmonic oscillators. These developments are done in the spirit of our recent contributions  103, 40, 111, 105, 112, 428 resulting from collaborations with the cavity quantum electrodynamics group of Laboratoire Kastler Brossel.

3.4 Stabilization by measurement-based feedback

The protection of quantum information via efficient QEC is a combination of (i) tailored dynamics of a quantum system in order to protect an informational qubit from certain decoherence channels, and (ii) controlled reaction to measurements that efficiently detect and correct the dominating disturbances that are not rejected by the tailored quantum dynamics.

In such feedback scheme, the system and its measurement are quantum objects whereas the controller and the control input are classical. The stabilizing control law is based on the past values of the measurement outcomes. During our work on the LKB photon box, we have developed, for single input systems subject to quantum non-demolition measurement, a systematic stabilization method  42: it is based on a discrete-time formulation of the dynamics, on the construction of a strict control Lyapunov function and on an explicit compensation of the feedback-loop delay. Keeping the QND measurement assumptions, extensions of such stabilization schemes will be investigated in the following directions: finite set of values for the control input with application to the convergence analysis of the atomic feedback scheme experimentally tested in  121; multi-input case where the construction by inversion of a Metzler matrix of the strict Lyapunov function is not straightforward; continuous-time systems governed by diffusive master equations; stabilization towards a set of density operators included in a target subspace; adaptive measurement by feedback to accelerate the convergence towards a stationary state as experimentally tested in  93. Without the QND measurement assumptions, we will also address the stabilization of non-stationary states and trajectory tracking, with applications to systems similar to those considered in  75, 53.

3.5 Filtering, quantum state and parameter estimations

The performance of every feedback controller crucially depends on its online estimation of the current situation. This becomes even more important for quantum systems, where full state measurements are physically impossible. Therefore the ultimate performance of feedback correction depends on fast, efficient and optimally accurate state and parameter estimations.

A quantum filter takes into account imperfection and decoherence and provides the quantum state at time $t\ge 0$ from an initial value at $t=0$ and the measurement outcomes between 0 and $t$. Quantum filtering goes back to the work of Belavkin   47 and is related to quantum trajectories  54, 59. A modern and mathematical exposure of the diffusive models is given in  44. In  73 a first convergence analysis of diffusive filters is proposed. Nevertheless the convergence characterization and estimation of convergence rate remain open and difficult problems. For discrete time filters, a general stability result based on fidelity is proven in  103, 111. This stability result is extended to a large class of continuous-time filters in  41. Further efforts are required to characterize asymptotic and exponential stability. Estimations of convergence rates are available only for quantum non-demolition measurements  48. Parameter estimations based on measurement data of quantum trajectories can be formulated within such quantum filtering framework  65, 91.

We will continue to investigate stability and convergence of quantum filtering. We will also exploit our fidelity-based stability result to justify maximum likelihood estimation and to propose, for open quantum system, parameter estimation algorithms inspired of existing estimation algorithms for classical systems. We will also investigate a more specific quantum approach: it is noticed in  52 that post-selection statistics and “past quantum” state analysis  66 enhance sensitivity to parameters and could be interesting towards increasing the precision of an estimation.

3.6 Stabilization by interconnections

In such stabilization schemes, the controller is also a quantum object: it is coupled to the system of interest and is subject to decoherence and thus admits an irreversible evolution. These stabilization schemes are closely related to reservoir engineering and coherent feedback   96, 86. The closed-loop system is then a composite system built with the original system and its controller. In fact, and given our particular recent expertise in this domain 8108, 70, this subsection is dedicated to further developing such stabilization techniques, both experimentally and theoretically.

The main analysis issues are to prove the closed-loop convergence and to estimate the convergence rates. Since these systems are governed by Lindblad differential equations (continuous-time case) or Kraus maps (discrete-time case), their stability is automatically guaranteed: such dynamics are contractions for a large set of metrics (see  95). Convergence and asymptotic stability is less well understood. In particular most of the convergence results consider the case where the target steady-state is a density operator of maximum rank (see, e.g., 43[chapter 4, section 6]). When the goal steady-state is not full rank very few convergence results are available.

We will focus on this geometric situation where the goal steady-state is on the boundary of the cone of positive Hermitian operators of finite trace. A specific attention will be given to adapt standard tools (Lyapunov function, passivity, contraction and Lasalle's invariance principle) for infinite dimensional systems to spin/spring structures inspired of 8, 6108, 70 and their associated Fokker-Planck equations for the Wigner functions.

We will also explore the Heisenberg point of view in connection with recent results of the INRIA project-team MAXPLUS (algorithms and applications of algebras of max-plus type) relative to Perron-Frobenius theory  69, 68. We will start with  107 and  99 where, based on a theorem due to Birkhoff  49, dual Lindblad equations and dual Kraus maps governing the Heisenberg evolution of any operator are shown to be contractions on the cone of Hermitian operators equipped with Hilbert's projective metric. As the Heisenberg picture is characterized by convergence of all operators to a multiple of the identity, it might provide a mean to circumvent the rank issues. We hope that such contraction tools will be especially well adapted to analyzing quantum systems composed of multiple components, motivated by the facts that the same geometry describes the contraction of classical systems undergoing synchronizing interactions 116 and by our recent generalized extension of the latter synchronizing interactions to quantum systems 88.

Besides these analysis tasks, the major challenge in stabilization by interconnections is to provide systematic methods for the design, from typical building blocks, of control systems that stabilize a specific quantum goal (state, set of states, operation) when coupled to the target system. While constructions exist for so-called linear quantum systems 92, this does not cover the states that are more interesting for quantum applications. Various strategies have been proposed that concatenate iterative control steps for open-loop steering 119, 84 with experimental limitations. The characterization of Kraus maps to stabilize any types of states has also been established 50, but without considering experimental implementations. A viable stabilization by interaction has to combine the capabilities of these various approaches, and this is a missing piece that we want to address.

3.7 On-chip microwave engineering

The rapid development of circuitQED over the past 20 years was enabled by commercially available microwave components such as filters, switches and circulators, which allow experimentalists to shape and route measurement and control signals in and out of quantum systems. However, these components are intrinsically bulky, lossy and are imperfectly impedance-matched, leading to spurious reflections at their ports. In order to implement a full-scale quantum computer based on superconducting circuits, it is crucial that these functionalities be enabled reliably on-chip.

On-chip filters commonly used in circuitQED experiments are far from the level of variety and refinement of commercially available components. The near exclusive strategy known as "Purcell-filtering"  100 consists in placing $\lambda /4$ stubs  97 on all feed lines. This cancels the admittance of the environment seen by a superconducting qubit at its resonance frequency, inhibiting spontaneous relaxation. An issue with this strategy is that given the modest width of the stub stopband, performances are degraded as soon as the qubit is not perfectly in resonance. Moreover, this approach is not suited for multiplexed control and measurements, in which a single feed line addresses simultaneously several qubits. Notable alternatives include highpass waveguide filters only available in 3D circuitQED  98, and a recent implementation of a bandpass filter  64.

On-chip non-reciprocal elements, such as isolators, circulators and gyrators are at a very early stage of development. So far, the most promising approach to break reciprocity without resorting to strong magnetic fields—which are incompatible with superconducting circuit technology—relies on the differential phase impinged on a signal during parametric down-conversion with respect to the reverse process of up-conversion. Combining coherently several conversion paths with well-chosen phases, one obtains a constructive forward interference, and a destructive backward one. In circuitQED, frequency conversion is enabled by a non-linear Josephson circuit  110, 57, 39, or by electromechanical coupling to nanoresonators  46, 94. A serious drawback of this approach is that it relies on a destructive interference effect to obtain the reverse isolation, which limits the operational bandwidth: the highest value reported so far is a 23 dB isolation over a 8 MHz band  39. For completeness, we mention a recent implementation of a forward amplifier based on resistively shunted Josephson junctions  115 that reaches a 100 MHz bandwidth at the cost of added noise, and the long term prospect of harnessing the anomalous Hall effect to implement a gyrator  118, 87.

In this project, we propose to develop novel on-chip filters and isolators based on 1D photonic crystals, which could reach unprecedented bandwidth, tunable range and on/off or forward/backward transmission ratios. The central idea is that a microwave transmission line with periodically modulated electrical properties behaves as a robust stopband filter, with attenuation scaling exponentially with the line length.

By fabricating lines whose properties are modulated by design, we plan to demonstrate the efficiency of this novel type of stopband filters. These lines will be fabricated in a high-kinetic inductance material (such as chains of Josephson junctions or granular aluminium), we will overcome the main weakness of this approach, which is the large on-chip footprint required when fabricating with conventional superconductors. Extending the numerical simulation methods developed in thsi work, we plan to design other types of filters (bandpass, highpass, lowpass) based on a similar technology.

We will then change perspective and modulate a line properties parametrically instead of by design to implement non-reciprocal elements. The idea is to design a line that possesses two traveling mode 1 and 2, with different propagation phase-velocity $v_1\ll v_2$. If a low-frequency pump wave propagates on 1 modulates the electrical properties of the mode 2 thanks to shared non-linear inductance—for instance Josephson junction participating in both modes inductance—we obtain a situation in which mode 2 has spatially modulated electrical properties, with the phase of this modulation slowly evolving in time with at the pump period. A stopband appears in the transmission of a probe signal on mode 2, whose central frequency depends on whether the probe propagates in the same direction as the pump or not. It is then straightforward to turn this non-reciprocal filter into an isolator on circulator.

4.1 Quantum engineering

A new field of quantum systems engineering has emerged during the last few decades. This field englobes a wide range of applications including nano-electromechanical devices, nuclear magnetic resonance applications, quantum chemical synthesis, high resolution measurement devices and finally quantum information processing devices for implementing quantum computation and quantum communication. Recent theoretical and experimental achievements have shown that the quantum dynamics can be studied within the framework of estimation and control theory, but give rise to new models that have not been fully explored yet.

The QUANTIC team's activities are defined at the border between theoretical and experimental efforts of this emerging field with an emphasis on the applications in quantum information, computation and communication. The main objective of this interdisciplinary team is to develop quantum devices ensuring a robust processing of quantum information.

On the theory side, this is done by following a system theory approach: we develop estimation and control tools adapted to particular features of quantum systems. The most important features, requiring the development of new engineering methods, are related to the concept of measurement and feedback for composite quantum systems. The destructive and partial 1 nature of measurements for quantum systems lead to major difficulties in extending classical control theory tools. Indeed, design of appropriate measurement protocols and, in the sequel, the corresponding quantum filters estimating the state of the system from the partial measurement record, are themselves building blocks of the quantum system theory to be developed.

On the experimental side, we develop new quantum information processing devices based on quantum superconducting circuits. Indeed, by realizing superconducting circuits at low temperatures and using microwave measurement techniques, the macroscopic and collective degrees of freedom such as the voltage and the current are forced to behave according to the laws of quantum mechanics. Our quantum devices are aimed to protect and process quantum information through these integrated circuits.

6.1 Designing High-Fidelity Zeno Gates for Dissipative Cat Qubits

Participants: Ronan Gautier, Mazyar Mirrahimi, Alain Sarlette.

This paper 15, published in PRX Quantum, gathers several different system design possibilities in order to perform fast operations on cat qubits while strongly reducing the decoherence induced by the fast actuation on the system. The decoherence is due to the fact that a dissipative element is present on purpose in order to stabilize the qubit, while simple enough actuation Hamiltonians cannot drive all necessary system operations without activating this stabilizing dissipation; in this way, fast actuation would also induce rather fast information loss. One proposed solution, also submitted for patenting, is to introduce back-action from the output of the main dissipation channel, thus re-injecting the part of the evacuated entropy which correlates to qubit information which we want to maintain. Other proposed solutions include the engineering of small nonlinearities in the actuation Hamiltonian, or designing jump-activated operations. This has been a major part of the thesis of Ronan Gautier, successfully defended in December 2023.

6.2 Stabilization of approximate GHZ states with quasi-local couplings

Participants: Vincent Martin, Alain Sarlette.

This work 30, currently under review, comprises essentially 3 contributions. First, we solve the objective of stabilizing intrinsically long-range entanglement, for which impossibility results have been known, on a prototypical example. Second, our solution proposes and investigates the use of engineered reservoirs — thus an autonomous system with steady couplings — whose aim is to mimic the behavior of automata with little but nonzero memory. We establish the effectiveness of several options and workings, involving: introducing one auxiliary system per data qubit; adding a third level to the data qubit for operation purposes ; and inducing waves of effects propagating through the overall system at stochastic speeds. A third contribution concerns the analysis method, where we bound the Lindblad master equation evolution by a Markov chain involving hypothetical measurements of the dissipation channels, and then analyze the associated classical Markov chain.

6.3 Stability and decoherence rates of a GKP qubit protected by dissipation

Participants: Lev-Arcady Sellem, Rémi Robin, Philippe Campagne-Ibarcq, Pierre Rouchon.

In this communication at the IFAC WC 202320, We analyze an experimentally accessible Lindblad master equation for a quantum harmonic oscillator. It approximately stabilizes finite-energy periodic grid states called Gottesman-Kitaev-Preskill (GKP) states, that can be used to encode and protect a logical qubit. We give explicit upper bounds for the energy of the solutions of the Lindblad master equation. Using three periodic observables to define the Bloch sphere coordinates of a logical qubit, we show that their dynamics is governed by a diffusion partial differential equation on a 2D-torus with a Witten Laplacian. We show that the evolution of these logical coordinates is exponentially slow even in presence of small diffusive noise processes along the two quadratures of the phase space. Numerical simulations indicate similar results for other physically relevant noise processes.

6.4 Complete Positivity Violation in Higher-order Quantum Adiabatic Elimination

Participants: Masaaki Tokieda, Alain Sarlette, Pierre Rouchon.

This communication at the IFAC WC 2023 21 adresses quantum adiabatic elimination and complete-positivity. When a composite Lindblad system consists of weakly coupled sub-systems with fast and slow timescales, the description of slow dynamics can be simplified by discarding fast degrees of freedom. This model reduction technique is called adiabatic elimination. While second-order perturbative expansion with respect to the timescale separation has revealed that the evolution of a reduced state is completely positive, this paper presents an example exhibiting complete positivity violation in the fourth-order expansion. Despite the non-uniqueness of slow dynamics parametrization, we prove that complete positivity cannot be ensured in any parametrization. The violation stems from correlation in the initial state.

6.5 Correlation functions for realistic continuous quantum measurement

Participants: Pierre Guilmin, Pierre Rouchon, Antoine Tilloy.

This communication at the IFAC WC 2023 25 proposes a self-contained and accessible derivation of an exact formula for the n-point correlation functions of the signal measured when continuously observing a quantum system. The expression depends on the initial quantum state and on the Stochastic Master Equation (SME) governing the dynamics. This derivation applies to both jump and diffusive evolutions and takes into account common imperfections of realistic measurement devices. We show how these correlations can be efficiently computed numerically for commonly filtered and integrated signals available in practice.

6.6 Heisenberg formulation of adiabatic elimination for open quantum systems with two time-scales

Participants: François-Marie Le Regent, Pierre Rouchon.

This communication at the IEEE CDC 2023 19,considers an open quantum system governed by a Gorini, Kossakowski, Sudarshan, Lindblad (GKSL) master equation with two times-scales: a fast one, exponentially converging towards a linear subspace of quasi-equilibria; a slow one resulting from perturbations (small arbitrary decoherence and Hamiltonian dynamics). Usually adiabatic elimination is performed in the Schrödinger picture. We propose here an Heisenberg formulation where the invariant operators attached to the fast decay dynamics towards the quasi-equilibria subspace play a key role. Based on geometric singular perturbations, asympotic expansions of the Heisenberg slow dynamics and of the fast invariant linear subspaces are proposed. They exploit Carr's approximation lemma from center-manifold and bifurcation theory. Second-order expansions are detailed and shown to ensure preservation, up to second-order terms, of the trace and complete positivity for the slow dynamics on a slow time-scale. Such expansions can be exploited numerically.

6.7 Adiabatic elimination for composite open quantum systems: reduced model formulation and numerical simulations

Participants: François-Marie Le Régent, Pierre Rouchon.

This preprint under review in Physical Review A 32 proposes a numerical method for simulation of composite open quantum systems. It is based on Lindblad master equations and adiabatic elimination. Each subsystem is assumed to converge exponentially towards a stationary subspace, slightly impacted by some decoherence channels and weakly coupled to the other subsystems. This numerical method is based on a perturbation analysis with an asymptotic expansion. It exploits the formulation of the slow dynamics with reduced dimension. It relies on the invariant operators of the local and nominal dissipative dynamics attached to each subsystem. Second-order expansion can be computed only with local numerical calculations. It avoids computations on the tensor-product Hilbert space attached to the full system. This numerical method is particularly well suited for autonomous quantum error correction schemes. Simulations of such reduced models agree with complete full model simulations for typical gates acting on one and two cat-qubits (Z, ZZ and CNOT) when the mean photon number of each cat-qubit is less than 8. For larger mean photon numbers and gates with three cat-qubits (ZZZ and CCNOT), full model simulations are almost impossible whereas reduced model simulations remain accessible. In particular, they capture both the dominant phase-flip error-rate and the very small bit-flip error-rate with its exponential suppression versus the mean photon number.

6.8 Convergence of bipartite open quantum systems stabilized by reservoir engineering

Participants: Rémi Robin, Pierre Rouchon, Lev-Arcady Sellem.

This preprint 34 considers a generic family of Lindblad master equations modeling bipartite open quantum systems, where one tries to stabilize a quantum system by carefully designing its interaction with another, dissipative, quantum system - a strategy known as quantum reservoir engineering. We provide sufficient conditions for convergence of the considered Lindblad equations; our setting accommodates the case where steady states are not unique but rather supported on a given subspace of the underlying Hilbert space. We apply our result to a Lindblad master equation proposed for the stabilization of so-called cat qubits, a system that received considerable attention in recent years due to its potential applications in quantum information processing.

6.9 High-sensitivity AC-charge detection with a MHz-frequency fluxonium qubit

Participants: Angela Riva, Marius Villiers, Alain Sarlette, Zaki Leghtas.

This work 31, provisionally accepted in PRX, is the first step of results in the framework of the ANR project MecaFlux led by Samuel Deléglise, whose ultimate goal is to stabilize quantum states in a mechanical oscillator thanks to its coupling to a superconducting circuit in so-called "heavy fluxonium" configuration. The first step demonstrates the capabilities of such a fluxonium, in terms of actively cooling it down to the ground state, maintaining qubit-type quantum states, reading out such state. It also demonstrates experimentally how this circuit does couple to the degree of freedom (MHz charge fluctuations) where the mechanical oscillator is to be placed; thus we have a fully operational building block for the overall project, and which may be of independent interest.

6.10 Quantum control of a cat-qubit with bit-flip times exceeding ten seconds

Participants: Ulysse Reglade, Adrien Bocquet, Ronan Gautier, Lev-Arcady Sellem, Pierre Rouchon, Alain Sarlette, Mazyar Mirrahimi, Philippe Campagne-Ibarcq, Zaki Leghtas.

Binary classical information is routinely encoded in the two metastable states of a dynamical system. Since these states may exhibit macroscopic lifetimes, the encoded information inherits a strong protection against bit-flips. A recent qubit - the cat-qubit - is encoded in the manifold of metastable states of a quantum dynamical system, thereby acquiring bit-flip protection. An outstanding challenge is to gain quantum control over such a system without breaking its protection. If this challenge is met, significant shortcuts in hardware overhead are forecast for quantum computing. In this experiment, we implement a cat-qubit with bit-flip times exceeding ten seconds. This is a four order of magnitude improvement over previous cat-qubit implementations, and six orders of magnitude enhancement over the single photon lifetime that compose this dynamical qubit. This was achieved by introducing a quantum tomography protocol that does not break bit-flip protection. We prepare and image quantum superposition states, and measure phase-flip times above 490 nanoseconds. Most importantly, we control the phase of these superpositions while maintaining the bit-flip time above ten seconds. This work demonstrates quantum operations that preserve macroscopic bit-flip times, a necessary step to scale these dynamical qubits into fully protected hardware-efficient architectures.

This work has given rise to the preprint 33.

6.11 Observation of Josephson Harmonics in Tunnel Junctions

Participants: Zaki Leghtas.

Superconducting quantum processors have a long road ahead to reach fault-tolerant quantum computing. One of the most daunting challenges is taming the numerous microscopic degrees of freedom ubiquitous in solid-state devices. State-of-the-art technologies, including the world's largest quantum processors, employ aluminum oxide (AlOx) tunnel Josephson junctions (JJs) as sources of nonlinearity, assuming an idealized pure sinφ current-phase relation (CφR). However, this celebrated sinφ CφR is only expected to occur in the limit of vanishingly low-transparency channels in the AlOx barrier. Here we show that the standard CφR fails to accurately describe the energy spectra of transmon artificial atoms across various samples and laboratories. Instead, a mesoscopic model of tunneling through an inhomogeneous AlOx barrier predicts $\%$-level contributions from higher Josephson harmonics. By including these in the transmon Hamiltonian, we obtain orders of magnitude better agreement between the computed and measured energy spectra. The reality of Josephson harmonics transforms qubit design and prompts a reevaluation of models for quantum gates and readout, parametric amplification and mixing, Floquet qubits, protected Josephson qubits, etc. As an example, we show that engineered Josephson harmonics can reduce the charge dispersion and the associated errors in transmon qubits by an order of magnitude, while preserving anharmonicity.

This work has led to the preprint 38.

6.12 Robust suppression of noise propagation in Gottesman-Kitaev-Preskill error correction

Participants: Christian Siegele, Philippe Campagne Ibarcq.

The recently reported generation and error-correction of GKP qubits in trapped ions and superconducting circuits thus holds great promise for the future of quantum computing architectures based on such encoded qubits. However, these experiments rely on error-syndrome detection via an auxiliary physical qubit, whose noise may propagate and corrupt the encoded GKP qubit. In [Siegele, C., & Campagne-Ibarcq, P. (2023). Robust suppression of noise propagation in Gottesman-Kitaev-Preskill error correction. Physical Review A, 108(4), 042427.], we propose a simple module composed of two oscillators and a physical qubit, operated with two experimentally accessible quantum gates and elementary feedback controls to implement an error-corrected GKP qubit protected from such propagating errors. In the idealized setting of periodic GKP states, we develop efficient numerical methods to optimize our protocol parameters and show that errors of the encoded qubit stemming from flips of the physical qubit and diffusion of the oscillators state in phase-space may be exponentially suppressed as the noise strength over individual operations is decreased. Our approach circumvents the main roadblock towards fault-tolerant quantum computation with GKP qubits.

This work was published in Phys. Rev. A 18.

6.13 A GKP qubit protected by dissipation in a high-impedance superconducting circuit driven by a microwave frequency comb

Participants: Lev-Arcady Sellem, Alain Sarlette, Zaki Leghtas, Mazyar Mirrahimi, Pierre Rouchon, Philippe Campagne-Ibarcq.

In the preprint 35, we propose a novel approach to generate, protect and control GKP qubits. It employs a microwave frequency comb parametrically modulating a Josephson circuit to enforce a dissipative dynamics of a high impedance circuit mode, autonomously stabilizing the finite-energy GKP code. The encoded GKP qubit is robustly protected against all dominant decoherence channels plaguing superconducting circuits but quasi-particle poisoning. In particular, noise from ancillary modes leveraged for dissipation engineering does not propagate at the logical level. In a state-of-the-art experimental setup, we estimate that the encoded qubit lifetime could extend two orders of magnitude beyond the break-even point, with substantial margin for improvement through progress in fabrication and control electronics. Qubit initialization, readout and control via Clifford gates can be performed while maintaining the code stabilization, paving the way toward the assembly of GKP qubits in a fault-tolerant quantum computing architecture.

6.14 Robust sparse IQP sampling in constant depth

Participants: Louis Paletta, Alain Sarlette, Mazyar Mirrahimi.

Between NISQ (noisy intermediate scale quantum) approaches without any proof of robust quantum advantage and fully fault-tolerant quantum computation, we propose a scheme to achieve a provable superpolynomial quantum advantage (under some widely accepted complexity conjectures) that is robust to noise with minimal error correction requirements. We choose a class of sampling problems with commuting gates known as sparse IQP (Instantaneous Quantum Polynomial-time) circuits and we ensure its fault-tolerant implementation by introducing the tetrahelix code. This new code is obtained by merging several tetrahedral codes (3D color codes) and has the following properties: each sparse IQP gate admits a transversal implementation, and the depth of the logical circuit can be traded for its width. Combining those, we obtain a depth-1 implementation of any sparse IQP circuit up to the preparation of encoded states. This comes at the cost of a space overhead which is only polylogarithmic in the width of the original circuit. We furthermore show that the state preparation can also be performed in constant depth with a single step of feed-forward from classical computation. Our construction thus exhibits a robust superpolynomial quantum advantage for a sampling problem implemented on a constant depth circuit with a single round of measurement and feed-forward.

This work 23 has been accepted for a plenary presentation at the conference QIP 2024 in Taipei and is under revision for Quantum.

7.1 Bilateral contracts with industry

• New PhD contracts with Alice&Bob for 2 new students: Emilio Rui , Anissa Jacob .

8.1 International research visitors

8.2 European initiatives

8.3 National initiatives

• PEPR NISQ2LSQ: Quantic is a PI and the coordinator of WP1 of this PEPR project which started in 2022. The goal is to accelerate French research on the topic of bosonic and LDPC codes for preparing the ground for hardware-efficient and fault-tolerant quantum computation.
• PEPR RobustSuperQ: Quantic is a PI and the coordinator of WP1 of this PEPR project which started in 2022. The goal is to accelerate French research on the topic of high quality, noise resilient, superconducting qubits.
• ANR project HAMROQS: In the framework of the ANR program JCJC, Alain Sarlette has received a funding for his research program "High-accuracy model reduction for open quantum systems". This grant of 212k euros started on april 2019 and will run for 4 years.
• ANR project Mecaflux: Alain Sarlette is a PI of this ANR Grant that started in 2022 and runs for 4 years. This project aims to couple mechanical oscillators with superconduncting circuits at the quantum level, using a new circuit architecture allowing near-resonant coupling. The project is coordinated by mechanical oscillators expert Samuel Deléglise (LKB, U.Sorbonne), other project PIs are Alain Sarlette and Zaki Leghtas (QUANTIC project-team), Emmanuel Flurin and Hélène LeSueur (CEA Saclay). Our new recruit Antoine Tilloy may join with quantum gravity expertise if the level of control attains the objective where those effects become significant. The PhD thesis of Angela Riva is funded on this ANR.
• ANR project OCTAVES: Mazyar Mirrahimi is a PI of this ANR Grant that started in 2022 and will run for 4 years. This project aims in studying the measurement problem in circuit QED (non QND effects in presence of probe drives) as well as limitations to the parametric driving for cat qubit stabilization. The project is coordinated by Olivier Buisson (Institut Néel, Grenoble) and other project PIs are Benjamin Huard (ENS Lyon), Mazyar Mirrahimi (Quantic project-team), and Dima Shepelyansky (LPT, Toulouse). The PhD thesis of Linda Greggio is funded on this ANR.

8.4 Regional initiatives

• Alain Sarlette is a member of the steering committee of DIM Quantip.

9.1 Promoting scientific activities

9.2 Teaching - Supervision - Juries

9.3 Popularization

10.1 Major publications

• 1 articleS.Simon Apers and A.Alain Sarlette. Quantum Fast-Forwarding: Markov chains and graph property testing.Quantum Information & ComputationApril 2019HAL
• 2 articleP.Philippe Campagne-Ibarcq, A.Alec Eickbusch, S.Steven Touzard, E.Evan Zalys-Geller, N. E.Nicholas E. Frattini, V. V.Volodymyr V. Sivak, P.Philip Reinhold, S.Shruti Puri, S.Shyam Shankar, R. J.Robert J. Schoelkopf, L.Luigi Frunzio, M.Mazyar Mirrahimi and M. H.Michel H. Devoret. Quantum error correction of a qubit encoded in grid states of an oscillator.Nature584Text and figures edited for clarity. The claims of the paper remain the same. Author list fixedAugust 2020HAL
• 3 articleJ.Jérémie Guillaud and M.Mazyar Mirrahimi. Repetition Cat Qubits for Fault-Tolerant Quantum Computation.Physical Review Xhttps://arxiv.org/abs/1904.09474 - 22 pages, 11 figuresDecember 2019HALDOI
• 4 articleZ.Zaki Leghtas, S.Steven Touzard, I. M.Ioan M. Pop, A.Angela Kou, B.Brian Vlastakis, A.Andrei Petrenko, K. M.Katrina M. Sliwa, A.Anirudh Narla, S.Shyam Shankar, M. J.Michael J. Hatridge, M.Matthew Reagor, L.Luigi Frunzio, R. J.Robert J. Schoelkopf, M.Mazyar Mirrahimi and M. H.Michel H. Devoret. Confining the state of light to a quantum manifold by engineered two-photon loss.Science3476224February 2015, 853-857HALDOIback to text
• 5 articleR.Raphaël Lescanne, M.Marius Villiers, T.Théau Peronnin, A.Alain Sarlette, M.Matthieu Delbecq, B.Benjamin Huard, T.Takis Kontos, M.Mazyar Mirrahimi and Z.Zaki Leghtas. Exponential suppression of bit-flips in a qubit encoded in an oscillator.Nature PhysicsMarch 2020HALDOI
• 6 articleM.Mazyar Mirrahimi, Z.Zaki Leghtas, V. V.Victor V Albert, S.Steven Touzard, R. J.Robert J Schoelkopf, L.Liang Jiang and M. H.Michel H Devoret. Dynamically protected cat-qubits: a new paradigm for universal quantum computation.New Journal of Physics164apr 2014, 045014back to textback to text
• 7 articleN.N. Ofek, A.A. Petrenko, R.R. Heeres, P.P. Reinhold, Z.Z. Leghtas, B.B. Vlastakis, Y.Y. Liu, L.L. Frunzio, S.S.M. Girvin, L.L. Jiang, M.M. Mirrahimi, M. H.M. H. Devoret and R. J.R. J. Schoelkopf. Extending the lifetime of a quantum bit with error correction in superconducting circuits.Nature5362016, 5back to text
• 8 articleA.A. Sarlette, J.-M.J.-M. Raimond, M.M. Brune and P.P. Rouchon. Stabilization of nonclassical states of the radiation field in a cavity by reservoir engineering.Phys. Rev. Lett.1070104022011back to textback to textback to text
• 9 articleC.C. Sayrin, I.I. Dotsenko, X.X. Zhou, B.B. Peaudecerf, T.T. Rybarczyk, S.S. Gleyzes, P.P. Rouchon, M.M. Mirrahimi, H.H. Amini, M.M. Brune, J.-M.J.-M. Raimond and S.S. Haroche. Real-time quantum feedback prepares and stabilizes photon number states.Nature4772011, 73--77back to text
• 10 articleW. C.William C Smith, M.Marius Villiers, A.Antoine Marquet, J.Jose Palomo, M.Matthieu Delbecq, T.Takis Kontos, P.Philippe Campagne-Ibarcq, B.Benoît Douçot and Z.Zaki Leghtas. Magnifying quantum phase fluctuations with Cooper-pair pairing.Physical Review X122April 2022, 021002HALDOI

10.2 Publications of the year

10.3 Cited publications

• 39 articleB.Baleegh Abdo, O.Oblesh Jinka, N. T.Nicholas T Bronn, S.Salvatore Olivadese and M.Markus Brink. On-chip single-pump interferometric Josephson isolator for quantum measurements.arXiv preprint arXiv:2006.019182020back to textback to text
• 40 articleH.H. Amini, M.M. Mirrahimi and P.P. Rouchon. Stabilization of a delayed quantum system: the Photon Box case-study.IEEE Trans. Automatic Control5782012, 1918--1930back to textback to text
• 41 articleH.H Amini, C.C. Pellegrini and P.P. Rouchon. Stability of continuous-time quantum filters with measurement imperfections.Russian Journal of Mathematical Physics212014, 297--315back to text
• 42 articleH.H. Amini, A.A. Somaraju, I.I. Dotsenko, C.C. Sayrin, M.M. Mirrahimi and P.P. Rouchon. Feedback stabilization of discrete-time quantum systems subject to non-demolition measurements with imperfections and delays.Automatica4992013, 2683--2692back to textback to textback to text
• 43 bookS.S. AttalA.A. JoyeC.-A.C.-A. PilletOpen Quantum Systems III: Recent Developments.Springer, Lecture notes in Mathematics 18802006back to text
• 44 bookA.A. Barchielli and M.M. Gregoratti. Quantum Trajectories and Measurements in Continuous Time: the Diffusive Case.Springer Verlag2009back to text
• 45 articleJ.J.T. Barreiro, M.M. Muller, P.P. Schindler, D.D. Nigg, T.T. Monz, M.M. Chwalla, M.M. Hennrich, C.C.F. Roos, P.P. Zoller and R.R. Blatt. An open-system quantum simulator with trapped ions.Nature4704862011back to text
• 46 articleS.Shabir Barzanjeh, M.Matthias Wulf, M.Matilda Peruzzo, M.Mahmoud Kalaee, P.PB Dieterle, O.Oskar Painter and J. M.Johannes M Fink. Mechanical on-chip microwave circulator.Nature communications812017, 1--7back to text
• 47 articleV.V.P. Belavkin. Quantum stochastic calculus and quantum nonlinear filtering.Journal of Multivariate Analysis4221992, 171--201back to text
• 48 articleT.T. Benoist and C.C. Pellegrini. Large Time Behavior and Convergence Rate for Quantum Filters Under Standard Non Demolition Conditions.Communications in Mathematical Physics2014, 1-21URL: http://dx.doi.org/10.1007/s00220-014-2029-6back to text
• 49 articleG.G. Birkhoff. Extensions of Jentzch's theorem.Trans. Amer. Math. Soc.851957, 219--227back to text
• 50 articleS.S. Bolognani and F.F. Ticozzi. Engineering stable discrete-time quantum dynamics via a canonical QR decomposition.IEEE Trans. Autom. Control552010back to text
• 51 bookV.V. Braginski and F.F. Khalili. Quantum Measurements.Cambridge University Press1992back to text
• 52 articleP.P. Campagne-Ibarcq, L.L. Bretheau, E.E. Flurin, A.A. Auffèves, F.F. Mallet and B.B. Huard. Observing Interferences between Past and Future Quantum States in Resonance Fluorescence.Phys. Rev. Lett.11218040218May 2014, URL: http://link.aps.org/doi/10.1103/PhysRevLett.112.180402DOIback to text
• 53 articleP.P. Campagne-Ibarcq, E.E. Flurin, N.N. Roch, D.D. Darson, P.P. Morfin, M.M. Mirrahimi, M. H.M. H. Devoret, F.F. Mallet and B.B. Huard. Persistent Control of a Superconducting Qubit by Stroboscopic Measurement Feedback.Phys. Rev. X30210082013back to textback to text
• 54 bookH.H. Carmichael. An Open Systems Approach to Quantum Optics.Springer-Verlag1993back to text
• 55 bookH.H. Carmichael. Statistical Methods in Quantum Optics 2: Non-Classical Fields.Spinger2007back to text
• 56 bookJ.J. Carr. Application of Center Manifold Theory.Springer1981back to text
• 57 articleB. J.Benjamin J Chapman, E. I.Eric I Rosenthal, J.Joseph Kerckhoff, B. A.Bradley A Moores, L. R.Leila R Vale, J.JAB Mates, G. C.Gene C Hilton, K.Kevin Lalumiere, A.Alexandre Blais and K.KW Lehnert. Widely tunable on-chip microwave circulator for superconducting quantum circuits.Physical Review X742017, 041043back to text
• 58 articleJ.J. Cohen and M.M. Mirrahimi. Dissipation-induced continuous quantum error correction for superconducting circuits.Phys. Rev. A902014, 062344back to text
• 59 articleJ.J. Dalibard, Y.Y. Castin and K.K. Mölmer. Wave-function approach to dissipative processes in quantum optics.Phys. Rev. Lett.6851992, 580--583back to text
• 60 miscM. H.M. H. Devoret, A.A. Wallraff and J.J.M. Martinis. Superconducting Qubits: A Short Review.arXiv:cond-mat/04111742004back to text
• 61 articleN.Nicolas Didier, J.Jérémie Guillaud, S.Shyam Shankar and M.Mazyar Mirrahimi. Remote entanglement stabilization and concentration by quantum reservoir engineering.Physical Review Ahttps://arxiv.org/abs/1703.03379 - 5 pages, 4 figuresJuly 2018HALDOIback to text
• 62 articleI.I. Dotsenko, M.M. Mirrahimi, M.M. Brune, S.S. Haroche, J.-M.J.-M. Raimond and P.P. Rouchon. Quantum feedback by discrete quantum non-demolition measurements: towards on-demand generation of photon-number states.Physical Review A80: 013805-0138132009back to text
• 63 articleN.N. Fenichel. Geometric singular perturbation theory for ordinary differential equations.J. Diff. Equations311979, 53--98back to text
• 64 articleV. S.Vinicius S Ferreira, J.Jash Banker, A.Alp Sipahigil, M. H.Matthew H Matheny, A. J.Andrew J Keller, E.Eunjong Kim, M.Mohammad Mirhosseini and O.Oskar Painter. Collapse and Revival of an Artificial Atom Coupled to a Structured Photonic Reservoir.arXiv preprint arXiv:2001.032402020back to text
• 65 articleJ.J. Gambetta and H. M.H. M. Wiseman. State and dynamical parameter estimation for open quantum systems.Phys. Rev. A644042105September 2001, URL: http://link.aps.org/doi/10.1103/PhysRevA.64.042105back to text
• 66 articleS.S. Gammelmark, B.B. Julsgaard and K.K. Mölmer. Past Quantum States of a Monitored System.Phys. Rev. Lett.11116160401October 2013, URL: http://link.aps.org/doi/10.1103/PhysRevLett.111.160401back to text
• 67 bookC.C.W. Gardiner and P.P. Zoller. Quantum Noise.Springer2010back to text
• 68 articleS.S. Gaubert and Z.Z. Qu. Checking the strict positivity of Kraus maps is NP-hard.arXiv:1402.14292014back to text
• 69 articleS.S. Gaubert and Z.Z. Qu. The contraction rate in Thompson's part metric of order-preserving flows on a cone - Application to generalized Riccati equations.Journal of Differential Equations2568April 2014, 2902--2948URL: http://www.sciencedirect.com/science/article/pii/S0022039614000424back to text
• 70 articleK.K. Geerlings, Z.Z. Leghtas, I.I.M. Pop, S.S. Shankar, L.L. Frunzio, R.R.J. Schoelkopf, M.M. Mirrahimi and M. H.M. H. Devoret. Demonstrating a Driven Reset Protocol of a Superconducting Qubit.Phys. Rev. Lett.1101205012013back to textback to textback to textback to textback to textback to text
• 71 articleD.D. Gottesman, A.A. Kitaev and J.J. Preskill. Encoding a qubit in an oscillator.Phys. Rev. A640123102001back to text
• 72 articleC.C. Guerlin, J.J. Bernu, S.S. Deléglise, C.C. Sayrin, S.S. Gleyzes, S.S. Kuhr, M.M. Brune, J.-M.J.-M. Raimond and S.S. Haroche. Progressive field-state collapse and quantum non-demolition photon counting.Nature4482007, 889-893back to text
• 73 articleR.R. van Handel. The stability of quantum Markov filters.Infin. Dimens. Anal. Quantum Probab. Relat. Top.122009, 153--172back to text
• 74 bookS.S. Haroche and J.-M.J.-M. Raimond. Exploring the Quantum: Atoms, Cavities and Photons.Oxford University Press2006back to textback to text
• 75 articleM.M. Hatridge, S.S. Shankar, M.M. Mirrahimi, F.F. Schackert, K.K. Geerlings, T.T. Brecht, K.K.M. Sliwa, B.B. Abdo, L.L. Frunzio, S.S.M. Girvin, R.R.J. Schoelkopf and M. H.M. H. Devoret. Quantum back-action of an individual variable-strength measurement.Science3392013, 178--181back to textback to text
• 76 articleE.E.T. Holland, B.B. Vlastakis, R.R.W. Heeres, M. J.M. J. Reagor, U.U. Vool, Z.Z. Leghtas, L.L. Frunzio, G.G. Kirchmair, M. H.M. H. Devoret, M.M. Mirrahimi and R.R.J. Schoelkopf. Single-photon-resolved cross-Kerr interaction for autonomous stabilization of photon-number states.Phys. Rev. Lett.1152015, 180501back to text
• 77 bookT.T. Kato. Perturbation Theory for Linear Operators.Springer1966back to text
• 78 articleE.E. Knill, R.R. Laflamme and G.G.J. Milburn. A scheme for efficient quantum computation with linear optics.Nature409462001back to text
• 79 articleH.H. Krauter, C.C.A. Muschik, K.K. Jensen, W.W. Wasilewski, J.J.M. Petersen, J.J.I. Cirac and E.E.S. Polzik. Entanglement Generated by Dissipation and Steady State Entanglement of Two Macroscopic Objects.Phys. Rev. Lett.1070805032011back to text
• 80 articleZ.Z. Leghtas, G.G. Kirchmair, B.B. Vlastakis, M. H.M. H. Devoret, R. J.R. J. Schoelkopf and M.M. Mirrahimi. Deterministic protocol for mapping a qubit to coherent state superpositions in a cavity.Phys. Rev. A870423152013back to text
• 81 articleZ.Z. Leghtas, G.G. Kirchmair, B.B. Vlastakis, R. J.R. J Schoelkopf, M. H.M. H. Devoret and M.M. Mirrahimi. Hardware-efficient autonomous quantum memory protection.Phys. Rev. Lett.1111205012013back to text
• 82 articleZ.Z. Leghtas, A.A. Sarlette and P.P. Rouchon. Adiabatic passage and ensemble control of quantum systems.J. Phys. B441540172011back to text
• 83 articleZ.Z. Leghtas, U.U. Vool, S.S. Shankar, M.M. Hatridge, S.S.M. Girvin, M. H.M. H. Devoret and M.M. Mirrahimi. Stabilizing a Bell state of two superconducting qubits by dissipation engineering.Phys. Rev. A880238492013back to textback to text
• 84 articleJ.-S.Jr-Shin Li and N.N. Khaneja. Ensemble control of Bloch equations.IEEE Trans. Autom. Control542009, 528--536back to text
• 85 articleY.Y. Lin, J.J.P. Gaebler, F.F. Reiter, T.T.R. Tan, R.R. Bowler, A.A.S. Sorensen, D.D. Leibfried and D.D.J. Wineland. Dissipative production of a maximally entangled steady state of two quantum bits.Nature5042013, 415--418back to text
• 86 articleS.S. Lloyd. Coherent quantum feedback.Phys. Rev. A620221082000back to textback to text
• 87 articleA. C.Alice C Mahoney, J. I.James I Colless, L.Lucas Peeters, S. J.Sebastian J Pauka, E. J.Eli J Fox, X.Xufeng Kou, L.Lei Pan, K. L.Kang L Wang, D.David Goldhaber-Gordon and D. J.David J Reilly. Zero-field edge plasmons in a magnetic topological insulator.Nature communications812017, 1--7back to text
• 88 articleL.L. Mazzarella, A.A. Sarlette and F.F. Ticozzi. Consensus for quantum networks: from symmetry to gossip iterations.IEEE Trans. Automat. Controlin press2014back to text
• 89 conferenceM.M. Mirrahimi, B.B. Huard and M. H.M. H. Devoret. Strong measurement and quantum feedback for persistent Rabi oscillations in circuit QED experiments.IEEE Conference on Decision and ControlIEEE Conference on Decision and Control2012back to text
• 90 articleK.K.W. Murch, U.U. Vool, D.D. Zhou, S.S.J. Weber, S.S.M. Girvin and I.I. Siddiqi. Cavity-assisted quantum bath engineering.Phys. Rev. Lett.1091836022012back to text
• 91 articleA.A Negretti and K.K Mölmer. Estimation of classical parameters via continuous probing of complementary quantum observables.New Journal of Physics15121250022013, URL: http://stacks.iop.org/1367-2630/15/i=12/a=125002back to text
• 92 articleH.H.I. Nurdin, M.M.R. James and I.I.R. Petersen. Coherent quantum LQG control.Automatica452009, 1837--1846back to text
• 93 articleB.B. Peaudecerf, T.T. Rybarczyk, S.S. Gerlich, S.S. Gleyzes, J.-M.J.-M. Raimond, S.S. Haroche, I.I. Dotsenko and M.M. Brune. Adaptive Quantum Nondemolition Measurement of a Photon Number.Phys. Rev. Lett.1128080401Feb 2014, URL: http://link.aps.org/doi/10.1103/PhysRevLett.112.080401DOIback to text
• 94 articleG. A.Gabriel A Peterson, F.F Lecocq, K.Katarina Cicak, R. W.Raymond W Simmonds, J.J Aumentado and J. D.John D Teufel. Demonstration of efficient nonreciprocity in a microwave optomechanical circuit.Physical Review X732017, 031001back to text
• 95 articleD.D. Petz. Monotone Metrics on matrix spaces.Linear Algebra and its Applications2441996, 81--96back to text
• 96 articleJ.J.F. Poyatos, J.J.I. Cirac and P.P. Zoller. Quantum Reservoir Engineering with Laser Cooled Trapped Ions.Phys. Rev. Lett.77231996, 4728--4731back to textback to text
• 97 bookD. M.David M Pozar. Microwave engineering.John wiley & sons2011back to text
• 98 articleM.Matthew Reagor, W.Wolfgang Pfaff, C.Christopher Axline, R. W.Reinier W Heeres, N.Nissim Ofek, K.Katrina Sliwa, E.Eric Holland, C.Chen Wang, J.Jacob Blumoff, K.Kevin Chou and others. Quantum memory with millisecond coherence in circuit QED.Physical Review B9412016, 014506back to text
• 99 articleD.D. Reeb, M. ..M .J. Kastoryano and M. ..M .M. Wolf. Hilbert's projective metric in quantum information theory.Journal of Mathematical Physics528082201August 2011, URL: http://dx.doi.org/10.1063/1.3615729back to text
• 100 articleM. D.Matthew D Reed, B. R.Blake R Johnson, A. A.Andrew A Houck, L.Leonardo DiCarlo, J. M.Jerry M Chow, D. I.David I Schuster, L.Luigi Frunzio and R. J.Robert J Schoelkopf. Fast reset and suppressing spontaneous emission of a superconducting qubit.Applied Physics Letters96202010, 203110back to text
• 101 articleD.D. Ristè, J.J.V. Leeuwen, H.-S.H.-S. Ku, K.K. Lehnert and L.L. Dicarlo. Initialization by measurement of a superconducting quantum bit circuit.Phys. Rev. Lett.1090505072012back to text
• 102 articleN.N. Roch, E.E. Flurin, F.F. Nguyen, P.P. Morfin, P.P. Campagne-Ibarcq, M. H.M. H. Devoret and B.B. Huard. Widely tunable, non-degenerate three-wave mixing microwave device operating near the quantum limit.Phys. Rev. Lett.1081477012012back to text
• 103 articleP.P. Rouchon. Fidelity is a Sub-Martingale for Discrete-Time Quantum Filters.IEEE Transactions on Automatic Control56112011, 2743--2747back to textback to text
• 104 articleA.A. Roy, Z.Z. Leghtas, A.A.D. Stone, M.M.H. Devoret and M.M. Mirrahimi. Continuous generation and stabilization of mesoscopic field superposition states in a quantum circuit.Phys. Rev. A912015, 013810back to text
• 105 articleA.A. Sarlette, Z.Z. Leghtas, M.M. Brune, J.-M.J.-M. Raimond and P.P. Rouchon. Stabilization of nonclassical states of one- and two-mode radiation fields by reservoir engineering.Phys. Rev. A860121142012back to text
• 106 articleD.D.I. Schuster, A.A.A. Houck, J.J.A Schreier, A.A. Wallraff, J.J.M. Gambetta, A.A. Blais, L.L. Frunzio, J.J. Majer, B.B. Johnson, M. H.M. H. Devoret, S.S.M. Girvin and R. J.R. J. Schoelkopf. Resolving photon number states in a superconducting circuit.Nature4452007, 515--518back to text
• 107 inproceedingsR.R. Sepulchre, A.A. Sarlette and P.P. Rouchon. Consensus in non-commutative spaces.Decision and Control (CDC), 2010 49th IEEE Conference on2010, 6596--6601back to text
• 108 articleS.S. Shankar, M.M. Hatridge, Z.Z. Leghtas, K.K.M. Sliwa, A.A. Narla, U.U. Vool, S.S.M. Girvin, L.L. Frunzio, M.M. Mirrahimi and M. H.M. H. Devoret. Autonomously stabilized entanglement between two superconducting quantum bits.Nature5042013, 419--422back to textback to textback to textback to textback to text
• 109 articleP.P. Shor. Scheme for reducing decoherence in quantum memory.Phys. Rev. A521995, 2493--2496back to text
• 110 articleK.KM Sliwa, M.M Hatridge, A.A Narla, S.S Shankar, L.L Frunzio, R.RJ Schoelkopf and M.MH Devoret. Reconfigurable Josephson circulator/directional amplifier.Physical Review X542015, 041020back to text
• 111 inproceedingsA.A. Somaraju, I.I. Dotsenko, C.C. Sayrin and P.P. Rouchon. Design and Stability of Discrete-Time Quantum Filters with Measurement Imperfections.American Control Conference2012, 5084--5089back to textback to text
• 112 articleA.A. Somaraju, M.M. Mirrahimi and P.P. Rouchon. Approximate stabilization of infinite dimensional quantum stochastic system.Reviews in Mathematical Physics2513500012013back to textback to text
• 113 articleA.A. Steane. Error Correcting Codes in Quantum Theory.Phys. Rev. Lett7751996back to textback to text
• 114 articleL.L. Sun, A.A. Petrenko, Z.Z. Leghtas, B.B. Vlastakis, G.G. Kirchmair, K.K.M. Sliwa, A.A. Narla, M.M. Hatridge, S.S. Shankar, J.J. Blumoff, L.L. Frunzio, M.M. Mirrahimi, M. H.M. H. Devoret and R. J.R. J. Schoelkopf. Tracking photon jumps with repeated quantum non-demolition parity measurements.Nature5112014, 444--448back to text
• 115 articleT.T Thorbeck, S.S Zhu, E.E Leonard Jr, R.R Barends, J.J Kelly, J. M.John M Martinis and R.R McDermott. Reverse isolation and backaction of the SLUG microwave amplifier.Physical Review Applied852017, 054007back to text
• 116 articleJ.J.N. Tsitsiklis. Problems in decentralized decision making and computation.PhD Thesis, MIT1984back to text
• 117 articleR.R. Vijay, C.C. Macklin, D.D.H. Slichter, S.S.J. Weber, K.K.W. Murch, R.R. Naik, A.A.N. Korotkov and I.I. Siddiqi. Stabilizing Rabi oscillations in a superconducting qubit using quantum feedback.Nature4902012, 77--80back to textback to text
• 118 articleG.Giovanni Viola and D. P.David P DiVincenzo. Hall effect gyrators and circulators.Physical Review X422014, 021019back to text
• 119 articleL.L. Viola, E.E. Knill and S.S. Lloyd. Dynamical decoupling of open quantum system.Phys. Rev. Lett.821999, 2417-2421back to text
• 120 articleB.B. Vlastakis, G.G. Kirchmair, Z.Z. Leghtas, S.S.E. Nigg, L.L. Frunzio, S.S.M. Girvin, M.M. Mirrahimi, M. H.M. H. Devoret and R. J.R. J. Schoelkopf. Deterministically encoding quantum information using 100-photon Schrödinger cat states.Science3422013, 607--610back to text
• 121 articleX.X. Zhou, I.I. Dotsenko, B.B. Peaudecerf, T.T. Rybarczyk, C.C. Sayrin, S.S. Gleyzes, J.-M.J.-M. Raimond, M.M. Brune and S.S. Haroche. Field locked to Fock state by quantum feedback with single photon corrections.Physical Review Letter1082436022012back to text