2023Activity reportProject-TeamFACTAS
RNSR: 201822627W- Research center Inria Centre at Université Côte d'Azur
- Team name: Functional Analysis for ConcepTion and Assessment of Systems
- Domain:Applied Mathematics, Computation and Simulation
- Theme:Optimization and control of dynamic systems
Keywords
Computer Science and Digital Science
- A6.1.1. Continuous Modeling (PDE, ODE)
- A6.2.1. Numerical analysis of PDE and ODE
- A6.2.5. Numerical Linear Algebra
- A6.2.6. Optimization
- A6.3.1. Inverse problems
- A6.3.3. Data processing
- A6.3.4. Model reduction
- A6.4.4. Stability and Stabilization
- A6.4.5. Control of distributed parameter systems
- A6.5.4. Waves
- A8.2. Optimization
- A8.3. Geometry, Topology
- A8.4. Computer Algebra
Other Research Topics and Application Domains
- B1.2.3. Computational neurosciences
- B2.6.1. Brain imaging
- B3.1. Sustainable development
- B3.3. Geosciences
- B4.5. Energy consumption
1 Team members, visitors, external collaborators
Research Scientists
- Juliette Leblond [Team leader, INRIA, Senior Researcher, HDR]
- Laurent Baratchart [INRIA, Emeritus]
- Sylvain Chevillard [INRIA, Researcher]
- Martine Olivi [INRIA, Researcher, HDR]
- Dmitry Ponomarev [INRIA, ISFP]
Post-Doctoral Fellow
- Cristóbal Villalobos Guillén [INRIA, Post-Doctoral Fellow, until Nov 2023]
PhD Students
- Paul Asensio [INRIA, until Jul 2023]
- Masimba Nemaire [UNIV BORDEAUX, until Jan 2023, (he was paid by INRIA from Feb 2023 until April 2023)]
- Mubasharah Khalid Omer [UNIV COTE D'AZUR, from Oct 2023]
- Anass Yousfi [UNIV COTE D'AZUR]
Interns and Apprentices
- Mubasharah Khalid Omer [INRIA, Intern, from Apr 2023 until Sep 2023]
Administrative Assistant
- Florence Barbara [INRIA]
Visiting Scientist
- Bernard Hanzon [UCC CORK, from Sep 2023]
External Collaborators
- Vanna Lisa Coli [UNIV COTE D'AZUR]
- Jean-Paul Marmorat [CMA, Mines ParisTech]
- Fabien Seyfert [HighFSolutions, Nice]
2 Overall objectives
The team develops constructive, function-theoretic approaches to inverse problems arising in modeling and design, in particular for electro-magnetic systems as well as in the analysis of certain classes of signals.
Data typically consist of measurements or desired behaviors. The general thread is to approximate them by families of solutions to the equations governing the underlying system. This leads us to consider various interpolation and approximation problems in classes of rational and meromorphic functions, harmonic gradients, or solutions to more general elliptic partial differential equations (PDE), in connection with inverse potential problems. A recurring difficulty is to control the singularities of the approximants.
The mathematical tools pertain to complex and harmonic analysis, approximation theory, potential theory, system theory, differential topology, optimization and computer algebra. Targeted applications mostly concern non-destructive control from field measurements in medical engineering (source recovery in magneto/electro encephalography), paleomagnetism (determining the magnetization of rock samples), and since recently, obstacle identification (finding electrical characteristics of an object), for which an endeavor of the team is to develop algorithms resulting in dedicated software.
3 Research program
3.1 Introduction
Within the extensive field of inverse problems, much of the research by Factas deals with reconstructing solutions of classical elliptic PDEs from their boundary behavior. Perhaps the simplest example lies with harmonic identification of a stable linear dynamical system: the transfer-function
Practice is not nearly as simple, for
-
Step 1: To determine a complete model, that is, one which is defined at every frequency, in a sufficiently versatile function class (e.g., Hardy spaces). This ill-posed issue requires regularization, for instance by adding constraints on the behavior at non-measured frequencies.
-
Step 2: To compute a reduced order model. This typically consists of rational approximation of the complete model obtained in step 1, or phase-shift thereof to account for delays. We emphasize that deriving a complete model in step 1 is crucial to achieve stability of the reduced model in step 2.
Step 1 relates to extremal problems and analytic operator theory, see Section 3.3.1. Step 2 involves optimization, and some Schur analysis to parametrize transfer matrices of given Mc-Millan degree when dealing with systems having several inputs and outputs, see Section 3.3.2. It also makes contact with the topology of rational functions, in particular to count critical points and to derive bounds, see Section 3.3.2. Step 2 raises further issues in approximation theory regarding the rate of convergence and the extent to which singularities of the approximant (i.e., its poles) tend to singularities of the approximated function; this is where logarithmic potential theory becomes instrumental, see Section 3.3.3.
Applying a realization procedure to the result of step 2 yields an identification procedure from incomplete frequency data which was first demonstrated in 85 to tune resonant microwave filters. Harmonic identification of nonlinear systems around a stable equilibrium can also be envisaged by combining the previous steps with exact linearization techniques from 36.
The previous example of harmonic identification quickly suggests a generalization of itself. Indeed, on identifying
Inverse potential problems are severely indeterminate because infinitely many measures within an open set of
To recap, the gist of our approach is to approximate boundary data by (boundary traces of) fields arising from potentials of measures with specific support. This differs from standard approaches to inverse problems, where descent algorithms are applied to integration schemes of the direct problem; in such methods, it is the equation which gets approximated (in fact: discretized).
Along these lines, Factas advocates the use of steps 1 and 2 above, along with some singularity analysis, to approach issues of nondestructive control in 2-D and 3-D 1, 45, 49. The team is currently engaged in the generalization to inverse source problems for the Laplace equation in 3-D, to be described further in Section 3.2.1. There, holomorphic functions are replaced by harmonic gradients; applications are to inverse source problems in neurosciences (in particular in EEG/MEG) and inverse problems in geosciences.
The approximation-theoretic tools developed by Factas to handle issues mentioned so far are outlined in Section 3.3. In Section 3.2 to come, we describe in more detail which problems are considered and which applications are targeted.
We also began to investigate inverse scattering problems of plane waves by obstacles (playing here the role of a source term), with partners at LEAT. Such problems are again governed by Maxwell's equations and, in the time-harmonic regime, these reduce to Helmholtz equations depending on the frequency of the plane wave. Such issues have applications to detection and identification of metal objects, and this is part of LEAT research program, but at this early stage our study has remained academic (see Section 6.4).
3.2 Range of inverse problems
3.2.1 Elliptic partial differential equations (PDE)
Participants: Paul Asensio, Laurent Baratchart, Sylvain Chevillard, Mubasharah Khalid Omer, Juliette Leblond, Masimba Nemaire, Dmitry Ponomarev, Cristóbal Villalobos Guillén, Anass Yousfi.
By standard properties of conjugate differentials, reconstructing Dirichlet-Neumann boundary conditions for a function harmonic in a plane domain, when these conditions are already known on a subset
Such issues naturally arise in nondestructive testing of 2-D (or 3-D cylindrical) materials from partial electrical measurements on the boundary. For instance, the ratio between the tangential and the normal currents (the so-called Robin coefficient) tells one about corrosion of the material. Thus, solving Problem
Studying Hardy spaces of conjugate Beltrami equations is another interesting topic. For Sobolev-smooth coefficients of exponent greater than 2, they were investigated in 5, 37. The case of the critical exponent 2 is treated in 33, which apparently provides the first example of well-posed Dirichlet problem in the non-strictly elliptic case: the conductivity may be unbounded or zero on sets of zero capacity and, accordingly, solutions need not be locally bounded. More importantly perhaps, the exponent 2 is also the key to a corresponding theory on very general (still rectifiable) domains in the plane, as coefficients of pseudo-holomorphic functions obtained by conformal transformation onto a disk are merely of
Generalized Hardy classes as above are used in 34 where we address the uniqueness issue in the classical Robin inverse problem on a Lipschitz domain of
The 3-D version of step 1 in Section 3.1 is another subject investigated by Factas: to recover a harmonic function (up to an additive constant) in a ball or a half-space from partial knowledge of its gradient. This prototypical inverse problem (i.e., inverse to the Cauchy problem for the Laplace equation) often recurs in electromagnetism. At present, Factas is involved with solving instances of this inverse problem arising in two fields, namely medical imaging, e.g., for electroencephalography (EEG) or magneto-encephalography (MEG), and paleomagnetism (recovery of rocks magnetization) 1, 41, see Section 6.1. The question is considerably more difficult than its 2-D counterpart, due mainly to the lack of multiplicative structure for harmonic gradients. Still, substantial progress has been made over the last years using methods of harmonic analysis and operator theory.
The team is further concerned with 3-D generalizations and applications to non-destructive control of step 2 in Section 3.1. A typical problem is here to localize inhomogeneities or defaults such as cracks, sources or occlusions in a planar or 3-dimensional object, knowing thermal, electrical, or magnetic measurements on the boundary. These defaults can be expressed as a lack of harmonicity of the solution to the associated Dirichlet-Neumann problem, thereby posing an inverse potential problem in order to recover them. In 2-D, finding an optimal discretization of the potential in Sobolev norm amounts to solve a best rational approximation problem, and the question arises as to how the location of the singularities of the approximant (i.e., its poles) reflects the location of the singularities of the potential (i.e., the defaults we seek). This is a fairly deep issue in approximation theory, to which the project Apics (predecessor of Factas2) contributed convergence results for certain classes of fields (expressed as Cauchy integrals over extremal contours for the logarithmic potential 7, 42, 60). Initial schemes to locate cracks or sources via rational approximation on planar domains were obtained this way 45, 49, 61. It is remarkable that finite inverse source problems in 3-D balls, or more general algebraic surfaces, can be approached using these 2-D techniques upon slicing the domain into planar sections 9, 46. More precisely, each section cuts out a planar domain, the boundary of which carries data which can be proved to match an algebraic function. The singularities of this algebraic function are not located at the 3-D sources, but are related to them: the section contains a source if and only if some function of the singularities in that section meets a relative extremum. Using bisection it is thus possible to determine an extremal place along all sections parallel to a given plane direction, up to some threshold which has to be chosen small enough that one does not miss a source. This way, we reduce the original source problem in 3-D to a sequence of inverse poles and branch-points problems in 2-D. This bottom line generates a steady research activity within Factas, and again applications are sought to medical imaging and geosciences, see Sections 4.2, 4.3 and 6.1.
Conjectures may be raised on the behavior of optimal potential discretization in 3-D, but answering them is an ambitious program still in its infancy.
3.2.2 Systems, transfer and scattering
Participants: Laurent Baratchart, Sylvain Chevillard, Adam Cooman, Martine Olivi, Fabien Seyfert.
Through contacts with CNES (French space agency), members of the team became involved in identification and tuning of microwave electromagnetic filters used in space telecommunications.
The initial problem was to recover, from band-limited frequency measurements, physical parameters of the device under examination. The latter consists of interconnected dual-mode resonant cavities with negligible loss, hence its scattering matrix is modeled by a
This is where system theory comes into play, through the so-called realization process mapping a rational transfer function in the frequency domain to a state-space representation of the underlying system of linear differential equations in the time domain. Specifically, realizing the scattering matrix allows one to construct a virtual electrical network, equivalent to the filter, the parameters of which mediate in between the frequency response and the geometric characteristics of the cavities (i.e., the tuning parameters).
Hardy spaces provide a framework to transform this ill-posed issue into a series of regularized analytic and meromorphic approximation problems. More precisely, the procedure sketched in Section 3.1 goes as follows:
- infer from the pointwise boundary data in the bandwidth a stable transfer function (i.e., one which is holomorphic in the right half-plane), that may be infinite dimensional (numerically: of high degree). This is done by solving a problem analogous to
- A stable rational approximation of appropriate degree to the model obtained in the previous step is performed. For this, a descent method on the compact manifold of inner matrices of given size and degree is used, based on an original parametrization of stable transfer functions developed within the team 29, 12.
- Realizations of this rational approximant are computed. To be useful, they must satisfy certain constraints imposed by the geometry of the device. These constraints typically come from the coupling topology of the equivalent electrical network used to model the filter. This network is composed of resonators, coupled according to some specific graph. This realization step can be recast, under appropriate compatibility conditions 62, as solving a zero-dimensional multivariate polynomial system. To tackle this problem in practice, we use Gröbner basis techniques and continuation methods which team up in the Dedale-HF software (see Section 3.4.2).
Factas also investigates issues pertaining to design rather than identification. Given the topology of the filter, a basic problem in this connection is to find the optimal response subject to specifications that bear on rejection, transmission and group delay of the scattering parameters. Generalizing the classical approach based on Chebyshev polynomials for single band filters, we recast the problem of multi-band response synthesis as a generalization of the classical Zolotarev min-max problem for rational functions 28, 80. Thanks to quasi-convexity, the latter can be solved efficiently using iterative methods relying on linear programming. These were implemented in the software easy-FF. Currently, the team is engaged in the synthesis of more complex microwave devices like multiplexers and routers, which connect several filters through wave guides. Schur analysis plays an important role here, because scattering matrices of passive systems are of Schur type (i.e., contractive in the stability region). The theory originates with the work of I. Schur 84, who devised a recursive test to check for contractivity of a holomorphic function in the disk. The so-called Schur parameters of a function may be viewed as Taylor coefficients for the hyperbolic metric of the disk, and the fact that Schur functions are contractions for that metric lies at the root of Schur's test. Generalizations thereof turn out to be efficient to parametrize solutions to contractive interpolation problems 31. Dwelling on this, Factas contributed differential parametrizations (atlases of charts) of lossless matrix functions 29, 81, 72 which are fundamental to our rational approximation software RARL2 (see Section 3.4.5). Schur analysis is also instrumental to approach de-embedding issues, and provides one with considerable insight into the so-called matching problem. The latter consists in maximizing the power a multiport can pass to a given load, and for reasons of efficiency it is all-pervasive in microwave and electric network design, e.g., of antennas, multiplexers, wifi cards and more. It can be viewed as a rational approximation problem in the hyperbolic metric. Factas made significant contributions to this subject 6, in particular within the framework of the (defense funded) ANR Cocoram.
In recent years, our attention was driven by CNES and UPV (Bilbao) to questions about stability of high-frequency amplifiers. Contrary to previously discussed devices, these are active components. The response of an amplifier can be linearized around a set of primary current and voltages, and then admittances of the corresponding electrical network can be computed at various frequencies, using the so-called harmonic balance method. The initial goal is to check for stability of the linearized model, so as to ascertain existence of a well-defined working state. The network is composed of lumped electrical elements namely inductors, capacitors, negative and positive resistors, transmission lines, and controlled current sources. Our research so far has focused on describing the algebraic structure of admittance functions, so as to set up a function-theoretic framework where the two-steps approach outlined in Section 3.1 can be put to work. The main discovery is that the unstable part of each partial transfer function is rational and can be computed by analytic projection, see 10. We now start investigating the linearized harmonic transfer-function around a periodic cycle, to check for stability under non necessarily small inputs.
3.3 Approximation
Participants: Laurent Baratchart, Sylvain Chevillard, Juliette Leblond, Martine Olivi, Masimba Nemaire, Dmitry Ponomarev, Cristóbal Villalobos Guillén.
3.3.1 Best analytic approximation
In dimension 2, the prototypical problem to be solved in step 1 of Section 3.1 may be described as: given a domain
To find an analytic function
There,
To fix terminology, we refer to
(
In the case
Various modifications of
In view of our current research on stability of active devices via analyticity of the harmonic transfer function, on inverse magnetization issues, and on inverse scattering via identification of the frequency response, bounded extremal problems for analytic functions are receiving renewed interest by the team. In such issues, a function on an interval of the real line (or an arc of the circle) must be approximated by the trace of a function holomorphic in the half-plane (or the disk), that meets suitable size constraints.
The analog of Problem
Though originally considered in dimension 2, Problem
When
On the ball, the analog of Problem
When
Just like solving problem
Problem
Companion to problem
Note that
3.3.2 Best meromorphic and rational approximation
The techniques set forth in this section are used to solve step 2 in Section 3.2 and they are instrumental to approach inverse boundary value problems for the Poisson equation
Scalar meromorphic and rational approximation
We put
A natural generalization of problem
(
Only for
The case where
The Miaou project (predecessor of Apics) already designed a dedicated steepest-descent algorithm for the case
In order to establish global convergence results, the team has undertaken a deeper study of the number and nature of critical points (local minima, saddle points, ...), in which tools from differential topology and operator theory team up with classical interpolation theory 51, 54. Based on this work, uniqueness or asymptotic uniqueness of the approximant was proved for certain classes of functions like transfer functions of relaxation systems (i.e., Markov functions) 56 and more generally Cauchy integrals over hyperbolic geodesic arcs 57. These are the only results of this kind. Research on this topic remained dormant for a while by reasons of opportunity, but revisiting the work 32 in higher dimension is a worthy and timely endeavor today. Meanwhile, an analog to AAK theory was carried out for
A common feature to the above-mentioned problems is that critical point equations yield non-Hermitian orthogonality relations for the denominator of the approximant. This stresses connections with interpolation, which is a standard way to build approximants, and in many respects best or near-best rational approximation may be regarded as a clever manner to pick interpolation points. This was exploited in 58, 59, and is used in an essential manner to assess the behavior of poles of best approximants to functions with branched singularities, which is of particular interest for inverse source problems (cf. Sections 3.4.3 and 6.1).
In higher dimensions, the analog of Problem
Besides, certain constrained rational approximation problems, of special interest in identification and design of passive systems, arise when putting additional requirements on the approximant, for instance that it should be smaller than 1 in modulus (i.e., a Schur function). In particular, Schur interpolation lately received renewed attention from the team, in connection with matching problems. There, interpolation data are subject to a well-known compatibility condition (positive definiteness of the so-called Pick matrix), and the main difficulty is to put interpolation points on the boundary of
Matrix-valued rational approximation
Matrix-valued approximation is necessary to handle systems with several inputs and outputs but it generates additional difficulties as compared to scalar-valued approximation, both theoretically and algorithmically. In the matrix case, the McMillan degree (i.e., the degree of a minimal realization in the System-Theoretic sense) generalizes the usual notion of degree for rational functions. For instance when poles are simple, the McMillan degree is the sum of the ranks of the residues.
The basic problem that we consider now goes as follows: let
The scalar approximation algorithm derived in 35 and mentioned in Section 3.3.2 generalizes to the matrix-valued situation 69. The first difficulty here is to parametrize inner matrices (i.e., matrix-valued functions analytic in the unit disk and unitary on the unit circle) of given McMillan degree
Difficulties relative to multiple local minima of course arise in the matrix-valued case as well, and deriving criteria that guarantee uniqueness is even more difficult than in the scalar case. The case of rational functions of degree
Let us stress that RARL2 seems the only algorithm handling rational approximation in the matrix case that demonstrably converges to a local minimum while meeting stability constraints on the approximant. It is still a working pin of many developments by Factas on frequency optimization and design.
3.3.3 Behavior of poles of meromorphic approximants
Participants: Paul Asensio, Laurent Baratchart.
We refer here to the behavior of poles of best meromorphic approximants, in the
Generally speaking, in approximation theory, assessing the behavior of poles of rational approximants is essential to obtain error rates as the degree goes large, and to tackle constructive issues like uniqueness. However, as explained in Section 3.2.1, the original twist by Apics, now Factas, is to consider this issue also as a means to extract information on singularities of the solution to a Dirichlet-Neumann problem. The general theme is thus: how do the singularities of the approximant reflect those of the approximated function? This approach to inverse problem for the 2-D Laplacian turns out to be attractive when singularities are zero- or one-dimensional (see Section 4.2). It can be used as a computationally cheap initial condition for more precise but much heavier numerical optimizations which often do not even converge unless properly initialized. As regards crack detection or source recovery, this approach boils down to analyzing the behavior of best meromorphic approximants of given pole cardinality to a function with branch points, which is the prototype of a polar singular set. For piecewise analytic cracks, or in the case of sources, we were able to prove (7, 49, 42), that the poles of the approximants accumulate, when the degree goes large, to some extremal cut of minimum weighted logarithmic capacity connecting the singular points of the crack, or the sources 45. Moreover, the asymptotic density of the poles turns out to be the Green equilibrium distribution on this cut in
The case of two-dimensional singularities is still an outstanding open problem.
It is remarkable that inverse source problems inside a sphere or an ellipsoid in 3-D can be approached with such 2-D techniques, as applied to planar sections, see Section 6.1. The technique is implemented in the software FindSources3D, see Section 3.4.3.
Another, extremely classical technique to approximate –more accurately: extrapolate– a function given pointwise values is to compute a rational interpolant of minimal degree to match the values. This method, know as Padé (or multipoint Padé) approximation has been intensively studied for decades 30 but fails to produce pointwise convergence, even if the data are analytic. The best it can give in general is convergence in capacity, at least to functions whose singular set has capacity zero, and this does not prevent spurious poles of the approximant from wandering about the domain of analyticity of the approximated function 79. This phenomenon is standard in numerical practice, and gives rise in physics and engineering circles to a distinction between “mathematical” and “physical” poles; note that this distinction ignores the possibility that the function has other singularities than poles (for example branch-points or essential singularities). A modification of the multipoint Padé technique, where the degree is kept much smaller than the number of data and only approximate interpolation is performed in the least-square sense, has become especially popular over the last decade under the name vector fitting; this is in trend with the soaring development of computational methods in the frequency domain. Although their behavior looks similar to the one of multipoint Padé approximants from a numerical viewpoint, there seems to be no convergence result available for such approximate interpolants so far. Motivated by the behavior of numerical schemes developed at LEAT to recover resonance frequencies of conductors under electromagnetic inverse scattering (see section 4.5), we started investigating the behavior of such least-square rational approximants to functions with polar singular set, see section 6.4.
3.4 Software tools of the team
In addition to the above-mentioned research activities, Factas develops and maintains a number of long-term software tools that either implement and illustrate effectiveness of the algorithms theoretically developed by the team or serve as tools to help further research by team members. We present briefly the most important of them.
3.4.1 pisa
-
Name:
pisa
-
Keywords:
Electrical circuit, Stability
-
Functional Description:
To minimise prototyping costs, the design of analog circuits is performed using computer-aided design tools which simulate the circuit's response as accurately as possible.
Some commonly used simulation tools do not impose stability, which can result in costly errors when the prototype turns out to be unstable. A thorough stability analysis is therefore a very important step in circuit design. This is where pisa is used.
pisa is a Matlab toolbox that allows designers of analog electronic circuits to determine the stability of their circuits in the simulator. It analyses the impedance presented by a circuit to determine the circuit's stability. When an instability is detected, pisa can estimate location of the unstable poles to help designers fix their stability issue.
-
Release Contributions:
First version
- URL:
- Publications:
-
Authors:
Adam Cooman, David Martinez Martinez, Fabien Seyfert, Martine Olivi
-
Contact:
Fabien Seyfert
3.4.2 DEDALE-HF
-
Keyword:
Microwave filter
-
Scientific Description:
Dedale-HF consists in two parts: a database of coupling topologies as well as a dedicated predictor-corrector code. Roughly speaking each reference file of the database contains, for a given coupling topology, the complete solution to the coupling matrix synthesis problem associated to particular filtering characteristics. The latter is then used as a starting point for a predictor-corrector integration method that computes the solution to the coupling matrix synthesis problem corresponding to the user-specified filter characteristics. The reference files are computed off-line using Gröbner basis techniques or numerical techniques based on the exploration of a monodromy group. The use of such continuation techniques, combined with an efficient implementation of the integrator, drastically reduces the computational time.
Dedale-HF has been licensed to, and is currently used by TAS-Espana.
-
Functional Description:
Dedale-HF is a software dedicated to solve exhaustively the coupling matrix synthesis problem in reasonable time for the filtering community. Given a coupling topology, the coupling matrix synthesis problem consists in finding all possible electromagnetic coupling values between resonators that yield a realization of given filter characteristics. Solving the latter problem is crucial during the design step of a filter in order to derive its physical dimensions as well as during the tuning process where coupling values need to be extracted from frequency measurements.
- URL:
-
Contact:
Fabien Seyfert
-
Participant:
Fabien Seyfert
3.4.3 FindSources3D
-
Keywords:
Health, Neuroimaging, Visualization, Compilers, Medical, Image, Processing
-
Scientific Description:
Though synthetic data could be static, actual signal recordings are dynamical. The time dependency is either neglected and the data processed instant by instant, or separated from the space behavior using a singular value decomposition (SVD). This preliminary step allows to estimate the number of independent activities (uncorrelated sources) and to select the corresponding quantity of principal static components. After a first data transmission (“cortical mapping”) step of the static data, using the harmonicity property of the potential in the outermost layers (solving BEP problems on spherical harmonics bases), FS3D makes use of best rational approximation on families of 2-D planar cross-sections and of the software RARL2 in order to locate singularities and to determine the expected quantity of sources. From those planar singularities, the 3-D sources are finally estimated, together with their moment, in a last clustering step. Through this process, FS3D is able to recover time correlated sources, which is an important advantage. When simultaneously available, EEG and MEG data can now be processed together, and this also improves the recovery performance. In case of dynamical data, a recent additional step is to find the linear combination of the preliminary selected static components (change of basis) that produces source estimates which minimize the error w.r.t. data, an original criterion, which allows to improve the recovery quality.
-
Functional Description:
FindSources3D (FS3D) is a software program written in Matlab dedicated to the resolution of inverse source problems in brain imaging, electroencephalography (EEG) and magnetoencephalography (MEG). From data consisting in pointwise measurements of the electrical potential taken by electrodes on the scalp (EEG), or of a component of the magnetic field taken on a helmet (MEG), FS3D estimates pointwise dipolar current sources within the brain in a spherical layered model. Each layer (brain, skull, scalp) is assumed to have a constant conductivity.
- URL:
-
Contact:
Juliette Leblond
-
Participants:
Jean-Paul Marmorat, Juliette Leblond, Maureen Clerc, Nicolas Schnitzler, Théodore Papadopoulo
3.4.4 PRESTO-HF
-
Keywords:
CAO, Telecommunications, Microwave filter
-
Scientific Description:
For the matrix-valued rational approximation step, Presto-HF relies on RARL2. Constrained realizations are computed using the Dedale-HF software. As a toolbox, Presto-HF has a modular structure, which allows one for example to include some building blocks in an already existing software.
The delay compensation algorithm is based on the following assumption: far off the pass-band, one can reasonably expect a good approximation of the rational components of S11 and S22 by the first few terms of their Taylor expansion at infinity, a small degree polynomial in 1/s. Using this idea, a sequence of quadratic convex optimization problems are solved, in order to obtain appropriate compensations. In order to check the previous assumption, one has to measure the filter on a larger band, typically three times the pass band.
This toolbox has been licensed to (and is currently used by) Thales Alenia Space in Toulouse and Madrid, Thales airborne systems and Flextronics (two licenses). Xlim (University of Limoges) is a heavy user of Presto-HF among the academic filtering community and some free license agreements have been granted to the microwave department of the University of Erlangen (Germany) and the Royal Military College (Kingston, Canada).
-
Functional Description:
Presto-HF is a toolbox dedicated to low-pass parameter identification for microwave filters. In order to allow the industrial transfer of our methods, a Matlab-based toolbox has been developed, dedicated to the problem of identification of low-pass microwave filter parameters. It allows one to run the following algorithmic steps, either individually or in a single stroke:
- Determination of delay components caused by the access devices (automatic reference plane adjustment),
- Automatic determination of an analytic completion, bounded in modulus for each channel,
- Rational approximation of fixed McMillan degree,
- Determination of a constrained realization.
- URL:
-
Contact:
Fabien Seyfert
-
Participants:
Fabien Seyfert, Jean-Paul Marmorat, Martine Olivi
3.4.5 RARL2
-
Name:
Réalisation interne et Approximation Rationnelle L2
-
Keyword:
Approximation
-
Scientific Description:
The method is a steepest-descent algorithm. A parametrization of MIMO systems is used, which ensures that the stability constraint on the approximant is met. The implementation, in Matlab, is based on state-space representations.
RARL2 performs the rational approximation step in the software tools PRESTO-HF and FindSources3D. It is distributed under a particular license, allowing unlimited usage for academic research purposes. It was released to the universities of Delft and Maastricht (the Netherlands), Cork (Ireland), Brussels (Belgium), Macao (China) and BITS-Pilani Hyderabad Campus (India).
-
Functional Description:
RARL2 is a software for rational approximation. It computes a stable rational L2-approximation of specified order to a given L2-stable (L2 on the unit circle, analytic in the complement of the unit disk) matrix-valued function. This can be the transfer function of a multivariable discrete-time stable system. RARL2 takes as input either:
- its internal realization,
- its first N Fourier coefficients,
- discretized (uniformly distributed) values on the circle. In this case, a least-square criterion is used instead of the L2 norm.
It thus performs model reduction in the first or the second case, and leans on frequency data identification in the third. For band-limited frequency data, it could be necessary to infer the behavior of the system outside the bandwidth before performing rational approximation.
An appropriate Möbius transformation allows to use the software for continuous-time systems as well.
- URL:
-
Contact:
Martine Olivi
-
Participants:
Jean-Paul Marmorat, Martine Olivi
3.4.6 Sollya
-
Keywords:
Computer algebra system (CAS), Supremum norm, Proof synthesis, Code generator, Remez algorithm, Curve plotting, Numerical algorithm
-
Functional Description:
Sollya is an interactive tool where the developers of mathematical floating-point libraries (libm) can experiment before actually developing code. The environment is safe with respect to floating-point errors, i.e., the user precisely knows when rounding errors or approximation errors happen, and rigorous bounds are always provided for these errors.
Among other features, it offers a fast Remez algorithm for computing polynomial approximations of real functions and also an algorithm for finding good polynomial approximants with floating-point coefficients to any real function. As well, it provides algorithms for the certification of numerical codes, such as Taylor Models, interval arithmetic or certified supremum norms.
It is available as a free software under the CeCILL-C license.
- URL:
-
Contact:
Sylvain Chevillard
-
Participants:
Christoph Lauter, Jérôme Benoit, Marc Mezzarobba, Mioara Joldes, Nicolas Jourdan, Sylvain Chevillard
-
Partners:
CNRS, UPMC, ENS Lyon, LIP6, UCBL Lyon 1, Loria
4 Application domains
4.1 Introduction
Application domains are naturally linked to the problems described in Sections 3.2.1 and 3.2.2, under a common umbrella of function-theoretic techniques as described in Section 3.3.
4.2 Inverse source problems in EEG
Participants: Paul Asensio, Laurent Baratchart, Juliette Leblond, Jean-Paul Marmorat, Masimba Nemaire.
Solving over-determined Cauchy problems for the Laplace equation on a spherical layer (in 3-D) in order to extrapolate incomplete data (see Section 3.2.1) is a necessary ingredient of the team's approach to inverse source problems, in particular for applications to EEG, see 9. Indeed, the latter involves propagating the initial conditions through several layers of different conductivities, from the boundary shell down to the center of the domain where the singularities (i.e., the sources) lie. Once propagated to the innermost sphere, it turns out that traces of the boundary data on 2-D cross sections coincide with analytic functions with branched singularities in the slicing plane 7, 46. The singularities are related to the actual location of the sources, namely their moduli reach in turn a maximum when the plane contains one of the sources. Hence we are back to the 2-D framework of Section 3.3.3, and recovering these singularities can be performed via best rational approximation. The goal is to produce a fast and sufficiently accurate initial guess on the number and location of the sources in order to run heavier descent algorithms on the direct problem, which are more precise but computationally costly and often fail to converge if not properly initialized. Our belief is that such a localization process can add a geometric, valuable piece of information to the standard temporal analysis of EEG signal records.
In this connection, a dedicated software FindSources3D (FS3D, see Section 3.4.3) has been developed, in collaboration with the Inria team Athena (now Cronos) and the CMA - Mines ParisTech. Its Matlab version now incorporates the treatment of MEG data, the aim being to handle simultaneous EEG–MEG recordings available from our partners at INS, hospital la Timone, Marseille. Indeed, it is now possible to use simultaneously EEG and MEG measurement devices, in order to measure both the electrical potential and a component of the magnetic field (its normal component on the MEG helmet, that can be assumed to be spherical). Solving the inverse source problem from joint EEG and MEG data actually improves accuracy of the source estimation. Note that FS3D takes as inputs actual EEG and MEG measurements, like time signals, and performs a suitable singular value decomposition in order to separate independent sources.
It appears that, in the rational approximation step, multiple poles possess a nice behavior with respect to branched singularities. This is due to the very physical assumptions on the model from dipolar current sources: for EEG data that correspond to measurements of the electrical potential, one should consider triple poles; this will also be the case for MEG – magneto-encephalography – data. However, for (magnetic) field data produced by magnetic dipolar sources within rocks, one should consider poles of order five. Though numerically observed in 9, there is no mathematical justification so far why multiple poles generate such strong accumulation of the poles of the approximants. This intriguing property, however, is definitely helping source recovery and will be the topic of further study. It is used in order to automatically estimate the “most plausible” number of sources (numerically: up to 3, at the moment).
Furthermore, other approaches are being studied for EEG and other brain imaging modalities. They consist in regularizing the inverse source problem by a total variation constraint on the source term (a measure), added to the quadratic data approximation criterion (see Section 6.1.2) and presently focus on surface-distributed models. This is similar to the path that is taken for inverse magnetization problems (see Sections 4.3 and 6.1.1 ).
4.3 Inverse magnetization problems
Participants: Laurent Baratchart, Sylvain Chevillard, Mubasharah Khalid Omer, Juliette Leblond, Masimba Nemaire, Dmitry Ponomarev, Cristóbal Villalobos Guillén, Anass Yousfi.
Generally speaking, inverse potential problems, similar to the one appearing in Section 4.2, occur naturally in connection with systems governed by Maxwell's equation in the quasi-static approximation regime. In particular, they arise in magnetic reconstruction issues. A specific application is to geophysics, which led us to form the Inria Associate Team Impinge (Inverse Magnetization Problems IN GEosciences) together with MIT and Vanderbilt University that reached the end of its term in 2018.
To set up the context, recall that the Earth's geomagnetic field is generated by convection of the liquid metallic core (geodynamo) and that rocks become magnetized by the ambient field as they are formed or after subsequent alteration. Their remanent magnetization provides records of past variations of the geodynamo, which is used to study important processes in Earth sciences like motion of tectonic plates and geomagnetic reversals. Rocks from Mars, the Moon, and asteroids also contain remanent magnetization which indicates the past presence of core dynamos. Magnetization in meteorites may even record fields produced by the young sun and the protoplanetary disk which may have played a key role in solar system formation.
For a long time, paleomagnetic techniques were only capable of analyzing bulk samples and compute their net magnetic moment. The development of SQUID microscopes has recently extended the spatial resolution to sub-millimeter scales, raising new physical and algorithmic challenges. The associate team Impinge aimed at tackling them, experimenting with the SQUID microscope set up in the Paleomagnetism Laboratory of the department of Earth, Atmospheric and Planetary Sciences at MIT. Typically, pieces of rock are sanded down to a thin slab, and the magnetization has to be recovered from the field measured on a planar region at small distance from the slab.
Mathematically speaking, both inverse source problems for EEG from Section 4.2 and inverse magnetization problems described presently amount to recover the (3-D valued) quantity
outside the volume
Another timely instance of inverse magnetization problems lies with geomagnetism. Satellites orbiting around the Earth measure the magnetic field at many points, and nowadays it is a challenge to extract global information from those measurements. In collaboration with C. Gerhards (Geomathematics and Geoinformatics Group, Technische Universität Bergakademie Freiberg, Germany), we started to work on the problem of separating the magnetic field due to the magnetization of the globe's crust from the magnetic field due to convection in the liquid metallic core. The techniques involved are variants, in a spherical context, from those developed within the Impinge associate team for paleomagnetism, see Section 6.1.1.
4.4 Structure and stability of active devices
Participants: Laurent Baratchart, Sylvain Chevillard, Jean-Paul Marmorat, Martine Olivi, Fabien Seyfert.
Through contacts with CNES (Toulouse) and UPV (Bilbao), the team got involved in the design of amplifiers which, unlike filters, are active devices. A prominent issue here is stability. Twenty years ago, it was not possible to simulate unstable responses, and only after building a device could one detect instability. The advent of so-called harmonic balance techniques, which compute steady state responses of linear elements in the frequency domain and look for a periodic state in the time domain of a network connecting these linear elements via static non-linearities made it possible to compute the harmonic response of a (possibly nonlinear and unstable) device 87. This has had tremendous impact on design, and there is a growing demand for software analyzers. The team is also becoming active in this area.
In this connection, there are two types of stability involved. The first is stability of a fixed point around which the linearized transfer function accounts for small signal amplification. The second is stability of a limit cycle which is reached when the input signal is no longer small and truly nonlinear amplification is attained (e.g., because of saturation). Initial applications by the team have been concerned with the first type of stability, and emphasis was put on defining and extracting the “unstable part” of the response, see Section 6.2. Since then, the stability check for limit cycles has made important theoretical advances. Specifically, the exponential stability of the high frequency limit of a circuit was established last year in 4, implying that there are at most finitely many unstable poles and no other unstable singularity for the monodromy operator around the cycle. Furthermore, the links between the monodromy operator and the (operator-valued) harmonic transfer function of the linearized system along the trajectory were brought to light in 23. Numerical algorithms are now under investigation, while important pending issues involve: (i) whether poles of the harmonic transfer function must be poles of each entry thereof, at least generically and (ii) describing the stable spectrum of the harmonic transfer function of the linearized system, in particular understand its continuous and essential part.
4.5 Identification of resonating frequencies of compact metallic objects in electromagnetic inverse scattering
Participants: Paul Asensio, Laurent Baratchart, Juliette Leblond, Martine Olivi, Dmitry Ponomarev, Fabien Seyfert.
One of the best training grounds for function-theoretic applications by the team is the identification and design of physical systems whose performance is assessed frequency-wise. This is the case of electromagnetic resonant systems which are of common use in telecommunications.
In space telecommunications (satellite transmissions), constraints specific to on-board technology lead to the use of filters with resonant cavities in the microwave range. These filters serve multiplexing purposes (before or after amplification), and consist of a sequence of cylindrical hollow bodies, magnetically coupled by irises (orthogonal double slits). The electromagnetic wave that traverses the cavities satisfies the Maxwell equations, forcing the tangent electrical field along the body of the cavity to be zero. A deeper study of the Helmholtz equation states that an essentially discrete set of wave vectors is selected.
Study of resonances also led us to another inverse problem. There, the singularity expansion method featuring the above-mentioned discrete set of wave vectors is instrumental to the object detection. In this respect, we started an academic collaboration with LEAT (Univ. Côte d'Azur, France, J.-Y. Dauvignac, N. Fortino, Y. Zaki) on the topic of inverse scattering using frequency dependent measurements. As opposed to classical electromagnetic imaging where several spatially located sensors are used to identify the shape of an object by means of scattering data at a single frequency, a discrimination process between different metallic objects is here being sought for by means of a single, or a reduced number of sensors that operate on a whole frequency band. For short the spatial multiplicity and complexity of antenna sensors is here traded against a simpler architecture performing a frequency sweep.
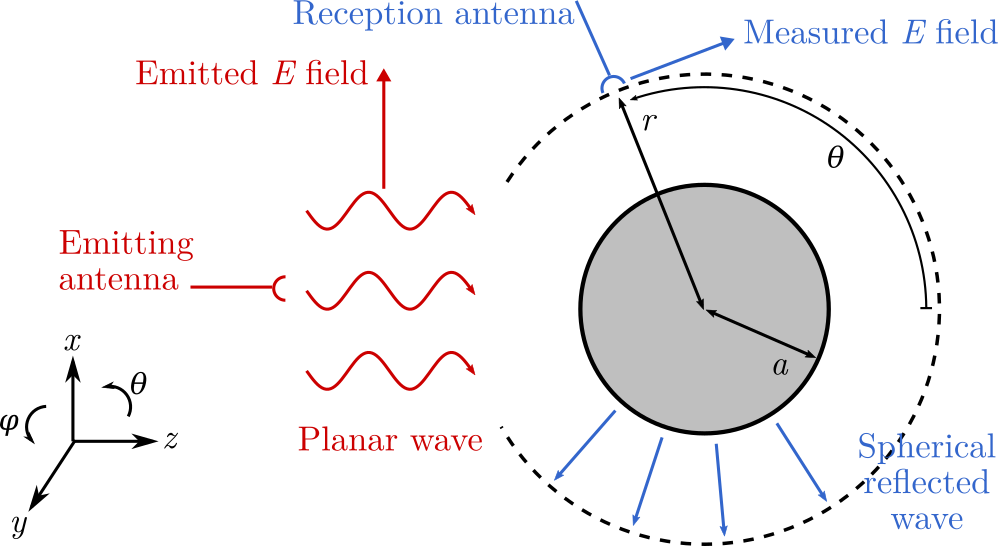
A schematic view of the problem: an antenna (horizontal and on the left) emits a planar wave (propagating horizontally, towards the right and shown in red) with an emitted electric field
The setting is shown on Figure 1. The total field
The subscripts
In order to gain some insight we started a full study of the particular case when the scatterer is a spherical PEC (Perfectly Electric Conductor). In this case Maxwell equations can be solved «explicitly» by means of expansions in series of vectorial spherical harmonics. We showed in particular that in this case
where
In order to perform the rational approximation of the function
where
where the coefficients
Numerical simulations showed that even though the creeping wave part is negligible in front of the optic part at high frequencies, it needs to be taken into account around the band of measured frequencies for the rational approximation.
Furthermore, the physical interpretation of these two terms leads to consider that the creeping wave part should carry more information about the scatterer and we want to investigate the conjecture that the poles of
We plan in the future to investigate a generalization of this form for other PEC scatterers. See Section 6.4.
5 Social and environmental responsibility
Participants: Sylvain Chevillard, Martine Olivi.
- M. Olivi is a member of the CLDD (Commission Locale de Développement Durable) and in charge of coordination. She is a member of the GDS EcoInfo (CNRS) and of Labos1point5.
- M. Olivi co-animated, with S. Quinton (Inria, SPADES), a workshop SEnS (Science, Environment, Society) at Toulon university.
- S. Chevillard and M. Olivi organized (together with L. Deneire, S. Icart and G. Urvoy-Keller) a one-day training program for PhD students (“Formation doctorale”) on the topic “Science, environment and society”.
6 New results
6.1 Inverse problems for Poisson-Laplace and Helmholtz equations
Participants: Paul Asensio, Laurent Baratchart, Sylvain Chevillard, Mubasharah Khalid Omer, Juliette Leblond, Jean-Paul Marmorat, Masimba Nemaire, Dmitry Ponomarev, Cristóbal Villalobos Guillén, Anass Yousfi.
6.1.1 Inverse magnetization issues for planar and volumetric samples
The goal is to invert magnetizations carried by a rock sample from measurements of the magnetic field nearby. A typical application is when the sample is shaped into thin slabs, with measurements taken by a superconducting quantum interference device (SQUID). Figure 2 sketches the corresponding experimental set up, brought up to our knowledge by collaborators from the Earth and Planetary Sciences Laboratory at MIT, in the case when the sample is modeled as a parallelepiped.
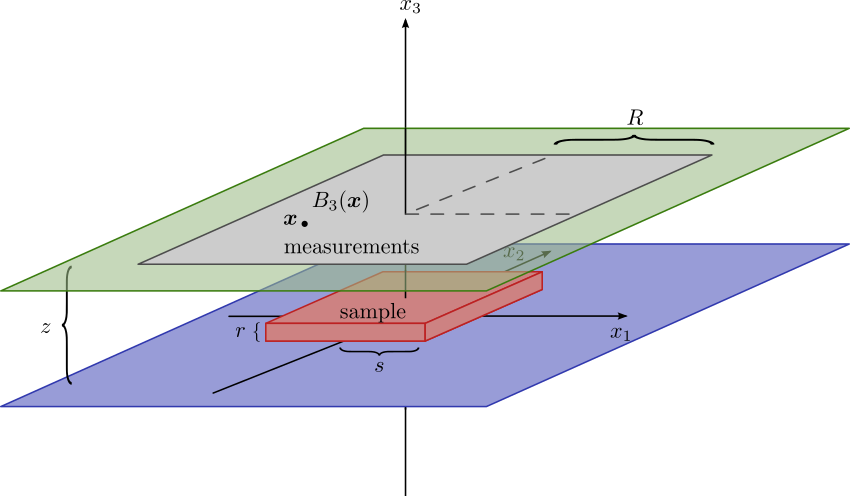
Schematic view of the experimental setup : the horizontal plane on which the rock sample lies (the sample being a parallelepiped with height
When the measurement area is a disk (or even a cylinder, if its thickness is not negligible), the issue of asymptotic estimates has undergone new developments. As part of A. Yousfi's thesis work, we derived a much more straightforward way of establishing the asymptotic estimates of the moments of
Instability of high-order asymptotic formulas for the net moment and the lack of the measurements naturally give rise to two aspects: noise filtering and field prolongation. One way to attempt both issues at once is to use the so-called spectral extrapolation. Such an approach consists in finding a suitable choice of basis functions which are adapted to problem structure and geometry, but at the same time are naturally defined outside of the original region. Using an eigenbasis of the suitable integral operator related to the forward problem, we attempt to construct a field extrapolant which would extend the available measurements in a way respecting the structure of the problem (such as harmonicity, support of the source, qualitative behavior at infinity). Restricting the number of terms in the expansion over this basis also provides a regularization strategy achieving a desired degree of the field denoising.
The work on the field extrapolation initiated at the end of 2022 has been advanced further. In particular, there were several directions taken to develop a different methodology.
First, after realizing that the previously developed spectral extrapolation procedure based on the forward operator featuring only one kernel function (the vertical derivative of the Poisson kernel) was somewhat naive, we have demonstrated that the extrapolation results could be improved by introducing several local extrapolation steps. Namely, by a procedure reminiscent of the chain method for local analytic continuation, we gradually increase the extrapolation area up to some intermediate size after which the previous naive procedure can be applied with a better outcome. The local stepping is computationally expensive and the details of its particular implementation should be investigated more thoroughly for its further improvement.
However, we have delved into developing another approach in a somewhat similar fashion. Namely, it was observed that the spectral extrapolation approach could be made exact if the basis functions are chosen as vector-eigenfunctions of a
A third approach for the field extrapolation is much more in the spirit of previous works of the team. Here, we use the techniques of constrained approximation in Hilbert spaces. Namely, given a tolerance level for measurement errors, we search for an extrapolant which agrees in a best possible manner with the original field data respecting the harmonicity of the field. Currently, we have done it only for the scalar potential simulated data (a toy problem for the internship work of M. Khalid Omer) rather than the vertical field component data (that would correspond to the physical SQUID setup at EAPS, MIT). The extension of this methodology to the latter case must be straightforward.
Finally, we have also started exploring a possibility of obtaining an extrapolant directly from solution of some integral equation. Even though, we have succeeded obtaining such an equation, no constructive results are yet available here. Any progress in this direction would allow dealing with volumetric magnetization sources.
Besides, we pursued a joint research effort with E. A. Lima and B. P. Weiss (EAPS dept. MIT, USA), C. S. Borlina (EAPS dept. Johns Hopkins Univ. USA) and D. Hardin (Vanderbilt Univ. USA), investigating the question whether is it possible to estimate the moment of an unknown magnetization by the one of a magnetization producing a field close enough to the observations? In other words, is full-inversion a demonstrably good (though admittedly costly) method to estimate the moment? We showed in 2022 that the answer is essentially no. More precisely, the field-to-moment map (that exists because silent magnetizations have zero moment) is discontinuous, even when magnetizations are endowed with a rather weak topology (like the weak-star topology on distributions of fixed order and given support), even if models for the magnetization are restricted to a very small class. In 2023, we started studying the speed of approximation of a given field by the field of a multipole when the degree of the latter goes large. This speed must be compared with the norm of the operator mapping the field (or the observed portion thereof) to the moment within the model class of multipoles, in order to decide if full inversion allows one to efficiently estimate the moment this way. This line of investigation is still in progress, and an article has been published that describes the method 15.
In collaboration with D. Hardin at Vanderbilt University (Nashville, USA), We also made theoretical progress describing the outcome of regularization techniques that penalize the total variation of an unknown measure modeling the magnetization, for inverse magnetization problems on compact samples of zero 3-D Lebesgue measure with connected complement. Namely, we proved that natural discretizations of the regularized criterion have a unique minimizer in this case, which allows us to establish the consistency of such schemes. Also when the sample is volumic (like a ball), we showed that these schemes select a solution on the boundary of the sample (which is the balayage of the “true” magnetization, whatever the latter is).
In another connection, the development of QDM sensors has heightened
the need for 3D-inversion of field maps. Indeed, while the QDM can be put very close to the surface of the sample which makes for improved resolution,
the thickness of the sample becomes appreciable compared to the
distance to the sensor and can no longer be neglected. In
his thesis 20, Masimba Nemaire has taken up the study of
silent magnetization of
6.1.2 Inverse problems in medical imaging
Our software FindSources3D (FS3D, see Sections 3.4.3, 4.2) dedicated to pointwise source estimation in EEG–MEG is now used by some of our collaborators. Together with M. Darbas (LAGA, Univ. Sorbonne Paris Nord) and P.-H. Tournier (labo. JLL, Sorbonne Univ), we considered the EEG inverse problem with a variable conductivity in the intermediate skull layer, in order to model hard / spongy bones, especially for neonates. Coupled with FS3D, the related transmission step is performed using a mixed variational regularization and finite elements (FreeFem++) on tetrahedral meshes, and furnishes very promising results 14.
We studied the uniqueness of a critical point of the quadratic criterion in the electroencephalography (EEG) problem for a single dipole situation (PhD of P. Asensio 19, see also 22). This issue is essential for the use of descent algorithms. This leads to the study of the following criterion:
where
where
We considered a different class of source models, not necessarily dipolar, and related estimation algorithms. Such models may be supported on the surface of the cortex or in the volume of the encephalon. We represent sources by vector-valued measures, and in order to favor sparsity in this infinite-dimensional setting we use a TV (i.e., total variation) regularization term as in Section 6.1.1. The approach follows that of 8 and is implemented through two different algorithms, whose convergence properties were studied in the PhD thesis of M. Nemaire 20. Tests on synthetic data provided good quality results, though they are quite numerically costly to obtain.

Three nested ovoids illustrating the three layers of the head (denoted
Progresses were made on the inverse problem of “Stereo” EEG (SEEG), where the potential is measured by deep electrodes and sensors within the brain as in the scheme of Figure 3. Assuming that the
current source term
The associated forward and inverse problems were solved for both an infinite medium conductor and a more realistic single model of the brain
The numerical implementation was done by approximating the density
The inverse problem for SEEG is ill-posed and a Tychonov regularization is used in order to solve the problem: find a distribution
We now consider
We are now able to handle MEG, EEG, SEEG modalities, simultaneously or not. The simultaneous handling of the different modalities is made the more straightforward by coupling the source localization problem and the inverse transmission problem.
The data transmission needs to be considered in the source localization problem so that the problem remains faithful to the data (electric or magnetic) when realistic head geometries are used in the computations. This also allows one to exploit properties of the problem that bring more regularity to the solutions. These considerations warrant that the transmission problem be solved simultaneously with the source localization problem, but in this connection the vector spaces used to model the sources and electrical properties need to be handled with care. The mathematical formulation allows for the source to be a distribution whereas the electric potential is always a function. This leads to the consideration that the optimal methods to recover the source and electric potential may not be the same. We proposed an alternating minimization procedure to solve the problem. We managed to show that this procedure converges to the desired solution and converges to the Tychonov functional linearly.
For more general source terms (vector valued measures,
6.2 Stability assessment of microwave circuits
Participants: Laurent Baratchart, Sylvain Chevillard, Fabien Seyfert, Adam Cooman.
This year we continued the study, initiated in 23 in collaboration with J.-B. Pomet from the McTao team (Inria Sophia Antipolis), of the harmonic transfer function (HTF) of a linear difference delay control system having finitely many (possibly non-commensurable) delays and periodic coefficients (having the same period). That is, an input-output system of the form:
where
6.3 Hardy-Hodge decomposition with applications to silent sources
Participants: Laurent Baratchart, Juliette Leblond, Masimba Nemaire, Cristóbal Villalobos Guillén.
In a joint work with T. Qian and P. Dang from the university of Macao
53,
we proved that on a compact hypersurface
Incented by these works, we developed a version of the Hardy-Hodge decomposition
for the Helmholtz equation, in collaboration with
H. Haddar from the team IDEFIX (Inria Saclay & ENSTA-Paris Tech), 25.
Our motivation to study such issues stems from a linearized model for an acoustic
scattering problem with
anisotropic layer, in the limit as the thickness of the layer goes to 0.
From the mathematical point of view, the problem generalizes
the one of recovering magnetizations mentioned above.
Here, we work with sources
where
Like in the Poisson case, it can be shown that
We also pursued our work on a direct characterization of silent
When
6.4 Identification of resonating frequencies of compact metallic objects in electromagnetic inverse scattering
Participants: Paul Asensio, Laurent Baratchart, Juliette Leblond, Martine Olivi, Fabien Seyfert, Dmitry Ponomarev.
Some fundamental aspects of the temporal behavior of the solution of the time-domain wave equation (in spatial dimensions 1, 2 and 3) have been studied in a recent work 21 (previously a preprint, now completed and submitted for journal publication) in connection with designing an efficient time-domain solver for the Helmholtz equation in high-frequency regime and with smooth coefficients. In physical 3D settings, the rate of the stabilization of the solution is linked to the location and the strength of the resonances (poles of meromorphic continuation of the resolvent operator). Since the information on the resonances is useful for the inverse problems (object identification), some of the already developed numerical methods could be employed for their efficient computation. Those methods would often rely on the complex-scaling (PML) approach to the open-domain problems 75 and have yet to be compared with the present rational-approximation viewpoint adopted by the team.
Another connection between time-domain methods for elliptic PDE problems and inverse problems is the so-called controllability approach which has recently received a renewed interest 65. In such an approach, one seeks to accelerate temporal convergence to the stationary solution of the time-domain wave equation with a periodic source term by minimizing the difference of the initial solution and the solution after one time-period. Once properly set up, this would yield the solution to the original Helmholtz equation without large computational costs of simulating the time-domain problem for long times. Potential extension of such methods could be a possibility for future joint work with Atlantis team.
Related to our collaboration with LEAT, the rational approximation of the transfer function
where
This year we were able to obtain an analog of the Nuttal-Pommerenke theorem on the converge in capacity, not for problem (3) as we seek in the framework of our collaboration with LEAT, but for a least square analog of Padé approximation that goes as follows:
where
6.5 Other elliptic PDE problems: from direct to inverse problems
Participants: Juliette Leblond, Dmitry Ponomarev.
Some direct problems have been considered from an analytical viewpoint that could shed light on their inverse counterparts.
The work 18 (previously a preprint, now a published work) deals with the extension of a classical model of contact problem with sliding punch in presence of material wear. The semi-explicit form of the obtained results and the generality of the model call for looking into relevant inverse problems. These could include, for example, determining an optimal initial shape of the punch profile that would minimize the worn volume.
In the joint work 17 with E. Pozzi (Saint Louis Univ., Missouri, USA), we present an operator theoretical approach of some inverse problems. It gives an overview of the solution to linear forward and inverse problems for a class of elliptic PDE in two-dimensional domains, in the framework of Banach spaces and operators. We focused on the conductivity equation, with smooth enough conductivity (see Section 3.2.1), and with Dirichlet or Neumann or mixed boundary conditions. For domains with Dini-smooth boundary, the approach is based on the properties of generalized Hardy spaces.
6.6 Spectra of Toeplitz operators
Participants: Juliette Leblond, Masimba Nemaire.
When solving fixed point problems using iterative methods, the rate of convergence is determined by the spectral radius of the operator and how quickly the iterative methods settle to the convergence rates is determined by the growth of the resolvent of the operator. It is therefore of interest to study the spectra of operators, here we studied the spectra of Toeplitz operators, together with S. Kupin (IMB, Univ. Bordeaux) and L. Golinskii (B. Verkin Institute for Low Temperature Physics and Engineering, Kharkiv, Ukraine).
Let
6.7 Tools for numerically guaranteed computations
Participants: Sylvain Chevillard.
The overall and long-term goal is to enhance the quality of numerical computations.
This year, we worked on improving the implementation of the
We did a full error analysis of the proposed implementation, including an exhaustive search on some selected sub-intervals to find the actual worst cases for the range reduction, the evaluation error and the combined error. We also proposed new values for the coefficients of the polynomial, in order to make it practically optimal among all polynomials in term of the maximal total error (i.e., the error combining the range reduction error, the approximation error and the evaluation error). This work was done in collaboration with G. Melquiond (Toccata, Inria) and P. Zimmermann (Caramba, Inria). S. Chevillard presented it at the “Rencontres nationales du groupe de travail Arith du GDR IM” (RAIM 2023) and a report is currently being written on the subject.
7 Partnerships and cooperations
7.1 International research visitors
7.1.1 Visits of international scientists
Other international visits to the team
Maxim Yattselev
-
Status:
Associate Professor
-
Institution of origin:
Indiana University-Purdue University Indianapolis
-
Country:
Indiana, USA
-
Dates:
June 2023
-
Context of the visit:
This is a long term research effort to describe the behavior of poles and zeros of asymptotically optimal meromorphic approximant to functions with singular set of capacity zero.
-
Mobility program/type of mobility:
Research stay
Bernhard Hanzon
-
Status:
Professor
-
Institution of origin:
University College Cork
-
Country:
Ireland
-
Dates:
September to December 2023
-
Context of the visit:
Writing a book on former joint research with members of the team
-
Mobility program/type of mobility:
Research stay, lecture
7.2 European initiatives
7.2.1 Other european programs/initiatives
Participants: Paul Asensio, Laurent Baratchart, Masimba Nemaire, Martine Olivi, Cristóbal Villalobos Guillén.
Factas is part of the European Research Network on System Identification (ERNSI) since 1992. System identification deals with the derivation, estimation and validation of mathematical models of dynamical phenomena from experimental data.
7.3 National initiatives
Participants: Paul Asensio, Laurent Baratchart, Sylvain Chevillard, Juliette Leblond, Masimba Nemaire, Martine Olivi, Dmitry Ponomarev, Cristóbal Villalobos Guillén, Anass Yousfi.
ANR REPKA
ANR-18-CE40-0035, “REProducing Kernels in Analysis and beyond” (2019–2023).
Led by Aix-Marseille Univ. (IMM), involving Factas team, together with Bordeaux (IMB), Paris-Est, Toulouse Universities.
The project consists of several interrelated tasks dealing with topical problems in modern complex analysis, operator theory and their important applications to other fields of mathematics including approximation theory, probability, and control theory. The project is centered around the notion of the so-called reproducing kernel of a Hilbert space of holomorphic functions. Reproducing kernels are very powerful objects playing an important role in numerous domains such as determinantal point processes, signal theory, Sturm-Liouville and Schrödinger equations.
This project supported the PhD of M. Nemaire within Factas, co-advised by IMB partners.
GDR AFHP
GDR “Analyse Fonctionnelle, Harmonique et Probabilités”.
Led by Gustave Eiffel Univ. (LAMA), involving Factas team, together with several universities.
The GDR is concerned with five main axes: linear dynamics, Banach spaces and their operators, holomorphic dynamics, harmonic analysis, analysis and probability, and with the interactions between them.
8 Dissemination
Participants: Laurent Baratchart, Sylvain Chevillard, Juliette Leblond, Martine Olivi, Dmitry Ponomarev.
8.1 Promoting scientific activities
8.1.1 Scientific events: organisation
General chair, scientific chair
- J. Leblond was a member of the scientific committee of the GDR Analyse Fonctionnelle, Harmonique et Probabilités (AFHP) days, October 2023, Porquerolles. She was also within the scientific committee of the 1st international conference on artificIAl Intelligence and applied MAthematics for History and Archaeology (IAMAHA), November 2023, Nice.
Member of the organizing committees
- F. Barbara, S. Chevillard, and J. Leblond were members of the organizing committee of the GDR AFHP days, October 2023, Porquerolles.
Member of the conference program committees
L. Baratchart is a member of the program committee of the conference Inverse problems: modeling and simulation (IPMS). “
8.1.2 Journal
Member of the editorial boards
L. Baratchart is a member of the editorial board of the journals Computational Methods and Function Theory (CMFT) and Complex Analysis and Operator Theory (CAOT).
Reviewer - reviewing activities
- S. Chevillard was a reviewer for Reliable Computing.
- J. Leblond was a reviewer for Arima.
- M. Olivi was a reviewer for Enseigner les enjeux socio-écologiques dans le supérieur.
- D. Ponomarev was a reviewer for Complex Analysis and Operator Theory (CAOT) and Mathematical Reviews.
8.1.3 Invited talks
- L. Baratchart was an invited speaker at the workshop “Orthogonal polynomials and special functions” of the Foundations of Computational Mathematics (FoCM) conference, June 19-21, 2023, Paris.
- L. Baratchart and D. Ponomarev were invited speakers at the GDR AFHP days, October 24-27, 2023, Porquerolles.
- D. Ponomarev was an invited speaker at Journée Analyse Harmonique et EDP, 27 March 2023, Bordeaux, and at the minisymposium “Inverse Problems in Imaging and Tomographic Issues, Theory and Practice” of SIAM GS23 conference, June 19-22, Bergen, Norway.
8.1.4 Contributed talks
- S. Chevillard gave a presentation at the “Rencontres nationales du groupe de travail Arith du GDR IM” (RAIM 2023), November 2023, Nancy.
- M. Nemaire gave a communication at the workshop Mathematics of electrical imaging: modeling, theory and implementation, June 2023, Toulouse.
- D. Ponomarev gave presentations (poster and oral, respectively) at the conference IEEE AIM 2023 Advances in Magnetics (January 15-18, 2023, Moena, Italy) and at the workshop Complex Analysis: Techniques, Applications and Computations - Perspectives 2023 (July 24-28, 2023, Cambridge, UK).
- C. Villalobos Guillén gave presentations at the Journées du GDR AFHP, October 2023, Porquerolles, and at the Winter-School Sound and Fury of Modeling, November 2023, Arpino, Italy. She also presented a poster at the Conference: 30 ans de mathématiques pour l'imagerie optique, September 2023, Marseille.
8.1.5 Research administration
- J. Leblond is a member of the “Conseil Scientifique” and of the “Commission Administrative Paritaire” of Inria.
- M. Olivi is a member of the CLDD (Commission Locale de Développement Durable) and in charge of coordination.
- During the period March-November 2023, the team members were actively involved in an experimental project led by the Direction of the Research Center for the design of new Inria office spaces. This included participation in a series of workshops “Ateliers de co-conception de l'Espace UX” (S. Chevillard, D. Ponomarev), complemented by discussions within the “Comité de pilotage” (D. Ponomarev) and general meetings with the other concerned teams.
8.2 Teaching - Supervision - Juries
8.2.1 Teaching
- S. Chevillard gives “Colles” (oral examination preparing undergraduate students for the competitive examination to enter French Engineering Schools) at Centre International de Valbonne (CIV) (2 hours per week). In 2023, he also gave a course on Carbon Footprints at Polytech Sophia Antipolis, as a participant of the course Environmental Issues addressed to all students of Polytech, whatever their pathway (level L3; the course on Carbon Footprints was 2 hours long and represented a fifth of the whole course; it was repeated 4 times, due to the year group being divided into four sub-groups).
- S. Chevillard and M. Olivi gave a one-day Doctoral Workshop on Science, Environment and Society (in collaboration with G. Urvoy-Keller, S. Icart and L. Deneire, I3S). The first half-day was inspired by the workshop SEnS, created by S. Quinton (Inria, SPADES) and E. Tannier (Inria, BEAGLE). The second half-day was more specifically dedicated to the environmental crisis and the study of prospective scenarios.
8.2.2 Supervision
- PhD: P. Asensio, Inverse source estimation problems in EEG and MEG, defended September 2023, advisers: L. Baratchart, J. Leblond.
- PhD: M. Nemaire, Inverse potential problems with application to quasi-static electromagnetics, defended March 2023, advisers: L. Baratchart, J. Leblond, S. Kupin (IMB, Univ. Bordeaux).
- PhD in progress: A. Yousfi, Methods to estimate the net magnetic moment of rocks, since October 2022, advisers: S. Chevillard, J. Leblond.
- PhD in progress: M. Khalid Omer, Field preprocessing and treatment of complex samples in the paleomagnetic context, since October 2023, advisers: J. Leblond, D. Ponomarev.
- Post-doc.: C. Villalobos Guillén, Inverse source problems for magnetization, primary current and inverse scattering, adviser: L. Baratchart.
- Internship: M. Khalid Omer, Field preprocessing in inverse magnetisation problems, April-August 2023, advisers: J. Leblond, D. Ponomarev.
8.2.3 Juries
- J. Leblond was a member of the examining committees for the defense of the PhD theses of B. Laville (Univ. Côte d'Azur, ED STIC, September) and of N. Nasr (Univ. Bordeaux, ED MI, December).
- M. Olivi was a member of the “Comité de sélection MCF61” of Centrale Lyon EEA/Ampère.
8.3 Popularization
8.3.1 Internal or external Inria responsibilities
J. Leblond and M. Olivi are members of Terra Numerica.
8.3.2 Interventions
- M. Olivi participated in a meeting with scholars at Collège la Chênaie, in the context of “la cordée de la réussite” : Un parcours scientifique sans préjugés : brisons le plafond de verre !. She gave four conferences “CHICHE” at Lycée Simone Veil in Valbonne. She gave a the c@fé-in of the research center, Martine à la COP : au delà des slogans, quelles solutions ?, and a general audience conference at Terra Numerica on the same subject.
- D. Ponomarev gave a presentation at C@fé ADSTIC, 30 November 2023, Sophia Antipolis.
9 Scientific production
9.1 Major publications
- 1 articleBounded extremal and Cauchy-Laplace problems on the sphere and shell.J. Fourier Anal. Appl.162Published online Nov. 20092010, 177--203URL: http://dx.doi.org/10.1007/s00041-009-9110-0back to textback to textback to text
- 2 articleMagnetic moment estimation and bounded extremal problems.Inverse Problems and Imaging 131February 2019, 29HALDOI
-
3
articleMinimax principle and lower bounds in
- 4 articleSufficient Stability Conditions for Time-varying Networks of Telegrapher's Equations or Difference Delay Equations.SIAM Journal on Mathematical Analysis532021, 1831–1856HALDOIback to text
- 5 articleHardy spaces of the conjugate Beltrami equation.Journal of Functional Analysis25922010, 384-427URL: http://dx.doi.org/10.1016/j.jfa.2010.04.004back to textback to text
- 6 articleBoundary Nevanlinna-Pick interpolation with prescribed peak points. Application to impedance matching.SIAM Journal on Mathematical Analysis2017HALDOIback to text
- 7 articleWeighted Extremal Domains and Best Rational Approximation.Advances in Mathematics2292012, 357-407URL: http://hal.inria.fr/hal-00665834back to textback to textback to text
- 8 articleInverse Potential Problems for Divergence of Measures with Total Variation Regularization.Foundations of Computational Mathematics202020, 1273-1307HALDOIback to text
- 9 articleSource localization using rational approximation on plane sections.Inverse Problems285May 2012, Article number 055018 - 24 pagesHALDOIback to textback to textback to text
- 10 articleModel-Free Closed-Loop Stability Analysis: A Linear Functional Approach.IEEE Transactions on Microwave Theory and Techniqueshttps://arxiv.org/abs/1610.032352017HALback to text
- 11 inbookOn some extremal problems for analytic functions with constraints on real or imaginary parts.Advances in Complex Analysis and Operator Theory: Festschrift in Honor of Daniel Alpay's 60th BirthdayBirkhauser2017, 219-236HALDOIback to text
- 12 articleIdentification of microwave filters by analytic and rational H2 approximation.Automatica492January 2013, 317-325HALDOIback to textback to text
9.2 Publications of the year
International journals
- 13 articleA layer potential approach to inverse problems in brain imaging.Journal of Inverse and Ill-posed ProblemsFebruary 2023HALDOIback to text
- 14 articleNumerical resolution of the inverse source problem for EEG using the quasi-reversibility method.Inverse Problems39112023, 115003HALDOIback to text
- 15 articleEstimating the Net Magnetic Moment of Geological Samples From Planar Field Maps Using Multipoles.Geochemistry, Geophysics, Geosystems247July 2023, 38HALDOIback to text
Scientific book chapters
- 16 inbookOn discrete spectra of Bergman-Toeplitz operators with harmonic symbols.291From Complex Analysis to Operator Theory: A PanoramaIn Memory of Sergey NabokoOperator Theory: Advances and ApplicationsSpringer International Publishing2023HALDOIback to text
- 17 inbookAn operator theoretical approach of some inverse problems.Function Spaces, Theory and ApplicationsFields Institute Communications ; vol 87Springer2023HALDOIback to text
- 18 inbookGeneralised Model of Wear in Contact Problems: The Case of Oscillatory Load.Integral Methods in Science and Engineering: Analytic and Computational ProceduresBirkhäuserNovember 2023HALDOIback to text
Doctoral dissertations and habilitation theses
- 19 thesisInverse problems of source localization with applications to EEG and MEG.Université cote d'azurSeptember 2023HALback to textback to text
- 20 thesisInverse potential problems, with applications to quasi-static electromagnetics.Université de BordeauxMarch 2023HALback to textback to text
Reports & preprints
- 21 miscOn the limiting amplitude principle for the wave equation with variable coefficients.December 2023HALback to text
- 22 miscCritical points for least-squares estimation of dipolar sources in inverse problems for Poisson equation.January 2023HALback to text
- 23 miscExponential stability of linear periodic difference-delay equations.2023HALback to textback to text
- 24 miscIntegral representation formula for linear non-autonomous difference-delay equations.2023HALback to text
- 25 miscSilent sources on a surface for the Helmholtz equation and decomposition of L² vector fields.December 2023HALback to text
-
26
miscSilent sources in
- 27 miscMagnetisation Moment of a Bounded 3D Sample: Asymptotic Recovery from Planar Measurements on a Large Disk Using Fourier Analysis.September 2023HALback to text
9.3 Cited publications
- 28 bookElements of the Theory of Elliptic Functions.AMS1990back to text
- 29 articleOn the Differential Structure of Matrix-Valued Rational Inner Functions.Operator Theory~: Advances and Applications731994, 30--66back to textback to textback to text
- 30 bookPadé approximants.Cambridge University Press2010back to text
- 31 bookInterpolation of rational matrix functions.Birkhäuser1990back to text
-
32
articleA remark on uniqueness of best rational approximants of degree 1 in
- 33 articlePseudo-holomorphic functions at the critical exponent.Journal of the European Mathematical Society1892016HALDOIback to textback to text
- 34 articleUniqueness results for inverse Robin problems with bounded coefficient.Journal of Functional Analysis2016HALDOIback to textback to text
-
35
articleIdentification and rational
- 36 articleA Grobman-Hartman theorem for control systems.J. Dyn. Differential Eqs.192007, 75-107back to text
- 37 articleDirichlet/Neumann problems and Hardy classes for the planar conductivity equation.Complex Variables and Elliptic Equations2014, 41HALDOIback to textback to text
- 38 articleDecomposition of L2-vector fields on Lipschitz surfaces: characterization via null-spaces of the scalar potential.SIAM Journal on Mathematical Analysis5342021, 4096 - 4117HALDOIback to text
- 39 articleUnique reconstruction of simple magnetizations from their magnetic potential.Inverse Problems3710September 2021, 105006HALDOIback to text
- 40 inproceedingsOrthogonal rational functions and nonstationary stochastic processes: a Szegő theory.Proc. 19th Symposium on Mathematical Theory of Networks and SystemsBudapest2010back to text
- 41 articleCharacterizing kernels of operators related to thin-plate magnetizations via generalizations of Hodge decompositions.Inverse Problems2912013, URL: https://hal.inria.fr/hal-00919261DOIback to textback to textback to text
- 42 articleZero distributions via orthogonality.Annales de l'Institut Fourier5552005, 1455--1499back to textback to text
- 43 articleMultipoint Schur algorithm and orthogonal rational functions: convergence properties, I.Journal d'Analyse1122011, 207-255URL: https://arxiv.org/abs/0812.2050v3back to text
-
44
articleHardy approximation to
- 45 articleHow can meromorphic approximation help to solve some 2D inverse problems for the Laplacian?Inverse Problems1511999, 79--90URL: https://dx.doi.org/10.1088/0266-5611/15/1/012back to textback to textback to text
- 46 articleSources identification in 3D balls using meromorphic approximation in 2D disks.Electronic Transactions on Numerical Analysis (ETNA)252006, 41--53back to textback to text
-
47
articleHardy approximation to
- 48 articleConstrained extremal problems in H2 and Carleman's formulas.Matematicheskii Sbornik20972018, 36HALback to text
- 49 article2D inverse problems for the Laplacian: a meromorphic approximation approach.Journal de Math. Pures et Appliquées862008, 1-41back to textback to textback to textback to text
-
50
articleCritical points and error rank in best
-
51
articleIndex of critical points in
-
52
inproceedingsOn the
- 53 unpublishedHardy-Hodge decomposition of vector fields on compact Lipschitz hypersurfaces., in preparationback to text
-
54
articleA criterion for uniqueness of a critical point in
-
55
articleAn
-
56
articleAsymptotic uniqueness of best rational approximants of given degree to Markov functions in
-
57
incollectionAsymptotic uniqueness of best rational approximants to complex Cauchy transforms in
- 58 articleConvergent Interpolation to Cauchy Integrals over Analytic Arcs with Jacobi-Type Weights.International Mathematics Research Notices2010222010, 4211--4275URL: https://hal.inria.fr/hal-00508314back to text
- 59 articleConvergent interpolation to Cauchy integrals over analytic arcs.Found. Comp. Math.962009, 675--715back to text
- 60 articleMeromorphic approximants for complex Cauchy transforms with polar singularities.Mat. Sbornik20092009, 3-40back to text
- 61 articleSources recovery from boundary data: a model related to electroencephalography.Mathematical and Computer Modelling4911--122009, 2213--2223URL: https://dx.doi.org/10.1016/j.mcm.2008.07.016back to textback to text
- 62 articleExhaustive approach to the coupling matrix synthesis problem and application to the design of high degree asymmetric filters.International Journal of RF and Microwave Computer-Aided Engineering1712007, 4--12URL: https://hal.inria.fr/hal-00663777back to text
- 63 articleLogarithmic stability estimates for a Robin coefficient in 2D Laplace inverse problems.Inverse Problems2012004, 49--57URL: https://dx.doi.org/10.1088/0266-5611/20/1/003back to text
- 64 phdthesisAnalyse complexe et problèmes de Dirichlet dans le plan : équation de Weinstein et autres conductivités non bornées.Mathématiques et Informatique de Marseille2013back to text
- 65 articleA controllability method for Maxwell's equations.arXiv preprint arXiv:2106.028582021back to text
- 66 articleFactorization theorems for Hardy spaces in several variables.Ann. Math.1031976, 611--635back to text
- 67 phdthesisApproximation des des classes de fonctions analytiques généralisées et résolution de problèmes inverses pour les tokamaks.Univ. Nice Sophia Antipolis2011, URL: https://tel.archives-ouvertes.fr/tel-00643239/back to text
- 68 articleDetermining cracks by boundary measurements.Indiana Univ. Math. J.3831989, 527--556back to text
-
69
articleMatrix rational
- 70 bookBounded analytic functions.Academic Press1981back to text
- 71 articleA topological approach to Nevanlinna-Pick interpolation.SIAM J. Math. Anal.1851987, 1248--1260back to text
- 72 articleBalanced realizations of discrete-time stable all-pass systems and the tangential Schur algorithm.Linear Algebra and its Applications4182006, 793-820URL: https://dx.doi.org/10.1016/j.laa.2006.09.029back to textback to textback to text
- 73 articleFrequency domain analysis and analytic selections.Indiana Univ. Math. J.3911990, 157--184back to text
- 74 bookGeometric function theory and non-linear analysis.Oxford Univ. Press2001back to text
- 75 articleThe computation of resonances in open systems using a perfectly matched layer.Mathematics of Computation782672009, 1375--1398back to text
- 76 articleOn the construction of frames for spaces of distributions.Journal of Functional Analysis2572009, 2159--2187back to text
- 77 bookFoundations of modern potential theory.Springer-Verlag1972back to text
- 78 bookSome Improperly Posed Problems of Mathematical Physics.Springer1967back to text
- 79 inbookTopics in Classical and Modern Analysis. Applied and Numerical Harmonic Analysis.M.M. AbellE.E. IacobA.A. StokolosS.S. TaylorS.S. TikhonovJ.J. ZhuBirkhäuser2019, The Spurious Side of Diagonal Multipoint Padé Approximantsback to text
- 80 articleCertified Computation of Optimal Multiband Filtering Functions.IEEE Transactions on Microwave Theory and Techniques5612008, 105-112URL: https://dx.doi.org/10.1109/TMTT.2007.912234back to text
- 81 articleNudelman Interpolation, Parametrization of Lossless Functions and balanced realizations.Automatica432007, 1329--1338URL: https://dx.doi.org/10.1016/j.automatica.2007.01.020back to textback to textback to text
- 82 bookHankel Operators and their Applications.Springer2003back to textback to text
- 83 articleConstrained Hardy space approximation.J. Approx. Theory82010, 1466--1483back to text
- 84 articleÜber Potenzreihen die im innern des einheitskreises beschränkt sind.J. Reine Angew. Math.1471917, 205--232back to text
- 85 inproceedingsExtraction of coupling parameters for microwave filters: determinati on of a stable rational model from scattering data.2003 IEEE MTT-S International Microwave Symposium Digest1Philadelphie, États-UnisIEEE2003, 25--28URL: https://hal.inria.fr/hal-00663504back to text
- 86 bookHarmonic Analysis.Princeton University Press1993back to text
- 87 bookStability analysis of nonlinear microwave circuits.Artech House2003back to text
-
88
inproceedingsCounterexamples with harmonic gradients in
- 89 inproceedingsComparison of SEM Methods for Poles Estimation from Scattered Field by Canonical Objects.Renaissance meets advancing technologyFlorence, ItalySeptember 2020, 6URL: https://hal.archives-ouvertes.fr/hal-02941637DOIback to text

