EN FR
EN FR
VALSE - 2024
VALSE - 2024

2024Activity reportProject-TeamVALSE

RNSR: 201923115X
  • Research center Inria Centre at the University of Lille
  • In partnership with:Ecole Centrale de Lille, Université de Lille
  • Finite-time control and estimation for distributed systems
  • In collaboration with:Centre de Recherche en Informatique, Signal et Automatique de Lille
  • Domain:Applied Mathematics, Computation and Simulation
  • Theme:Optimization and control of dynamic systems

Keywords

Computer Science and Digital Science

  • A5.9.2. Estimation, modeling
  • A6.4.1. Deterministic control
  • A6.4.4. Stability and Stabilization
  • A6.4.5. Control of distributed parameter systems
  • A9.5. Robotics

Other Research Topics and Application Domains

  • B1.1.8. Mathematical biology
  • B2.1. Well being
  • B5.6. Robotic systems
  • B7.2.1. Smart vehicles

1 Team members, visitors, external collaborators

Research Scientists

  • Denis Efimov [Team leader, INRIA, Senior Researcher, HDR]
  • Jin Gyu Lee [INRIA, ISFP, until Jul 2024]
  • Andrey Polyakov [INRIA, Researcher, HDR]
  • Rosane Ushirobira [INRIA, Researcher, HDR]

Post-Doctoral Fellows

  • Ajul Dinesh [INRIA, Post-Doctoral Fellow, from Mar 2024]
  • Radoslaw Patelski [INRIA, Post-Doctoral Fellow, from Oct 2024]

PhD Students

  • Isaac Ambit Brao [INRIA, from Oct 2024]
  • Mohamed Yassine Arkhis [INRIA]
  • Mericel Ayamou [UNIV LILLE]
  • Mahugnon Dadjo [INRAE]
  • Min Li [CSC Scholarship]
  • Danilo Rodrigues De Lima [INRIA]
  • Yu Zhou [CSC Scholarship, until Oct 2024]

Interns and Apprentices

  • Thim Van Zandbeek [INRIA, Intern, from Feb 2024 until May 2024]

Administrative Assistants

  • Isabelle Aslani [INRIA]
  • Aurore Dalle [INRIA]
  • Anne Rejl [INRIA]
  • Amélie Supervielle [INRIA]

Visiting Scientist

  • Manuel Mera [IPN MEXICO, from Sep 2024 until Oct 2024]

External Collaborator

  • Gerald Dherbomez [CNRS]

2 Overall objectives

The VALSE team studies estimation and control problems arising in the analysis and the design of distributed, uncertain, and interconnected dynamical systems:

  • Using the concepts of finite-time/fixed-time/hyperexponential convergence and stability, the main idea is to separate and hierarchize in time the control and estimation processes, which are distributed in space. These approaches greatly simplify their analysis and the design of large-scale solutions.
  • The main areas of investigation and application for the team are the Internet of Things (IoT) and Cyber-Physical Systems (CPS).
  • The team aims to draw up algorithms for decentralized finite-time control and estimation. The methodology to be developed includes extensions of the theory of homogeneous systems and finite-time/fixed-time/hyper-exponential convergence and stability notions. Particular attention is given to applications in real-world scenarios.
  • It is a joint proposal with the CNRS CRIStAL UMR 9189.

3 Research program

The VALSE team works in the domains of control science: dynamical systems, stability analysis, estimation, and automatic control. Our developments are focused on the theoretical and applied aspects related to the control and estimation of large-scale multi-sensor and multi-actuator systems based on the use of the theories of finite-time/fixed-time/hyperexponential convergence and homogeneous systems. The Lyapunov function method and other methods of analysis of dynamical systems form a basis for the studies in the VALSE team.

The key idea of the research program for the team is that a fast (non-asymptotic) convergence of the regulation and estimation errors increases the reliability of intelligent distributed actuators and sensors in complex scenarios, such as interconnected cyber-physical systems (CPS).

The expertise of VALSE's members in theoretical developments of control and estimation theory (finite-time control and estimation algorithms in centralized context 72, 58, 69, 68, 65, homogeneity framework for differential equations 73, 60, 59, 61, 63, 74, 70, time-delay systems 62, 64, 77, distributed systems 71 and algebraic-based methods for estimation 75, 76) is an essential ingredient to achieve our objective.

The generic chart of goals and tasks included in the scientific work program of VALSE and the interrelations between them are presented in Fig. 1. We have selected three main objectives to pursue with the related tasks to fulfill:

  • The first objective is to design control and estimation solutions for CPS and IoT, which is the principal aim of VALSE. It will contain the main outcomes of our research.
  • The second objective is more theoretical and needed to make the basement for our design and analysis parts in the last goal.
  • The third objective deals with applications that will drive the team and motivate the theoretical studies and selected design performances.

All these objectives are interconnected: from a particular problem in an IoT application, it is planned to design control or estimation algorithms, leading to the development of theoretical tools; and vice versa, a new theoretical advance can provide a possibility for the development of novel tools that can be used in applications.

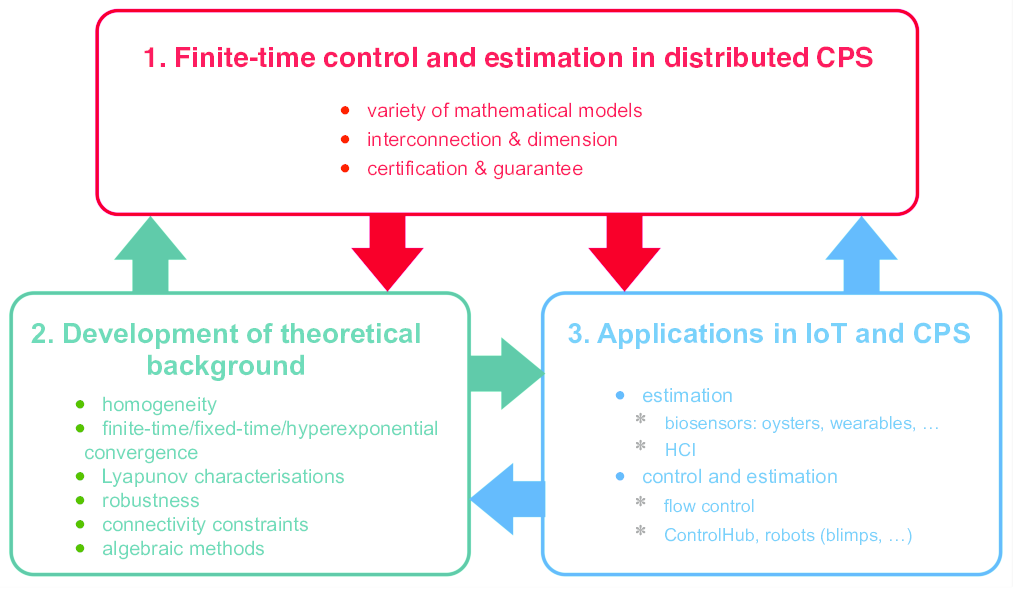
Figure 1

Graphical presentation of objectives given in the text

Figure 1:

Structure of the objectives and tasks treated in VALSE

To explain our motivation: why use finite time? Applying any method for control/estimation has a price in terms of its advantages and disadvantages. There is no universal framework that is the best always and everywhere. Finite-time may appear as a luxurious property for a physical system, requiring nonlinear tools. Of course, if an asymptotic convergence and a linear model are enough for solving a given problem, then there is no reason to develop something else. However, most of the present problems in CPS and IoT are nonlinear (i.e., they have various local behaviors that cannot be collected in only one linear model). Design and analysis of various local linearized models and solutions are luxurious, too. The theory of homogeneity can go beyond linearity, offering many new features while not appearing as severe as other nonlinear tools and having almost all hints of the linear framework. Suppose that, thanks to the homogeneity theory, finite-time/fixed-time can be obtained with little difficulty while adding the bonuses of stronger robustness and faster convergence compared to the linear case? We are convinced that the price of going beyond linear control and estimation can be strongly dropped by maturing the theory of homogeneity and finite/fixed-time convergence. We are also convinced that it will be compensated in terms of robustness and speed, which can be demanded in the new areas of application such as IoT for example.

4 Application domains

One of the objectives of the team is to apply the developed control and estimation algorithms for different scenarios in IoT or CPS. Participation in various potential applications allows the VALSE team to better understand the features of CPS and their required performances and to properly formulate the control and estimation problems that must be solved. Here is a list of ongoing, past, and potential applications addressed by the team:

  • smart bivalve-based biosensor for water quality monitoring (ANR project WaQMoS, the developed sensor is shown in Fig. 2): in living beings, the presence of persistent external perturbations may be difficult to measure, and important model uncertainties render the application of conventional techniques complicated; another issue for estimation is the consensus-seeking between animals for contamination detection 56;
    Figure 2

    Underwater photo of bivalves connected to computer

    Figure 2:

    The valvometer used in the ANR project WaQMoS

  • control and estimation for flying vehicles, e.g. quadrotors or blimps given in Fig. 3: the nonlinearity of the model and its uncertainty coupled with important aerodynamic perturbations have to be compensated by fast (finite- or fixed-time) and robust control and estimation algorithms;
    Figure 3.a
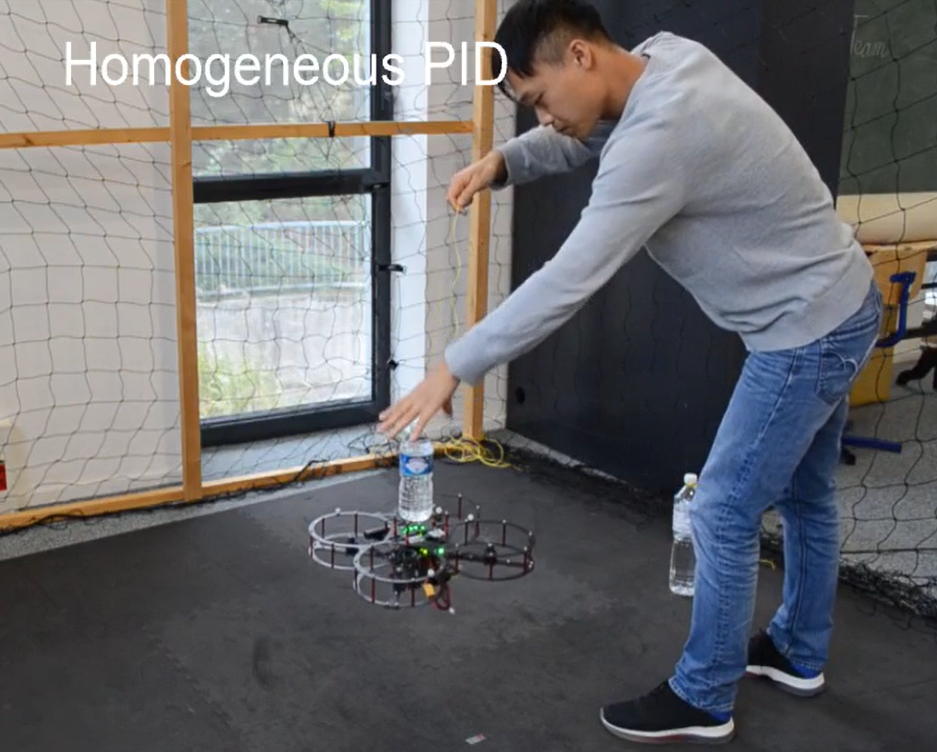
    Figure 3.b

    1) Photo of blimp robot, big ball with helium with a small computer at the bottom. 2) S. Wang placing a bottle of water on the flying drone

    1) Photo of blimp robot, big ball with helium with a small computer at the bottom. 2) S. Wang placing a bottle of water on the flying drone

    Figure 3:

    Blimp and quadrotor robots

  • human behavior modeling and identification with the posterior design of algorithms for human-computer interaction (HCI, with the Inria team LOKI): robust finite-time differentiators demonstrate good estimation capabilities needed for prediction in this application 76, 57;
  • human physiological characteristics estimation (like emotion detection, galvanic skin response filtering, fatigue evaluation in collaborations with Neotrope and Ellcie Healthy): intelligent robust filtering and finite-time distributed estimation are key features in these scenarios;
  • path planning for autonomous vehicles taking into account the behavior of humans (with the Inria team SCOOL): application of interval and finite-time adaptive estimation and prediction techniques allows for treating the uncertainty of the environment by reducing the computational complexity of reinforcement learning 671;
  • flow control 66: the case of control and estimation of a distributed-parameter system with very fast and uncertain dynamics, where finite-time solutions developed by VALSE are necessary (an example of results is given in Fig. 4);
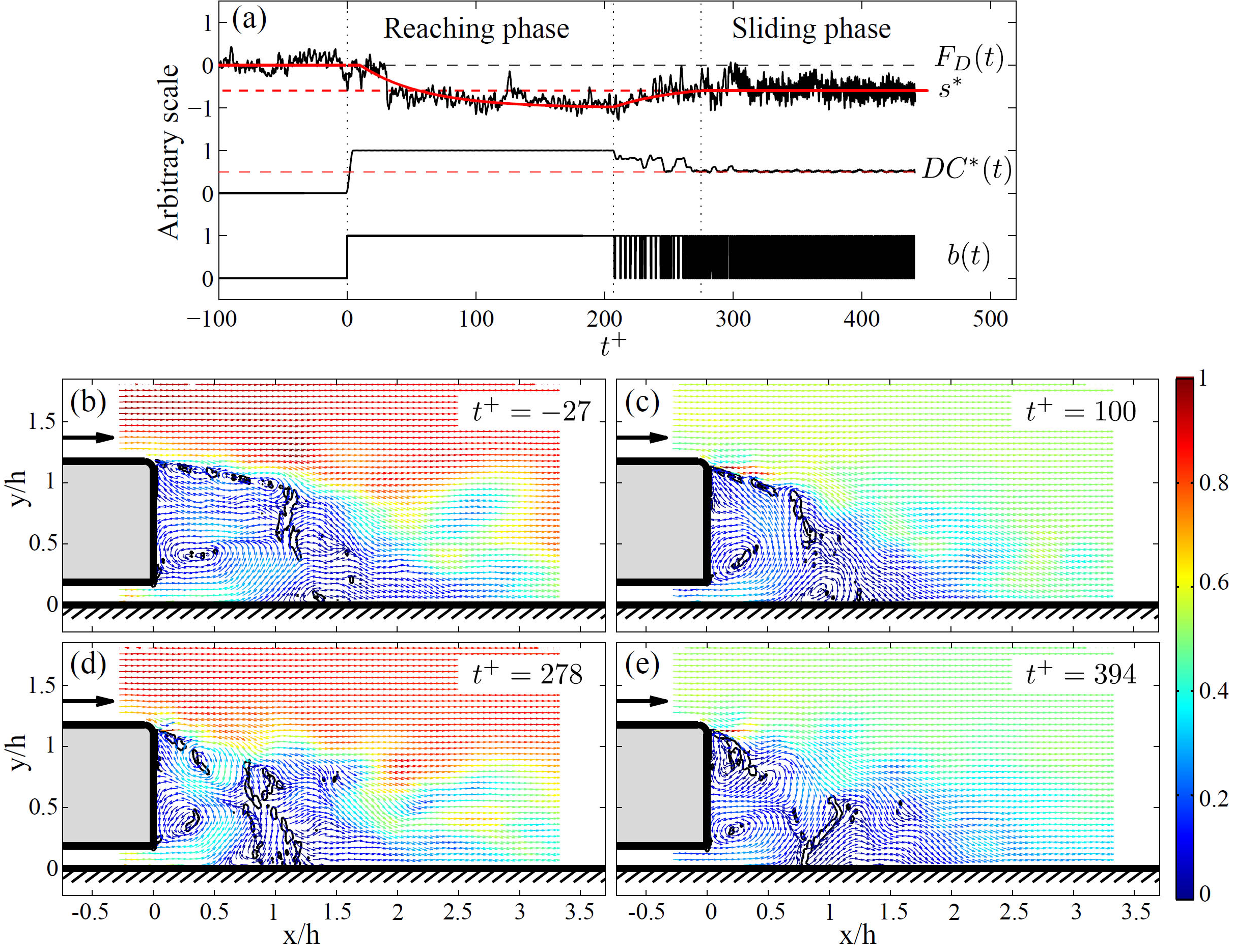
    Figure 4

    Different graphics demonstrating flow behavior and the control input

    Figure 4:

    Particle Image Velocimetry on flow control for an Ahmed body (LAMIH wind tunnel)

  • control of bioreactors (in the framework of IPL COSY): here again, the problem is an important uncertainty of the model, which can be handled by robust sliding mode control algorithms, or by applying adaptive finite-time estimation and identification tools;

It is worth highlighting a widespread distribution of various scientific domains in the list of applications for the team given above. Such interdisciplinarity for VALSE is unsurprising since control theory is a science of systems whose interest today is, by nature, to interface with other disciplines and their fields of application. This is also well aligned with the domain of CPS, which by its origin requires multidisciplinary competencies.

5 Social and environmental responsibility

Activities of the team related to social responsibility:

  • Engaging in outreach programs to promote mathematics education and awareness in local communities: Rosane Ushirobira participates in the work of CIMPA.
  • Collaborating with educational institutions to support the development of math skills for the young generation: Rosane Ushirobira made several CHICHE sessions in high schools of Lille and in the metropolitan area.
  • Participating in mentorship programs to encourage underrepresented groups to pursue careers in mathematics and related fields: Rosane Ushirobira participated in the days of young girls in informatics and mathematics (RJMI Inria Lille 2024).
  • Contributing to interdisciplinary research that addresses societal challenges, such as healthcare, by applying mathematical modeling and analysis: the ANR project NOCIME has VALSE participation in the analysis of mathematical epidemiological models. In addition, there are publications on this subject.
  • Mentoring and supporting early-career researchers to foster a diverse and inclusive research community.

Activities of the team related to environmental responsibility:

  • Developing mathematical models and the methods for their design and analysis to support sustainable energy systems: the ANR project SyNPiD was devoted to this issue
  • Collaborating with environmental scientists, engineers, and policymakers to provide mathematical insights and solutions for environmental challenges and their mitigations: a Ph.D. student is supervised by INRAE and VALSE on the wastewater treatment problems

Overall, social and environmental responsibility for researchers in the team involves using mathematical expertise to address societal issues, promote inclusivity, and contribute to environmental sustainability through research, collaboration, and outreach efforts.

6 Highlights of the year

  • Four members of the team attended IEEE CDC in Milan, where our team had 12 accepted publications.
  • Among 20 journal publications of the team this year, 4 are in Automatica and 7 in IEEE TAC (the top journals in the domain of the theory of control), which is 55 % of scientific production.

7 New software, platforms, open data

7.1 New software

7.1.1 HCS Toolbox

  • Name:
    Homogeneous Systems Control Toolbox (HSC Toolbox) for MATLAB
  • Keywords:
    Control design, Matlab, Homogeneity
  • Functional Description:
    Homogeneous Systems Control Toolbox (HCS Toolbox) for MATLAB is a collection of functions for design and tuning of control systems with improved control quality (faster convergences, better robustness, smaller overshoots, etc) based on the concept of a dilation symmetry (homogeneity). Homogeneous controllers/observers design well as procedures for upgrading of existing linear controllers/observers to nonlinear (homogeneous) ones are developed for both Single-Input Single-Output (SISO) and Multiply-Input Multiply-Output (MIMO) systems.
  • Release Contributions:

    HCS Toolbox for MATLAB ver. 0.1

    This is the first release of HCS Toolbox for MATLAB. The list of MATLAB functions provided for homogeneous control systems design: (Homogeneous Objects) hnorm - computation of homogeneous norm hproj - computation of homogeneous projection hcurve - generation of points of a homogeneous curve hsphere - generation of a random grid on a homogeneous sphere (Homogeneous Control Design) hpc_design - Homogeneous Proportional Control (HPC) design hpci_design - Homogeneous Proportional-Integral Control (HPIC) design hsmc_design - Homogeneous Sliding Mode Controller (HSMC) design hsmci_design - design of HSMC with Integral action fhpc_design - Fixed-time HPC design fhpic_design - Fixed-time HPIC design lpc2hpc - upgrading Linear Proportional Control (LPC) to HPC lpic2hpc - upgrading Linear PI control (LPIC) to HPIC (Discretization of Homogeneous Control) e_hpc - explicit discretization of HPC e_hpc - semi-implicit discretization of HPC c_hpc - consistent discretization of HPC e_hpic - explicit discretization of HPIC e_hsmc - explicit discretization of HSMC si_hsmc - semi-implicit explicit discretization of HSMC e_hsmci - explicit discretization of HSMC with Integral action e_fhpc - explicit discretization of Fixed-time HPC si_fhpc - semi-implicit discretization of Fixed-time HPC e_fhpic - explicit discretization of Fixed-time HPIC (Homogeneous Observer Design) ho_design - Homogeneous Observer (HO) design fho_design - Fixed-time HO design lo2ho - upgrading Linear Observer (LO) to HO (Discretization of Homogeneous Observer) e_ho - explicit Euler discretization of HO e_fho - explicit Euler discretization of FHO si_ho - semi-implicit discretization of HO si_fho - semi-implicit discretization of FHO (Block forms ) block_con - transformation to block controlability form bloc_obs - transformation to block bservability form trans_con - transformation to partial block controlability form trans_con - transformation to partial block observability form output_form - transformation to reduced order output control system (Examples) demo_hnorm - demo of computation of a homogeneous norm demo_hsphere - plot of homogeneous balls in 2D demo_hpc - demo of HPC design and simulation demo_hpic - demo of HPIC design and simulation demo_hsmc - demo of HSMC design and simulation demo_hsmci - demo of HSMCI design and simulation demo_fhpc - demo of FHPC design and simulation demo_fhpic - demo of FHPIC design and simulation demo_lpc2hpc - demo of upgrading LPC to HPC/FHPC demo_lpic2hpic - demo of upgrading LPIC to HPIC/FHPIC demo_ho - demo of HO design and simulation demo_fho - demo of FHO design and simulation demo_lo2ho - demo of upgrading LO to HO/FHO For more details please read the documentation: HCS_doc.pdf

  • URL:
    https://­gitlab.­inria.­fr/­polyakov/­hcs-toolbox-for-matlab
  • Contact:
    Andrey Polyakov

8 New results

8.1 Analysis and design of homogeneous and finite-time stable systems

Participants: Denis Efimov, Andrey Polyakov, Rosane Ushirobira.

In 22, it is presented a linear time-varying state observer for a chain of integrators having bounded disturbances in the last equation. The authors demonstrate that the estimation error converges to zero for the continuous-time realization with a hyper-exponential rate (faster than any exponential) uniformly in the disturbance in the noise-free setting. An implicit discretization scheme of the observer is proposed, which preserves all main properties of the continuous-time counterpart in discrete time. In addition, the discrete-time estimation error is robustly stable with respect to the measurement noise. The efficiency of the suggested observer is illustrated by comparing it with a linear high-gain observer and a sliding mode high-order differentiator.

The paper 27 considers a class of homogeneous systems with sector nonlinearities. Sufficient finite-time (input-to-state) stability conditions are established using new constructive modifications of the Implicit Lyapunov Function approach. The proposed conditions are given in the form of linear matrix inequalities. The theoretical results are supported with numerical examples.

The paper 36 applies the physics-informed neural network approach to approximate globally defined Lyapunov functions and stabilizing controls for homogeneous dynamical systems. The advantage of this class of systems is that all analysis and design can be made locally on a suitably defined unit sphere, which corresponds perfectly to the applicability conditions of neural networks.

In 18, the authors develop a finite/fixed-time control design procedure for an infinite dimensional system modeled by an abstract evolution equation in a Hilbert space. It is based on solving certain operator equations and inequalities. For a class of PDE models, the corresponding equations/inequalities are shown to be algebraic and solvable in many cases. Examples of controlled PDE models support theoretical results.

8.2 Analysis and design for systems involving delays

Participants: Denis Efimov, Andrey Polyakov.

In 6, it is proposed conditions for local input-to-state stability for a second-order system with vector position, either constant or time-varying delays, and a power nonlinearity of the degree higher than one, which does not contain a velocity-proportional damping term. The result is based on applying the Lyapunov-Razumikhin approach, for which new time estimates on the decay of solutions are obtained. The approach is extended to attitude stabilization of a rigid body, and simulations illustrate it.

In 7, the stability problem for nonlinear homogeneous systems with distributed delay and variable kernel is studied. Both the Lyapunov–Krasovskii and the Razumikhin approaches are applied. It is proved that the global asymptotic stability of the zero solution for an auxiliary delay-free homogeneous system implies the local asymptotic stability of the zero solution for the original system with distributed delay. Moreover, the impact of nonlinear time-varying perturbations on the system dynamics is analyzed by applying the averaging techniques. The results are illustrated by a mechanical system described by a Lienard equation and an indirect control linear system design.

A static non-linear homogeneous feedback for a fixed-time stabilization of a linear time-invariant (LTI) system is proposed in 17. This feedback is designed so that the settling time is assigned exactly to a prescribed constant for all nonzero initial conditions. The constant convergence time is achieved due to a dependence on the feedback gain of the system's initial state. The robustness of the closed-loop system with respect to measurement noise and exogenous perturbations is studied using the concept of Input-to-State Stability (ISS). Both delay-free and input-delay systems are considered. Numerical simulations illustrate theoretical results.

8.3 Control and estimation for Persidskii systems

Participants: Denis Efimov, Rosane Ushirobira.

Generalized Persidskii systems (or Lur'e systems) represent the dynamics described by the superposition of a linear part with multiple sector nonlinearities and exogenous perturbations. They can be used to model many physical and engineering phenomena.

The paper 37 proposes a state estimator for a class of nonlinear systems that includes the Persidskii systems with bilinear cross-terms. The estimation error analysis is based on the input-to-output stability theory and formulated using linear matrix inequalities. Simulations are provided for a model of consumer-resource interaction.

8.4 Control of robotic systems

Participants: Denis Efimov, Andrey Polyakov, Rosane Ushirobira.

In 24, the attitude tracking problem for a full-actuated rigid body in 3D is studied using a system model based on the Lie algebra so(3). A nonlinear homogeneous controller is designed to track a smooth attitude trajectory in a finite/fixed time. A global settling time estimate is obtained and easily adjustable by tuning the homogeneity degree. The input-to-state stability of the control system with respect to measurement noises and additive perturbations is studied. Simulations illustrating the performance of the proposed algorithm are presented.

The paper 19 proposes designing a robust control strategy for the trajectory tracking problem in perturbed unicycle mobile robots. The proposed strategy comprises the design of a robust control law based on an Integral Sliding–Mode Control (ISMC) approach and an interval predictor–based state–feedback controller through a Model Predictive Control (MPC) scheme. The robust controller deals with some perturbations in the kinematic model, which represent slipping effects, and with state and input constraints that are related to restrictions on the workspace and saturated actuators, respectively. The proposed approach guarantees the exponential convergence to zero of the tracking error. The proposed approach's performance is validated through simulations tracking a lemniscate and a circumference curve.

A sampled robust controller for the trajectory tracking problem in constrained unicycle mobile robots is proposed in 34. The controller comprises the design of an aperiodic control law based on an event-triggered controller and a periodic control law based on a constant sampled state-feedback controller. The state feedback-based event-triggered control is designed using the attractive ellipsoid method and the barrier Lyapunov function and provides a safe set where the state constraints are not transgressed and a switching set that defines the region where each part of the controller is active. The periodic sampled control part is designed considering a maximum sampling time and is active inside the switching set. The proposed strategy ensures the input-to-state stability of the tracking error dynamics concerning external disturbances. A constructive and simple method based on linear matrix inequalities is proposed to compute the controller gains. Simulation results illustrate the feasibility of the proposed approach.

The design of an evader controller for the pursuit-evasion problem is proposed in 38, where single integrator dynamics describe both agents. The proposed evader controller only requires a slight knowledge of the law and state governing the pursuer control. The control law guarantees the evasion of the pursuer under a proper design of the evader controller parameters. Moreover, the synthesis of the evader controller is constructive and simple to tune since it is a linear matrix inequality. The stability analysis of the tracking error dynamics is based on a Lyapunov-like function approach. The effectiveness of the proposed evader control design is illustrated through some simulation results.

8.5 State estimation for mathematical epidemiological models

Participants: Denis Efimov, Rosane Ushirobira.

Observation and identification are crucially important for the practical use of compartmental models in epidemiology. Typically, the state and parameters of the epidemic model are evaluated based on the number of infected individuals (prevalence) or the newly infected cases per time unit (incidence). However, when reinfections are possible, additional data, such as the number of primary infections, are retrievable. In 50, the authors investigate whether measuring the number of primary infections can improve estimation. To do so, they present several nonlinear adaptive observers for a simple infection model with waning immunity and subsequent reinfections. They then prove the practical asymptotic stability of the estimation errors using the Lyapunov function method. Finally, we illustrate the efficacy of the observers via simulations.

8.6 Parameter estimation via algebraic methods

Participants: Denis Efimov, Rosane Ushirobira.

A simple fixed-time converging estimation algorithm is presented in 12 for a linear regression using the dynamic regressor extension and mixing (DREM) method within a discrete-time setting, with a persistently excited regressor and bounded measurement noises. The solution is based on Kreisselmeier's filters and is computationally more straightforward than the existing analogs.

8.7 Application to the wastewater problem

Participants: Denis Efimov, Rosane Ushirobira.

In 30, the authors consider a simplified model of crop fertirrigation as a non-autonomous controlled system, with soil moisture, nitrogen content, and biomass as state variables and the delivered water flow rate as input. They study the problem of minimizing the total water quantity delivered during the agricultural season under the constraints that the crops are not suffering from water or nitrogen stress at any time. They establish sufficient conditions for the feasibility of the problem and depict several control strategies depending on the initial nitrogen content. In particular, they show that this problem can exhibit an infinity of singular trajectories of the same cost.

9 Bilateral contracts and grants with industry

9.1 Bilateral contracts with industry

Participants: Denis Efimov, Rosane Ushirobira.

  • 52 Hertz is a startup (Brest, France) that develops an underwater communication device for divers. This contract aimed to develop an intelligent filtering algorithm that compensates for voice deformation during underwater vocal communication through the device. The code for the filter was written in Matlab, with further help for its adaptation in Python and C.

10 Partnerships and cooperations

10.1 International initiatives

10.1.1 Participation in other International Programs

Inria Challenge ImAnAI

Participants: Denis Efimov.

  • Title:
    Improved Bearings-only Target Motion Analysis Using AI Tools
  • Partner Institution(s):
    ITT Delhi, India and Naval Group
  • Date/Duration:
    2024 – 2027
  • Additionnal info/keywords:
    The conventional problem of bearings-only target motion analysis (BOTMA) is studied in the project. It assumes that the two mobile agents, observer and target, are on the same plane and that they (or at least the target) have a constant speed during the observation period. The goal is to estimate the position of a target, namely two coordinates of geographical location and the projections of the speed on two axes, from a set of available bearing measurements collected by the observer. To this end, IIT Delhi, Naval Group, and Inria researchers will develop various estimation and tracking algorithms by combining modern methods from the theories of control, estimation, tracking, and artificial intelligence. Denis Efimov is the PI.

ECOS NORD

Participants: Denis Efimov, Andrey Polyakov, Rosane Ushirobira.

  • Title:
    Artificial Intelligence-based Control Approaches for Multiple Mobile Robots
  • Partner Institution(s):
    Instituto Technológico de la Laguna, Mexico
  • Date/Duration:
    2021 – 2024
  • Additionnal info/keywords:
    This project was focused on studying several tracking tasks for autonomous mobile robot systems, particularly unmanned aerial vehicles and wheeled mobile robots. This project aimed to develop robust control and navigation schemes by combining the methods of artificial intelligence and control theory.

PHC Aurora

Participants: Denis Efimov, Rosane Ushirobira.

  • Title:
    Equivalent nonlinear control methods for digitalization in robotics and autonomous systems
  • Partner Institution(s):
    University of Agder, Norway
  • Date/Duration:
    2023 – 2024
  • Additionnal info/keywords:
    The robotic and autonomous systems rely heavily on the complex, often distributed, and hierarchical control systems, which ensure main functionalities with a flexible and safe operation. The classical automatic controllers with feedback from the acquisition and perception devices continue to experience new challenges coming from an increasing complexity in the dynamic behavior of the systems to be controlled on the one hand, and from the digitalization and associated transformations of data, their spatial availability and communication time delays on the other hand. To propose the advanced nonlinear control methods which, however, would be equivalent (in terms of simplicity of tuning and implementation) to the widely accepted standard linear controls (such as PID), and in this way would be more accessible for future applications, is the scientific scope of this project.

10.2 International research visitors

10.2.1 Visits of international scientists

Participants: Denis Efimov, Andrey Polyakov, Rosane Ushirobira.

Manuel Mera
  • Status:
    Researcher
  • Institution of origin:
    Instituto Politécnico National
  • Country:
    Mexico
  • Dates:
    September 3 to October 4
  • Context of the visit:
    Collaboration
  • Mobility program/type of mobility:
    Inria invited
Héctor Ríos
  • Status:
    Professor
  • Institution of origin:
    Instituto Technológico de la Laguna
  • Country:
    Mexico
  • Dates:
    September 3 to October 4
  • Context of the visit:
    Collaboration
  • Mobility program/type of mobility:
    ECOS NORD

10.3 National initiatives

10.3.1 ANR

  • NOCIME (New Observation and Control Issues Motivated by Epidemiology), coordinator Pierre-Alexandre Bliman (Inria, Paris)
  • SyNPiD (Synchronization in power networks with periodic dynamics), coordinators Denis Efimov (Inria, France) and Johannes Schiffer (Brandenburg University of Technology Cottbus-Senftenberg, Germany)

11 Dissemination

11.1 Promoting scientific activities

11.1.1 Scientific events: organisation

  • Denis Efimov, IEEE CDC, Milano, Italy
  • Denis Efimov, IEEE Workshop on Variable Structure Systems, Abu Dhabi, UAE

11.1.2 Journal

Member of the editorial boards
  • Denis Efimov, Associate editor, IEEE Transactions on Automatic Control
  • Denis Efimov, Associate editor, Automatica
  • Rosane Ushirobira, Associate editor, Asian Journal of Control
  • Rosane Ushirobira, Associate editor, Trends in Computational and Applied Mathematics (TEMA)

11.1.3 Invited talks

  • Denis Efimov, Louisiana State University, US
  • Denis Efimov, Nanjing South East University, China
  • Rosane Ushirobira, Instituto Technológico de la Laguna, Mexico

11.1.4 Leadership within the scientific community

  • Denis Efimov, Senior member IEEE
  • Denis Efimov, Member of IFAC TC 1.2. Adaptive and Learning Systems
  • Denis Efimov, Publication vice-chair of IFAC TC 9.2. Systems and Control for Societal Impact
  • Denis Efimov, Executive committee member, IEEE CSS Technical Committee on Variable Structure and Sliding Mode Control
  • Denis Efimov, Co-chair of European PhD Award on Control for Complex and Heterogeneous Systems
  • Rosane Ushirobira, Scientific Officer at CIMPA (Centre International de Mathématiques Pures et Appliquées), until Aug. 2024

11.1.5 Research administration

  • Rosane Ushirobira, Elected member of the Scientific Board of Inria
  • Andrey Polyakov, Jury member of the CRCN/ISFP Inria Lille
  • Rosane Ushirobira, Jury member of the CoS MCF CNU 61 U Valenciennes and CoS MCF CNU 61 CNAM Paris

11.2 Teaching - Supervision - Juries

11.2.1 Teaching

  • Licence: Rosane Ushirobira , Basic courses in Linear algebra and Calculus, 76h, L3, Polytech Lille

11.2.2 Juries

The members of the team participated in numerous Ph.D. defense committees.

11.3 Popularization

11.3.1 Internal Inria responsibilities

  • Denis Efimov, Member of IES comittee
  • Rosane Ushirobira, Scientific Officer for Scientific Popularization (Inria Lille) until June 2024
  • Rosane Ushirobira, Co-organizer of 30 MIN. de sciences (monthly seminar for scientists in the center)

11.3.2 Interventions

  • Rosane Ushirobira, Organizer of CHICHE sessions in Lille Academy, until June 2024. Gave 4 talks to high-school students within this framework
  • Rosane Ushirobira, Participated to the 4th Rendez-vous des Jeunes Mathématiciennes et Informaticiennes (RJMI) at Inria Lille

12 Scientific production

12.1 Major publications

12.2 Publications of the year

International journals

International peer-reviewed conferences

  • 29 inproceedingsM. Y.Mohamed Yassine Arkhis and D.Denis Efimov. Continuity-based robust stability condition of Lur'e systems.IEEE CDC 2024 - IEEE Conference on Decision and ControlMilan (Italie), ItalyDecember 2024HAL
  • 30 inproceedingsM.Mahugnon Dadjo, A.Alain Rapaport, J.Jérôme Harmand, R.Rosane Ushirobira and D.Denis Efimov. Minimal water consumption for a crop fertirrigation model.European Control Conference (ECC24)2024 European Control Conference, ECC 2024Stockholm, SwedenJuly 2024, 2380 - 2385HALDOIback to text
  • 31 inproceedingsD.Denis Efimov, M.Mata Khalili and S.Shiyu Liu. Global event-triggered regulation of unicycle dynamics by bounded control.IEEE CDC 2024 - IEEE Conference on Decision and ControlMilan, ItalyDecember 2024HAL
  • 32 inproceedingsD.Denis Efimov and Y.Yuri Orlov. On implicit discretization of prescribed-time differentiator.ECC 2024 - European Control Conference (ECC)Stockholm, SwedenJune 2024HAL
  • 33 inproceedingsD.Denis Efimov and Y. V.Yuri V Orlov. On implicit discretization of prescribed-time stabilizers.IEEE CDC 2024 - IEEE Conference on Decision and ControlMilan, ItalyDecember 2024HAL
  • 34 inproceedingsA.Ariana Gutiérrez, H.Hector Ríos, M.Manuel Mera, D.Denis Efimov and R.Rosane Ushirobira. An Event-Triggered Robust Control for Unicycle Mobile Robots with Communication, State, and Input Constraints.CDC 2024 - Conference on Decision and ControlMilan, ItalyDecember 2024HALback to text
  • 35 inproceedingsD. R.Danilo R Lima, R.Rosane Ushirobira and D.Denis Efimov. A new class of symmetry-preserving observers.63rd IEEE Conference on Decision and Control - CDC 2024MILAN, ItalyDecember 2024HAL
  • 36 inproceedingsD. R.Danilo R Lima, R.Rosane Ushirobira and D.Denis Efimov. A physics-informed neural network method to approximate homogeneous Lyapunov functions.European Control Conference (ECC24)Stockholm, SwedenJune 2024HALback to text
  • 37 inproceedingsD. R.Danilo R Lima, R.Rosane Ushirobira and D.Denis Efimov. State observer design for bilinear Persidskii systems.European Control Conference (ECC24)STOCKHOLM, SwedenJune 2024HALback to text
  • 38 inproceedingsM.Manuel Mera, H.Héctor Ríos and D.Denis Efimov. An Evader Controller for the Pursuit-Evasion Problem involving Single Integrator Dynamics.IEEE CDC 2024 - IEEE Conference on Decision and ControlMilan, ItalyDecember 2024HALback to text
  • 39 inproceedingsJ. Á.José Ángel Mercado Uribe, J.Jesús Mendoza-Avila, D.Denis Efimov and J.Johannes Schiffer. Sufficient Conditions for Global Boundedness of Solutions for Two Coupled Synchronverters.IEEE CDC 2024 - IEEE Conference on Decision and ControlMilan, ItalyDecember 2024HAL
  • 40 inproceedingsJ. A.José Antonio Ortega, D.Denis Efimov, A.Andrey Polyakov, J. A.Jaime A Moreno and L.Leonid Fridman. Stabilization of the Cart Pendulum using a Homogeneous Controller.Proc 17th Int. Workshop on Variable Structure Systems and Sliding Mode Control (VSS)Abu Dhab, United Arab EmiratesOctober 2024, 170 - 175HALDOI
  • 41 inproceedingsA.Andrey Polyakov and X.Xubin Ping. Homogeneous SMC Design Using Artificial Neural Network.17th International Workshop on Variable Structure Systems (VSS2024)Abu DHABI, United Arab EmiratesOctober 2024HAL
  • 42 inproceedingsH.Héctor Ríos, D.Denis Efimov and R.Rosane Ushirobira. Constant Parameter Identification: An Accelerated Heavy-Ball-based Approach.IEEE CDC 2024 - IEEE Conference on Decision and ControlMIlan, ItalyDecember 2024HAL
  • 43 inproceedingsR.Rosane Ushirobira, D.Denis Efimov, J. G.Jose Guadalupe Romero and R.Romeo Ortega. Estimation of non-separable regressions containing parameter dependent exponential functions.63rd IEEE Conference on Decision and Control - CDC 2024Milan, ItalyDecember 2024HAL
  • 44 inproceedingsJ.Junfeng Zhang, C.Christophe Combastel, D.Denis Efimov and A.Ali Zolghadri. Robust Stability Analysis for Continuous-Time Parameter-Varying Persidskii Systems.Proc. European Control Conference (ECC)Stockholm, SwedenJune 2024HAL
  • 45 inproceedingsK.Konstantin Zimenko, D.Denis Efimov, A.Andrey Polyakov and X.Xubin Ping. On Small-Gain Theorem for Interconnected Finite/Fixed-Time Input-to-State Stable Systems*.CDC 2024 - Conference on Decision and ControlMilan, ItalyDecember 2024HAL

Conferences without proceedings

  • 46 inproceedingsM.Mericel Ayamou, N.Nicolas Espitia, A.Andrey Polyakov and E.Emilia Fridman. Finite-dimensional homogeneous boundary control for a 1D reaction-diffusion equation.63rd IEEE Conference on Decision and Control (CDC 2024)Milan (Italie), FranceDecember 2024HAL
  • 47 inproceedingsA.Alexey Bobtsov, S.Stanislav Aranovskiy, D.Denis Efimov, A.Anton Pyrkin, V.Vladimir Vorobev and J.Jian Wang. Power noise filtration in DREM.2024 European Control Conference (ECC)Stockholm, SwedenIEEEJune 2024, 2259-2264HALDOI

Reports & preprints

  • 48 miscM. Y.Mohamed Yassine Arkhis and D.Denis Efimov. Open-loop control design for contraction in affine nonlinear systems.March 2025HAL
  • 49 miscM. Y.Mohamed Yassine Arkhis and D.Denis Efimov. Small-gain conditions for exponential incremental stability in feedback interconnections.March 2025HAL
  • 50 miscM.Marcel Fang, P.-A.Pierre-Alexandre Bliman, D.Denis Efimov and R.Rosane Ushirobira. Nonlinear adaptive observers for SIS system with primary infections.October 2024HALback to text
  • 51 miscA.Andrey Polyakov. Generalized Homogeneous Artificial Neural Network and Applications.July 2024HAL
  • 52 miscH.Hector Ríos, D.Denis Efimov and R.Rosane Ushirobira. A robust and accelerated heavy-ball-based algorithm for parameter identification.September 2024HAL
  • 53 miscH.Hemant Tyagi and D.Denis Efimov. Learning linear dynamical systems under convex constraints.January 2024HAL
  • 54 miscJ.Jian Wang, S.Stanislav Aranovskiy, R.Rosane Ushirobira and D.Denis Efimov. Regularization of the DREM procedure for parameter estimation under partial excitation.January 2025HAL
  • 55 miscJ.Jian Wanga, A.Alexey Bobtsov, S.Stanislav Aranovskiy, D.Denis Efimov and A.Anton Pyrkin. Power Series for Noise Attenuation in Linear Regression Parameter Estimation ⋆.May 2024HAL

12.3 Cited publications

  • 56 articleH.H. Ahmed, R.R. Ushirobira, D.D. Efimov, D.D. Tran and J.-C.J-C. Massabuau. Velocity estimation of valve movement in oysters for water quality surveillance.IFAC-PapersOnLine482015, 333--338back to text
  • 57 conferenceS.S. Aranovskiy, R.R. Ushirobira, D.D. Efimov and G.G. Casiez. Frequency Domain Forecasting Approach for Latency Reduction in Direct Human-Computer Interaction.Proc. 56th IEEE Conference on Decision and Control (CDC)Melbourne2017back to text
  • 58 articleE.Emmanuel Bernuau, D.Denis Efimov, W.Wilfrid Perruquetti and E.Emmanuel Moulay. Robust finite-time output feedback stabilization of the double integrator.International Journal of Control8832015, 451--460back to text
  • 59 articleE.E. Bernuau, D.D. Efimov, W.W. Perruquetti and A.A. Polyakov. On Homogeneity and Its Application in Sliding Mode.Int. J. Franklin Institute35142014, 1866--1901back to text
  • 60 articleE.E. Bernuau, A.A. Polyakov, D.D. Efimov and W.W. Perruquetti. Verification of ISS, iISS and IOSS properties applying weighted homogeneity.Systems & Control Letters622013, 1159--1167back to text
  • 61 articleD.Denis Efimov and W.Wilfrid Perruquetti. On conditions of oscillations and multi-homogeneity.Mathematics of Control, Signals, and Systems2812015, 1--37URL: http://dx.doi.org/10.1007/s00498-015-0157-yback to text
  • 62 articleD.D. Efimov, W.W. Perruquetti and J.-P.J.-P. Richard. Development of Homogeneity Concept For Time-Delay Systems.SIAM Journal on Optimization and Control5232014, 1403--1808back to text
  • 63 articleD.D. Efimov, A.A. Polyakov, A.A. Levant and W.W. Perruquetti. Realization and Discretization of Asymptotically Stable Homogeneous Systems.IEEE Trans. Automatic Control62112017, 5962--5969back to text
  • 64 articleD.D. Efimov, A.A. Polyakov, W.W. Perruquetti and J.-P.J.-P. Richard. Weighted Homogeneity for Time-Delay Systems: Finite-Time and Independent of Delay Stability.IEEE Trans. Automatic Control6112016, 210--215back to text
  • 65 articleN.N. Espitia, A.A. Polyakov, D.D. Efimov and W.W. Perruquetti. Boundary time-varying feedbacks for fixed-time stabilization of constant-parameter reaction-diffusion systems.Automatica1032019, 398--407URL: https://doi.org/10.1016/j.automatica.2019.02.013back to text
  • 66 articleM.M. Feingesicht, A.A. Polyakov, F.F. Kerhervé and J.-P.J.-P. Richard. SISO model-based control of separated flows: Sliding mode and optimal control approaches.International Journal of Robust and Nonlinear Control27182017, 5008-5027back to text
  • 67 conferenceE.E. Leurent, D.D. Efimov, T.T. Ra\"issi and W.W. Perruquetti. Interval Prediction for Continuous-Time Systems with Parametric Uncertainties.Proc. 58th IEEE Conference on Decision and Control (CDC)Nice2019back to text
  • 68 articleF.F. Lopez-Ramirez, A.A. Polyakov, D.D. Efimov and W.W. Perruquetti. Finite-time and Fixed-time Observer Design: Implicit Lyapunov function approach.Automatica8712018, 52-60back to text
  • 69 articleA.A. Polyakov, J.-M.J.-M. Coron and L.L. Rosier. On Homogeneous Finite-Time Control for Linear Evolution Equation in Hilbert Space.IEEE Transactions on Automatic Control2018back to text
  • 70 articleA.A. Polyakov, D.D. Efimov and B.B. Brogliato. Consistent Discretization of Finite-time and Fixed-time Stable Systems.SIAM Journal on Optimization and Control5712019, 78--103back to text
  • 71 articleA.A. Polyakov, D.D. Efimov, E.E. Fridman and W.W. Perruquetti. On Homogeneous Distributed Parameter Systems.IEEE Trans. Automatic Control61112016, 3657--3662back to text
  • 72 articleA.A. Polyakov, D.D. Efimov and W.W. Perruquetti. Finite-time and fixed-time stabilization: Implicit Lyapunov function approach.Automatica512015, 332-340back to text
  • 73 articleA.A. Polyakov. Nonlinear feedback design for fixed-time stabilization of linear control systems.IEEE Transactions on Automatic Control57(8)2012, 2106-2110back to text
  • 74 articleH.H. R\'ios, D.D. Efimov, J. A.J. A. Moreno, W.W. Perruquetti and J. G.J. G. Rueda-Escobedo. Time-Varying Parameter Identification Algorithms: Finite and Fixed-Time Convergence.IEEE Transactions on Automatic Control6272017, 3671--3678URL: https://dx.doi.org/10.1109/TAC.2017.2673413back to text
  • 75 conferenceR.R. Ushirobira, D.D. Efimov and P.-A.P-A. Bliman. Estimating the infection rate of a SIR epidemic model via differential elimination.Proceedings of ECCNaples2019back to text
  • 76 conferenceR.R. Ushirobira, D.D. Efimov, G.G. Casiez, N.N. Roussel and W.W. Perruquetti. A forecasting algorithm for latency compensation in indirect human-computer interactions.Proceedings of ECCAlborg2016, 1081--1086back to textback to text
  • 77 articleK.K. Zimenko, D.D. Efimov, A.A. Polyakov and W.W. Perruquetti. A note on delay robustness for homogeneous systems with negative degree.Automatica7952017, 178--184back to text
  1. 1The examples of interval prediction algorithm application can be consulted here.