Accessibility setting

Section: New Results

Waveguides, resonances, and scattering theory

An improved modal method in non uniform acoustic waveguides

Participant : Jean-François Mercier.

This topic is developed in collaboration with Agnès Maurel (Langevin Institute ESPCI).

We develop modal methods to study the scattering of an acoustic wave in a non uniform waveguide. Usual modal approaches are efficient only when a rather large number of evanescent modes are taken into account. An improved representation has been proposed in which an additional transverse mode and an additional unknown modal component are introduced. This so called boundary mode helps to better satisfy the Neumann boundary conditions at the varying walls. A system of coupled ordinary differential equations is obtained and is found to remain coupled in the straight part of the waveguide which implies that the classical radiation condition cannot be applied directly at the inlet/outlet of the scattering region.

We revisit the coupled mode equations in order to derive an improved system, in which the additional mode can be identified as evanescent mode, and then adapted to define radiation conditions. This makes possible the implementation of efficient numerical multimodal methods (like the admittance matrix method) and also approximate solutions can be found using the Born approximation. The numerical tests have shown that our method is very efficient to reduce the number of degree of freedom: adding to the boundary mode, it is sufficient to take only the propagative modes to get very good results. This is in particular interesting at low frequency when only the plane mode propagates. In the low frequency regime, the system can be solved analytically, using the Born approximation, leading to improved approximate equations compared to the usual Webster's approximation.

Construction of non scattering perturbations in a waveguide

Participants : Anne-Sophie Bonnet-Ben Dhia, Eric Lunéville.

This work is done in collaboration with Sergei Nazarov from Saint-Petersbourg University and during the internship of Yves Mbeutcha. We consider a two-dimensional homogeneous acoustic waveguide and we aim at designing deformations of the boundary which are invisible at a given frequency (or more generally at a finite number of given frequencies) in the sense that they are non scattering. To find such invisible perturbations, we take advantage of the fact that there are only a finite number of propagative modes at a given frequency in a waveguide. As a consequence, the invisibility is achieved by canceling a finite number of scattering coefficients, and an invisible deformation only produces an exponentially decreasing scattered field, not measurable in the far field.

The first step consists in studying the effect of a small deformation, of amplitude $\epsilon$. The asymptotic analysis allows to derive the first order terms of the scattering coefficients, as integrals involving the function describing the deformation. This leads to express the deformation as a linear combination of some explicit (compactly supported) functions, so that invisibility is satisfied if and only if the coefficients of the linear combination are solution of a fixed point equation. The key point is that we can prove, using the results of the asymptotic analysis, that the function of this fixed point equation is a contraction for $\epsilon$ small enough. This proves the existence of invisible deformations of amplitude $\epsilon$. Moreover, it provides a natural algorithm to compute the invisible deformation.

This has been tested numerically and the results are in perfect agreement with the theory. At low frequency, the good news is that $\epsilon$ can be taken quite large (the amplitude of the deformation may be half the size of the guide). But this deteriorates when the frequency increases.

Localized modes in unbounded perturbed periodic media

Participants : Patrick Joly, Sonia Fliss, Elizaveta Vasilevskaya.

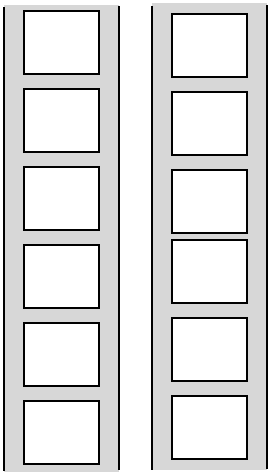
This topic is investigated in collaboration with Bérangère Delourme (Univ. Paris XIII) and constitutes the subject of the E. Vasilevskaya's PhD thesis. We are interested in a 2D propagation medium which is a localized perturbation of a reference homogeneous periodic reference medium. This reference medium is a "thick graph", namely a thin structure (the thinness being characterized by the parameter $\delta >0$) whose limit when $\delta$ tends to 0 is a periodic graph. This is for instance the case of the thick periodic ladder and the thick periodic rectangular grid of figure. The perturbation consists in changing only the geometry (and not the material properties) of the reference medium by modifying the thickness of one of the lines of the reference medium as illustrated by figure with the perturbed ladder and perturbed grid (see figure 3 ). The question we investigate is whether such a geometrical perturbation is able to produce localized eigenmodes (for the ladder) or guided modes (for the grid). We have investigated this question when the propagation model is the scalar Helmholtz equation with Neumann boundary conditions (in opposition to Dirichlet conditions that have been more studied in the literature - see the works by S. Nazarov for instance). This amounts to solving an eigenvalue problem for the Laplace operator in an unbounded domain : the associated self-adjoint operator has a continuous spectrum with a band gap structure and the eigenvalues are searched in the gaps.

With Neumann boundary conditions, we can use for the theoretical study an asymptotic analysis with respect to $\delta$: indeed, it is well known (see in particular the works by Exner, Kuchment, Post) the limit model when $\delta$ tends to 0, is the Helmholtz equation on the graph : 1D Helmholtz equations on each branch completed by continuity and Kirchoff transmission conditions at each node. The geometrical perturbation of the original medium results into a perturbation of the Kirchoff conditions on the nodes of the modified line. The spectral analysis of the limit problem can be done completely by hand and the existence of eigenmodes for the thick medium is ensured, for $\delta$ small enough, by the existence of corresponding eigenmodes for a limit "1D operator" whose spectrum appears to possess an infinity of band gaps in each of which eigenvalues can exist, due to the perturbation. Following this idea, we have been able to prove the existence of localized modes in the case of the ladder provided that the geometrical perturbation consists in diminishing the width of one rung. One can even prove that one can produce more and more localized modes, corresponding to larger and larger frequencies, when $\delta$ is smaller and smaller. On the contrary, we conjecture that there is no localized modes when we enlarge the rung. The extension of these results to the existence of guided modes in the case of the grid in progress.

For the numerical computation of such localized modes, we have adapted the DtN approach discussed in the activity report of 2012. We gave in figure 4 an example of computed localized mode in the case of the ladder : this mode is geometrically confined at the neighbourhood of the modified rung.