Section: New Results

Improving the flexibility of turbulence models for industrial applications

Figure 4. Left: Computation (Code_Saturne) of turbulent channel flow at 3 Reynolds numbers. Comparison with reference DNS of the results given by the EB-RSM integrated down to the wall (ItW, fine mesh) and the EB-RSM with analytical adaptive wall function (AAWF, 3 meshes). Right: EB-RSM computation (STARCCM+ code) of the wing-tip vortex generated by the flow around a NACA 0012 at 10 deg incidence. Visualisation of the streamlines colored with the streamwise vorticity.

In collaboration with industrial partners (EDF and CD-Adapco) developing CFD codes (code_Saturne and STARCCM+, respectively), we are working on the flexibility and robustness of the EB-RSM, an advanced Reynolds-stress turbulence model. Indeed, the two main problems that slow down the spreading of the use of such low-Reynolds number models (i.e., integrating the equations down to solid boundaries) in the industry are the impossiblity to control the near-wall mesh quality in the whole domain of a complex industrial application and the occurence of numerical instabilities due to spurious relaminarizations in some configurations.

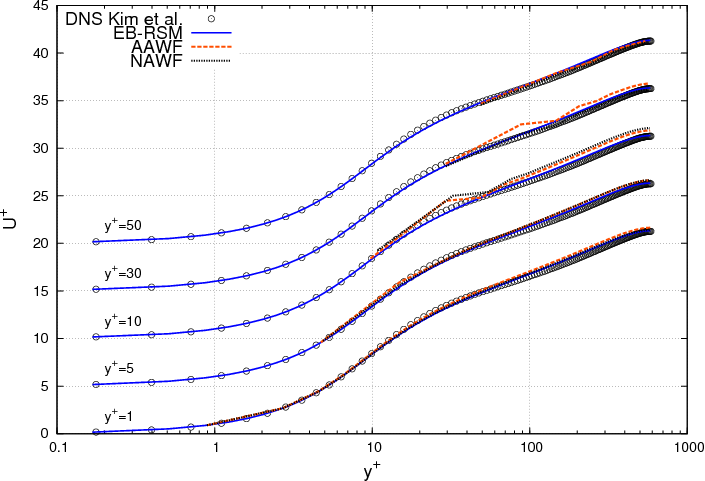
In order to address the first issue, we are working, in particular in the frame of the PhD thesis of J.-F. Wald, on the development of adaptive wall functions, i.e., non-homogeneous Dirichlet boundary conditions for the turbulent variables dependant on the size of the cell adjacent to the wall. These wall functions are based on the physical properties of turbulence in the different layers of the near-wall region (asymptotic behaviour in the viscous sublayer and log law in the equilibrium layer), such a way that the flow is correctly reproduced whatever the near-wall refinement of the mesh. Fig. 4 (left) shows that the reproduction of the mean velocity profile in turbulent channel flows obtained using a typical, industrial mesh ($y^{+}=50$) remains very close to the grid-converged solution.

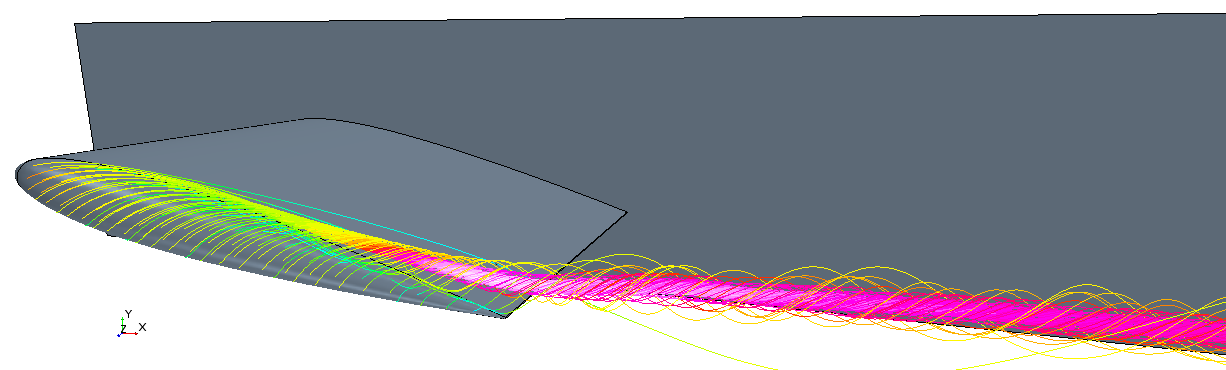
The second issue, the numerical instabilities due to local, spurious relaminarization of the model, can be addressed by investigating the solutions of the dynamical system formed by the model equations in homogeneous situations. Equilibrium solution are intersections of the nullclines (the locus of steady solutions for individual equations) and the stability properties of these fixed points can be visualized using trajectories in the phase space. By investigating the dependance of these stability properties on the parameters of the model, it is possible to eliminate undesired stable fixed points and thus to avoid the appearance of spurious laminarization. Fig. 4 (right) shows the fully turbulent solution obtained with the modified model in a case where the original model exhibited a severe, unphysical relaminarization of the wing-tip vortex.