Section: Research Program
Towards microwave quantum networks
The classical states of microwave radiation, are the so-called coherent states. They can be prepared by a commercial microwave generator (frequency ) followed by thermalization to using a chain of attenuators anchored at various stages of a dilution refrigerator.
Owing to the strength of its coupling to superconducting circuits [55] or Rydberg atoms [73] , microwave radiation can also be prepared in many possible non-classical states. Using a sequence of quanta exchanges between superconducting qubits and a microwave cavity, the direct preparation of an arbitrary superposition of Fock states has been demonstrated in 2009 [75] with about fidelity up to 5 photons. Recently, the physicists at Yale university in collaboration with the theorists of QUANTIC team, demonstrated a superposition of classical states, or Schrödinger cat, with 100 photons on average, using the dispersive coupling to a transmon qubit [122] .
An important class of states for quantum information processing with continuous variables is that of the Gaussian squeezed states [123] . These states can be seen as a coherent state for which the fluctuations on a quadrature are less than the zero point fluctuations. Of course, owing to Heisenberg uncertainty principle, this comes at the expense of larger fluctuations on the conjugated quadrature. In the optical domain, Gaussian light has been demonstrated and used with single and multimodes decades ago [123] . In the microwave domain, single mode squeezing of thermal noise had been demonstrated already in 1988 [128] but vacuum noise squeezing was only demonstrated in 2008 [53] . Since then, several groups have been able to generate single- and two-mode squeezing of microwave radiation, including us [59] , [125] , [91] , [95] , [61] . The two-mode squeezed states are of particular interest for quantum information processing, because they are maximally entangled for a given average number of quanta. In particular, the circuit developed by QUANTIC's experimentalists is able to directly generate two-mode squeezed states on separate transmission lines, at arbitrarily different frequencies [61] .
In the perspective of a quantum network using microwave radiation, one needs a way to store and preserve microwave fields in nodes. Arguably, creating a memory for quantum systems able to preserve indefinitely a quantum state is the next big challenge on the road towards quantum computing [56] , yet unrealized in any system. In a first step, we focus on a quantum node able to preserve a quantum state for a finite time.
In the optical domain, current implementations of quantum memories [114] rely mainly on two physical effects: the light deceleration due to electromagnetically induced transparency and the transfer of photonic quantum states onto collective atomic coherences (optical or spin). In the microwave domain, several quantum memories have emerged in the last years using spin ensembles [126] , [80] , [109] , mechanical resonators [98] , [99] or superconducting circuits [127] , [124] , such as our device described in [62] .
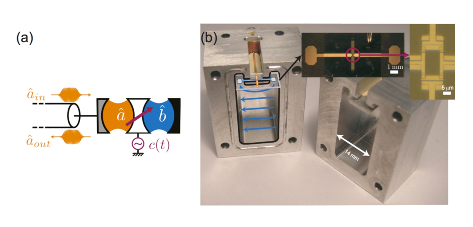
All these microwave implementations have pros and cons. However, only two of them, the mechanical oscillator of the Lehnert group [99] and our device [62] have demonstrated entanglement between the memory and a propagating microwave mode. Specifically, our device consists in a 3D storage microwave cavity whose coupling to a transmission line is performed using an active superconducting circuit: the Josephson ring modulator. In the frequency conversion regime, it acts as a tunable coupler whose rate is solely controlled by the amplitude of a pump signal. In the parametric down-conversion regime, it acts as an entanglement generator, similarly to the mechanical version of the Boulder group. However, the inherently small coupling rate between the transmission line and the mechanical resonator in [99] makes our device [62] a much stronger candidate for a quantum node. Apart from this crucial possibility to generate entanglement, our device is similar to the implementation of Santa Barbara [127] . Both have demonstrated fast tuning (up to 30 MHz for Santa Barbara) with high catching efficiency and storage time of 4 s. However we believe that two specificities make our route more promising. In their case it is a flux knob which allows tuning of the transparency of a 2D microwave cavity. The core of the device we propose is a 3D storage microwave, an architecture where there is plenty of room to improve the storage time and exceed this figure by orders of magnitude, even without quantum error correction [104] . Moreover the cavity transparency is controlled solely by the amplitude of a microwave tone, free of the complications of hysteresis inherent to fast flux tuning in a superconducting environment.
The quantum information protocols one can envision using the quantum node developed by QUANTIC's experimentalists gets a useful inspiration from what has been realized in the optical domain in the last 20 years. One of the most interesting protocols we would like to implement is the teleportation of a quantum state from the memory into a transmission line or another memory. In optics, this was performed already in 1998 for a coherent state [63] , and more recently for a Schrödinger-cat-like state [82] . We could readily reproduce these experiments in the microwave regime. The deterministic teleportation of a superconducting quantum bit was realized only in 2013 [118] but no experiments have shown teleportation of a continuous variable state in the microwave domain up to now. Furthermore, none of the protocols needed for quantum information processing (entanglement distillation and dilution for instance) have ever been realized in the microwave domain with Gaussian states [123] . It is thus of great interest to investigate where the tools specific to superconducting circuits will allow us to go beyond what can be done in the optical domain. In particular, the microwave quantum limited amplifiers [107] developed by QUANTIC's experimentalists lead to unmatched heterodyne measurement efficiencies. Finally using a qubit as a Fock number resolved photocounter unleashes many scenarios in the preparation and manipulation by measurement of an entangled state.
|