Section: Research Program

Design of complex models

Project team positioning

The originality of our work is the quantitative description of phenomena accounting for several time and spatial scales. Here, propagation has to be understood in a broad sense. This includes propagation of invasive species, chemotactic waves of bacteira, evoluation of age structures populations ... Our main objectives are the quantitative calculation of macroscopic quantities as the rate of propagation, and microscopic distributions at the edge and the back of the front. These are essential features of propagation which are intimately linked in the long time dynamics.

Recent results

• Population models.

  H. Leman works at the interface between mathematics and biology, thanks to probabilist and determinist studies of models of populations. More precisely, she studies and develops probabilistic models, called agent models that described the population at an individual level. Each individual is characterized by one or more phenotypic traits and by its position, which may influence at the same time its ecological behavior and its motion. From a biological point of view these models are particularly interesting since they allow to include a large variety of interactions between individuals. These processes may also be studied in details to obtain theoretical results which may be simulated thanks to exact algorithms. To get quantitative results H. Leman uses changes of scales in space and time (large population, rare mutations, long time), following various biological assumptions.

  In a first study , H. Leman tries to understand the interactions between sexual preference mechanisms and evolutive forces inside spatially structured populations. Recently she got interesting in the description of necessary conditions to facilitate the emergence of such preferences by individuals.

  As a second example, H. Leman is also interested in the modeling and study of cooperative bacterias and tries to understand the impact of spatial structures in the eco - evolutions of these bacterias. Space seems to be an essential factor to facilitate the emergence of cooperation between bacterias.

  Finally, H. Leman studied the large time behavior of continuous state branching processes with competition and Lévy environment. These kind of stochastic processes are used to represent the fluctuations of the size of a population. In particular, she studied the extinction time of such a process.

• Inviscid limit of Navier Stokes equations.

  The question of the behavior of solutions of Navier Stokes equations in a bounded domain as the viscosity goes to 0 is a classical and highly difficult open question in Fluid Mechanics. A small boundary layer, called Prandtl layer, appears near the boundary, which turns out to be unstable if the viscosity is small enough. The stability analysis of this boundary layer is highly technical and remained open since the first formal analysis in the 1940's by physicists like Orr, Sommerfeld, Tollmien, Schlichting or Lin. E. Grenier recently made a complete mathematical analysis of this spectral problem, in collaboration with T. Nguyen and Y. Guo. We rigorously proved that any shear layer is spectrally and linearly unstable if the viscosity is small enough, which is the first mathematical result in that field. We also get some preliminary nonlinear results. A book on this subject is in preparation, already accepted by Springer.

• Numerical analysis of complex fluids: the example of avalanches.

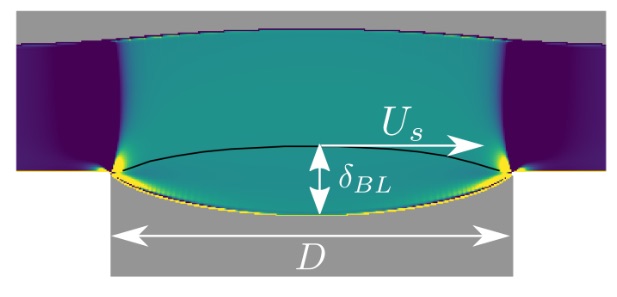
  This deals with the development of numerical schemes for viscoplastic materials (namely with Bingham or Herschel-Bulkley laws). Recently, with other colleagues, Paul Vigneaux finished the design of the first 2D well-balanced finite volume scheme for a shallow viscoplastic model. It is illustrated on the famous Taconnaz avalanche path in the Mont-Blanc (see figure 1), Chamonix, in the case of dense snow avalanches. The scheme deals with general Digital Elevation Model (DEM) topographies, wet/dry fronts and is designed to compute precisely the stopping state of avalanches, a crucial point of viscoplastic flows which are able to rigidify [cf joint Figure and Fernandez-Nieto et al. JCP 2018]. Currently, through a collaboration with IRSTEA Grenoble, we also revisit the theory of viscoplastic boundary layers (see figure (2) by extending the Oldroyd's asymptotic scaling (1947) to the cases of moderate Bingham numbers (or Herschel-Bulkley numbers). Also with IRSTEA, we are developping a joint study (numerical and experimental) of viscoplastic avalanches in the lab, to challenge various yield stress models.

  Figure 1. An example of avalanche simulation

  Figure 2. An example of boundary layer for complex flows

Collaborations

• Ecology: Orsay (C. Coron), Toulouse (IMT, M. Costa), MNHM Paris (V. Llaurens), LISC Paris (C. Smadi), ENS Paris (R. Ferrière, E. Abs), CIMAT (Mexique, J. C. P. Millan).

• Inviscid limit of Navier Stokes equations: Brown University (Y. Guo, B. Pausader), Penn State University (T. Nguyen), Orsay University (F. Rousset).

• Numerical analysis of complex fluids: Enrique D. Fernandez - Nieto (Univ. de Sevilla, Spain), Jose Maria Gallardo (Univ. de Malaga, Spain).

• Comparison between numerical simulations and physical experiments for the dam-break of viscoplastic materials: collaboration with IRSTEA (now INRAE, since Jan. 2020).