2023Activity reportProject-TeamMAKUTU
RNSR: 202123948U- Research center Inria Centre at the University of Bordeaux
- In partnership with:Institut Polytechnique de Bordeaux, Université de Pau et des Pays de l'Adour, CNRS, TotalEnergies
- Team name: Experimental-based modeling and simulation of wave propagation to characterize geophysical and heliophysical media and to design complex objects
- In collaboration with:Laboratoire de mathématiques et de leurs applications (LMAP)
- Domain:Digital Health, Biology and Earth
- Theme:Earth, Environmental and Energy Sciences
Keywords
Computer Science and Digital Science
- A3.4.6. Neural networks
- A6.1. Methods in mathematical modeling
- A6.1.1. Continuous Modeling (PDE, ODE)
- A6.1.4. Multiscale modeling
- A6.1.5. Multiphysics modeling
- A6.2. Scientific computing, Numerical Analysis & Optimization
- A6.2.1. Numerical analysis of PDE and ODE
- A6.2.7. High performance computing
- A6.3.1. Inverse problems
- A6.3.4. Model reduction
- A6.5. Mathematical modeling for physical sciences
- A6.5.1. Solid mechanics
- A6.5.4. Waves
Other Research Topics and Application Domains
- B3. Environment and planet
- B3.3. Geosciences
- B3.3.1. Earth and subsoil
- B4. Energy
- B9.2.1. Music, sound
- B9.5.2. Mathematics
- B9.5.3. Physics
- B9.5.5. Mechanics
1 Team members, visitors, external collaborators
Research Scientists
- Helene Barucq [Team leader, INRIA, Senior Researcher, HDR]
- Henri Calandra [TotalEnergies, Industrial member]
- Juliette Chabassier [INRIA, Researcher]
- Julien Diaz [INRIA, Senior Researcher, HDR]
- Augustin Ernoult [INRIA, Researcher]
- Florian Faucher [INRIA, Researcher]
- Stefano Frambati [TotalEnergies, Industrial member]
- Ha Ngoc Pham Howard Faucher [INRIA, Researcher]
- Xavier Lacoste [TotalEnergies, Industrial member, until Jul 2023, part-time]
- Jie Meng [TotalEnergies, Industrial member, 75% of her time]
- Rami Nammour [TotalEnergies, Industrial member, from Jun 2023, 40% of his time]
Faculty Members
- Marc Durufle [BORDEAUX INP, Associate Professor]
- Sebastien Tordeux [UPPA, Associate Professor, HDR]
Post-Doctoral Fellows
- Thibaud Beltzung [INRIA, Post-Doctoral Fellow, from Apr 2023]
- Chengyi Shen [INRIA, Post-Doctoral Fellow]
PhD Students
- Corto Bastien [INRIA, from Nov 2023]
- Julien Besset [INRIA]
- Guillaume Castera [INRIA]
- Lola Chabat [UPPA]
- Marine Deheuvels [UPPA, until Sep 2023]
- Ibrahima Djiba [INRIA]
- Arjeta Heta [UPPA]
- Augustin Leclerc [INSA ROUEN NORMANDIE]
- Matthias Rivet [Institut polytechnique de Paris ]
- Manon Sarrouilhe [INRIA, from Nov 2023]
- Alexis Thibault [UPPA, until Aug 2023]
- Nicolas Victorion [INRIA]
Technical Staff
- Aurélien Citrain [INRIA, Engineer]
- Paloma Martinez [INRIA, Engineer]
Interns and Apprentices
- Andreas Autin [INRIA, Intern, from Apr 2023 until Sep 2023]
- Marie Compain [UNIV NANTES, Intern, from Jun 2023 until Jul 2023]
- Florian Delprat [INRIA, Intern, from May 2023 until Aug 2023]
- Anaelle Fresneau [INRIA, Intern, from Mar 2023 until Aug 2023]
- Louise Sophie Hourcade [INRIA, Intern, from May 2023 until Sep 2023]
- Israa Ibrahim [INRIA, Intern, from Mar 2023 until Aug 2023]
- Andrea Lagardere [INRIA, Intern, from Oct 2023]
- Andrea Lagardere [UPPA, Intern, from Mar 2023 until Aug 2023]
Administrative Assistants
- Fabienne Cuyollaa [INRIA, from Sep 2023]
- Sylvie Embolla [INRIA, until Sep 2023]
Visiting Scientist
- Rabia Djellouli [California State University at Northridge, from Aug 2023]
External Collaborators
- Daniel Brito [Université de Pau et des Pays de l'Adour]
- Damien Fournier [Max Planck Institute]
- Laurent Gizon [Max Planck Institute]
- Christian Gout [Insa Rouen, HDR]
- Ronan Madec [CS Group, from Sep 2023, part-time]
- Christina Morency [Lawrence Livermore National Laboratory]
- Mamadou N'diaye [Université Polytechnique des Hauts de France]
2 Overall objectives
Imagine trying to describe a place with exactness from more or less numerous and precise memories, or guessing the content and internal structures of an object after having observed it only partially, without ever touching it because it is inaccessible or very fragile? These are the objectives of Makutu team, where recordings of reflected waves correspond to the memories. Waves can be seismic, electromagnetic or acoustic and Makutu focuses its research on the characterization of Earth's subsurface, of the Sun, and of musical instruments. An important component of Makutu's work is the improvement of the resolution methods for direct problems, in order to simulate the propagation of waves in complex media. The characterization and reconstruction of objects using non-invasive approaches then need the resolution of an inverse problem, with efficient forward modeling at the center.
Makutu is an industrial Inria project-team joint with TotalEnergies, in partnership with University of Pau and Pays de l'Adour, Institut Polytechnique de Bordeaux and CNRS. The team is bi-located, one part is hosted by UPPA and the other is hosted by Inria on the University of Bordeaux campus. It is a follow-up of Magique-3D (Advanced Modeling in 3D Geophysics) and its research topics have expanded are summarized as “Experimental-based modeling and simulation of wave propagation to characterize geophysical and heliophysical media and to design complex objects" with the new name Makutu (magicians in Maori).
The numerical simulation of waves propagating in complex media (the direct problems solved by Makutu) requires the development of advanced numerical methods but the research does not stop there. Indeed, to probe a medium or reconstruct an object from reflected waves measurements (the inverse problems addressed by Makutu), it is important to accurately solve systems of partial differential equations that model all the waves that can be measured. As the complexity of the physical models goes hand in hand with the complexity of the calculations, Makutu is particularly committed in the development and analysis of appropriate mathematical models as well as in the design and study of advanced numerical methods taking into account the characteristics of the physics considered.
Makutu's research has many facets, with final goals ranging from the development of open-source prototype codes written to assess new ideas, to software packages to be ported to an industrial environment. Makutu's research activities are inspired by a strong interdisciplinary industrial and academic partnership. The team's contributions are at the interface of applied analysis, numerical analysis, and scientific computing. The size of some of our problems projects us into the High Performance Computing (HPC) environment and dictates the choices we make for certain approximation spaces that are conducive to massive parallelism.
Makutu has important contributions in the field of high-order discretization methods along with high-order time schemes. Whatever the application is, numerical schemes are all designed with a view to reduce computational time or limit memory consumption, while maintaining a high level of accuracy. Sometimes, it is also necessary to work on the mathematical models themselves whose brute complexity can be a source of difficulty or even blockage for the numerical simulations. One of the originality of the team is to collaborate with experimenters to compare measurements and numerical data in order to calibrate the models. All these contributions are significant steps to reach the final team's objectives which are expressed as the resolution of complex inverse problems. Large-scale computing is then an important part of our activity, which we carry out taking into account the three pillars that make up HPC, i.e. computing time, storage and precision. Recently, the team has started to work on the use of machine learning to assist the numerical schemes they develop in order to control the numerical pollution (or dispersion) which becomes very strong in large-scale computations. This is particularly the case in geophysics where several hundred wavelengths can be propagated. It is worth noting that we take particular care in developing our numerical methods so that they can be used for a wide range of applications, whether the calculations are done in an HPC environment or on a simple laptop.
3 Research program
Makutu's research program decomposes itself into four axes that are: (1) Methodological contributions to the simulation of mechanical and electromagnetic waves in complex media; (2) Seismic imaging; (3) Helioseismology; (4) Musical Acoustics. Each axis shares the same objective to realize simulations of real phénomena. To achieve this, one needs real data and advanced mathematical models and high-order numerical schemes that are compatible with high-performance computing architectures.
To obtain real data, in addition to its current collaborations with scientists both from Academia and Industry, Makutu is developing a new branch of research activities by carrying out its own laboratory measurements. For instance, in order to take into account porosity, parameters such as viscosity, attenuation, thermodynamic effects, etc., must be integrated, and their impact must be properly analyzed before considering using them to characterize the propagation media. This constitutes a clear step ahead for Makutu, and opens up new prospects of contributing to the characterization of very complex media based on wave field measurements.
Regarding the development of numerical schemes, Makutu is developing high-order Discontinuous Galerkin (DG) methods and high-order time schemes. The coupling of DG methods with other techniques of discretization is also under consideration. Trefftz-DG and Hybridizable DG methods have been developed both for poro-elastic waves and electromagnetic waves. HDG and HDG+ formulations are also under study for helioseismology.
The research activities of members of Makutu share a common theme of using numerically computed wavefield measurements to reconstruct the propagation medium they passed through before recording. The medium can be reconstructed by identifying either the physical parameters or the geometrical parameters that characterize it. In each case, the next step is to solve an inverse problem that is non-linear and ill-posed. To solve it, Makutu is focusing on the Full Waveform Inversion (FWI), which is a high-definition imaging method widely used in the field of geophysics.
4 Application domains
Makutu research program is organized around three principal domains of applications: geophysical exploration, solar imaging, and music. Each of them requires a relevant panel of significant contributions requiring achievements in laboratory measurements, modeling, mathematical analysis, advanced numerical schemes and massively parallel software development. Recently, the team has added experimental contributions to feed simulations with real data and also improve modeling through better calibration. Makutu's application domains can be regrouped into a long-standing activity dedicated to subsurface imaging, and two more recent activities dedicated to solar imaging and the development of numerical wind instruments. Each field of application is not compartmentalized in the methodological sense of the term: equations, numerical schemes and programming practices are shared and possibly adapted to the underlying application.
4.1 Geophysical exploration
Geophysical exploration is a historical field for the team (see e.g 50, 54, 55, 57.Geophysical exploration has been driven for a very long time by the goal of finding hydrocarbons. Today, it is evolving towards a very proactive direction in favor of renewable energies and Makutu commits part of its research activities in this direction, in the framework of industrial and international collaborations. Industrial partnership with TotalEnergies has evolved to the transformation of Makutu into an industrial project-team since January 2022. The dedicated research project targets monitoring of CO2 storage through the development of a new numerical branch in GEOSX for seismic propagation and inversion. As far as geothermical energy is concerned, Makutu is member of the international project SEE4GEO lead by C. Morency from Lawrence Livermore National Laboratory. The project combines experimental research in the field and in laboratory with numerical developements in the continuity of CHICkPEA project previously funded by UPPA (2018-2021).
Inversion is central for geophysical exploration and Makutu focuses on Full Waveform Inversion (FWI) as a high-fidelity solution methodology for reconstructing the physical parameters from observed data. FWI can be carried out in time-domain 53, 72, 82, 83 or in frequency domain 78, 77, 76. Its main feature is to avoid the formation of the large Jacobian matrix by computing the gradient of the misfit functional using the adjoint-state method 58. A detailed review of FWI for geophysical applications can be found in 75.
4.1.1 Deep geothermal energy
Obtaining accurate images of natural reservoirs is critical for their management and exploitation and seismic imaging is an efficient tool (see 71, 70 and their references therein. One example is with deep geothermal energy which requires precise imaging of deep fractured reservoirs filled with geothermal fluids. Standard seismic imaging is based upon inverting mechanical waves which have difficulties to detect them, whereas electromagnetic waves are more sensitive. We see here a clear interest of coupling seismic with electromagnetic methods and this is what Makutu began developing with CHICkPEA project ended in 2021. The team is now involved in project SEE4GEO funded by ADEME, in the framework of Geothermica call.
4.1.2 CO2 injection monitoring
The reduction of greenhouse gases in the atmosphere is a societal topic of the utmost importance, with the Paris Agreement setting ambitious goals for many countries. One fundamental pillar of greenhouse emission management is Carbon Capture Utilisation and Storage (CCUS) 84. With this strategy, carbon dioxide produced on- or off-site is sequestered and injected into depleted reservoirs, thus offsetting an important portion of current CO2 emissions. The successful and safe implementation of this strategy requires the prediction, monitoring and surveillance of stored CO2 over long periods, which presents significant challenges in terms of seismic acquisition, seismic inversion and numerical simulation. These tools, coupled with state-of-the-art flow simulations, are vital in order to support the injection operations with vital real-time and long-term information. Moreover, specific challenges related to the physics of injected CO2, such as viscosity, temperature and multi-phase fluid conditions push to the limits our current numerical models, and require ambitious new multi-physics simulations to support safe and cost-effective CO2 injection operations. For example, some recent publications like 81, 87 have shown that the combination of CO2-brine flow with wave propagation provides efficient simulations for the monitoring of sequestered CO2. Makutu is currently developing numerical methods for this new application, in collaboration with TotalEnergies, as a new computational branch of the open-source multiphysics simulator GEOSX.
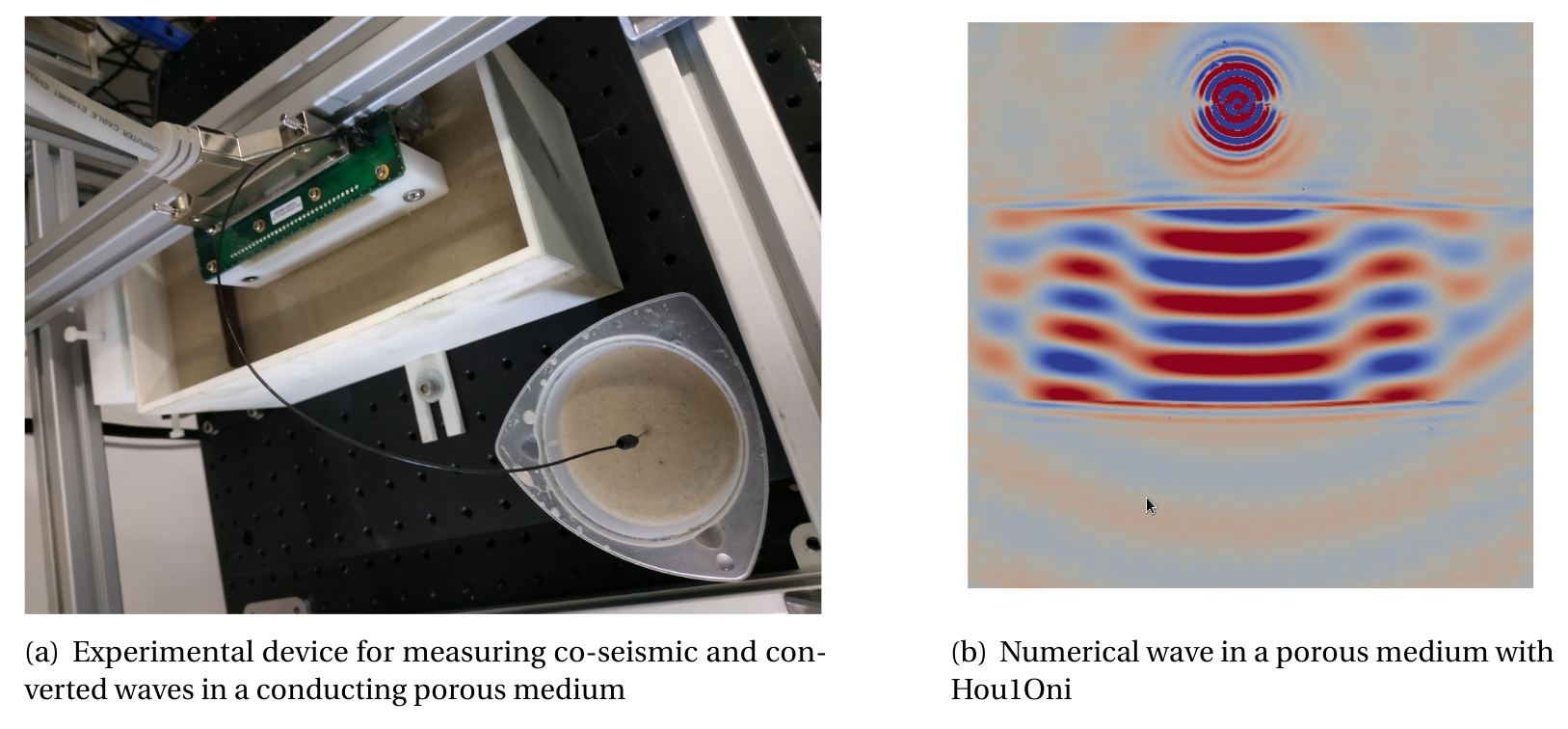
Experimental device and numerical simulation of waves in porous media, CHICkPEA, E2S
4.2 Solar imaging
Helioseismology studies the interior and dynamics of the Sun based on the observation of wave oscillation in the solar photosphere. These movements can be observed at the surface by the Dopplergrams given by ground-based or satellite-borne observatories. In recent years, methods for understanding Earth subsurfaces have opened up new ways to study the interior of the Sun as in the case with helioseismology and the interior of stars with aesteroseismology from oscillation observed at their surface. Techniques in helioseisomolgy is generally divided into global and local helioseismology. The first approach studies frequencies of oscillations modes, cf. 59. On the other hand, local helioseimology, which adapts techniques of geophysical seismic interferometry studies, measures local wave propagation and works with the full 3D observed wavefield, and is thus more adapted to study additional features such large-scale flows in active region, sun spots and plage, cf. 68, 67.
Makutu extends its activity on terrestrial seismology to studying the Sun, for the latter offers a vast wealth of problems to be explored both for direct modeling as well as inversion. The collaboration between Makutu and the solar group at the Max Planck institute for Solar research MPS at MPS brings together the expertise of MPS in solar physics and seismology and that of Makutu in numerical simulation of wave propagation and large-scale inversion in geophysics. This ongoing collaboration dating from 2016 obtains its official mark with the creation of associated team ANTS which started in 2019 and ended in 2022. The main goal of the collaboration is the creation of a computational framework for accurate and efficient simulation of solar oscillation to be used in full wave-form inversion, e.g. for 3D solar flow.
The stochastic nature of solar oscillation is described by random right-hand source term, and in using statistical analysis, under appropriate assumptions (e.g. the convenient source assumption), power spectrums and time-distance diagrams can obtained from the deterministic Green kernel of modeling wave equation, cf. 66. In this way, the Green kernel becomes a crucial object in local helioseismology, and its accurate and efficient computation is the main goal of forward modeling. In addition to appropriate numerical schemes, investigation of radiation boundary conditions is required in order to describe accurately waves above cut-off frequencies.
Up until 2021, the focus has been put on acoustic waves which are identified with p-ridges in observed solar power spectrum. Acoustic waves at low frequencies can be adequately described by a scalar equation. The thesis of Rouxelin 2021 studied convected Helmholtz which allows for a simplified description of the effect of convection on acoustic waves. Recent and ongoing works extend the investigation to vector wave equation to include gravity and differential rotation. The latter is particularly of interest due to the recent discovery of inertial waves in the Sun. This is subject of the thesis of Chatbat which starts in October 2022, and the goal of which is to create an in-house software to compare accurately eigenvalues for the solar wave equation with differential rotation. The remaining challenge is to include full 3d flow to the vector equation, which is the goal for the next year.
The above works lay the necessary foundation for inversion of solar parameters such as flow and active region sound speed. Current state-of-the art tools in these references is linear inversion using Born approximation 64. In additional they are carried out in 1D or 2D. It is thus interesting to apply nonlinear inversion such as Full Waveform Inversion in 3D cf. 63 to these problems.
4.3 Musical acoustics
This field of application is a subject of study for which the team is willing to take risks. We propose using a mix of experimental and numerical approach in order to study and design musical instruments. Makers have designed wind musical instruments (as flutes, trumpets, clarinets, bassoons...) in the past through “trial and error” procedures by performing a geometrical calibration of musical instruments in order to improve their accuracy, tone, homogeneity and even their sound volume, ergonomics, and robustness. During the past few decades, musical acoustics has been in a process of rationalizing the empiric understanding of instrument makers in order to formulate a scientific approach to future evolution. Our research proposal is along this axis of research by proposing new mathematical models based on our solid experience in terms of wave propagation in media with interfaces that can significantly change the sound. As was done in geophysical exploration, we propose to assist the modelling process with laboratory experiments. Direct comparison between simulations and experiments will allow to assess the model error. For this purpose, an experimental device has been developed in collaboration with I2M, Mechanics Laboratory of the University of Bordeaux and Humeau Factory, Montpon- Ménestérol, and is currently in use.
4.3.1 Modeling
Although the playing context should always be the final reference, some aspects of the behavior of a wind instrument can be firstly characterized by its entry impedance which quantifies the Dirichlet-to-Neumann map of the wave propagation in the pipe in the harmonic domain. This impedance can be both measured 65, 60 and computed with simulations based on accurate and concise models of the pipe 80, 5685, 61. A more realistic approach accounts for the embouchure 69, 56, 51, 52, 86, which is modeled as a nonlinear oscillator coupled with the pressure and acoustic velocity at the entry of the pipe, allowing to predict the sound qualities. Mathematical properties of the underlying models are not yet totally understood, and adequate models still need to be developed. This is particularly true when accounting for dissipation phenomena, junctions of pipes, pipe porosity and rugosity, embouchures...
To reproduce the sound of instruments, time-dependent models are more suitable. Here, nonlinear lumped elements induce an “auto-oscillatory” behavior of the instrument. The models currently available in the literature are meant to reproduce viscothermal effects, pipe junctions, pipe radiation, lips oscillation, etc. They do not necessarily possess adequate mathematical properties to ensure stable simulations and they should be improved using asymptotic analysis methods or Lagrangian formalism.
4.3.2 Numerical methods
As far as numerical developments are concerned, the accuracy of the calculations is essential. Indeed, for some aspects like the sounding frequency, a deviation of 1% between the predictions and the observations is unacceptable. Moreover, contrary to what the team is used to do for geophysics or astrophysics thanks to HPC, numerical methods for acoustical musics must be frugal to be run on personal computers by acousticians and makers. Makutu has a wide range of numerical methods that have been implemented in its codes for linear problems. New numerical schemes will have to be implemented to take into account the non-linearities of time-dependent models.
4.3.3 Virtual workshop
Beyond the idea of mathematically modeling musical instruments, Makutu wishes to develop a virtual workshop whose vocation will be twofold: (i) support the manufacturers to design new instruments; (ii) recreate the sound of old and historical instruments. To implement this idea, we propose to elaborate optimization techniques that are well-known in the team to define optimal geometries to meet given specifications. This can be used to reconstruct existing instruments from acoustic measurement or to design new instruments by fixing relevant quantitative objective which is a research activity by its own 62. Behind the idea of the virtual workshop is also the intention to hear the instruments, from the knowledge of their shape and playing regime. For that purpose, time-domain models are essential.
5 Social and environmental responsibility
Makutu recognizes the importance of conducting research in a responsible and sustainable way. We are committed to ensuring that our work has a positive impact on society and the environment.
In terms of social responsibility, Makutu members ensure that their research is inclusive and accessible to all members of society. The team prides itself on bringing together researchers from diverse social and cultural backgrounds. It makes its results and publications available to the general public and is involved in scientific dissemination activities.
In terms of environmental responsibility, Makutu strives to minimize the environmental impact of its research. Wherever possible, the team works to reduce its carbon footprint by implementing environmentally friendly practices and maintaining remote collaborations to limit international travel. It is also engaged in a research program dedicated to sustainable energy. In particular, it is contributing to the development of advanced software for monitoring CO2 storage and is studying complex models that can assist in the development of geothermal drilling by avoiding the devastating creation of micro-earthquakes.
Overall, Makutu is committed to conducting research in a responsible and sustainable manner and is committed to having a positive impact on society and the environment.
6 Highlights of the year
6.1 Incorwave: ERC research project
Florian Faucher has been awarded an ERC Starting Grant for the project Incorwave: Nonlinear inversion of correlation waveforms with hierarchical reconstructions. The project proposes to create new mathematical and computational framework for nonlinear inversion in terrestrial and extra-terrestrial seismology. Two specific problems are considered: first, for the reconstruction of visco-elasticity properties with applications to Earth's subsurface monitoring; secondly, for the reconstruction of three-dimensional flows in the Sun to characterize the properties of deep solar convection. The ERC Starting Grant Incorwave is a five-year project that starts in 2024.
6.2 Makutu@Numpex
Numpex (french acronysm of Digital for exascale) has been launched in 2023 as one of the national Priority Research Programs and Equipment to participate in meeting the challenges of exascale. Numpex is composed of 5 focused projects and Makutu is mainly involved in Exa-MA (Methods and Algorithms for Exascale).
6.3 Butterfly project
Butterfly is a bilateral research project with Göttingen University supported by ANR and DFG (German Research Foundation). It is a follow up of the associate team ANTS which ended in 2022. Its objective is to combine expertise in applied mathematics (Makutu) and helioseismology (U. Göttingen) to develop the computational tools required to learn about the surface distribution of magnetic activity on the surfaces of distant Sun-like stars using asteroseismology.
7 New software, platforms, open data
7.1 New software
7.1.1 OpenWind
-
Name:
Open Wind Instrument Design
-
Keywords:
Wave propagation, Inverse problem, Experimental mechanics, Time Domain, Physical simulation
-
Scientific Description:
Implementation of first order finite elements for wind musical instrument simulation. Implementation of the Full Waveform inversion method for wind musical instrument inversion. Implementation of energy consistent numerical schemes for time domain simulation of reed-type wind musical instrument.
-
Functional Description:
Simulation and inversion of wind musical instruments using one-dimensional finite element method with tonholes or valves and fingering chart. The software has three functionnalities. First, the software takes the shape of a wind instrument and computes the acoustical response (answer to a given frequential excitation). Second, the software takes the instrument shape and the control parameters of a musician, and computes the produced sound and the time evolution of many acoustical quantities. Last, the software takes a measured acoustical response and computes the corresponding instrument geometry (inner bore and tone holes parameters).
-
Release Contributions:
- Time domain and frequency domain simulations of flute-like instruments (sound synthesis and impedance computation) - Scripts used for generating figures for Alexis Thibault Thesis - Roughness parametric model - New scheme for Reed/lips model with dimensionless parameters - Effect of air composition (humidity and CO2 rates) on the acoustic propagation - Possibility to impose geometric constraints (linear and non-linear) during optimization process
- URL:
- Publications:
-
Contact:
Augustin Ernoult
-
Participants:
Juliette Chabassier, Augustin Ernoult, Alexis Thibault, Robin Tournemenne, Olivier Geber, Guillaume Castera, Tobias Van Baarsel
7.1.2 Hou10ni
-
Keywords:
2D, 3D, Elastodynamic equations, Acoustic equation, Elastoacoustic, Frequency Domain, Time Domain, Discontinuous Galerkin
-
Scientific Description:
Hou10ni simulates acoustic and elastic wave propagation in time domain and in harmonic domain, in 2D and in 3D. It is also able to model elasto acoustic coupling. The time domain solver is based on the second order formulation of the wave equation and the space discretization is achieved using Interior Penalty Discontinuous Galerkin (IPDG) Method. Both IPDG and Hybridizable Discontinuous Galerkin (HDG) Methods are implemented in the frequency domain solver. Recently, the HDG version has been extender to poroelastic and conducting poroelastic (poroelastic+electromagnetic) media.
-
Functional Description:
This software simulates the propagation of waves in heterogeneous 2D and 3D media in time-domain and in frequency domain. It is based on an Interior Penalty Discontinuous Galerkin Method (IPDGM) and allows for the use of meshes composed of cells of various order (p-adaptivity in space).
-
News of the Year:
In 2022, we have focuses the 3D seismoelectomagnetic equations and compared various approximation of the frequency-dependent coupling coefficient.
- URL:
-
Publications:
hal-01513597, hal-01957131, hal-01388195, hal-01972134, hal-01957147, hal-02152117, hal-02486942, hal-02408315, hal-02911686, hal-03464413, tel-03442300, tel-03014772, hal-01656440, hal-01662677, hal-01623953, hal-01623952, hal-01513597, hal-01519168, hal-01254194, hal-01400663, hal-01400656, hal-01400643, hal-01313013, hal-01303391, hal-01408981, tel-01304349, hal-01184090, hal-01223344, hal-01207897, hal-01184111, hal-01184110, hal-01184107, hal-01207906, hal-01184104, hal-01207886, hal-01176854, hal-01408705, hal-01408700, tel-01292824, hal-01656440, hal-00931852, hal-01096390, hal-01096392, hal-01096385, hal-01096324, hal-01096318, tel-01133713, tel-00880628
-
Contact:
Julien Diaz
-
Participants:
Conrad Hillairet, Elodie Estecahandy, Julien Diaz, Lionel Boillot, Marie Bonnasse, Marc Fuentes, Rose-cloe Meyer, Vinduja Vasanthan, Arjeta Heta
7.1.3 Hawen
-
Name:
time-HArmonic waVe modEling and INversion using Hybridizable Discontinuous Galerkin Discretization
-
Keywords:
Inverse problem, Wave Equations, Geophysics, Helioseismology, Discontinuous Galerkin, MPI, Large scale, Wave propagation, 3D, 2D
-
Scientific Description:
Many applications such as seismic and medical imaging, material sciences, helioseismology, and planetary science, aim to reconstruct properties of a non-directly accessible or non-visible interior. For this purpose, they rely on waves whose propagation through a medium interrelates with the physical properties (density, sound speed, etc.) of this medium. Hawen is a software designed to perform imaging with waves, following an algorithm that comprises of two main stages: In the data acquisition stage, the medium response to probing waves is recorded (e.g., seismic waves from Earthquakes recorded by ground network). In the second stage, we rely on a reconstruction procedure that iteratively updates an initial model of physical parameters, so that numerical simulations approach the measurements. This procedure is employed, for instance, for seismic (reconstruction of subsurface layers) and medical (disease diagnostic) imaging.
-
Functional Description:
The software allows the reconstruction of the physical properties of a media using waves propagating therein. For instance, Hawen allows the recovery of the physical properties of the Earth and the Sun for the observations of surface oscillations. Such applications are of interest in geophysics and helioseismology.
-
Release Contributions:
Compared with the 2/1/2023 version (v1.2.1) - Improved simulations in elastic media, - Improved inversion methods, - New features, such as the consideration of new type of sources for the wave propagation, - Compilation with CMAKE, - Incorporation of validation tests.
- URL:
- Publications:
-
Contact:
Florian Faucher
-
Participants:
Florian Faucher, Marc Fuentes, Ha Howard
-
Partner:
University of Vienna
7.1.4 MONTJOIE
-
Keywords:
High order finite elements, Edge elements, Aeroacoustics, High order time schemes
-
Scientific Description:
Montjoie is designed for the efficient solution of time-domain and time-harmonic linear partial differential equations using high-order finite element methods. This code is mainly written for quadrilateral/hexahedral finite elements, partial implementations of triangular/tetrahedral elements are provided. The equations solved by this code, come from the ”wave propagation” problems, particularly acoustic, electromagnetic, aeroacoustic, elastodynamic problems.
-
Functional Description:
Montjoie is a code that provides a C++ framework for solving partial differential equations on unstructured meshes with finite element-like methods (continuous finite element, discontinuous Galerkin formulation, edge elements and facet elements). The handling of mixed elements (tetrahedra, prisms, pyramids and hexahedra) has been implemented for these different types of finite elements methods. Several applications are currently available : wave equation, elastodynamics, aeroacoustics, Maxwell's equations.
- URL:
-
Contact:
Marc Durufle
-
Participants:
Juliette Chabassier, Marc Durufle, Morgane Bergot
7.1.5 GEOSX
-
Keywords:
Physical simulation, Multiphysics modelling
-
Functional Description:
GEOSX is an open-source, multiphysics simulator developed cooperatively by Lawrence Livermore National Laboratory, Stanford University, and TotalEnergies. Its goal is to open up new horizons in modeling carbon storage and other subsurface energy systems. This includes: - taking advantage of the ongoing revolution in high-performance computing hardware, which is enabling orders-of-magnitude gains in performance, but also forcing a fundamental rethink of our software designs, - enriching the physics used in industrial simulations, allowing complex fluid flow, thermal, and geomechanical effects to be handled in a seamless manner, - developing highly-scalable algorithms for solving these coupled systems, - and improving workflows for modeling faults, fractures, and complex geologic formations. Inria contributes to the seismic wave propagators of GEOSX, and to its python interface. Inria also contributes advanced workflows for seismic inversion, and CO2 storage an monitoring.
- URL:
-
Contact:
Randolph Settgast
7.1.6 Gar6more2D
-
Keywords:
Validation, Wave propagation
-
Functional Description:
This code computes the analytical solution of problems of waves propagation in two layered 3D media such as- acoustic/acoustic- acoustic/elastodynamic- acoustic/porous- porous/porous,based on the Cagniard-de Hoop method.
- URL:
- Publications:
-
Contact:
Julien Diaz
-
Participants:
Abdelaâziz Ezziani, Julien Diaz
-
Partner:
Université de Pau et des Pays de l'Adour
7.1.7 GoTem3
-
Keywords:
Trefftz, Electromagnetic waves, GMRES, Iterative method
-
Functional Description:
GoTem3 is domain decomposition platform based on the ultra-weak formualtion of Cessenat and Després for the solution of diffraction problems posed on regular grids. It uses matrix free strategies as well as local and global preconditioners to solve cases involving more than a billion degrees of freedom on a single computational core.
-
Contact:
Sebastien Tordeux
7.2 Open data
7.2.1 Synthetic dataset for visco-acoustic imaging
Participant: Florian Faucher.
Dataset link: https://hdl.handle.net/11353/10.1622130; https://data.mendeley.com/datasets/jh63d6jmmc/1.
We provide computationally generated dataset simulating propagation of ultrasonic waves in viscous tissues in two and three dimensional domains. The dataset contains physical parameters of a human breast with a high-contrast inclusion, the acquisition setup with positions of sources and receivers, and the associated pressure-wave data at ultrasonic frequencies. We simulated the wave propagation based on seven different viscous models using the physical parameters of the breast. Furthermore, different choices of conditions for the medium's boundaries are given, namely absorbing and reflecting boundaries. The dataset allows to evaluate the performance of reconstruction methods for ultrasound imaging under attenuation model uncertainty, that is, when the precise attenuation law that characterizes the medium is unknown. In addition, the dataset enables to evaluate the robustness of inverse scheme in the context of reflecting boundary conditions where multiple reflections illuminate the sample, and/or the performance of data-processing to suppress these multiple reflections.
This is a joint work with Otmar Scherzer (University of Vienna), this dataset is published in the Elsevier journal Data in Brief, 15.
7.2.2 WaveBench: Benchmarking Data-driven Solvers for Linear Wave Propagation PDEs
Participant: Florian Faucher.
Dataset link: https://zenodo.org/records/8015145; https://github.com/wavebench/wavebench.
Wave-based imaging techniques play a critical role in diverse scientific, medical, and industrial endeavors, from discovering hidden structures beneath the Earth’s surface to ultrasound diagnostics. They rely on accurate solutions to the forward and inverse problems for partial differential equations (PDEs) that govern wave propagation. Surrogate PDE solvers based on machine learning emerged as an effective approach to computing the solutions more efficiently than via classical numerical schemes. However, existing datasets for PDE surrogates offer only limited coverage of the wave propagation phenomenon. In this paper, we present WaveBench, a comprehensive collection of benchmark datasets for wave propagation PDEs. WaveBench (1) contains 24 datasets that cover a wide range of forward and inverse problems for time-harmonic and time-varying wave phenomena in 2D; (2) includes a user-friendly PyTorch environment for comparing learning-based methods; and (3) comprises reference performance and model checkpoints of popular PDE surrogates such as U-Nets and Fourier neural operators. Our evaluation on WaveBench demonstrates the impressive performance of PDE surrogates on in-distribution samples, while simultaneously unveiling their limitations on out-of-distribution (OOD) samples. This OOD-generalization limitation is noteworthy, especially since we use stylized wavespeeds and provide abundant training data to PDE surrogates. We anticipate that WaveBench will stimulate the development of accurate wave-based imaging techniques through machine learning.
This is a joint work with Tianlin Liu, Ivan Dokmanic and AmirEhsan Khorashadizadeh (University of Basel), Antonio Lara-Benitez and Maarten V. de Hoop (Rice University). The dataset is currently under review and will be made available after aproval from the reviewers.
8 New results
8.1 Methodological contributions to the simulation of mechanical and electromagnetic waves in complex media
8.1.1 Hybridizable Discontinuous Galerkin discretization for linear anisotropic elastic wave equation: Voigt-notation and stabilization
Participants: Hélène Barucq, Florian Faucher, Ha Pham.
This work is concerned with implementing the hybridizable discontinuous Galerkin (HDG) method to solve the linear anisotropic elastic equation in the frequency domain, focusing in particular on providing a compact description of the discrete problem and an optimal choice of stabilization in defining the HDG numerical traces. Voigt notation is employed in the description of the discrete problem in order to facilitate matrix operation and to provide efficient book-keeping of physical parameters. Additionally, a first-order formulation working with the compliance elasticity ten- sor is employed to allow for parameter variation within a mesh cell, for better representation of complex media. We determine an optimal choice of stabilization by constructing a hybridized Godunov-upwind flux for anisotropic elasticity possessing three distinct wavespeeds. This stabi- lization removes the need to choose judiciously scaling factors and can be used as a versatile choice for all materials. Its optimality is established by comparing with identity matrix-based stabiliza- tion in a wide range of values for the scaling factor. Numerical investigations are carried out in two and three dimensions, for isotropic elasticity and material with varying degree of anisotropy. Details are available in the research report 44
8.1.2 Stability and space/time convergence of Störmer-Verlet time integration of the mixed formulation of linear wave equations
Participants: Juliette Chabassier.
This work focuses on the mixed formulation of linear wave equations. It provides a proof of stability and convergence of time discretisation of a semi discrete linear wave equation in mixed form with Störmer-Verlet time integration, that is uniform as the time step reaches its largest allowed value for stability (Courant-Friedrich-Levy condition), contrary to the proofs recalled here from the literature. This has been submitted to M2AN 41.
8.1.3 Two implicit parametrized discretization strategies for the mixed formulation of linear wave equations
Participants: Juliette Chabassier.
This work introduces two parametric implicit strategies for discretizing the mixed formulation of linear wave equations, based for the first on the Störmer-Verlet scheme and for the second on the Crank-Nicolson scheme. If the initial conditions are well chosen, these two strategies are equivalent, and equivalence formulas allow the variables of one scheme to be reconstructed from the other. These two schemes are also equivalent to the -scheme discretization of the wave equation formulated at order 2. However, the first scheme has a lower complexity than the second one. Numerical results illustrate these equivalences and these efficiency comparisons. This is available in the report 42.
8.1.4 Space time convergence of implicit discretization strategies for the mixed formulation of linear wave equations
Participants: Juliette Chabassier.
This work addresses the space-time convergence analysis of two implicit discretization strategies for the mixed formulation of wave equations, based for the first on the Störmer-Verlet scheme and for the second on the Crank-Nicolson scheme. This analysis is uniform with respect to the space-time convergence ratio. For each discretization strategy, after obtaining an exploitable energy identity, a uniform stability result is achieved through a technique of projection onto well-chosen eigensubspaces of a certain symmetric real matrix appearing in the construction of the schemes. Uniform convergence is then demonstrated using increasingly refined techniques to reduce the regularity assumptions on the semi-discrete solution. This is available in the report 40.
8.1.5 Optimized Finite Differences methods and Machine Learning to reduce numerical dispersion for the wave equation
Participants: Hélène Barucq, Henri Calandra, Florian Faucher, Nicolas Victorion.
We consider the wave equation in acoustic media and evaluate the performance of Finite Difference (FD) schemes. The objective is to reduce the computational cost while maintaining the accuracy avoiding numerical dispersion. To do so, we study the size of stencils and optimized weight for the spatial derivative to obtain the best results in terms of computational cost. We review the optimized coefficients that have been introduced in the literature to minimize the numerical dispersion and we compare their performance on different test cases, with the perspective to reduce as much as possible the number of points per wavelength. To go further we propose a non conventional method, based on Machine Learning to remove numerical dispersion. Here, we simulate on a coarse grid and a small stencil. Hence the simulation is fast but numerical dispersion appears, which we correct with a Machine learning. This correction is applied during the simulation at selected time steps. Consequently, we reduce run time while avoiding the numerical dispersion.
This work has been presented at the Mathias conference, Journées Ondes Sud-Ouest and ROA 34, 32, 33. It is a collaboration with Emmanuel Franck (Tonus, Inria, Nancy Grand Est).
8.1.6 Modal computation for open waveguides
Participants: Hélène Barucq, Marc Duruflé, Augustin Leclerc.
The study of electromagnetic (EM) wave propagation is essential for considering the impact of Human’s technologies on the environment. For instance, the offshore wind energy is transported through twisted dynamic cables, which armours prevent a significant portion of the waves to irradiate outside the cable. Nevertheless, a remaining and possibly significant part might escape from the cable. Hence, our aim is to look at their scattering in the large stretch of sea water. To consider this problem, we propose to modelize the cable and the surrounding water by an open 3D waveguide, which is an invariant domain according to the cable direction and which is unbounded in the two other directions. We then take a modal approach for the resolution. As a first step, we have consider the Helmholtz equation which corresponds to Transverse Magnetic fields solutions to the Maxwell equations. We have developed new absorbing boundary conditions allowing to perform local computations yielding the behavior of the EM field in the vicinity of the cable. We have analyze their relevance, especially at low frequency. An article on ABCs has been submitted and a talk was given by Augustin Leclerc at ROA days (see). As a second step, we have considered the 3D problem governed by the Maxwell equations. The analysis is on going. This work is part of a PhD program which started in October 2021. The topic has been proposed by the SME Kraken Subsea and is now the heart of a collaboration between this SME, Makutu and INSA Rouen with Christian Gout and Antoine Tonnoir.
8.1.7 Trefftz methods for Maxwell equations
Participants: Matthias Rivet, Margot Sirdey, Sébastien Tordeux.
The simulation of time-harmonic electromagnetic waves requires a matrix inversion whose cost, especially in three-dimensional cases, increases quickly with the size of the computational domain. This is a tangible issue regarding the memory consumption. Trefftz methods consist in using a discontinuous Galerkin method whose basis functions are specific to the considered physical problem and thus reduce numerical dispersion phenomena. We propose an iterative Trefftz solver based on a domain decomposition method which will reduce considerably the memory consumption. However, iterative Trefftz methods based on a plane wave approximation are ill-conditioned. To overcome this problem, we propose improvements of the Cessenat and Després preconditioner and a basis reduction. A matrix-free strategy allows to avoid the assembly of the matrix associated to the linear system and GMRES solver does not require the computation of the inverse anymore. This is a joint work with Sébastien Pernet (ONERA) and a paper has been accepted 17. This year we have also developped an auxiliary solver based on the flux reconstruction method. A paper is underreview 45. .
8.1.8 Sparse direct solver applied to 3D wave propagation.
Participants: Florian Faucher.
We consider the Hybridizable Discontinuous Galerkin (HDG) discretization method for the numerical simulation of time-harmonic waves in acoustic and elastic media. The discretization results in large sparse systems of linear equations of the form AX = B to be solved for different frequencies. Although a significant amount of memory is required, these systems can be efficiently solved using sparse direct solvers, that exploit the block structure of A and that factorize A only once for multiple right-hand sides. We revisit the potential of the massively-parallel sparse multifrontal solver MUMPS and its recent features to efficiently solve our problem, using the Makutu software Hawen to conduct the experiments.
This is a joint work with the MUMPS group, in particular Patrick Amestoy (ENS Lyon), Jean-Yves L'Excellent (ENS Lyon), Théo Mary (Sorbonne University) and Chiara Puglisi. This work is part of the GENCI Grand Challenge ADASTRA for computational resources.
8.1.9 Iterative Trefftz methods for anisotropic acoustic problems equations
Participants: Hélène Barucq, Ibrahima Djiba, Sébastien Tordeux.
We are developing a numerical framework for the anisotropic wave equation thanks to a Trefftz method. We aim at solving a time harmonic problem without any matrix inversion. We resort to a Cessenat Després preconditioner and to a GMRES solver to accelerate the convergence of the algorithm wich allows to compute the solution of the considered problem with a low memory cost. A research report should be published in March 2023. This work has been presented at SMAI 26.
8.1.10 A HDG+ method for the diffusive-flux formulation of the convected Helmholtz equation
Participants: Hélène Barucq, Nathan Rouxelin, Sébastien Tordeux.
We construct HDG methods based on the diffusive flux formulation of the convected acoustic wave equation.We mostly describe the HDG+ method which involves different polynomial degrees for approximating the unknowns, hence leading to a more efficient method with a super-convergencelike behaviour. A detailed analysis of the methods including local and global well-posedness, as well as convergence estimates is carried out. The HDG+ method is also compared with a more conventional HDG method to demonstrate its effectiveness. A paper has been accepted 11 and another one is currently under review 36.
8.1.11 Out-of-distributional risk bounds for neural operators with applications to the Helmholtz equation
Participants: Florian Faucher.
Despite their remarkable success in approximating a wide range of operators defined by PDEs, existing neural operators (NOs) do not necessarily perform well for all physics problems. We focus here on high-frequency waves to highlight possible shortcomings. To resolve these, we propose a subfamily of NOs enabling an enhanced empirical approximation of the nonlinear operator mapping wave speed to solution, or boundary values for the Helmholtz equation on a bounded domain. The latter operator is commonly referred to as the ”forward” operator in the study of inverse problems. Our methodology draws inspiration from transformers and techniques such as stochastic depth. Our experiments reveal certain surprises in the generalization and the relevance of introducing stochastic depth. Our NOs show superior performance as compared with standard NOs, not only for testing within the training distribution but also for out-of-distribution scenarios. To delve into this observation, we offer an in-depth analysis of the Rademacher complexity associated with our modified models and prove an upper bound tied to their stochastic depth that existing NOs do not satisfy. Furthermore, we obtain a novel out-of-distribution risk bound tailored to Gaussian measures on Banach spaces, again relating stochastic depth with the bound. We conclude by proposing a hypernetwork version of the subfamily of NOs as a surrogate model for the mentioned forward operator.
This is a joint work with Antonio Lara-Benitez and Maarten V. de Hoop (Rice University), Takashi Furuya (Shimane University), Anastasis Kratsios (McMaster University) and Xavier Tricoche (Purdue University).
A preprint on this work has been written, 37.
8.2 Seismic imaging
8.2.1 Experimental characterization and modeling of electromagnetic waves generated by seismo-electric conversion at porous media interfaces
Participants: Hélène Barucq, Victor Martins Gomes.
Seismo-electromagnetic phenomena, electrokinetic in nature, take place whenever a seismic wave propagates in fluid-bearing media, its energy depending mainly on the electrical properties of the fluid and the hydraulic properties of the porous medium. They result from a conversion of mechanical into electromagnetic (EM) energy due to the transient ionic interactions occurring at the pore scale. Two of these phenomena are usually studied: the electric field accompanying seismic waves, and the EM field that travels independently, generated at discontinuities of physicochemical properties in the porous medium. Although the first event is sensitive to physical parameters of the surrounding medium, the second catches information about interfaces in the subsurface, with the resolution of seismic methods, making it very attractive to near surface exploration. In this context, we propose a new experimental setup where both phenomena can be simultaneously studied. At first, we use a porous medium composed of homogeneous water-saturated sand and study the characteristics of the coseismic electric field. Afterwards, a thin layer of Vosges sandstone is inserted into the sand, which allows the study of the EM waves generated at the two closely spaced sand-sandstone interfaces. We record the seismic displacement field at the upper surface of the sand volume using a laser vibrometer, and use stainless steel electrodes buried in the sand to acquire individual electric potentials rather than electric fields, seeking to favour the measurement of the EM interface-generated signals. With the help of direct numerical simulations, we compare experimental measurements and theoretical predictions, based on a well established set of seismoelectric governing equations. In both types of experiments, this comparison shows very good agreements between experimental and numerical waveforms, thus confirming the relevant theory. The electric potential data also show that the EM signals generated at interfaces are clearly recorded at distances of about 10 seismic P wavelengths away from the interface. By contrast, the same events are barely noticeable near the inserted layer when measured using classical electric dipolar arrays. This work has been done in collaboration with Daniel Brito (LFCR, CNRS, UPPA), Stéphane Garambois (Isterre, Grenoble) and Clarisse Bordes (LFCR, CNRS, UPPA) and Michel Dietrich (Isterre, Grenoble). An article has been published 16.
8.2.2 From a quantitative analysis of wave's amplitude to full waveform inversion
Participant: Marine Deheuvels, Florian Faucher.
In this work we investigate the recovery of heterogeneities of decimeter-scale samples using the measurement of seismic waves propagating therein. Our studies set a particular emphasis on the seismic attenuation property of the media, which is a frequency-dependent phenomenon. we develop a method that takes advantage of the reflections to recover an effective attenuation that is close to the actual attenuation of the seismic shear S-waves. Our method further allows us to deduce viscoelastic models and parameters of the samples. The method is first validated with thorough numerical experiments. Then, we consider experimental measurements on aluminum, Fontainebleau sandstone, Carrara marble, and diorite samples. We use an experimental setup made up of piezoelectric sources and an interferometer laser receivers that measure the wavefield on the boundary of the samples. From these measurements, we are able to reconstruct the elastic parameters of these samples using first-wave arrivals analysis, and then we reconstruct the viscous parameters with the aforementioned method that we introduce.
This is the subject of Marine Deheuvels Ph.D. at UPPA, successfully defended in October 2023. She was supervised by Daniel Brito (UPPA) and Florian Faucher.
8.2.3 Quantitative inverse wave problems in visco-acoustic and visco-elastic media
Participant: Florian Faucher.
We consider the propagation of waves in media with attenuation. Attenuation is a frequency-dependent phenomenon and several constitutive laws exist to consider it, each leading to different models of wave equations. In the inverse problem for the reconstruction of the physical properties from the measurements of waves, considering a medium with attenuation leads to additional unknown coefficients to recover (those that represent the attenuation), while the precise attenuation law characterizing the medium is also unknown a priori. In this work, we consider the inverse wave problem for visco-acoustic and visco-elastic media. We use time-harmonic formulations which allow us to unify the models of attenuation using complex-valued parameters and illustrate the wave propagation depending on the attenuation model. We carry out quantitative reconstruction with attenuation model uncertainty using an iterative minimization procedure. Furthermore, we also consider the case of reflecting boundaries surrounding the sample, which lead to multiple reflections within the domain and complicate the reconstruction.
The visco-acoustic case has been published in the Journal of Computational Physics, 14, with the associated dataset published online in the journal Data in Brief, 15. This work has been presented at the Workshop in Cambrige, 29 and conference AIP 30.
8.2.4 Numerical and experimental study of ultrasonic seismic waves propagation and attenuation on high quality factor samples
Participants: Marine Deheuvels, Florian Faucher.
We propose an approach for measuring seismic attenuation at ultrasonic frequencies on laboratory‐scale samples. We use a Gaussian filter to select a bandwidth of frequencies to identify the attenuation in a small window and, by moving the window across the frequency content of the data, we determine the frequency‐dependent attenuation function. We assess the validity of the method with 3D numerical simulations of seismic wave propagation across different sample geometries, using free surface boundary conditions. We perform the simulations using viscoelastic media under various seismic attenuation models. Our numerical results indicate that we can successfully recover the representative viscoelastic attenuation parameters of the media, regardless of the sample geometry, by processing the seismic signal recorded either within the volume or at the boundaries. Due to the equipartition phenomenon, the energy of S‐waves is consistently higher in seismic records than that of P‐waves. Therefore, we systematically recover the attenuating properties of S‐waves in the medium. We also conduct experiments of seismic wave propagation on samples of aluminum and Fontainebleau sandstone to validate our approach with real data. The quality factor of the S‐wave in the aluminum medium increases from 300 to 7000 between 60 kHz and 1.2 MHz. The Fontainebleau sandstone, which is more attenuating, exhibits a that increases from 200 at 60 kHz to 1000 at 1.2 MHz. With our approach, we are not only able to recover the attenuation property, but also identify the frequency‐dependent attenuation model of the samples. Our method allows for seismic attenuation recovery at ultrasonic frequencies in low‐attenuating media.
This is the subject of Marine Deheuvels Ph.D. at UPPA, she is supervised by Daniel Brito (UPPA) and Florian Faucher. This work is published in Geophysical Prospecting 12, it has been presented at the conference EAGE.
8.2.5 Precision of DAS simulation for CO2 monitoring and FWI application
Participants: Hélène Barucq, Henri Calandra, Florian Faucher, Stefano Frambati, Chengyi Shen.
Distributed acoustic sensing (DAS) is one of the latest and prominent measuring instruments based on the development of laser technology. DAS has a wide range of application domains, from geophysics to biology and natural sciences. DAS has gained increasing interest in geophysics during the last decade and the research on it is continuously growing. Seismic data quality, density and availability are crucial in both exploration and experimental geophysics. DAS can meet these needs by providing densely-measured data. In terms of DAS acquisition, the fibre-optic cable layout may be adapted to any geometry, it can be conveniently deplored permanently in a large scale, which is particularly suitable for 4D monitoring of CO2 plumes for instance. We perform numerical simulations to evaluate the accuracy of DAS.
This work is presented at EAGE 25
8.2.6 Frequency analysis of the seismoelectric coupling operator
Participants: Hélène Barucq, Julien Diaz, Arjeta Heta.
Pride's equations 79 model seismoelectric effects resulting from the coupling between seismic and electromagnetic waves in porous media. They are thus equations of dynamic in porous media coupled with Maxwell's equations. The coupling is achieved in terms of a coefficient which is defined in the time domain as a Fourier integral operator. The exact is thus difficult to discretize and is approximate by a leading term independent of the frequency. When considering the time harmonic equations, the associated is the inverse of a square root in the frequency. We address the idea of using approximate time-harmonic coupling terms defined as rational or polynomial functions of the frequency. By this way, it would be possible to switch from time-harmonic coupling terms to time-dependent ones. In practice, is replaced in the time domain by a constant coefficient which should only reproduce the coupling accurately in the low frequency range. We have obtained approximate expressions of the coupling terms which can be derived in a frequency regime limited by the cut-off frequency. We use Padé approximants to approximate the square root by rational functions of frequency. Numerical experiments show that actually reproduces correctly the seismoelectric effect at low frequencies while higher-order rational approximations of perform better at mid and high frequencies.
Another challenge for the numerical modelling in time domain is the coexistence of waves propagating a thousand times faster than seismic waves. To alleviate this difficulty, we can modify the dielectric permittivity, thereby reducing the propagation speed of electromagnetic waves. The use of a fictitious permittivity to address the diffusive nature of the EM signal has been introduced with success by other authors (e.g., 73, 74). In order to assess the accuracy of using a fictitious dielectric permittivity we compared numerical results provided by Hou10ni in frequency domain to numerical simulations in the time-domain, based on a spectral-element method. Comparisons were made in the low frequency regime, which is characteristic of field scale problems.
This work is the topic of Arjeta Heta's Ph.D. thesis, it is a collaboration with Cristina Morency in the framework of the SEE4GEO project 10.2. It was presented in a poster at the AGU conference in San Francisco (Dec.2023)49.
8.2.7 Optimized Full Waveform Inversion for seismic in GEOS
Participants: Hélène Barucq, Julien Besset, Henri Calandra, Stefano Frambati.
The reduction of energy carbon footprint justifies the recent major programs launched on CO2 storage in existing reservoirs known to geologists. Numerical simulation plays a key role in their implementation by providing a low-cost means of monitoring. This is a global concern that explains the use of open-source software platforms facilitating knowledge sharing and collaborations. Regarding monitoring aspects, Full Waveform Inversion (FWI) has demonstrated its ability in probing the subsurface accurately. FWI is an iterative process in which we need to solve forward problems in large-scale propagation domains whose discretization involves more than 108 cells each. Implementing an FWI algorithm needs thus an optimized architecture in terms of memory management and GPU-CPU computation. Among the existing open-source platforms, GEOSX targets such architecture. Moreover, GEOSX offers a multi-physics approach ready for reservoir simulation and offering the perspective of coupling with seismic.
During this year, we have addressed the idea of constructing reduced bases to provide faster computational software for large scale inversion. It turns out that standard construction techniques deliver reduced bases which depend on the velocity domain. Hence, with the purpose of reconstructing the velocity model, it is necessary to reconstruct the basis functions at each update of the model in the inversion workflow. We have thus proposed a new method of construction which involves Fréchet derivatives. This work is a new collaboration with Rabia Djellouli (CSUN). It has been presented at the conference Mathias Days in October 2023 (see 28). An article is currently being written.
8.2.8 Machine Learning approaches for CO2 geological storage monitoring: repeatability, inversion and generative models
Participants: Hélène Barucq, Henri Calandra, Stefano Frambati, Manon Sarrouilhe.
The goal of this work just begun is to investigate how current and future Machine Learning (ML) techniques can be used to improve CO2 injection monitoring techniques and help current approaches, based mainly on numerical analysis, raise to the standards required for large-scale CO2 injection operations. In particular, classical noise-reduction neural networks used in image processing are employed more and more for physical systems, and represent good candidate solutions to acquisition repeatability issues. Using current simulation codes, one can generate synthetic data that includes sources of systematic error such as source and receiver position, reservoir uplifting and specific reservoir response function change, which can be used to train networks and models. One of the goals of this thesis is therefore to investigate how these techniques can be adapted to include the knowledge of spatial distribution of the acquisition geometry, and used to distinguish the specific signature of systematic error in repeated seismic acquisition campaigns. Recent works on supervised learning for geophysics applications have shown that models based on convolutional neural networks (CNN) are able to achieve grater precision in the reconstruction of reservoir CO2 saturation distribution from surface gravimetry data, compared to standard inversion techniques. Adapting these approaches to seismic data, which is able to carry more information due to the hyperbolic character of its governing equations, is a very promising research avenue and could lead to much improved reservoir imaging with much sparser acquisition data. In particular, a significant reduction in the number of source shots can be achieved, with positive effect on both the computational and the experimental cost of an acquisition campaign. One goal of this thesis is to investigate the role of similar supervised learning models for direct reservoir inversion from seismic data. Furthermore, achieving this results paves the way for the integration of multiple physics (including, for example, gravity and electromagnetic waves, as well as reservoir production and injection data) into the constraining data of the reservoir model, leading to a much more complete and robust inversion scheme.
8.3 Helioseismology
8.3.1 Effects of Cowling approximation on solar oscillations in radial symmetry
Participants: Hélène Barucq, Lola Chabat, Florian Faucher, Ha Pham.
Helioseismology investigates the interior structures and the dynamics of the Sun from oscillations observed on its visible surface. Ignoring flow and rotation, time-harmonic adiabatic waves in a selfgravitating Sun in Eulerian-Lagrangian description are described by the Lagrangian displacement and the gravitational potential perturbation which satisfy Galbrun's equation coupled with a Poisson equation. In most works, perturbation to gravitational potential is neglected under Cowling's approximation. However, this approximation is known to shift the eigenvalues of the forward operator for low-order harmonic modes. Here, we study the effects of this approximation on numerical solutions and discuss its implication for the inverse problem. Removing Cowling's approximation allows us to accurately simulate waves for low-degree modes, and help us better characterize the deep interior of the Sun. The investigation is carried out for a Sun with minimum activity, called quiet Sun, whose background coefficients are given by the radially symmetric standard solar model Model S in the interior, with a choice of extension beyond the surface to include the presence of atmosphere. Radial symmetry is exploited to decouple the problem on each spherical harmonic mode to give a system of ordinary differential equations in radial variable.
This is the Ph.D. thesis of Lola Chabat, and it has been presented at the conference AIP 47, the Journées Ondes Sud-Ouest 48, and the ROA 23.
8.3.2 Imaging individual active regions on the Sun's far side
Participant: Hélène Barucq.
Earth-side observations of solar p modes can be used to image and monitor magnetic activity on the Sun's far side. Here we use magnetograms of the far side obtained by the Polarimetric and Helioseismic Imager (PHI) onboard Solar Orbiter (SO) to directly assess – for the first time – the validity of far-side helioseismic holography. We wish to co-locate the positions of active regions in helioseismic images and magnetograms, and to calibrate the helioseismic measurements in terms of magnetic field strength. We identify three magnetograms on 18 November 2020, 3 October 2021, and 3 February 2022 displaying a total of six active regions on the far side. The first two dates are from SO's cruise phase, the third from the beginning of the nominal operation phase. We compute contemporaneous seismic phase maps for these three dates using helioseismic holography applied to time series of Dopplergrams from the Helioseismic and Magnetic Imager (HMI) on the Solar Dynamics Observatory (SDO). Among the six active regions seen in SO/PHI magnetograms, five active regions are identified on the seismic maps at almost the same positions as on the magnetograms. One region is too weak to be detected above the seismic noise. To calibrate the seismic maps, we fit a linear relationship between the seismic phase shifts and the unsigned line-of-sight magnetic field averaged over the active region areas extracted from the SO/PHI magnetograms. SO/PHI provides the strongest evidence so far that helioseismic imaging provides reliable information about active regions on the far side, including their positions, areas, and mean unsigned magnetic field.
This work was the topic of a review article in collaboration with Laurent Gizon and Dan Yang of the Max Plank Institute for Solar system research in Göttingen (MPS), with contributions of many authors. It is published in Astronomy & Astrophysics, 18. It was preceded by another work in collaboration with Laurent Gizon and Dan Yang, developing a method for imaging active zones on the Sun's far side based upon holography 19.
8.4 Musical Acoustics
8.4.1 Modeling and numerical simulation of the piano touch
Participants: Juliette Chabassier, Guillaume Castera.
The question of the influence of piano touch on sound is often debated within the pianist and scientific communities. The hypothesis that touch can influence sound via longitudinal waves in the strings is widespread, but has never been verified, either experimentally or by physical modeling. Numerical simulation of the piano in the time domain, with a particular focus on longitudinal waves, enables us to address this question. The work carried out in 2023 consists of the application and mathematical analysis of energy quadratization methods for strings in a variational formalism, which has been the subject of an Inria research report 39. The modeling of rotational movements of the bridge for the coupling of the string to the soundboard has also been studied and is the subject of a research report 38. In addition, a regularized Coulomb model allows friction between the hammer and the string to be taken into account. An efficient numerical scheme that quadratizes a kinetic energy nonlinearity has been applied to the hammer's flexible handle and submitted to the M2AN journal in August 2023. The combination of these contributions and a multi-body model of striking mechanics enabled the study of the influence of piano touch on sound. The year ended with the drafting of the thesis manuscript, bringing together all the research and contributions, followed by the defense on December 12th.
8.4.2 Study of the influence of wall roughness on wave propagation in acoustic tubes
Participants: Alexis Thibault, Juliette Chabassier, Augustin Ernoult.
Wall roughness can increase dissipation in acoustic tubes. Using the theory of tubes with constant cross-sectional shape, various passive models have been proposed allowing numerical simulations in the harmonic domain, or in the time domain using diffusive representations. A preliminary experimental study based on acoustic measurements of grooved tubes has shown that it is possible to estimate the relative roughness factor from measurements, and that the ZK-HR model then accurately describes the the frequency dependence of the imaginary part of the wavenumber. This work was presented at the Journées Ondes du Sud-Ouest (March 14-16, 2023) and has been done in collaboration with Henri Boutin, Gérard Michel, Thomas Hélie.
8.4.3 Modeling, analysis and simulation of dissipative acoustics in porous or rough tubes, with application to wind instruments
Participants: Alexis Thibault, Juliette Chabassier.
Time simulations of wind instruments require accurate and efficient resolution of the acoustic equations in the resonator. In particular, acoustic losses present a number of difficulties: on the one hand, the imperfect nature of the material is often neglected in existing models; secondly, their pseudo-differential time behavior makes them difficult to analyze and discretize. This thesis proposes (I) robust simulation methods for non-dissipative acoustics in wind instruments, (II) models for visco-thermal dissipation in conical dissipation in conical tubes and porous or rough-walled tubes, and (III) theoretical analysis and numerical methods for dissipative acoustics. Alexis Thibault defended his thesis on October 18, 2023.
This thesis has been coadvised by Juliette Chabassier, Thomas Hélie and Henri Boutin.
8.4.4 Viscothermal effects in ducts at audible frequencies. Application to wind musical instruments
Participants: Alexis Thibault, Juliette Chabassier.
Viscous and thermal effects occur during the propagation of linear waves in a pipe. Many propagation models are used in musical acoustics, based on different assumptions that induce various model errors. The thermoviscous (or viscothermal) equations are derived from the non-linear 3D Navier-Stokes (NS) equations for a perfect gas by linearization around a uniform steady state. Analytical or numerical solutions can be proposed, after more or less deep modifications of the original system. These derived models will be summarized in a synthetic diagram specifying the assumptions performed for each one. Far from the walls, a standard 3D Helmholtz equation is valid while an effective boundary condition can replace the viscothermal "boundary layers" near the walls of the pipe. Available 1D models describe the propagation of the mean pressure on a well chosen surface and account for thermoviscous effects as a modification of the transmission line coefficients, for cylindrical and conical geometries. This work proposes an evaluation of some of these models (3D and 1D), through a quantitative estimation of model errors in relation to their domains of validity, as well as a numerical comparison for cylindrical and conical domains of propagation. This work has been presented at the Forum Acusticum 2023 - 10th Convention of the European Acoustics Association Sep 2023 22.
8.4.5 Openwind: a software to simulate wind instruments, as a tool for acoustic teachers
Participants: Augustin Ernoult, Jérémy Cabaret, Juliette Chabassier.
Openwind is a python library, (free and open source) dedicated to the simulation of wind instruments. It provides the computation of the acoustic response in the frequency domain (impedance or admittance) or in the time domain (impulse response), from the geometry of the instrument (main bore, side holes, valves). Several models are implemented and gives the possibility to take into account thermo-viscous losses, several radiation conditions etc. It is also possible to perform a sound simulation of brass and reed instruments. A graphical interface freely available online (https://demo-openwind. inria.fr) gives the possibility to perform frequency domain simulations without coding. During a course proposed by the ITEMM (school for instrument makers), the students in musical instrument making were asked to study several problems common in wind instruments, and especially the optimization of the main bore or the position of the side holes. From geometry design to impedance curves and resonances analysis, we will present how students learn to implement a general methodology for solving problems in wind instruments using this virtual prototyping tool.
This work has been presented at the Forum Acusticum 2023 - 10th Convention of the European Acoustics Association Sep 2023 24.
8.4.6 Bore reconstruction of complex wind instruments using the pulse reflectometry technique
Participants: Andréas Autin, Augustin Ernoult.
Today, the majority of musical instruments preserved in museums cannot be played, either because they have deteriorated or because their condition does not allow it (cracks, etc.). It is therefore worthwhile making copies of these instruments to enable instrumentalists to play them as part of historically informed interpretations, in particular to enrich knowledge of museum instruments. In order to make acoustically faithful copies, it is necessary to know precisely the internal geometry of the instrument (bore). To this end, during his master's internship Andréas Autin implemented acoustic reflectometry techniques in the openwind software. These methods, taken from the literature, enable internal geometry to be reconstructed from measurements of the instrument's acoustic impulse response, which are non-destructive and easy to set up. He has adapted the algorithms for use with the most widely-used acoustic impedance sensors, and introduced a technique for estimating the position of side holes. Preliminary results were presented at the Scrime day in July, and the techniques implemented are detailed in his internship report.
8.4.7 Predict the easiness of sound production in clarinet-type instruments
Participants: Anaëlle Fresneau, Augustin Ernoult.
In clarinet-type wind instruments, some fingerings (notes) are more difficult to sound than others. In order to provide support in instrument making, we have sought to predict this difficulty on the basis of the instrument's geometry. From this geometry it is possible to simulate wave propagation in the body of the instrument. Once coupled with a reed model, it is then possible to predict certain characteristics likely to explain this phenomenon, such as the minimum mouth pressure required to produce a sound (via a linear stability analysis) or the importance of non-linear losses at the holes. During her Master's internship, Anaëlle Fresneau implemented these simulation techniques in Openwind software, enabling her to design and build a simplified instrument with a wide range of these characteristics from one fingering to another. This instrument was used in a perceptive study with instrumentalists, and enabled him to show a strong correlation between non-linear losses at the holes and the difficulty of producing the sound felt by the musicians.
Preliminary results were presented in July at the Scrime days, and the final results are detailed in her internship dissertation.
8.4.8 Effect of air composition in the sound propagation for the modeling of wind musical instrument.
Participant: Augustin Ernoult.
The musical context is very demanding on the precision of many quantities. For example, the threshold of hearing the variation of the frequency of a sound is around 3 cents, which corresponds to a variation smaller than . In music acoustics, in order to have sufficiently precise predictions and to be able to compare calculations and measurements, it is therefore necessary to know the value of the physical quantities with great precision. This is particularly true for the speed of sound, which is directly related to resonance frequencies. When making measurements in the laboratory or when playing a wind instrument, the composition of the air can vary through the relative humidity and the carbon dioxide content. This composition influences the physical characteristics of the gas mixture inside the instrument and therefore its acoustic properties. The objective of this report is to review the literature dealing with the estimation of the values of the different quantities necessary for the calculation of wave propagation in wind instruments. The measurements and theoretical aspects gathered show that the humidity and carbon dioxide content modify these quantities in a non-negligible way with respect to the musical requirements. Approximate expressions allowing a simple implementation of these dependencies are also proposed and tested on a simple case (cylindrical tube).
This work was the subject of a research report 43. The formulas obtained have been included in the Openwind library 7.1.1.
8.4.9 Benchmark study on wind instrument models
Participants: Juliette Chabassier, Augustin Ernoult.
Continuing the work begun last year, the double benchmark on the simulation and measurement of the acoustic response (impedance) of wind musical instruments was pursued. The working group, comprising a dozen French research teams, continued to compare different numerical techniques and measurement devices on standard resonator geometries involving different propagation conditions (cylinder, cone, elbow, side hole, etc.) or radiation (closed, with baffle, etc.). New techniques have been included in the simulation benchmark (e.g. fast multipole and new 3D approach); and new experimental data sets have been acquired.
A one-day workshop was held in June at the CNAM (Paris) to discuss this project. More than 20 researchers were present on the day.
The new results were presented at the Forum Acusticum international conference in Turin in September 20.
9 Bilateral contracts and grants with industry
-
Makutu research agreement.
Period: January 2022 – December 2025; Management: INRIA Bordeaux Sud-Ouest, Amount: 350000 euro per year.
-
Numerical schemes assisted with Machine Learning for solving time-dependent seismic wave problems
Period: 2021 November - 2024 October, Management: INRIA Bordeaux Sud-Ouest, Amount: 90000 euros.
- Predoctoral contract with Airbus Period: October 2023 - April 2024, Management: INRIA Bordeaux Sud-Ouest Amount: 19200 euros
10 Partnerships and cooperations
10.1 International research visitors
10.1.1 Visits of international scientists
Rabia Djellouli, Professor of Mathematics at CSUN (California State University at Northridge) visited the team for 4 months.
10.2 European initiatives
SEE4GEO
-
Title:
SeismoElectric Effects for GEOthermal resource assessment and monitoring
-
Duration:
2021 -> 2024
-
Coordinator:
Christina Morency (morency1@llnl.gov) LLNL (Lawrence Livermore National Laboratory, US department of Energy)
-
Partners:
- University of Hawaii at Mänoa (USA);
- University of Pau and the Pays de l'Adour, UPPA (France);
- TLS Geothermics, TLS (France),
- NORCE (Norway)
-
Inria contact:
Hélène Barucq
-
See also:
SEE4GEO on Geothermica website.
-
Summary:
Geothermal systems involve the injection of large amounts of fluid into the subsurface. Identifying fracture networks is of great importance to assess geothermal resources. Traditional seismic imaging techniques fail to resolve fluid-phase properties, while purely electromagnetic (EM) approaches provide limited, low-resolution constraints on the rock structure. Seismoelectric effects (SEE) arise from the seismic-to-electromagnetic conversion in naturally charged porous media with a certain degree of fluid saturation. With SEE, we leverage seismic and EM technique sensitivities. In this project, we offer an integrated SEE assessment for geothermal systems relying on numerical modelling, laboratory experiments and field surveys. Makutu is involved in the project as a joint team with UPPA (University of Pau and Pays de l'Adour).
10.3 National initiatives
ANR JCJC 2023 - Own-Music
Participants: Corto Bastien, Augustin Ernoult.
-
Title:
CustOm design of Woodwind iNstruments: optiMization of the geometry of flUtes, thanks to the modeling of the note-to-note adaptation of the muSIcian's Control
-
Partner Institutions:
- Makutu team, Inria centre at the university of Bordeaux, France
- Institut Jean Le Rond d'Alembert, Sorbonne Université, Paris, France
- Pontificia Universidad Católica (PUC), Santiago, Chile
-
Date/Duration:
from 01/10/2023 to 31/03/2027 (42 month)
-
Coordinator:
Inria, Augustin Ernoult
-
Summary:
the objective is to develop model predicting the adaptation needed to play a series of notes on one instrument, based on acoustic model and experiment and perceptual studies
-
Amount:
268 995€; doctoral position of Corto Bastien and an engineer (to be attributed)
PEPR Numpex - Focused project Exa-MA (Methods and Algorithms for Exascale)
Participants: Hélène Barucq, Julien Diaz, Marc Duruflé, Florian Faucher, Sébastien Tordeux.
The french exascale program aims at designing and developing software bricks that will equip the future exascale computers. Makutu contributes to the topic of advanced discretization and to the design of demonstrators with a focus on large scale inverse problems as demonstrators. Research actions are in their early stages. First PhD theses should be launched in fall 2024 with collaborations with UNISTRA, CEA and other Inria Teams.
11 Dissemination
11.1 Promoting scientific activities
11.1.1 Scientific events: organisation
- Hélène Barucq helped to organize the JOSO event held at Onera Toulouse from March 14 to 16. The main aim of the event was to take a look at university and industrial activities in the field of mathematical modeling and numerical resolution of wave propagation phenomena, both nationally and in the south-west of France.
- Arjeta Heta was the main organizer of the ROA days. Their aim is to create or strengthen links between young researchers at LMI (INSA Rouen) and Makutu.
- Augustin Ernoult organized a one-day workshop "Model and Measure the impedance of wind musical instruments", the 28th of June, at CNAM Paris (link of the event)
Chair of conference program committees
- Augustin Ernoult chair and organized the structured session "Experimental methods for music acoustics" during the Forum Acusticum, 11th-15th September 2023, Torino, Italy.
11.1.2 Journal
Member of the editorial boards
- Hélène Barucq is member of the editorial board of MATCOM (Mathematics and Computers in Simulation, )
Reviewer - reviewing activities
Members of Makutu are regular reviewers for the following journals:
- Geophysics
- SIAM Journal on Scientific Computing
- SIAM Journal on Numerical Analysis
- Geophysical Journal International
- Journal of Computational and Applied Mathematics
- International Journal for Numerical Methods in Engineering
- Journal of Computational Physics
- Mathematics and Computers in Simulation
- Inverse Problems
11.1.3 Invited talks
- Hélène Barucq was a plenary speaker at the conference SMAI (22-26 May 2023) in Le Gosier, Guadeloupe 27
- Hélène Barucq was invited to give a 4-hour mini-course at the Jacques Louis Lions Franco-Spanish school, for PhD students and post-docs.
- Juliette Chabassier gave a talk at the Professional Seminar of the University of Nantes on January 27th 2023.
11.1.4 Leadership within the scientific community
- Augustin Ernoult is elected member of the "Groupe spécialisé d'acoustique musicale" (Gsam) of the french acoustical society.
- Hélène Barucq was a member of ECCOMAS PhD Award 2022 Committee meeting.
- Hélène Barucq is the co-leader of the specific project Exa-MA of PEPR Numpex,
- Hélène Barucq is the Chair of Thematic Committee CT6, Computer Science, Algorithms and Mathematics GENCI
- Hélène Barucq is member of the liaison office of GAMNI
11.1.5 Scientific expertise
- Hélène Barucq is an expert for FWO Review College. This is a panel who has in charge to award research projects in Belgium, on Flander side.
11.1.6 Research administration
- Hélène Barucq is appointed member of the scientific board of the LMA2S (Laboratory of Mathematics Applied to Aeronautics and Space) created to federate the activities in Applied Mathematics which are carried out in seven departments of the ONERA.
- Juliette Chabassier is member of the Research Position Commission of Inria Bordeaux Sud-Ouest.
- Julien Besset is elected member of Laboratory Commitee of LMAP.
- Julien Diaz is elected member of the "Comité Social d'Administration", of the "Formation Spécialisée en Santé et Sécurité au Travail" and of the Inria Administrative Board. He is appointed member of the Bureau du Comité des Projets (BCP) of Inria Bordeaux Sud-Ouest. Since 2018, he has been the head of the Mescal team of LMAP.
- Augustin Ernoult is member of the Center Commitee of Inria Bordeaux Sud-Ouest.
11.2 Teaching - Supervision - Juries
11.2.1 Teaching
- Master : Julien Diaz, Parallel computing, 32 eq. TD, Master, UPPA, France
- Licence : Arjeta Heta, Modèles mathématiques pour l’informatique, 10h Eq. TD, L2, UPPA, France
- Licence : Arjeta Heta, Analyse, 20h Eq. TD, L2, UPPA, France
- Master : Sébastien Tordeux, Outils Mathématiques pour la Mécanique, 49 eq. TD, Master1, UPPA, France
- Master : Sébastien Tordeux, Introduction to wave phenomena, 48 eq. TD, Master2, UPPA, France
- Licence : Sébastien Tordeux et Ibrahima Djiba, Mathématiques pour l'économie et la gestion, UPPA, Pau, France, 96 eq. TD.
- Licence : Matthias Rivet, Analyse numérique, UPPA, Pau, France, 96 eq. TD.
- Licence : Julien Besset, Calcul numérique, 12h Eq. TD, L2, UPPA, France
- Master : Marine Deheuvels, Signal Processing, UPPA, Pau, France, 18 eq. TD.
- Master : Florian Faucher, Inversion/Optimization, UPPA, Pau, France, 6 eq. TD.
11.2.2 Supervision
- PhD defended: Marine Deheuvels, From quantitative amplitude analysis to complete waveform inversion, October 10th, Florian Faucher and Daniel Brito (Univ. Pau et des Pays de l'Adour).
- PhD defended: Alexis Thibault, ?? , October 18th, Juliette Chabassier and ??.
- PhD in progress: Corto Bastien, Modeling the control homogeneity of wind instruments players, November 2023, Augustin Ernoult and Claudia Fritz (Institut d'Alembert, Sorbonne Université, Paris). Funding: ANR OWN-MUSIC.
- PhD in progress: Julien Besset, Development of an optimized computational framework including seismic un the monitoring of CO2 storage, January 2021, Hélène Barucq and Stefano Frambati (TotalEnergies). Funding: TotalEnergies.
- PhD in progress: Nicolas Victorion, Numerical schemes assisted with Machine Learning for solving time-dependent seismic wave problems, October 2021, Hélène Barucq, Florian Faucher and Emmanuel Franck (Inria Nancy Grand-Est, Macaron). Funding: TotalEnergies.
- PhD in progress: Arjeta Heta, Advanced numerical schemes to model seismoelectric effects and improve characterization of geological reservoirs, September 2021, Hélène Barucq and Julien Diaz. Funding: E2S UPPA
- PhD in progress: Augustin Leclerc, Modeling, and numerical simulation of electromagnetic wave propagation in offshore conducting cables. Applications to the environmental impact on marine ecosystem, October 2021, Hélène Barucq, Christian Gout and Antoine Tonnoir. Funding: Conseil Régional de Normandie
- PhD in progress: Ibrahima Djiba, Trefftz domain decomposition method for wave propagation in geophysics, October 2021, Hélène Barucq and Sébastien Tordeux. Funding: ISIFOR (Carnot UPPA) & Inria
- PhD in progress: Lola Chabat, Large scale spectral problems using hybridizable Galerkin discretization with application to helioseismology, October 2022, Hélène Barucq, Florian Faucher and Ha Pham. Funding: E2S UPPA & Inria
- PhD in progress: Manon Sarrouilhe, Machine Learning approaches for CO2 geological storage monitoring: repeatability, inversion and generative models, November 2023, Hélène Barucq, Henri Calandra and Stefano Frambati. Funding: TotalEnergies.
- PhD in progress: Mathias Rivet, Optimisation de flux numériques dans une méthode de décomposition de domaines de type Trefftz pour l'électromagnétisme : approche traditionnelle ou intelligence artificielle ? Ecole Polytechnique
- PhD in progress: Andrea Lagardère, Coupled Quasi-Trefftz for Aeroacoustics, prethesis grant from Airbus
11.2.3 Juries
Hélène Barucq participated in the following juries:
- PhD defense, Zoé Renat, , Université de Lorraine, January 2023, reviewer, Geosciences
- PhD defense, Capucine Legentil, , Université de Lorraine, January 2023, examiner, Geosciences
- Habilitation defense, Emmanuel Franck, Université de Strasbourg, January 2023, examiner, Mathematics
- PhD defense, Zakaria Kassali, , Université Côte d’Azur, January 2023, reviewer, Mathematics
- PhD defense, Jean-François Fritsch, Institut Polytechnique de Paris, June 2023, examiner, Mathematics
- PhD defense, Sofiane Benzait, Institut Polytechinque de Paris, September 2023, reviewer, Mathematics
- PhD defense, Marine Deheuvels, Université de Pau et des Pays de l'Adour, October 2023, examiner, Geosciences
- PhD defense, Matthieu Gerest, Sorbonne Université, November 2023, examiner, Informatics
- PhD defense, Sarah Perez, Université de Pau et des Pays de l'Adour, November 2023, examiner, Mathematics
- PhD defense, Morgane Steins, December 2023, reviewer, Mathematics
Juliette Chabassier was part of the PhD jury of Abbas Mousavi: “Computational analysis and design optimization for acoustic devices”. Umeå University
11.3 Popularization
11.3.1 Internal or external Inria responsibilities
- Sébastien Tordeux is member of the organizing commitee of the Cercle "Mathématique et Informatique" Sofia Kovaleskaïa
11.3.2 Articles and contents
11.3.3 Interventions
Juliette Chabassier participated to the « Bureau des Enquêtes » science outreach event organized by Cap Science on June 6th 2023.
12 Scientific production
12.1 Major publications
- 1 articleLocal strategies for improving the conditioning of the plane-wave Ultra-Weak Variational Formulation.Journal of Computational Physics441September 2021, 110449HALDOI
- 2 articlePolynomial-reproducing spline spaces from fine zonotopal tilings.Journal of Computational and Applied Mathematics402March 2022, 113812HALDOI
- 3 articleImplementation of Hybridizable Discontinuous Galerkin method for time-harmonic anisotropic poroelasticity in two dimensions..International Journal for Numerical Methods in Engineering2021HAL
- 4 articleOutgoing modal solutions for Galbrun's equation in helioseismology.Journal of Differential Equations286June 2021, 494-530HALDOI
- 5 articleConstruction and convergence analysis of conservative second order local time discretisation for linear wave equations.ESAIM: Mathematical Modelling and Numerical Analysis554July 2021, 1507-1543HALDOI
- 6 articleFull waveform inversion for bore reconstruction of woodwind-like instruments.Acta AcusticaNovember 2021HALDOI
- 7 articlePractical unstructured splines: Algorithms, multi-patch spline spaces, and some applications to numerical analysis.Journal of Computational Physics471December 2022, 111625HALDOI
- 8 thesisUnstructured Isogeometric Analysis with Applications to Seismic Wave Propagation.Université de Pau et des Pays de l'AdourDecember 2021HAL
- 9 articleEfficient hybrid method for the modal analysis of optical microcavities and nanoresonators.Journal of the Optical Society of America. A Optics, Image Science, and Vision388July 2021, 1224HALDOI
- 10 articleDissipative time-domain one-dimensional model for viscothermal acoustic propagation in wind instruments..Journal of the Acoustical Society of America1502August 2021, 1165-1175HALDOI
12.2 Publications of the year
International journals
Invited conferences
International peer-reviewed conferences
Conferences without proceedings
Reports & preprints
Other scientific publications
12.3 Cited publications
- 50 articleInverse problem for the Helmholtz equation with Cauchy data: reconstruction with conditional well-posedness driven iterative regularization.ESAIM: Mathematical Modelling and Numerical Analysis533May 2019, 1005-1030HALDOIback to text
- 51 articleThe mechanism producing initial transients on the clarinet.The Journal of the Acoustical Society of America14262017, 3376-3386back to text
- 52 articleQuasistatic nonlinear characteristics of double-reed instruments.The Journal of the Acoustical Society of America12112007, 536-546back to text
- 53 articleAbout the stability of the inverse problem in 1-D wave equations—Application to the interpretation of seismic profiles.Applied Mathematics and Optimization511979, 1--47back to text
- 54 articleSpace-time Trefftz-DG approximation for elasto-acoustics.Applicable Analysis00August 2018, 1 - 16HALback to text
- 55 articleStability analysis of heterogeneous Helmholtz problems and finite element solution based on propagation media approximation.Mathematics of Computation863072017, 2129 - 2157HALDOIback to text
- 56 articleDirect Simulation of Reed Wind Instruments.Computer Music Journal3342009, 43-55back to textback to text
- 57 articleHybridizable discontinuous Galerkin method for the two-dimensional frequency-domain elastic wave equations.Geophysical Journal International2131April 2018, 637--659HALDOIback to text
- 58 inproceedingsIdentification of functional parameters in partial differential equations.Joint Automatic Control Conference121974, 155--156back to text
- 59 articleHelioseismology.Reviews of Modern Physics7442002, 1073back to text
- 60 articleImproved precision in measurements of acoustic impedance spectra using resonance-free calibration loads and controlled error distribution.The Journal of the Acoustical Society of America12132007, 1471-1481back to text
- 61 articleTransfer matrix of a truncated cone with viscothermal losses: application of the WKB method.Acta Acustica42May 2020HALDOIback to text
- 62 articleWoodwind instrument design optimization based on impedance characteristics with geometric constraints.Journal of the Acoustical Society of America1485November 2020, 2864-2877HALDOIback to text
- 63 phdthesisContributions to Seismic Full Waveform Inversion for Time Harmonic Wave Equations: Stability Estimates, Convergence Analysis, Numerical Experiments involving Large Scale Optimization Algorithms.Université de Pau et des Pays de l'AdourNovember 2017HALback to text
- 64 articleSensitivity kernels for time-distance helioseismology-Efficient computation for spherically symmetric solar models.Astronomy & Astrophysics6162018, A156back to text
- 65 articleAcoustical impedance measurements by the two‐microphone‐three‐calibration (TMTC) method.The Journal of the Acoustical Society of America8861990, 2533-2545back to text
- 66 articleComputational helioseismology in the frequency domain: acoustic waves in axisymmetric solar models with flows.Astronomy and Astrophysics - A&A600April 2017, A35HALDOIback to text
- 67 articleLocal helioseismology.Living Reviews in Solar Physics212005, 6back to text
- 68 articleLocal helioseismology: three-dimensional imaging of the solar interior.Annual Review of Astronomy and Astrophysics482010, 289--338back to text
- 69 articleReal-time synthesis of clarinet-like instruments using digital impedance models.The Journal of the Acoustical Society of America11812005, 483-494back to text
- 70 inproceedingsSeismic imaging of supercritical geothermal reservoir using full-waveform inversion method.Proceedings2019back to text
- 71 article3-D seismic exploration across the deep geothermal research platform GroßSchönebeck north of Berlin/Germany.Geothermal Energy712019, 1--18back to text
- 72 inproceedingsThe seismic inverse problem as a sequence of before stack migrations.Conference on inverse scattering: theory and applicationSiam Philadelphia, PA1983, 206--220back to text
- 73 articleFast finite-difference time-domain modeling for marine-subsurface electromagnetic problems.Geophysics7222007, A19--A23back to text
- 74 articleHigh-order finite-difference simulations of marine CSEM surveys using a correspondence principle for wave and diffusion fields.Geophysics7512010, F33--F50back to text
- 75 articleA review of the adjoint-state method for computing the gradient of a functional with geophysical applications.Geophysical Journal International16722006, 495--503back to text
- 76 articleGauss--Newton and full Newton methods in frequency--space seismic waveform inversion.Geophysical Journal International13321998, 341--362back to text
- 77 articleTwo-dimensional velocity models from wide-angle seismic data by wavefield inversion.Geophysical Journal International12421996, 323--340back to text
- 78 articleINVERSE THEORY APPLIED TO MULTI-SOURCE CROSS-HOLE TOMOGRAPHY. PART 1: ACOUSTIC WAVE-EQUATION METHOD 1.Geophysical prospecting3831990, 287--310back to text
- 79 articleGoverning equations for the coupled electromagnetics and acoustics of porous media.Physical Review B50211994, 15678back to text
- 80 articleWebster's Horn Equation Revisited.SIAM Journal on Applied Mathematics6562005, 1981-2004back to text
- 81 articleProperty modelling of a potential CO2 storage site using seismic inversion.EGUGA2013, EGU2013--10470back to text
- 82 bookInverse problem theory and methods for model parameter estimation.89siam2005back to text
- 83 bookInverse problem theory: methods for data fitting and model parameter estimation.Amsterdam, NetherlandsElsevier Science Publishers1987back to text
- 84 articleDemonstrating storage of CO2 in geological reservoirs: The Sleipner and SACS projects.Energy299-102004, 1361--1369back to text
- 85 articleA comparison of a one-dimensional finite element method and the transfer matrix method for the computation of wind music instrument impedance.Acta Acustica united with Acustica52019, 838HALback to text
- 86 articleSound production in recorderlike instruments. II. A simulation model.The Journal of the Acoustical Society of America10151997, 2925-2939back to text
- 87 articleInfluence of fluid displacement patterns on seismic velocity during supercritical CO2 injection: Simulation study for evaluation of the relationship between seismic velocity and CO2 saturation.International Journal of Greenhouse Gas Control462016, 197--204back to text

