Section: New Results

Efficient and Accurate Spherical Kernel Integrals using Isotropic Decomposition

Participant : Cyril Soler [contact] .

Figure 4. Convergence of spherical ISD shading for increasing $L$. Top to bottom: isotropic alum-bronze (with pisa illumination), isotropic gold-paint and anisotropic yellow-satin (both using grace cathedral illumination). Reference images were ray-traced using 300K samples per pixel. False color error images in the bottom row visually illustrate the convergence of our RZH approximation to the reference rendering. Note that the dark region in the center of the spheres on the last row of renderings is indeed part of the underlying reflectance input data.

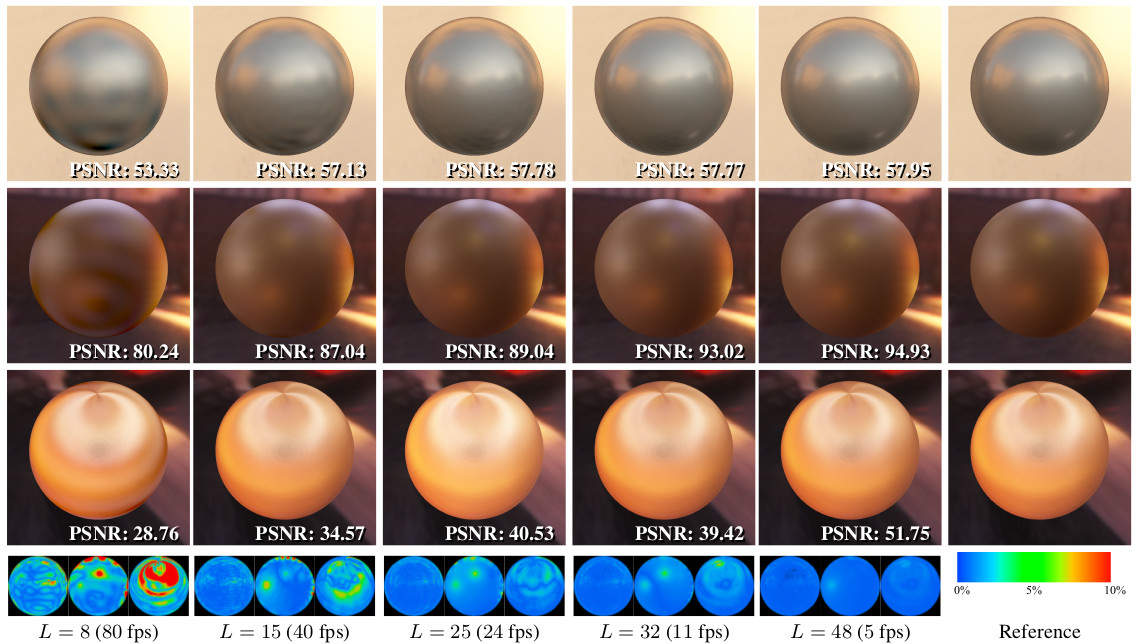
Spherical filtering is fundamental to many problems in image synthesis, such as computing the reflected light over a surface or anti-aliasing mirror reflections over a pixel. This operation is challenging since the profile of spherical filters (e.g., the view-evaluated BRDF or the geometry-warped pixel footprint, above) typically exhibits both spatial-and rotational-variation at each pixel, precluding precomputed solutions. We accelerate complex spherical filtering tasks using isotropic spherical decomposition (ISD), decomposing spherical filters into a linear combination of simpler isotropic kernels. Our general ISD is flexible to the choice of the isotropic kernels, and we demonstrate practical realizations of ISD on several problems in rendering: shading and prefiltering with spatially-varying BRDFs, anti-aliasing environment mapped mirror reflections, and filtering of noisy reflectance data. Compared to previous basis-space rendering solutions, our shading solution generates ground truth-quality results at interactive rates, avoiding costly reconstruction and large approximation errors. This paper was published in ACM Transactions on Graphics [4] and presented at Siggraph Asia 2015.