Section: New Results

A Hierarchical Approach for Regular Centroidal Voronoi Tessellations

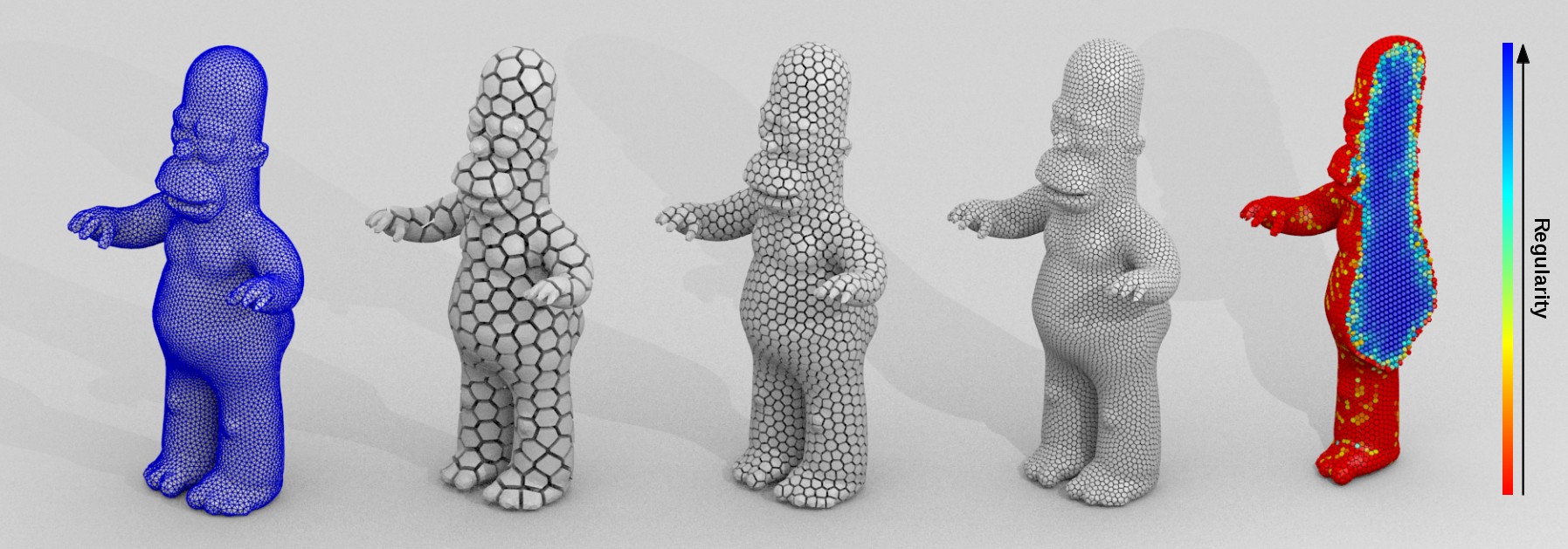
In this paper we consider Centroidal Voronoi Tessellations (CVTs) and study their regularity. CVTs are geometric structures that enable regular tessellations of geometric objects and are widely used in shape modeling and analysis. While several efficient iterative schemes, with defined local convergence properties, have been proposed to compute CVTs, little attention has been paid to the evaluation of the resulting cell decompositions. In this paper, we propose a regularity criterion that allows us to evaluate and compare CVTs independently of their sizes and of their cell numbers. This criterion allows us to compare CVTs on a common basis. It builds on earlier theoretical work showing that second moments of cells converge to a lower bound when optimising CVTs. In addition to proposing a regularity criterion, this paper also considers computational strategies to determine regular CVTs. We introduce a hierarchical framework that propagates regularity over decomposition levels and hence provides CVTs with provably better regularities than existing methods. We illustrate these principles with a wide range of experiments on synthetic and real models.

This work was published as a journal article in Computer Graphics Forum [7] .

Figure 8. Hierarchical computation of a centroidal Voronoi tessellation from a 3D mesh [7] . Inside cells are very regular.