Section: New Results

Matching problems and their applications

Participants : Laurent Baratchart, Martine Olivi, Gibin Bose, David Martinez Martinez, Fabien Seyfert.

Multiplexer synthesis via interpolation and common junction design

In the context of David Martinez Martinez's PhD funded partly by CNES the synthesis of multiplexer responses was considered using multipoint matching techniques. Indeed, synthesizing the response of multiplexer composed of a set of channel filters connected via common manifold junction to a common port can be seen as a matrix version application of our multipoint matching result for filters [7]. For short a simultaneous matching solutions is sought for, where each channel filter matches the load it is connected at specified matching frequencies. The difficulty here is that the load seen by each filter, depends explicitly of the response of the other filters by means of the common junction's response: the multiplexer synthesis problem is therefore, in general, strictly harder than the filter multipoint matching problem, and can't be solved by a sequential solving of independent «scalar» problems. A notable exception to this statement is obtained when a totally decoupling common junction is considered. This somehow artificial situation was taken as a start of a continuation algorithm, during which the decoupling junction response is moved step by step via a linear trajectory towards the target junction while the simultaneous matching problem is solved all along via a differential predictor corrector method. Whereas all «accidents» of branch point type that can occur during this procedure are not classified yet, one major obstruction to the continuation process is the occurrence of manifold peaks. The latter are due to resonances occurring in the manifold junction and yield total reflection at some frequencies of the channel ports. When latter coincide with the matching frequencies of a particular channel filter, the simultaneous matching problem has no solution, and the continuation algorithm fails irredeemably.

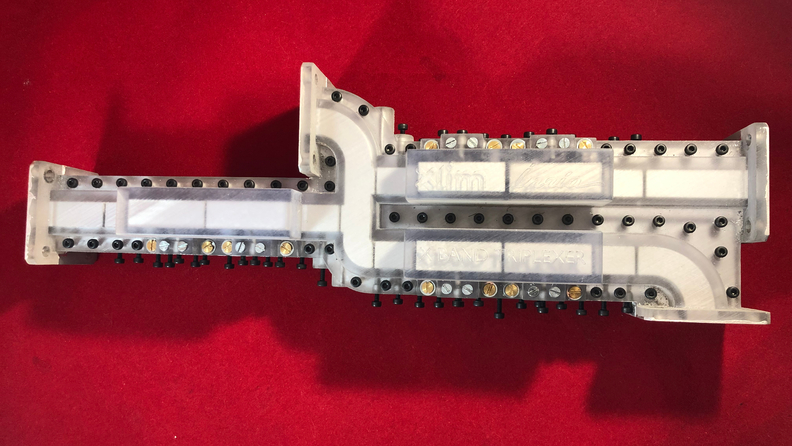
We therefore gave a full characterization of this manifold peaks and designed a heuristic approach to avoid their appearance during the continuation process. We showed that they only depend on the out of band response of the channel filters, and can in first approximation be considered as constant along the continuation process and estimated by a full wave simulation of each channel filter. This is then used within a triangular adjustment procedure that looks for possible manifold length adjustments (within the channel filters, and between that channel filters and the manifold junction) that guaranties the absence of manifold peaks within the band of each channel filter. Details of this procedure that give important information to the designer about the feasibility of an effective multiplexer response by means of given manifold T-junction, and this before any channel filter optimization procedure, are detailed in [23], [18] and were presented at Eumc 2019. In connection with the previously described continuation procedure, it was used to design a compact triplexer, based on frequency specifications considered as «hard to fulfill» and furnished by CNES. The triplexer was then realized using 3D printing techniques at Xlim (S. Bila and O. Tantot) our long standing academical partners on these topics (see Figure 8). This work is part of the PhD thesis [14] defended by David Martinez Martinez at the end of June.

Uniform matching and global optimality considerations: application to a reference tracking problem

This problem was proposed by Pauline Kergus, PhD student at Onera (Toulouse). In her PhD, she studied the following data driven problem: given frequency measurements of a plant, find a controller which allows to follow a given reference model. The approach she proposed was to directly identify the controller from frequency measurements induced on the controller by the closed loop. Of course the quality of the controller, and in particular its stability, highly depend on the chosen reference model. The question is thus: how to choose a good reference model $M$ with a minimum of information on the plant? The reference model is linked to the sensitivity function $S$ by the relation $M+S=1$. The sensitivity function is an important design tool in control. To ensure closed loop stability, it should be stable and satisfy some interpolation conditions at the unstable poles and zeros of the plant [28]. Its shape reflect the performances of the closed loop: $S$ should be small at low frequencies to ensure a good tracking accuracy, as well as disturbance rejection; while to ensure noise rejection, $S$ should go to 1 at infinity (the reference model $1-S$ should be small at high frequencies). The shaping problem for the sensitivity function can be stated in a manner almost similar to the matching problem described below: find a Schur function with minimum infinite norm in a frequency band $[0,w_c]$, where $w_c$ is the chosen cutoff frequency, while satisfying some interpolatory constraints. The main difference that prevents for using the convex relaxation method proposed below is the condition at infinity. An alternative optimization method is under study. To get a non-optimal solution to the problem, Pauline Kergus proposed a simple way to enforce the interpolation conditions from a given well-shaped reference model. To compute the unstable poles and zeros of the plant, which is the minimal required information, she uses our software PISA https://project.inria.fr/pisa/working/project/. Examples illustrating the advantages and limitations of the method were studied. The results were reported in the journal paper [17] and presented at the CDC 2019 in Nice.