Section: New Results

Corotated meshless implicit dynamics for deformable bodies

Participants : Jean-Nicolas Brunet, Vincent Magnoux, Benoît Ozell, Stéphane Cotin.

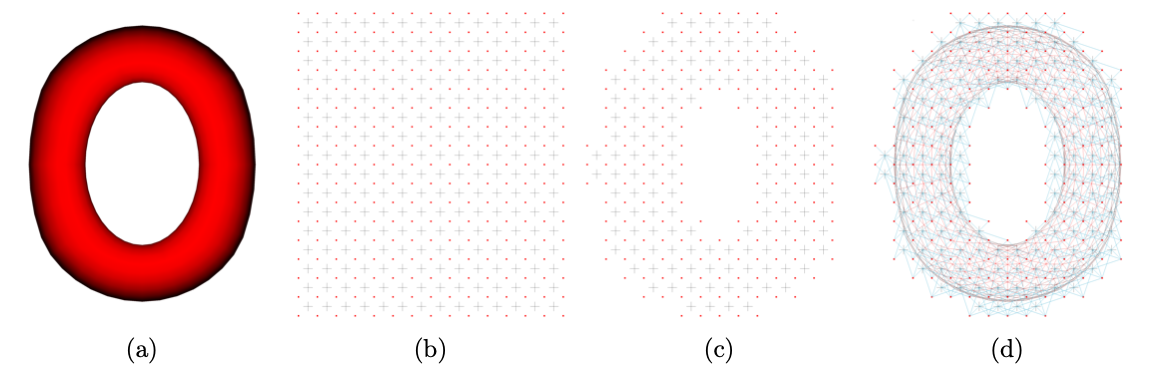
We proposed a fast, stable and accurate meshless method to simulate geometrically non-linear elastic behaviors. To address the inherent limitations of finite element (FE) models, the discretization of the domain is simplified by removing the need to create polyhedral elements. The volumetric locking effect exhibited by incompressible materials in some linear FE models is also completely avoided. Our approach merely requires that the volume of the object be filled with a cloud of points (see figure 7). To minimize numerical errors, we constructed a corotational formulation around the quadrature positions that is well suited for large displacements containing small deformations. The equations of motion was integrated in time following an implicit scheme. The convergence rate and accuracy were validated through both stretching and bending case studies. Finally, results were presented using a set of examples that show how we can easily build a realistic physical model of various deformable bodies with little effort spent on the discretization of the domain. We presented our work at WSCG 2019 [21]. (Fig. 7).

Figure 7. Volumetric discretizations of a 3D surface. (a) Surface mesh provided by the user. (b) Background grid where the grid's cubes are used to place the DOFs(degrees of freedom) and the integration points. (c) DOFs and integration points are cropped to fit the surface mesh. (d) A neighborhood of the closest particles is built around each integration point.